On this page you will find:
1. Meridional structures of the Hough functions for the realistic background state of the mean zonal wind and for the background state at rest,
2. Horizontal structures of the Hough harmonics (Kelvin wave, mixed Rossby-gravity wave, Rossby and inertia-gravity waves) for different equivalent depths.
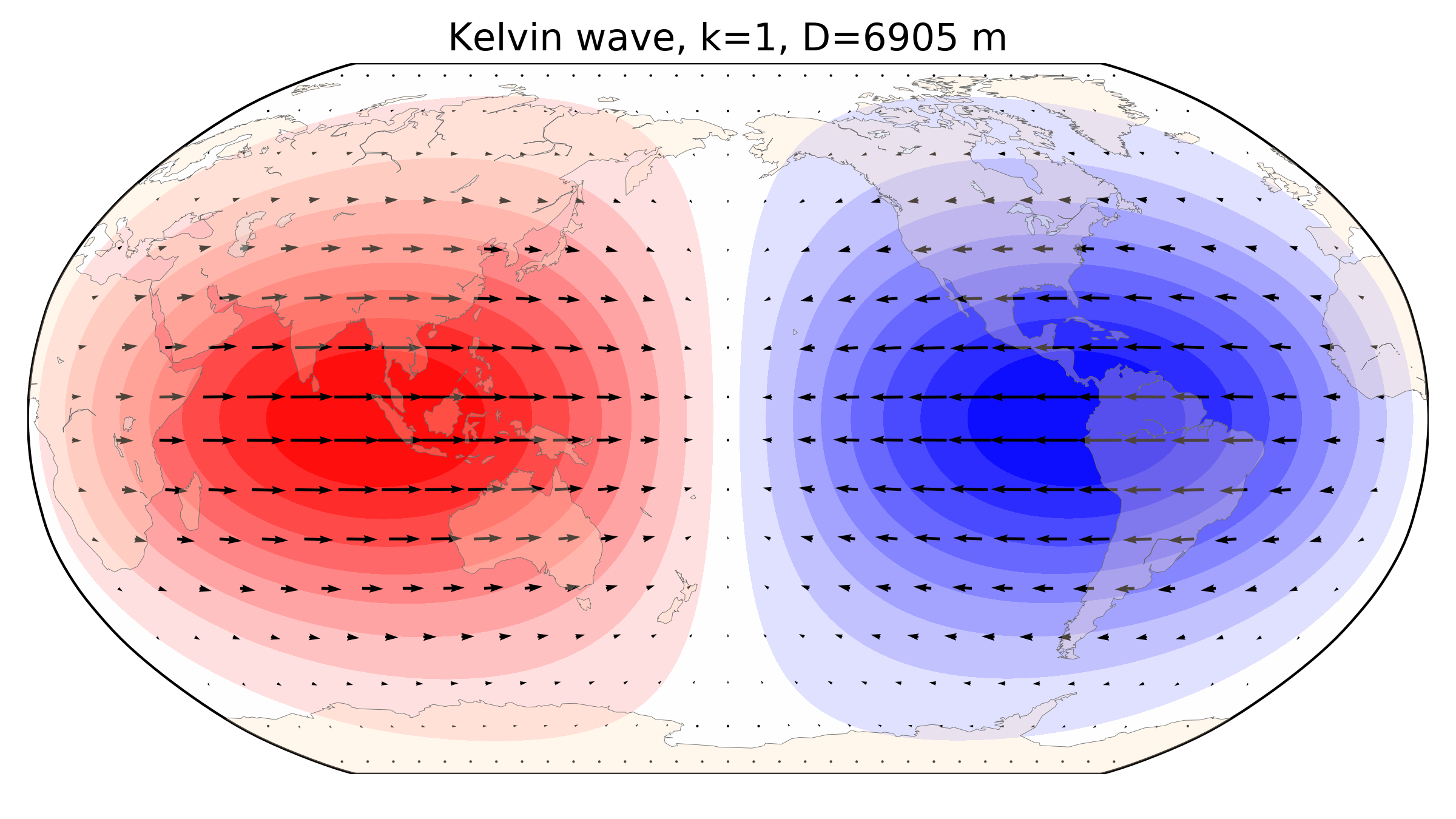
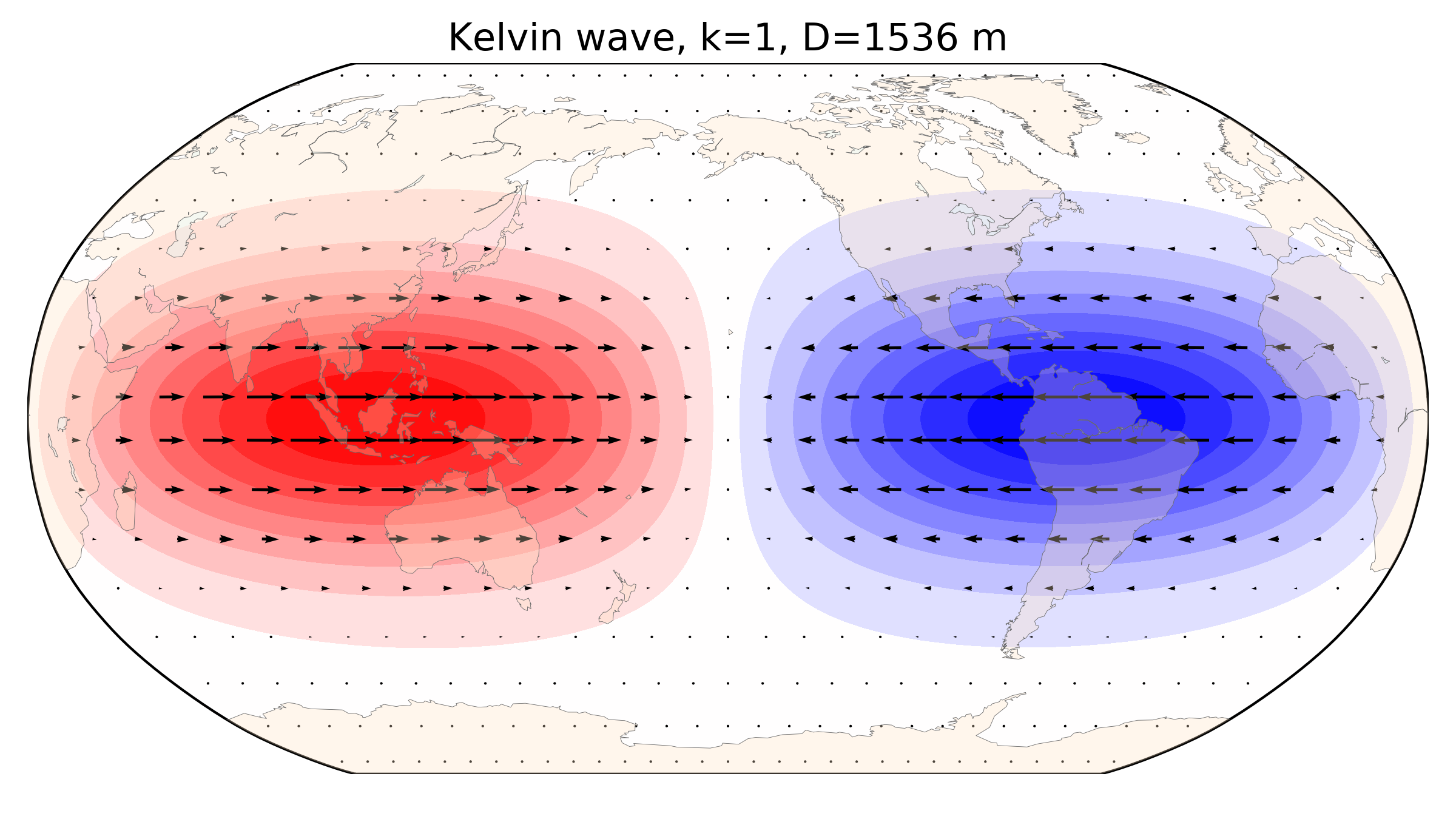
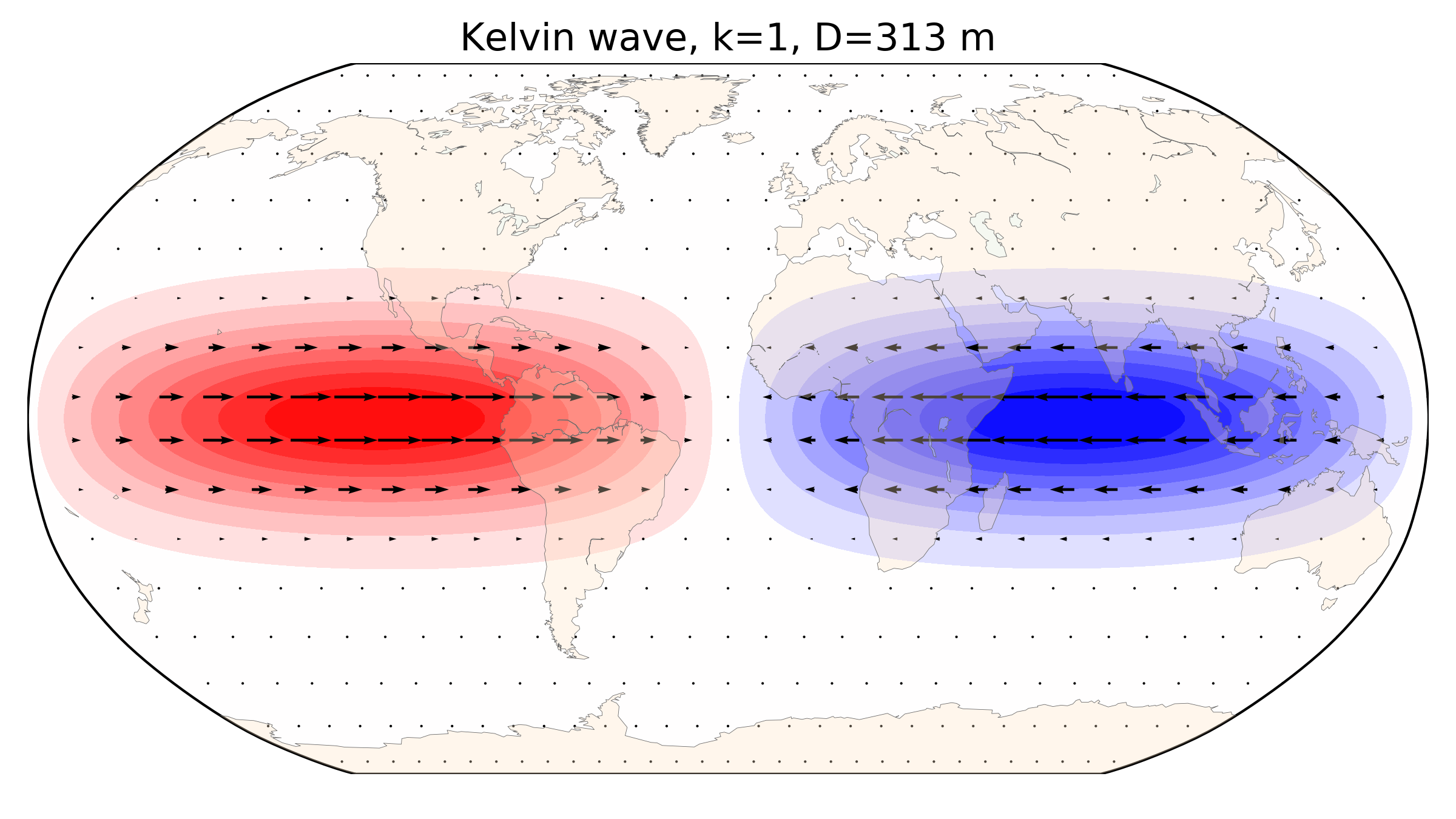
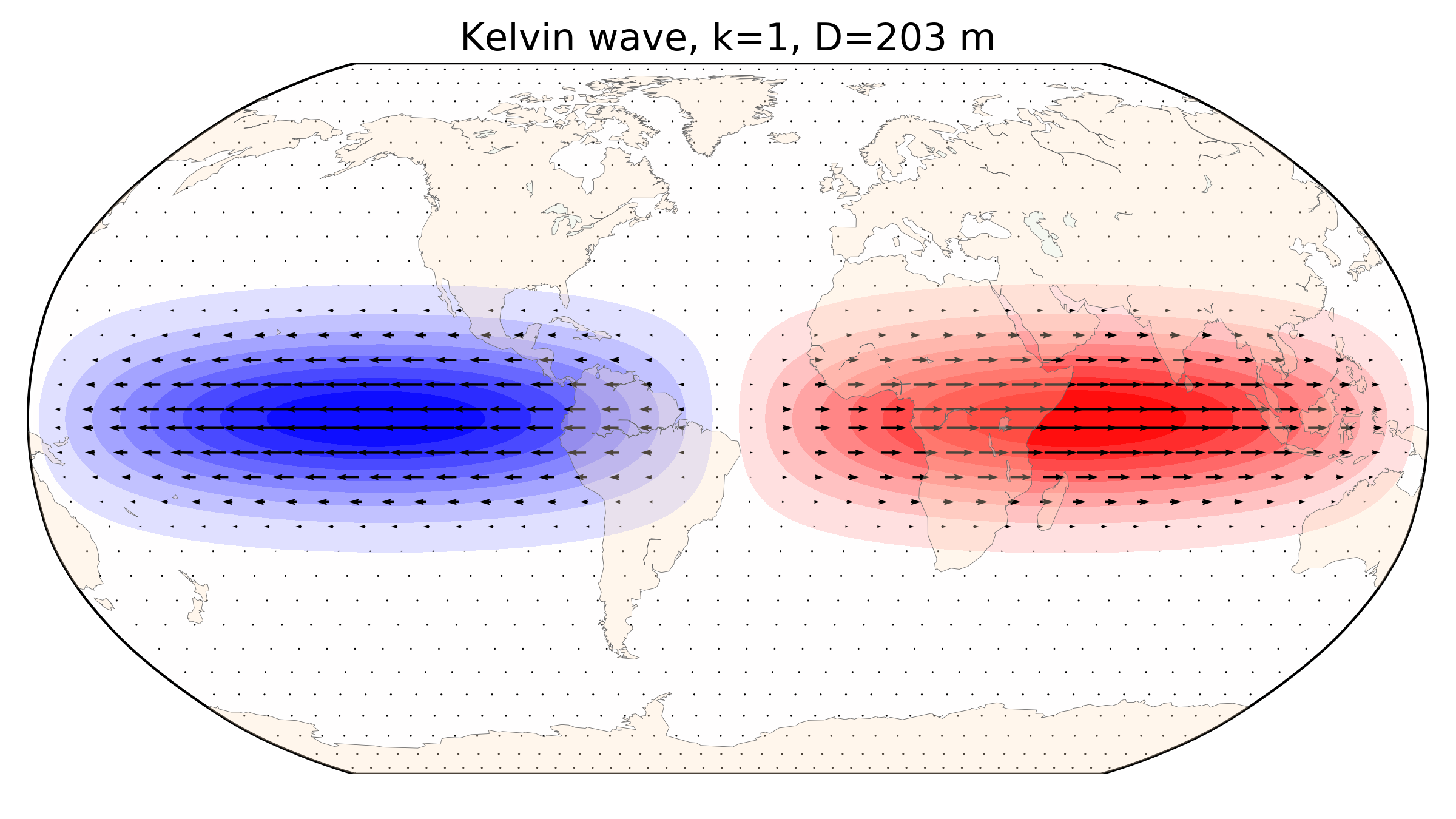
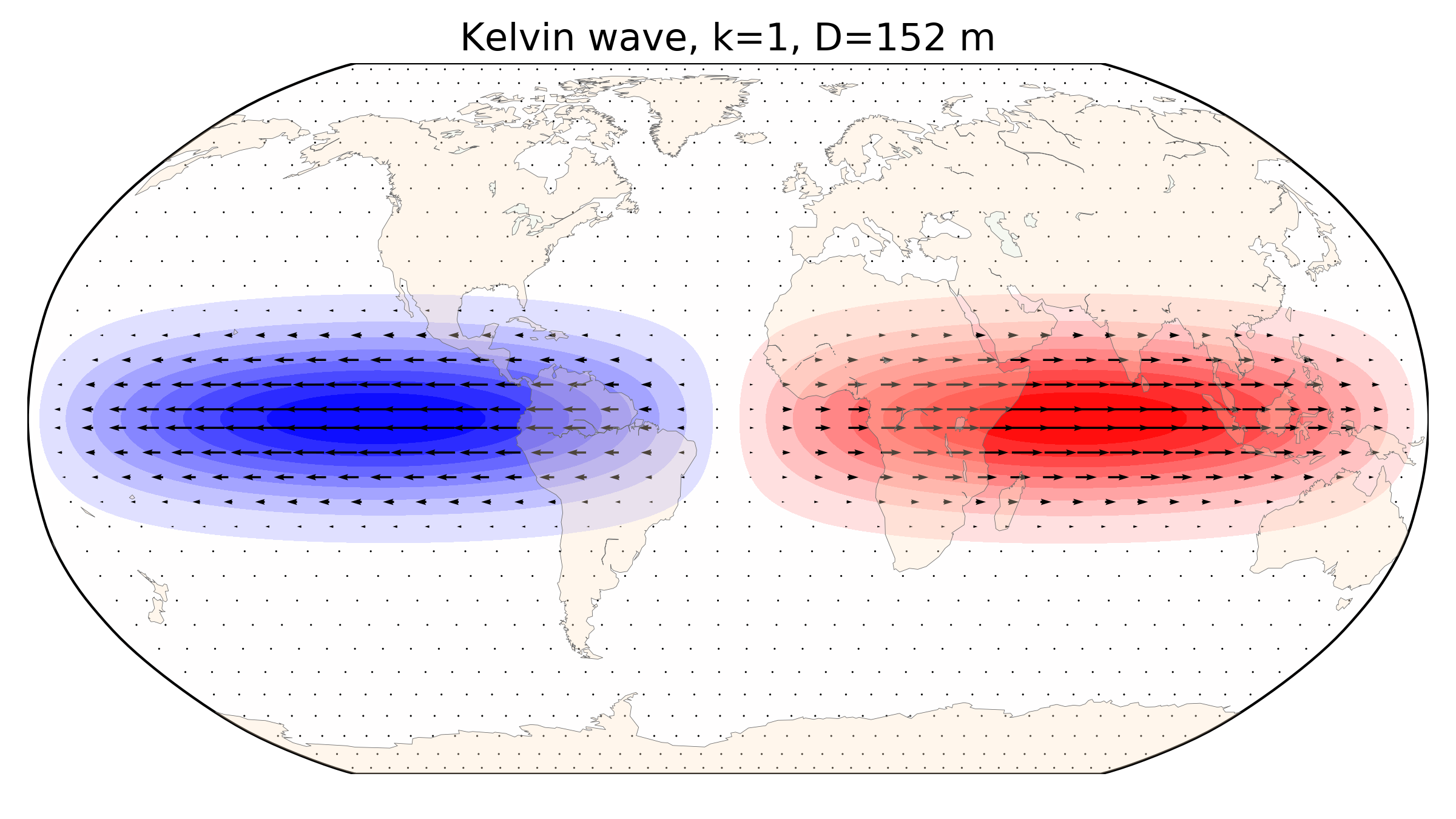
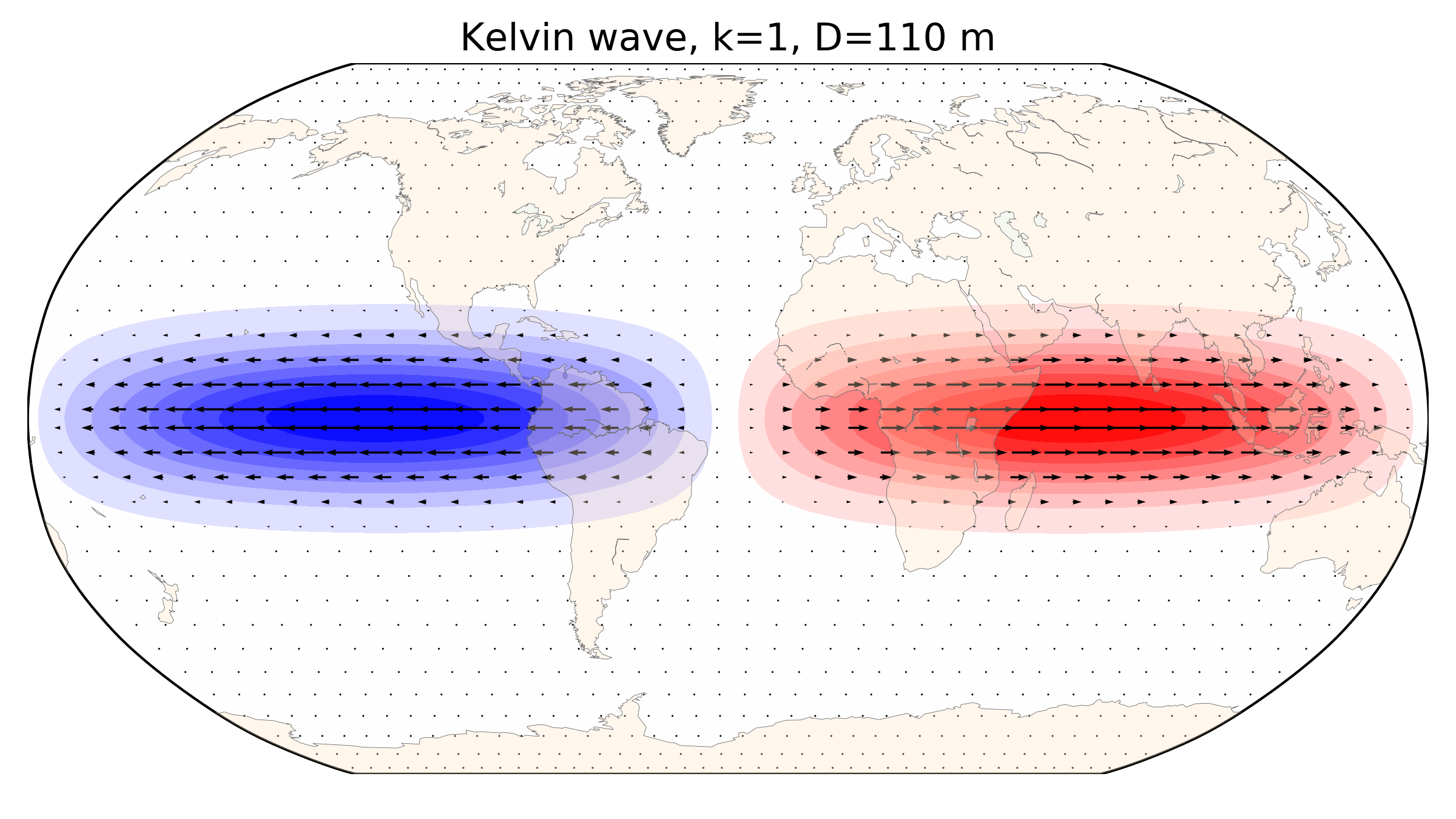
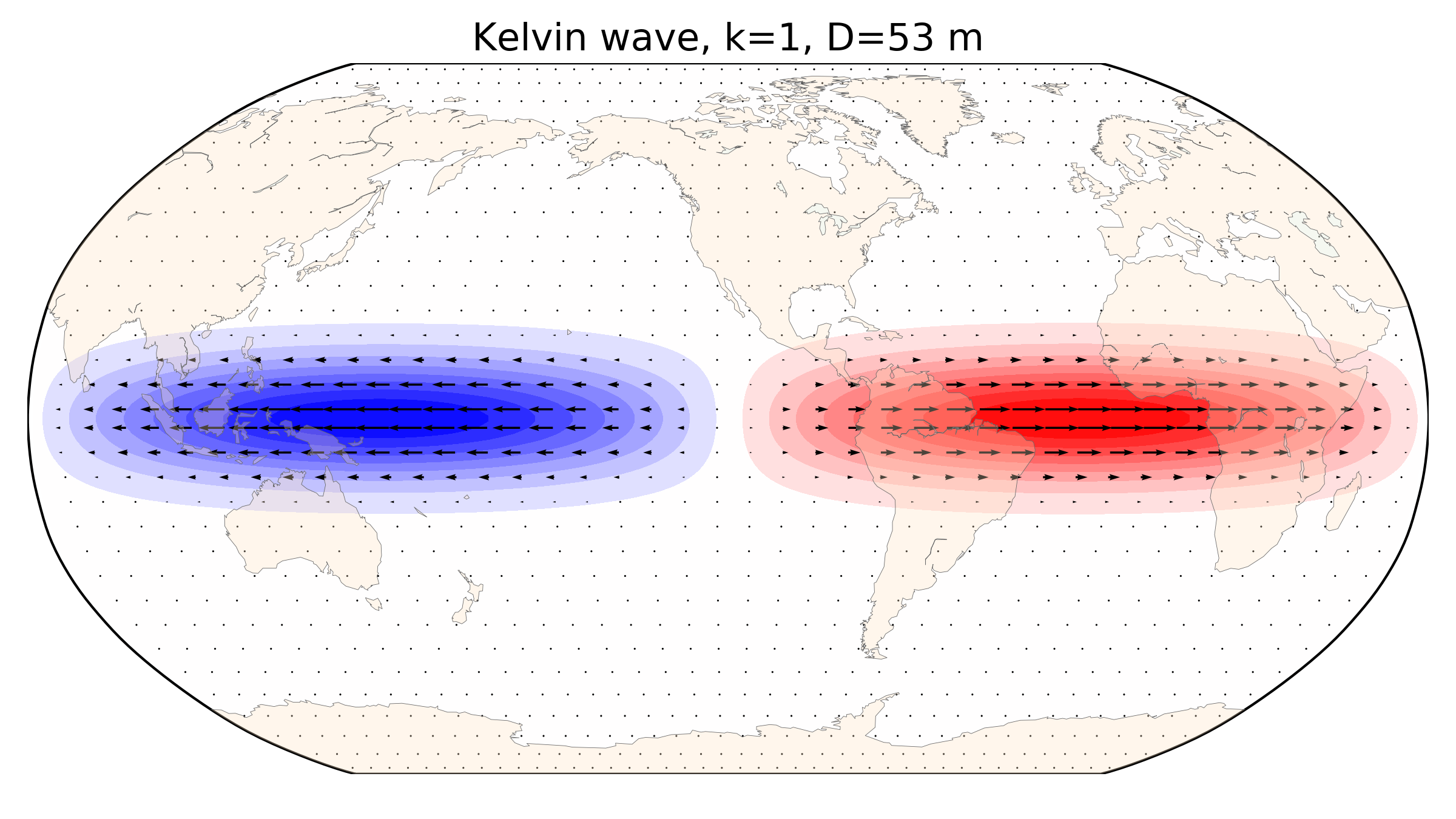
(a) Kelvin Waves
Horizontal structure of the n=0 eastward-propagating inertia-gravity mode on the sphere, Kelvin wave.
Geopotential height is scaled by the maximal height. Colours vary between -1 and +1 with positive/negative perturbations in red/blue.
Wind components are scaled by the maximal wind speed. Kelvin wave is the slowest eastward-propagating eigensolution of the linearized primitive equations on the sphere, associated with a large part of atmosphere and ocean variability in the tropics. Together with the MRG wave, Kelvin wave fills the frequency gap between the Rossby and inertia-gravity modes in the tropics. In the case of linearized equations on the equatorial beta-plane, the Kelvin wave is sometimes denoted n=-1 eastward-propagating inertia-gravity mode.
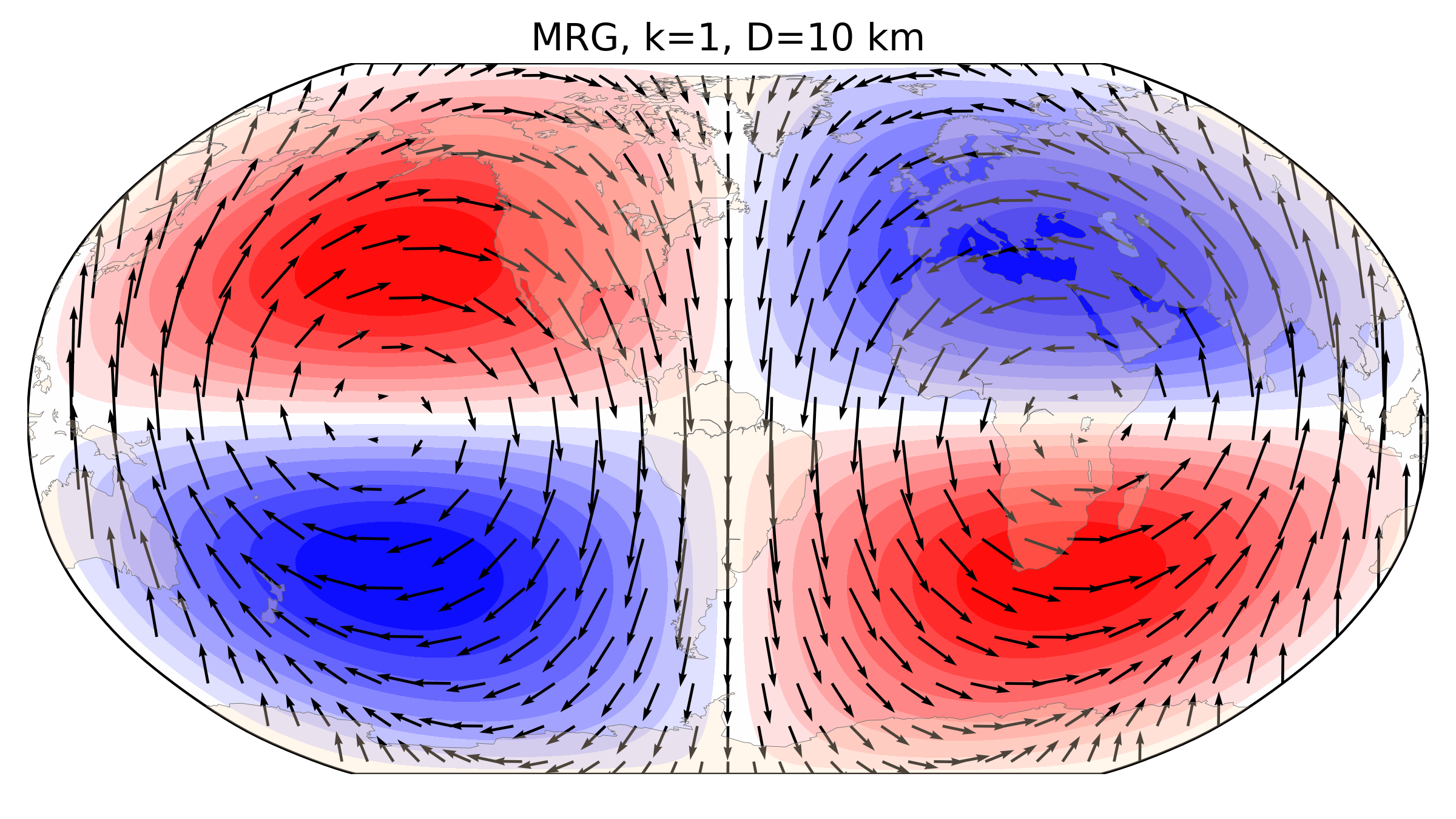
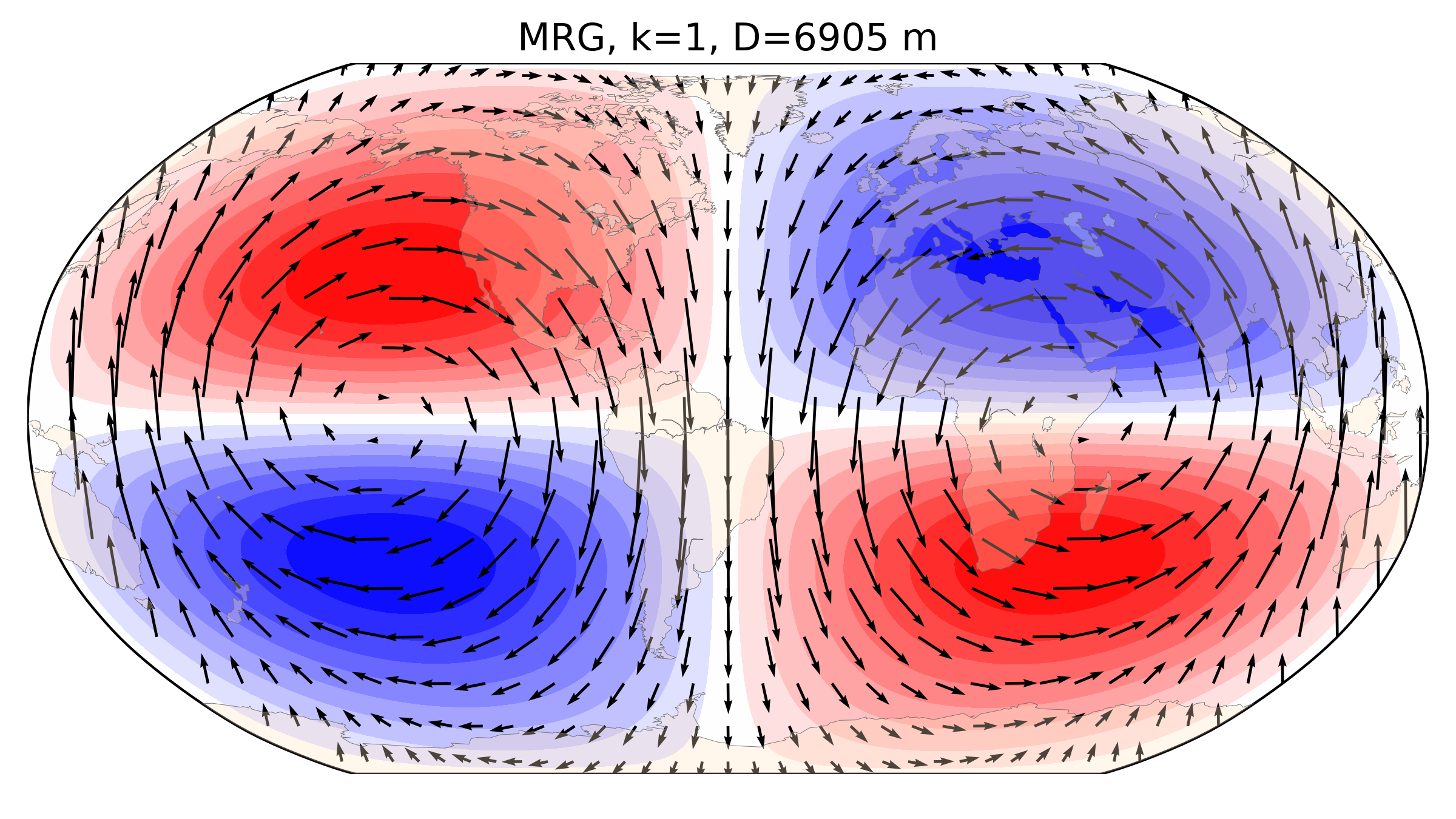
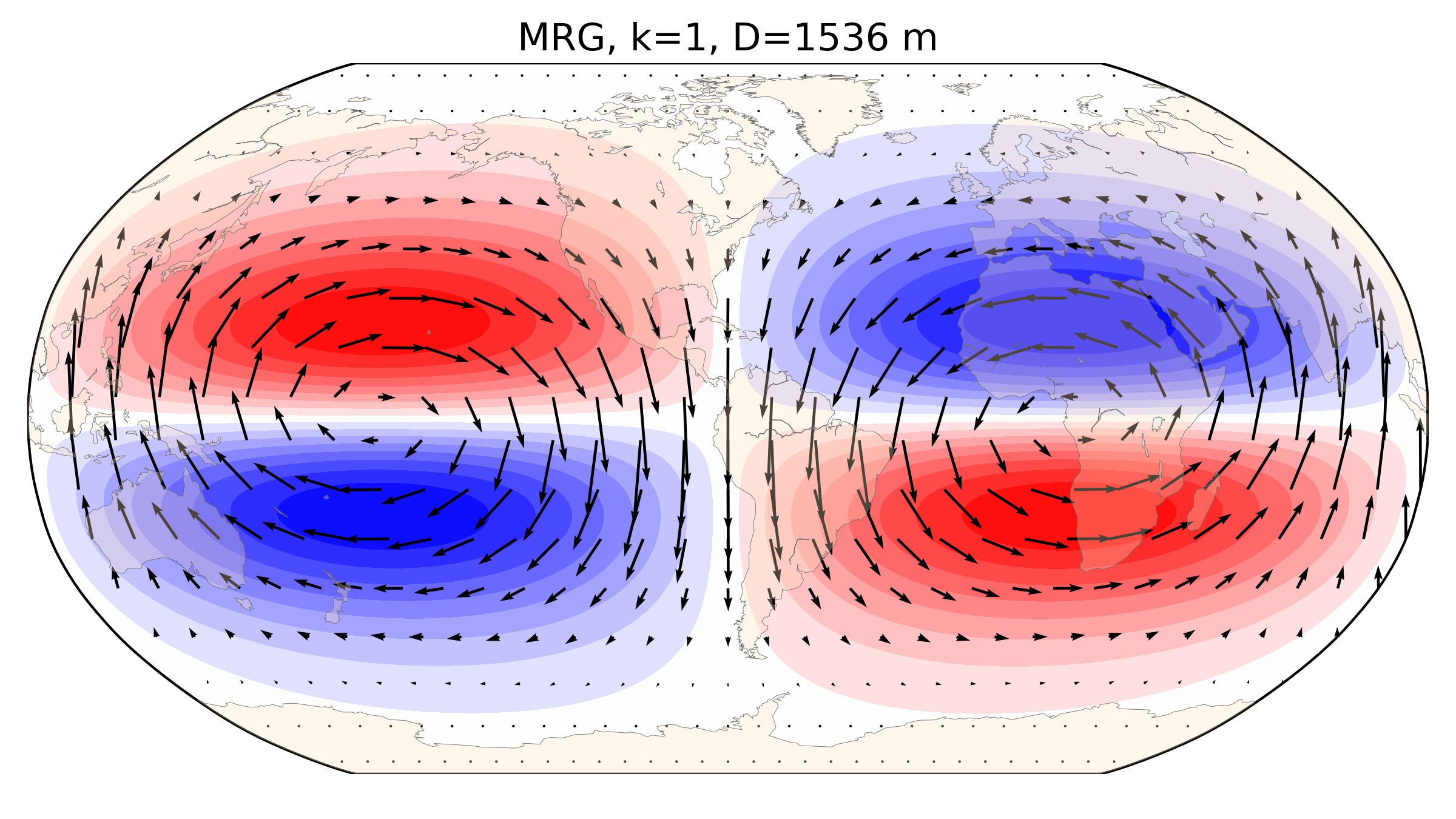
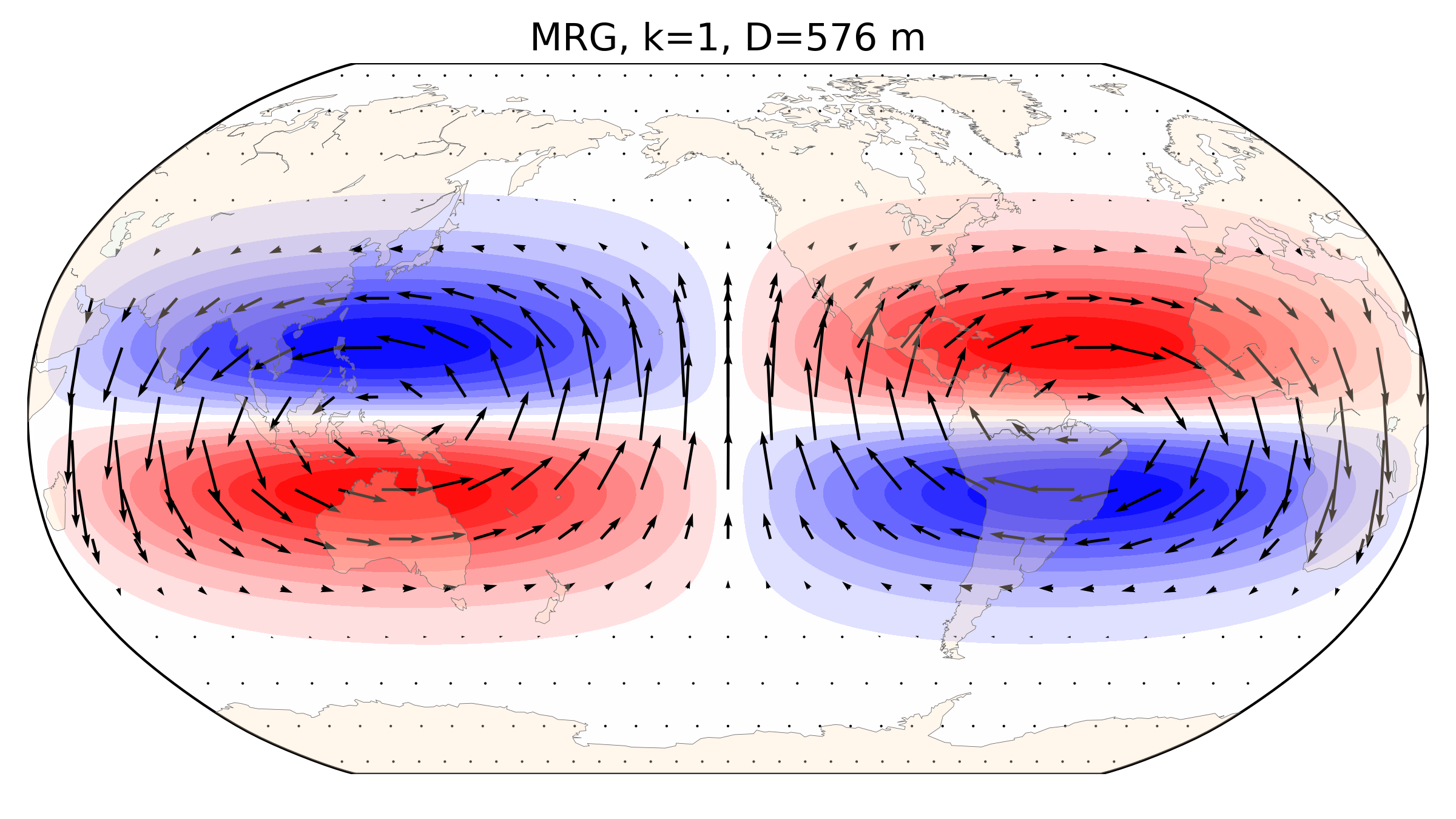
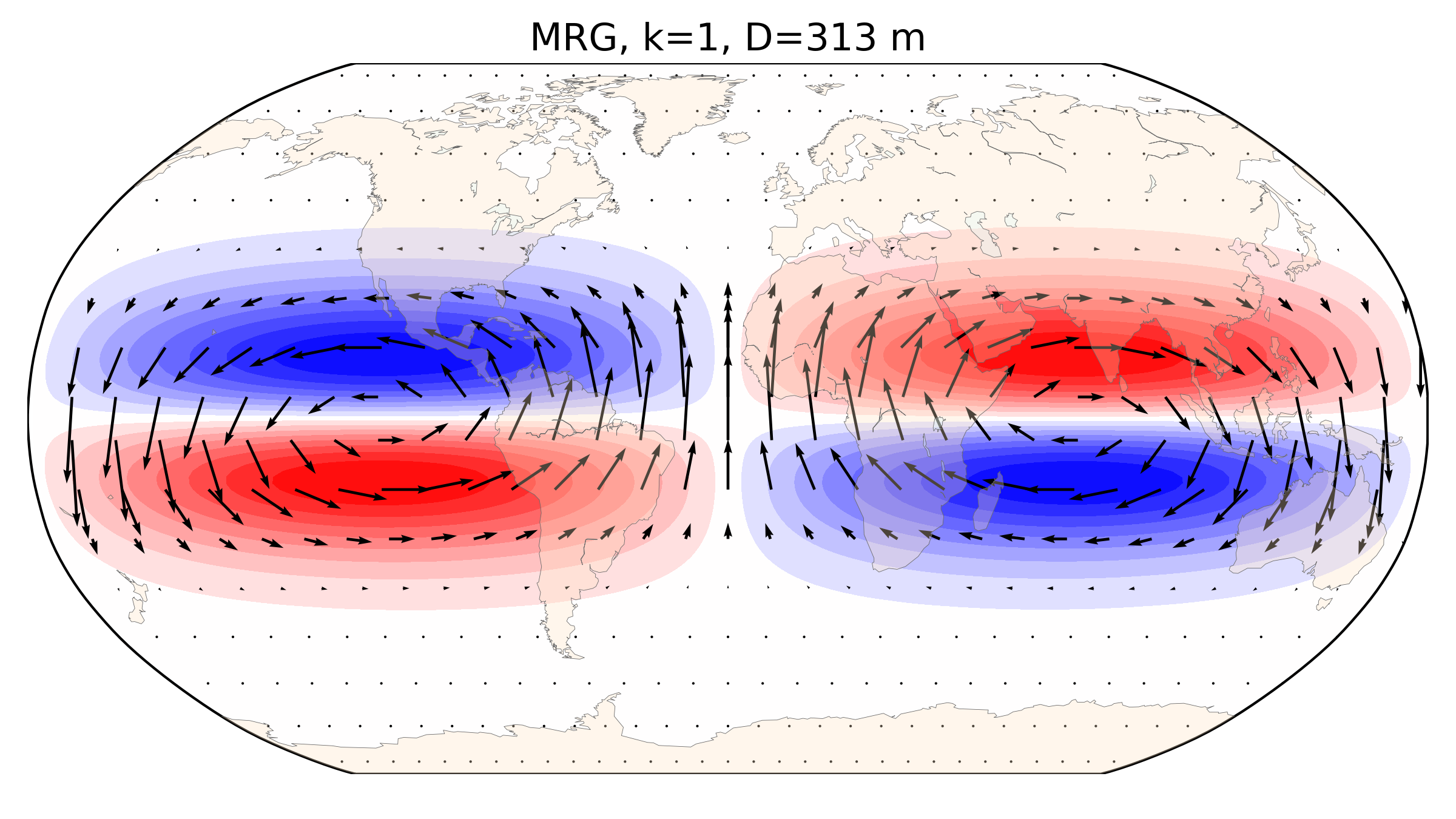
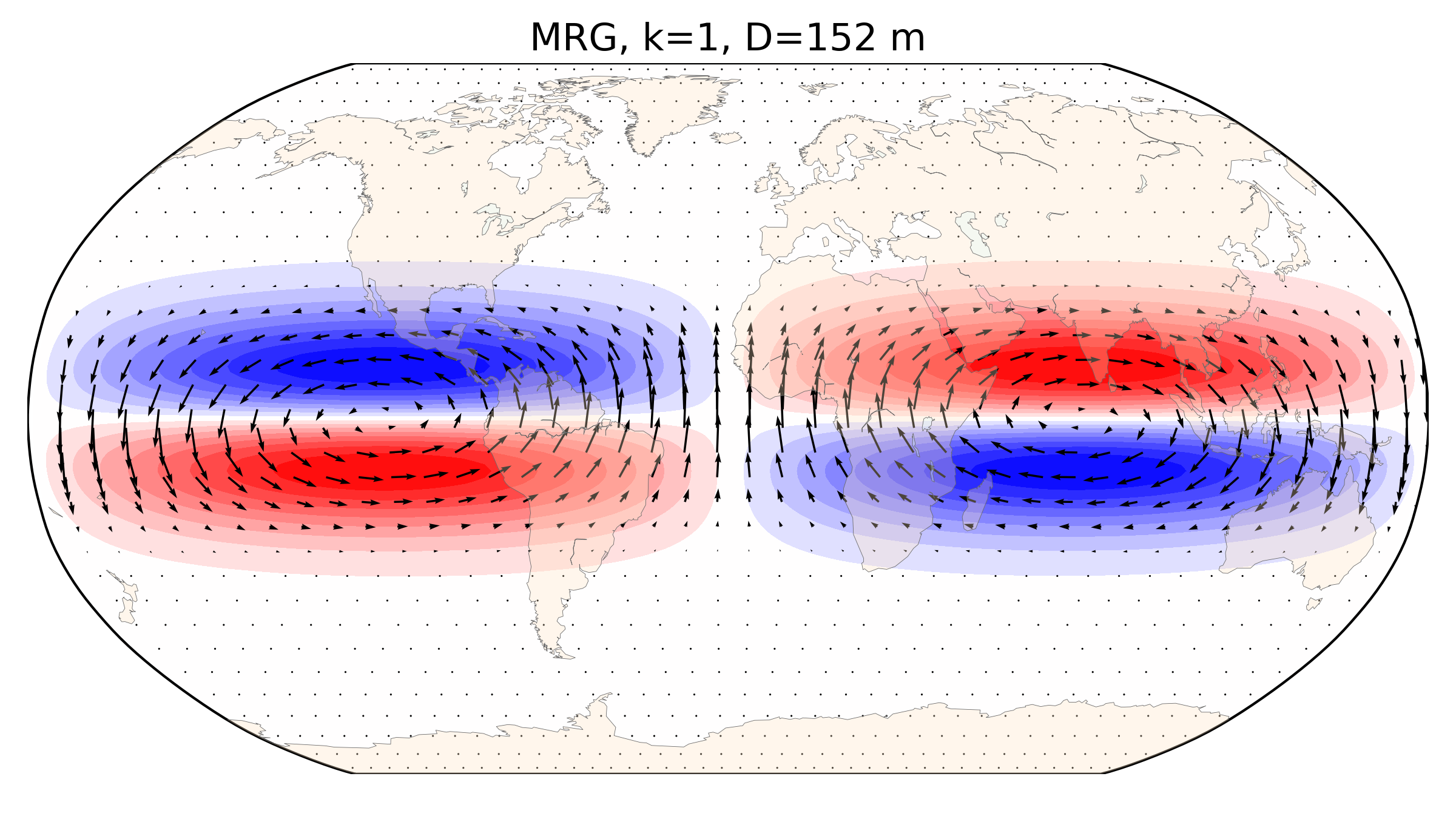
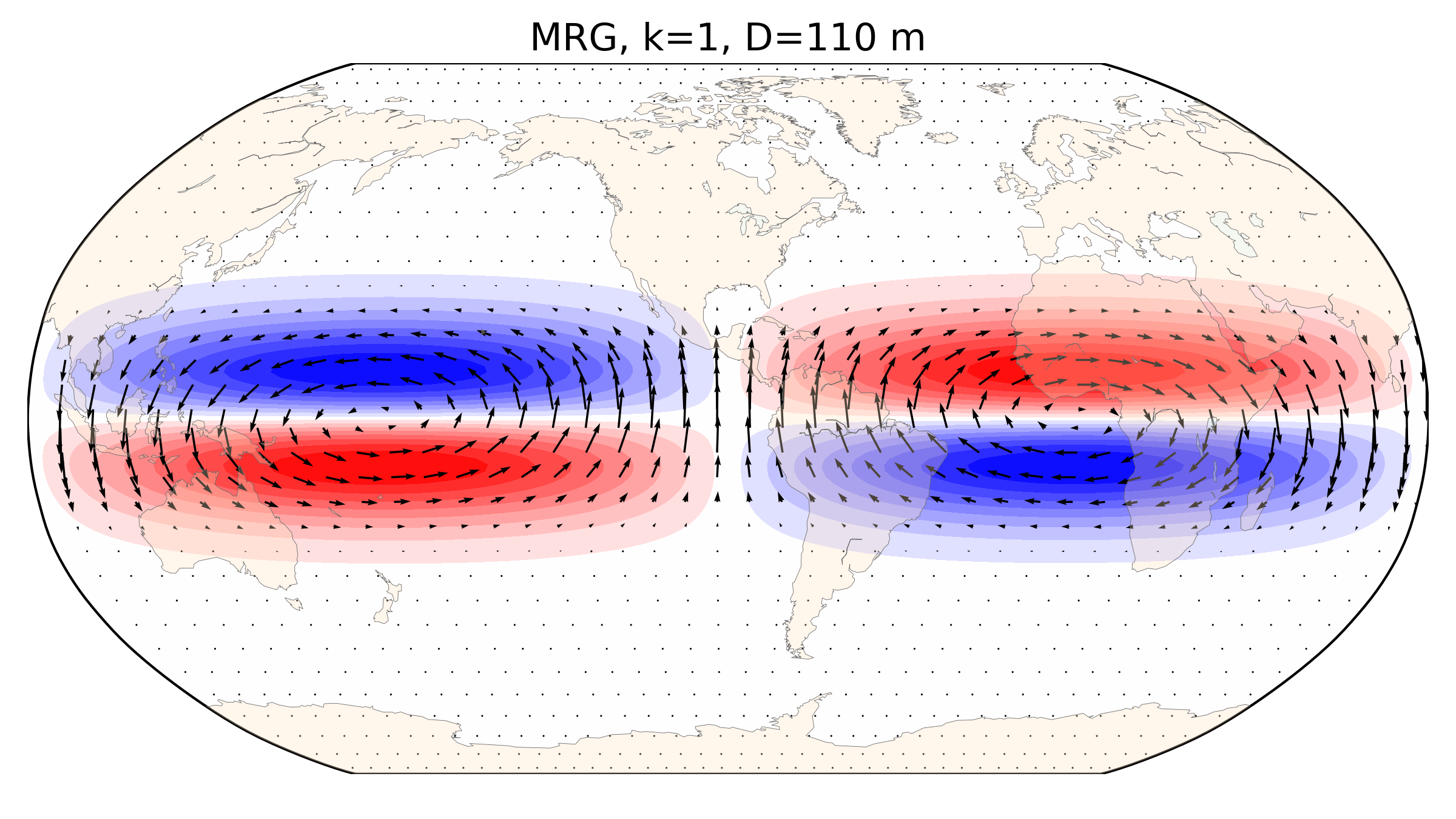
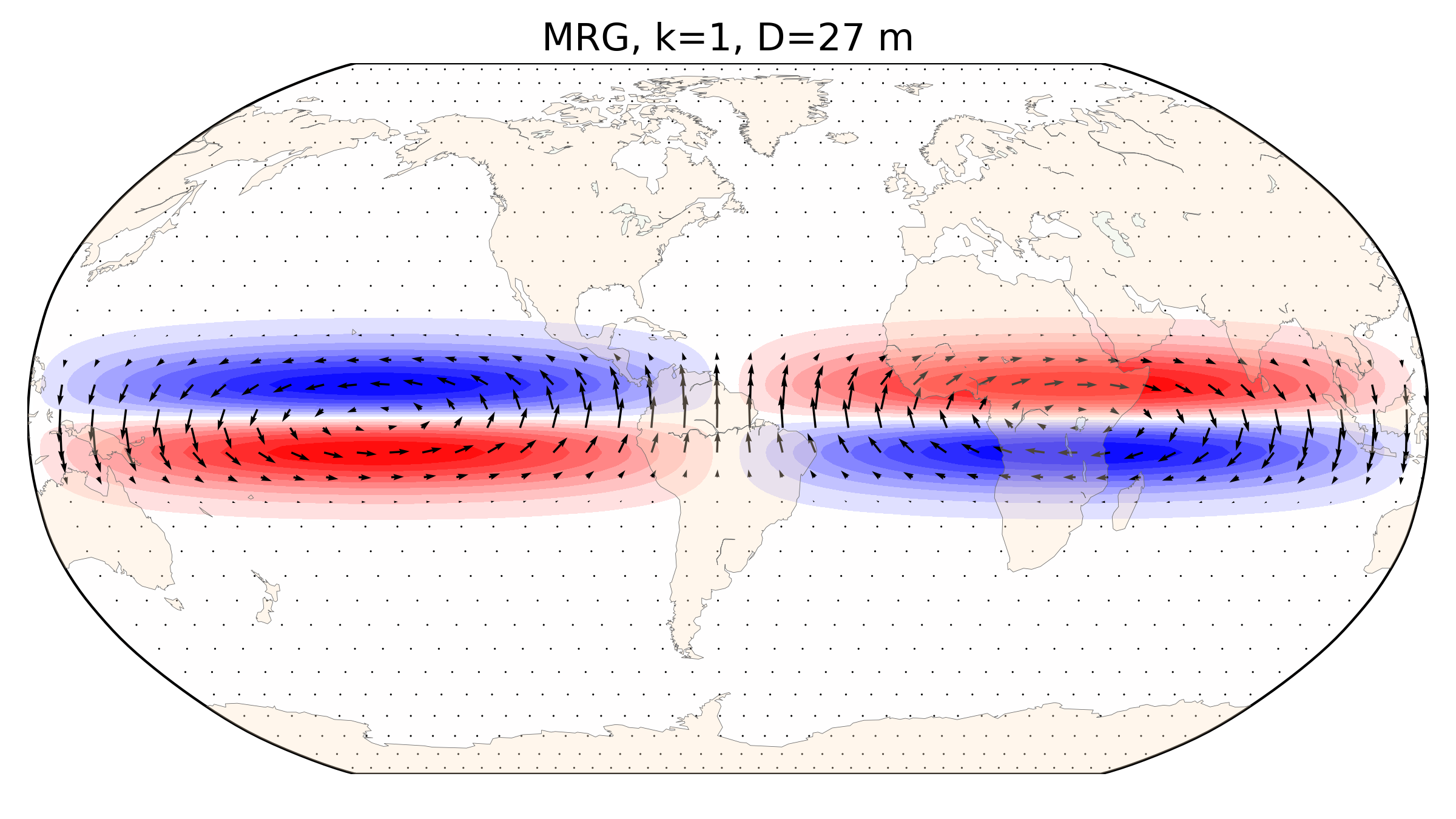
(b) Mixed Rossby-gravity Waves
Horizontal structure of the n=0 westward-propagating rotational mode on the sphere, mixed Rossby-gravity wave.
Geopotential height is scaled by the maximal height. Colours vary between -1 and +1 with positive/negative perturbations in red/blue.
Wind components are scaled by the maximal wind speed. Mixed Rossby-gravity (MRG) wave is the equatorially-trapped mode which together with the Kelvin wave fills the frequency gap between the Rossby and gravity modes in the tropics. As its zonal wavenumber increases, the MRG wave becomes increasingly more rotational mode. In the case of linearized equations on the equatorial beta-plane, the mixed Rossby-gravity wave is denoted the westward-propagating MRG mode.
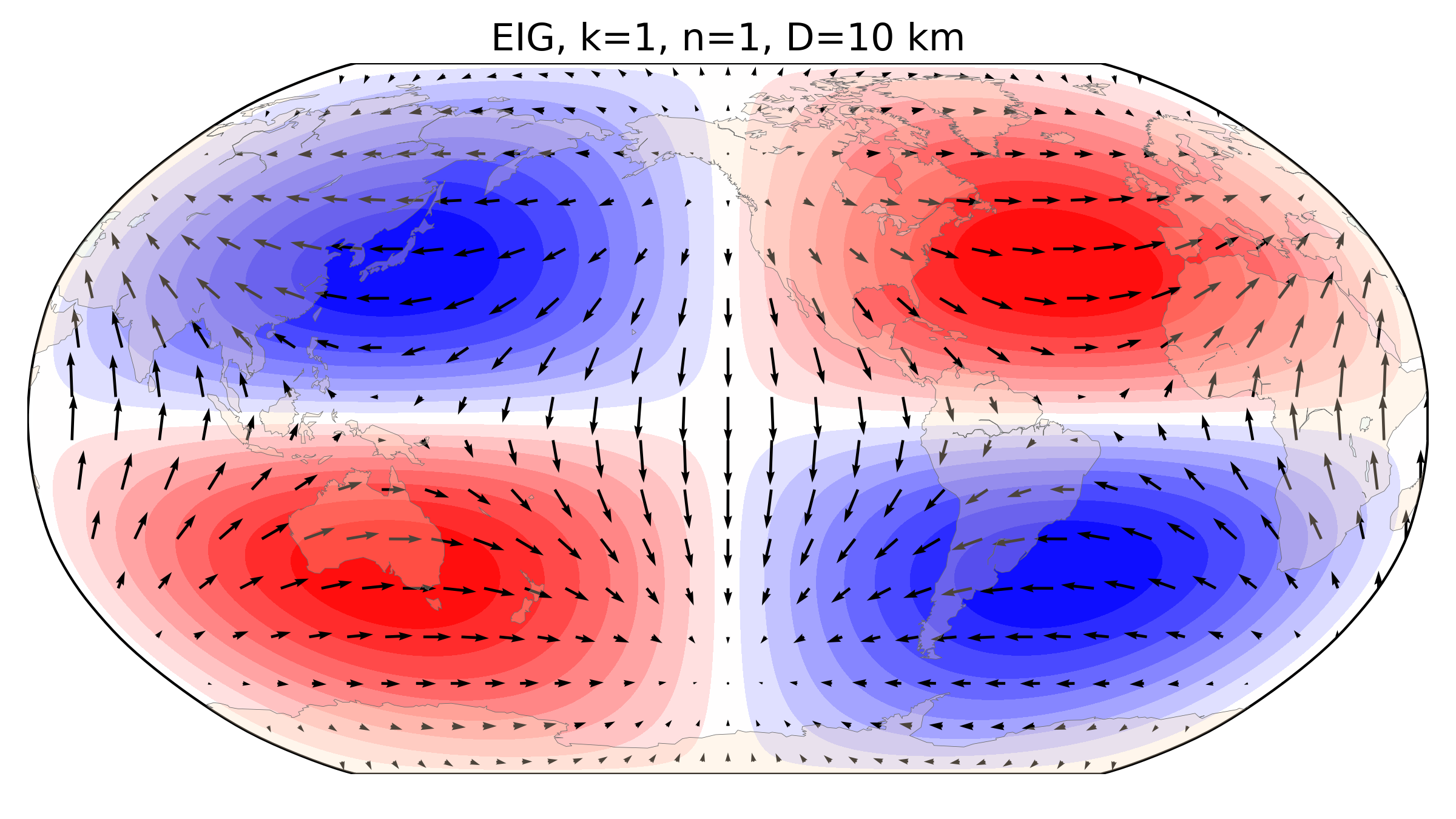
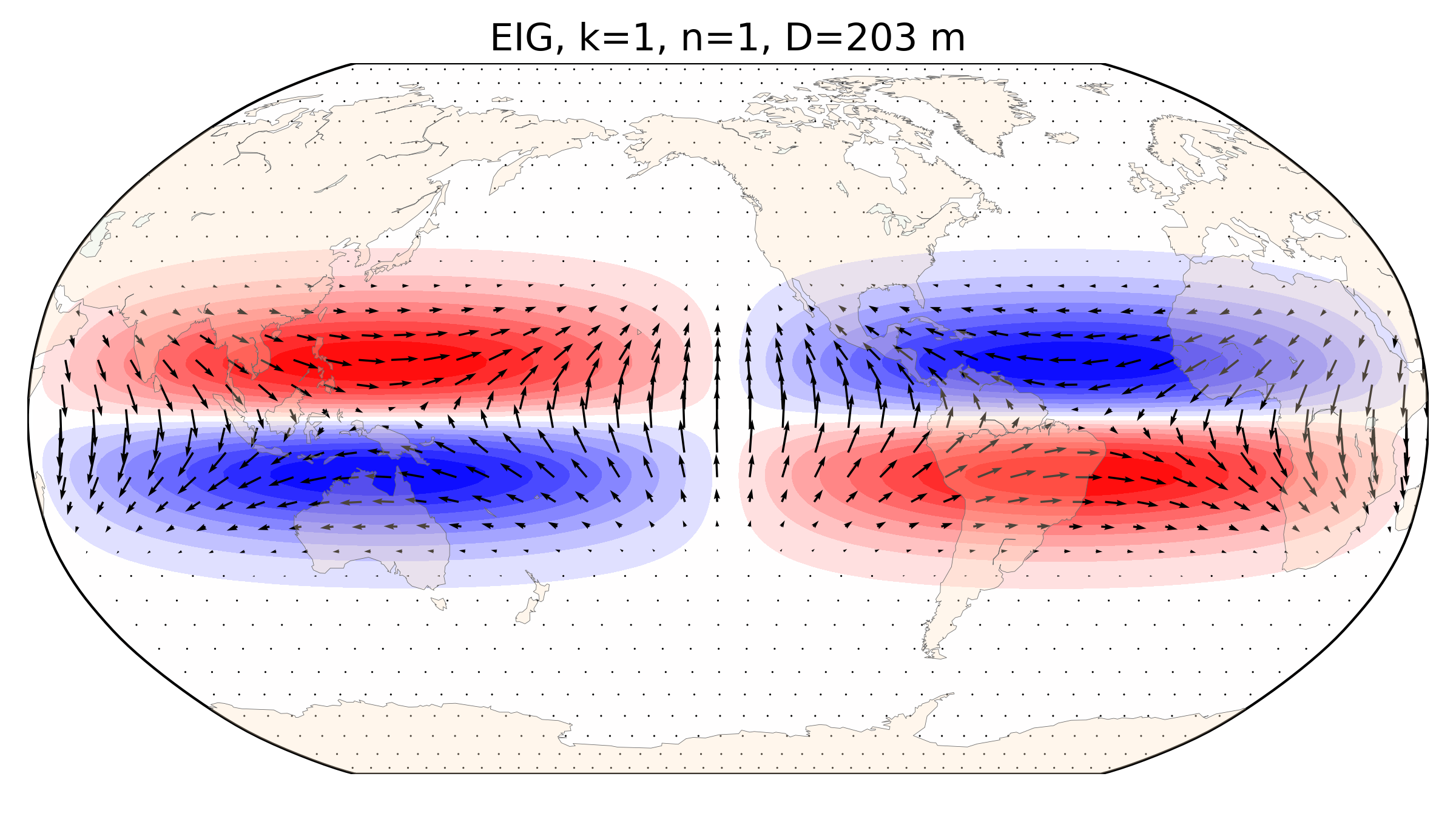
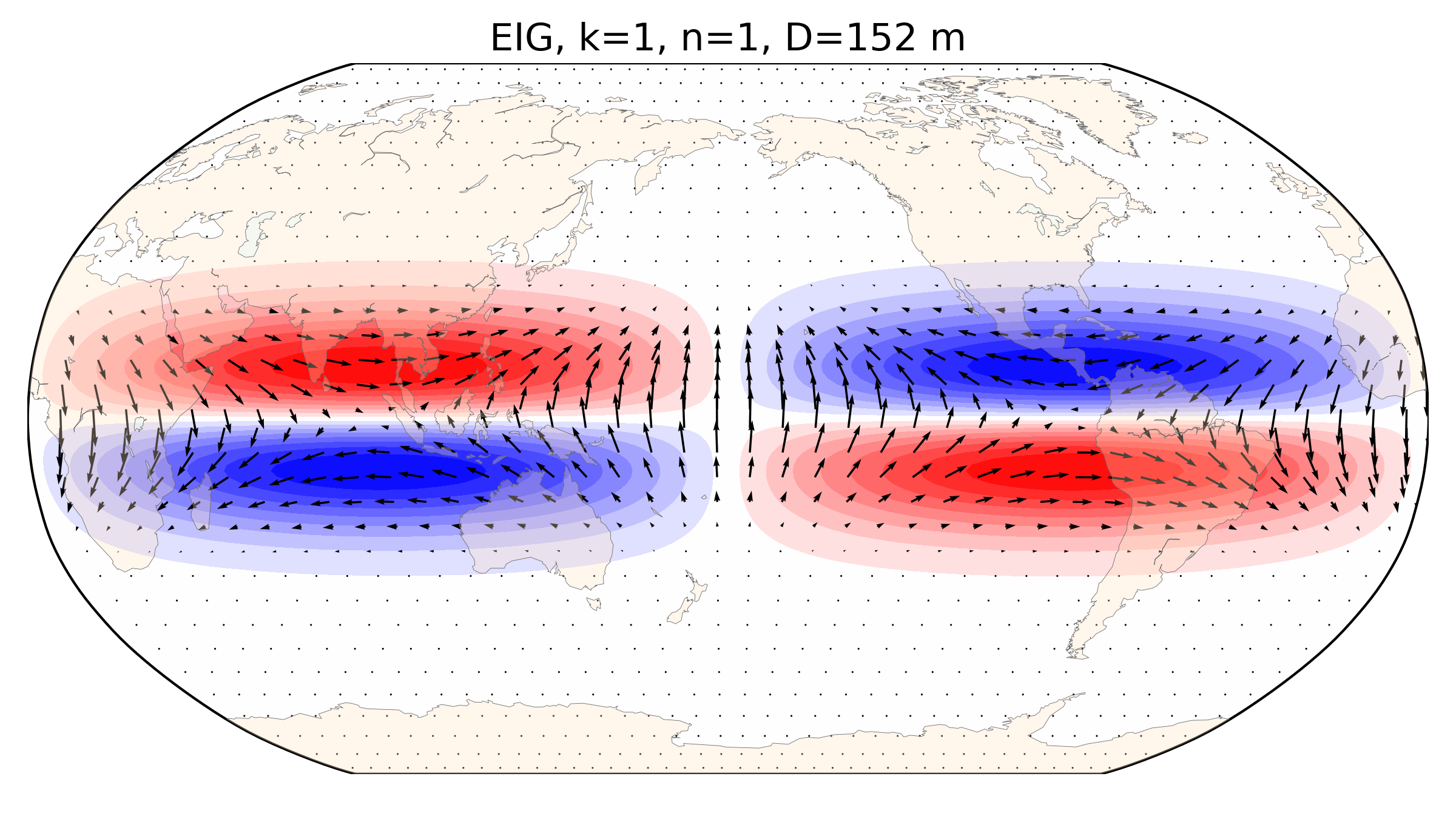
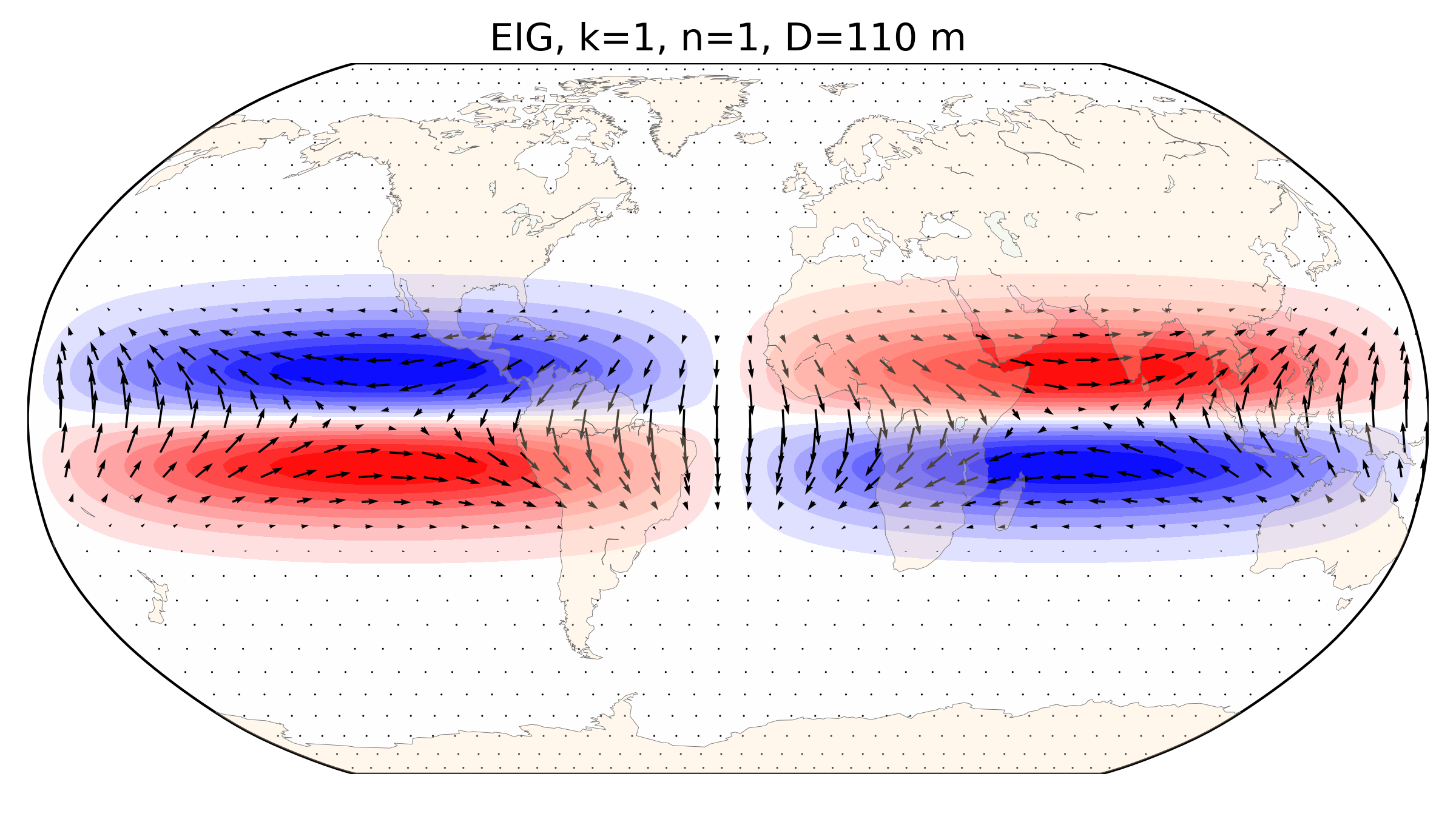
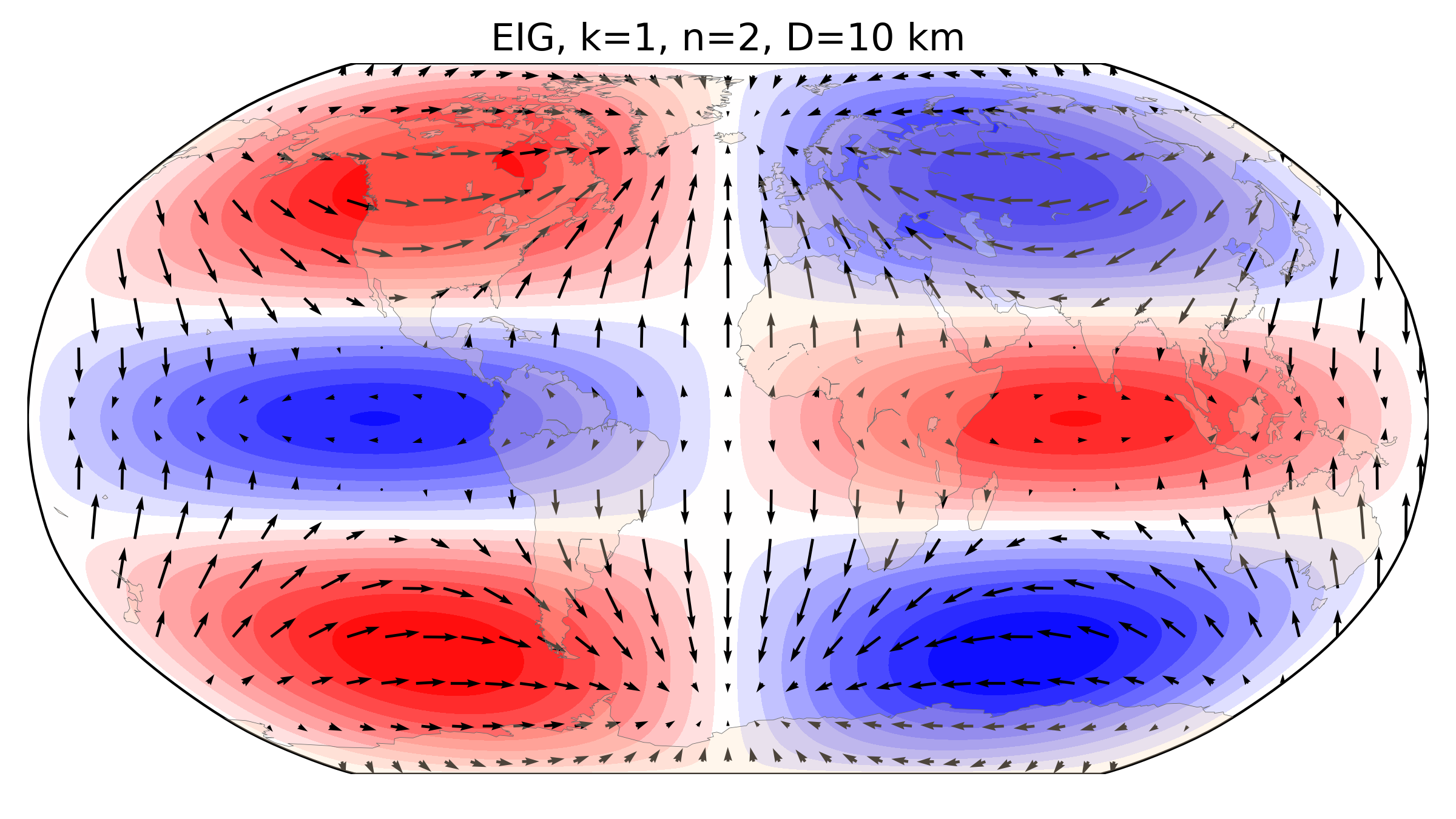
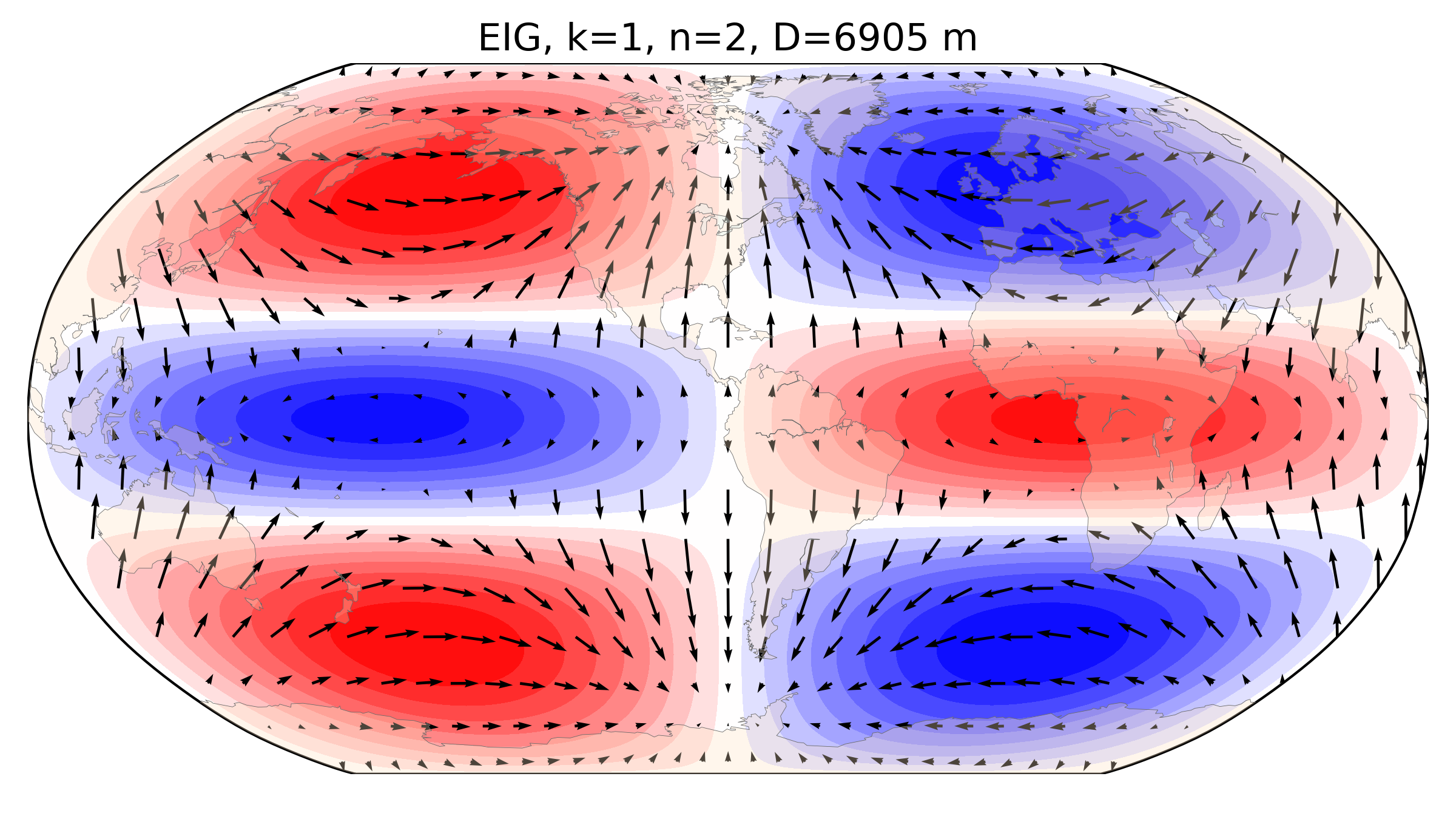
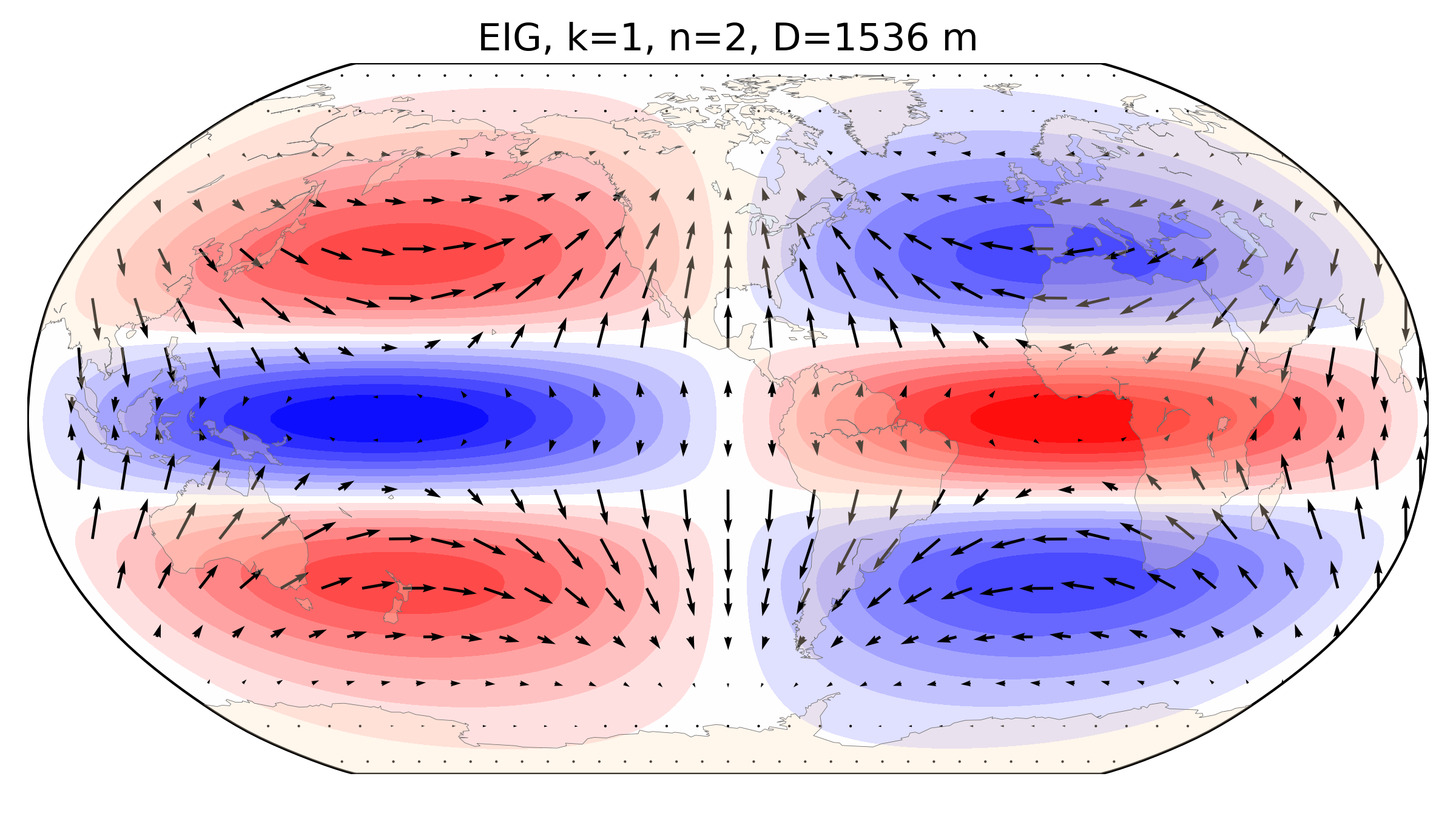
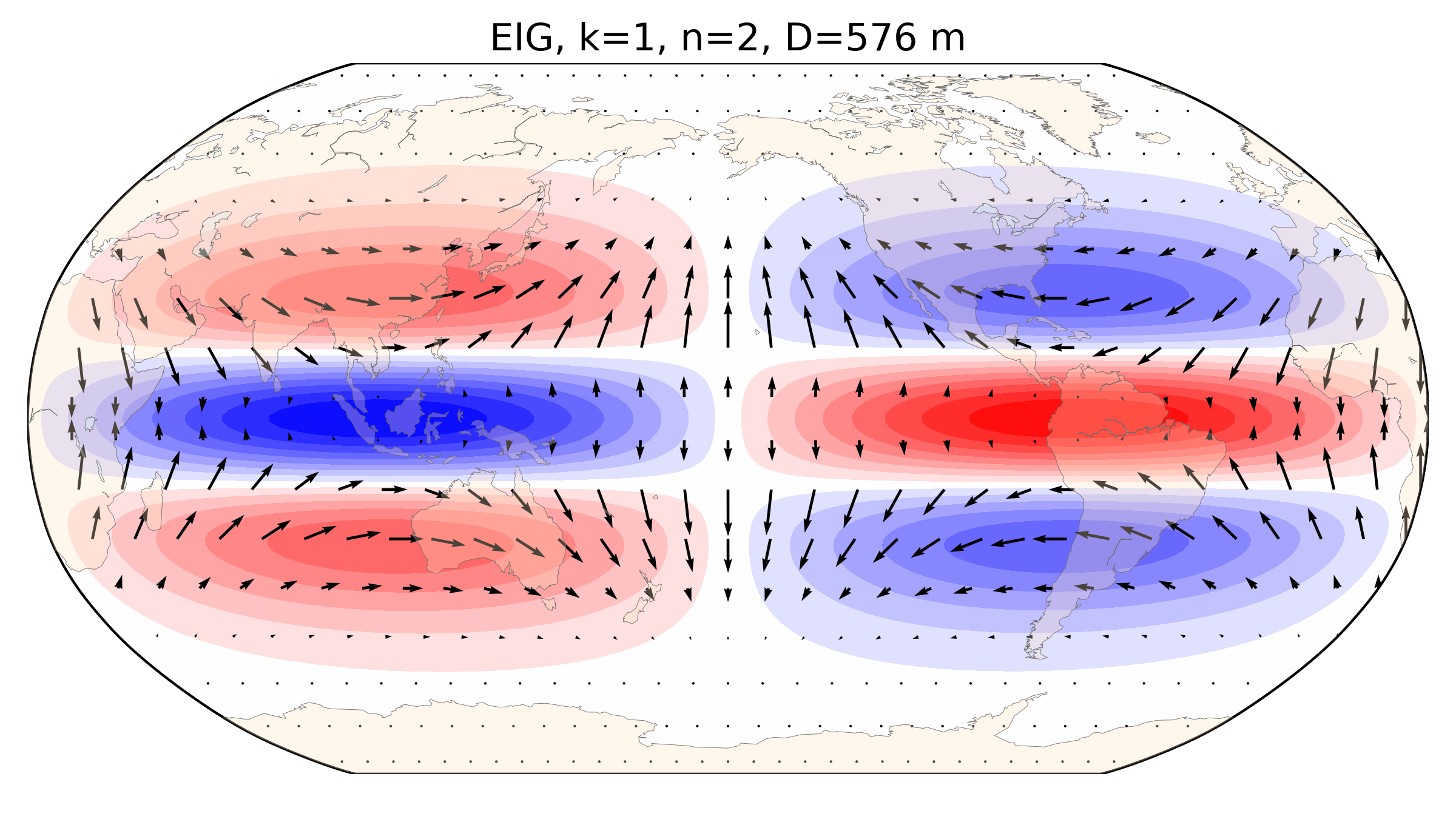
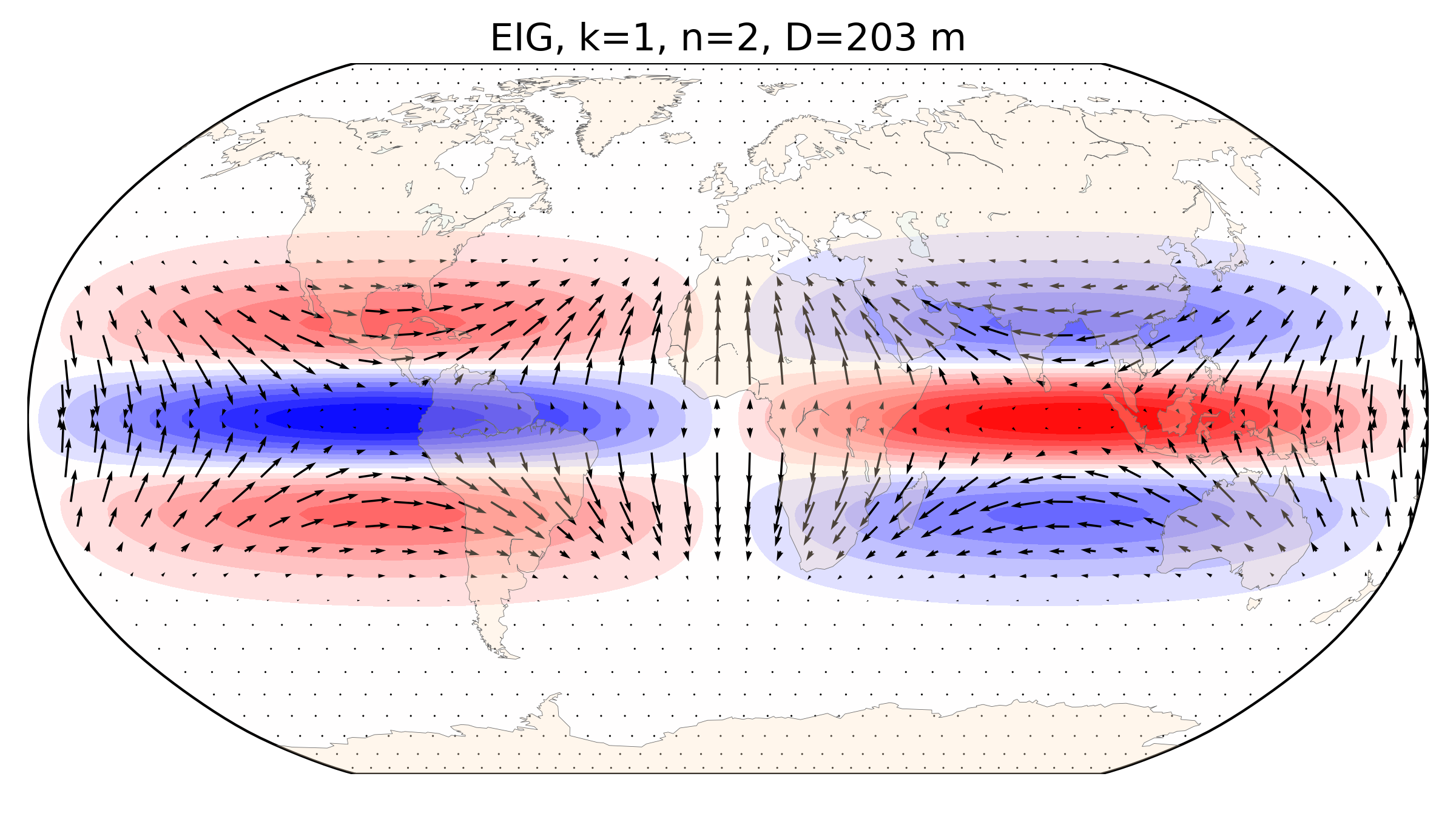
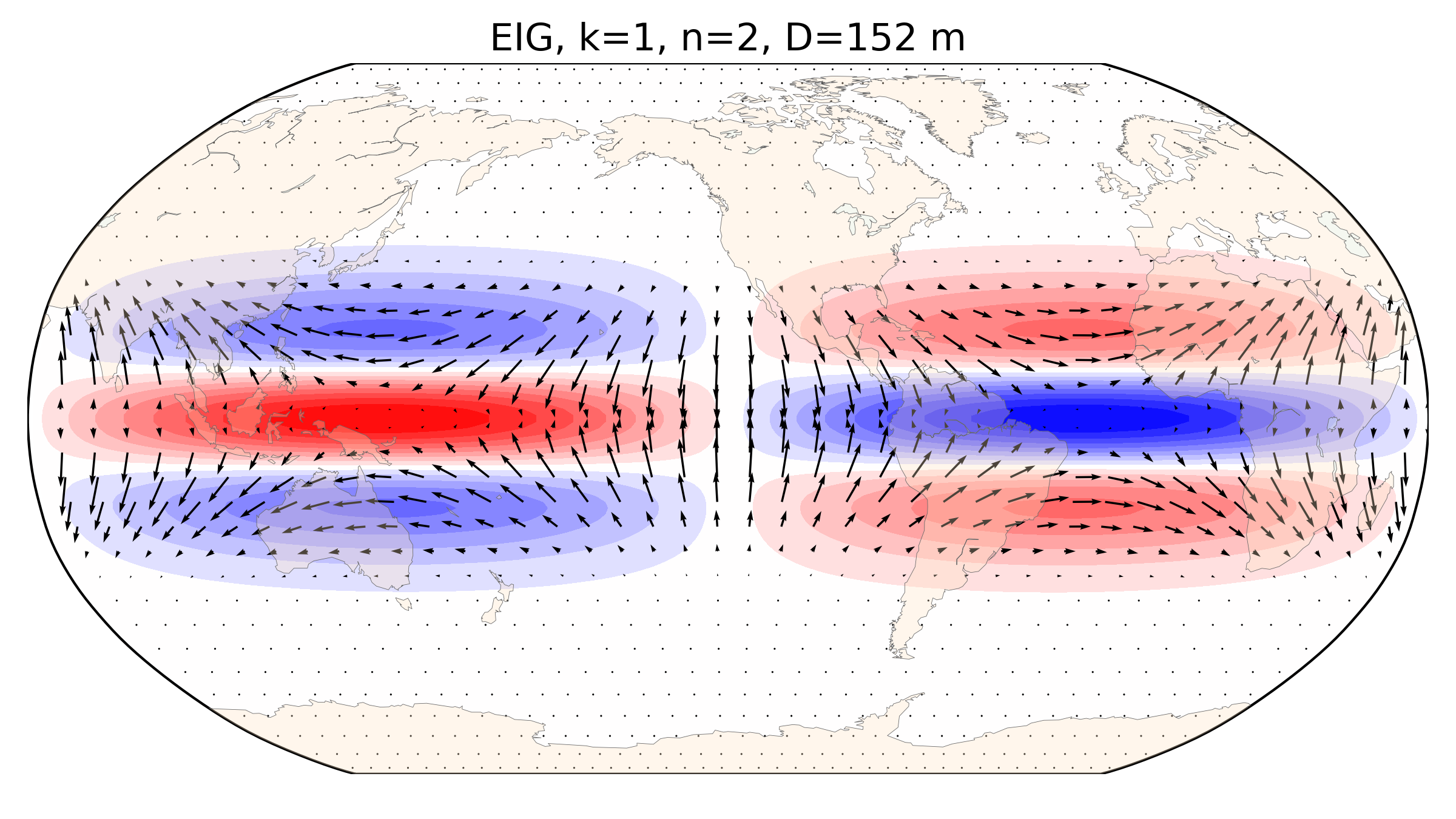
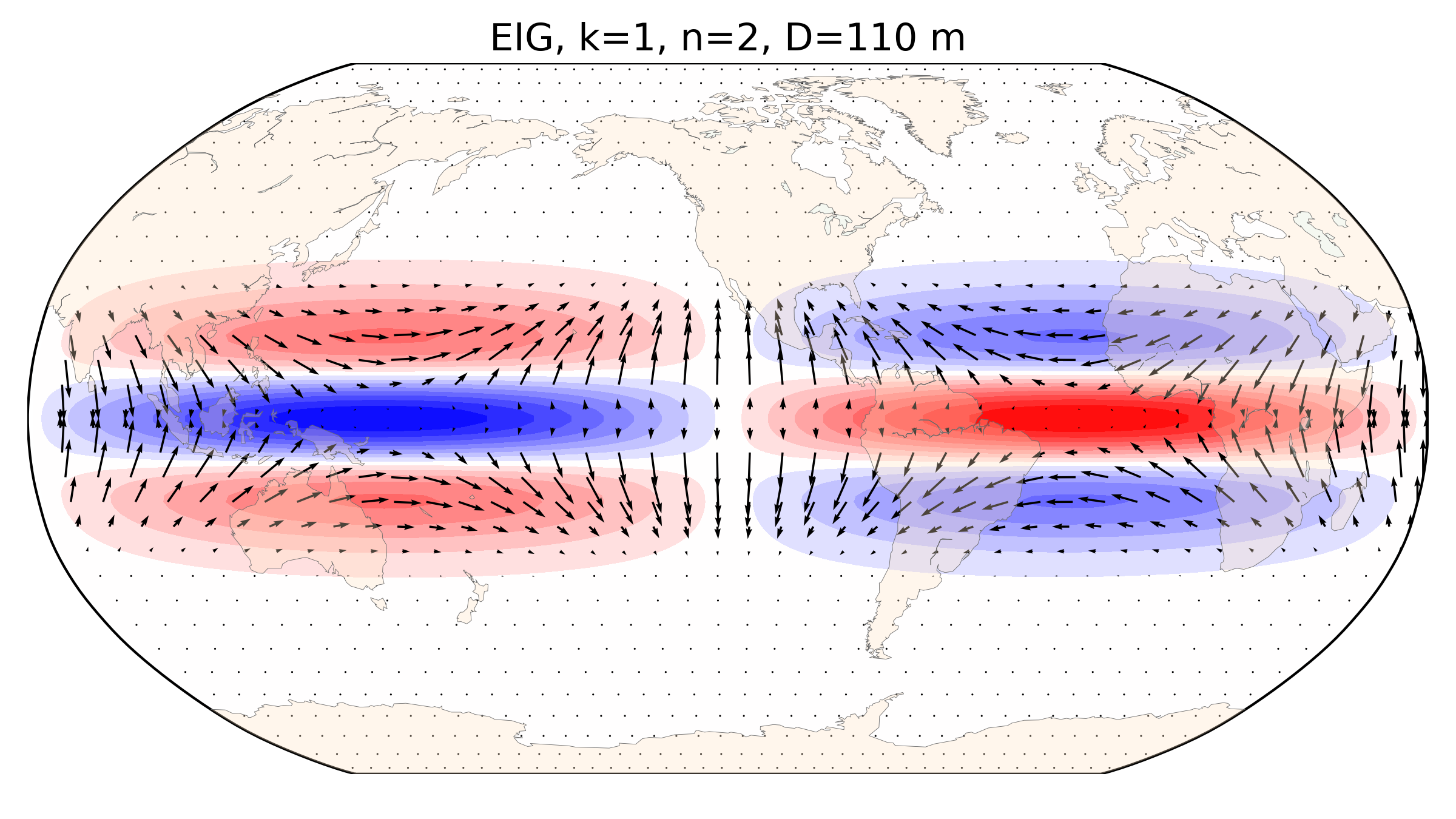
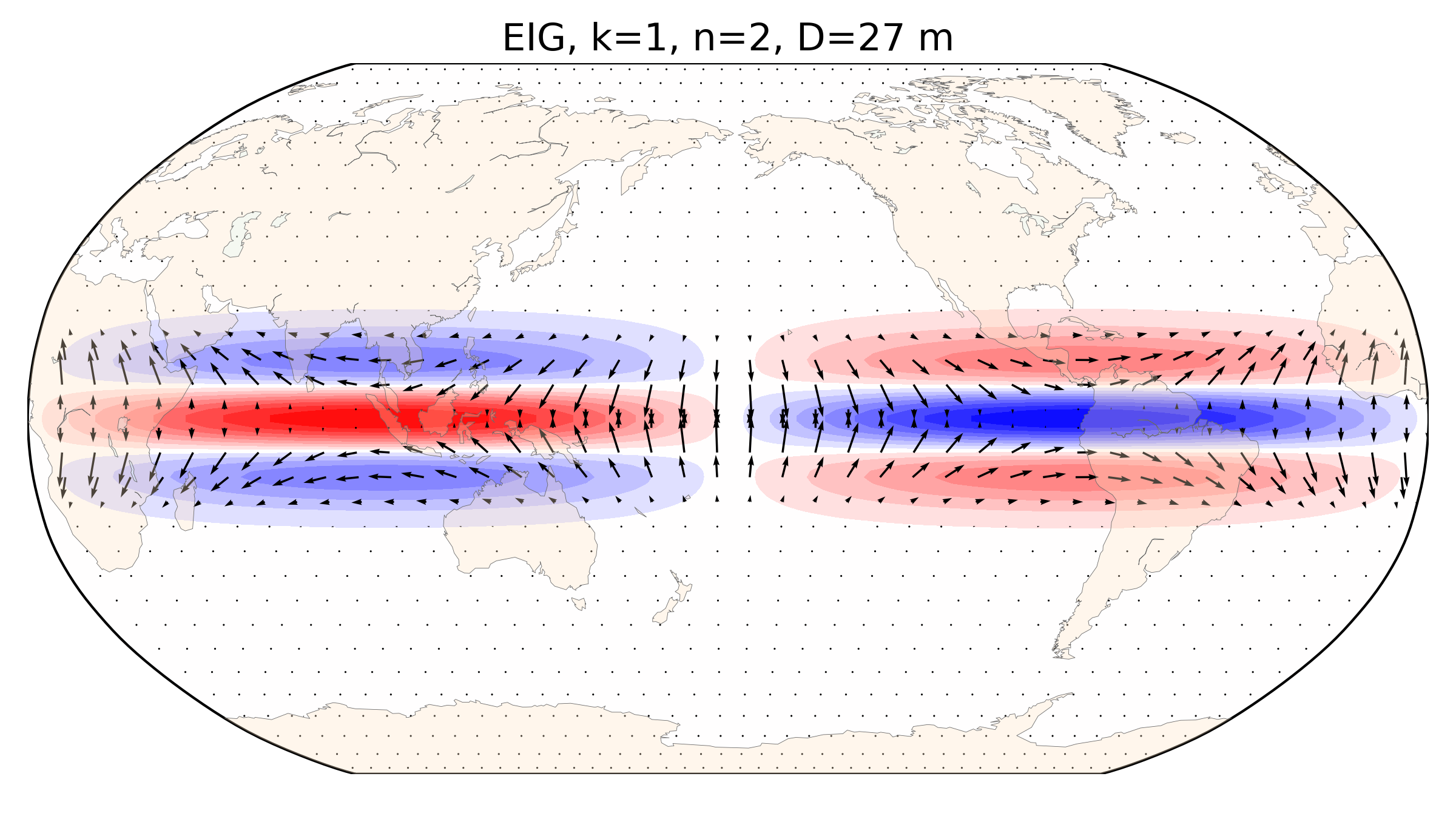
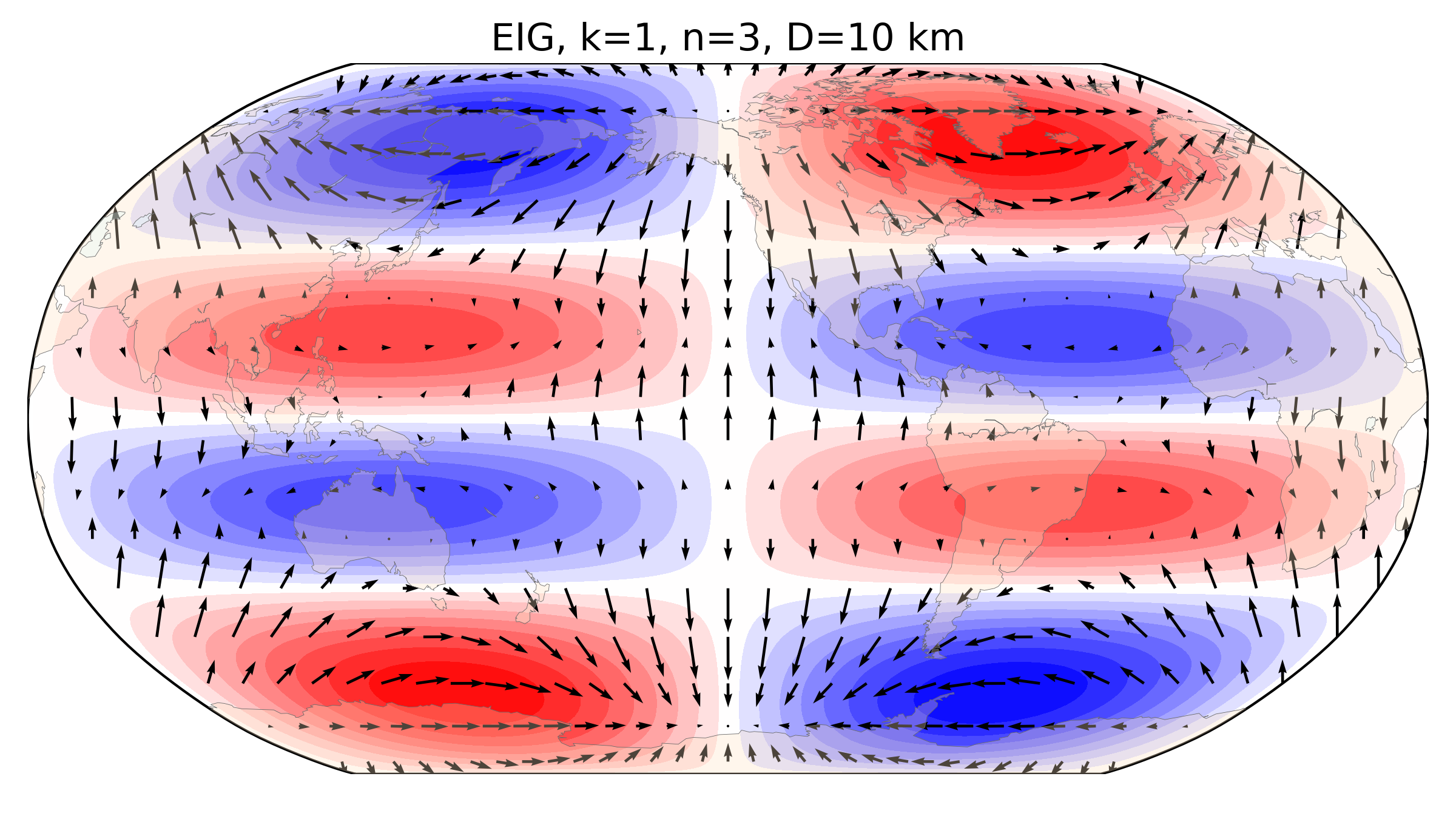
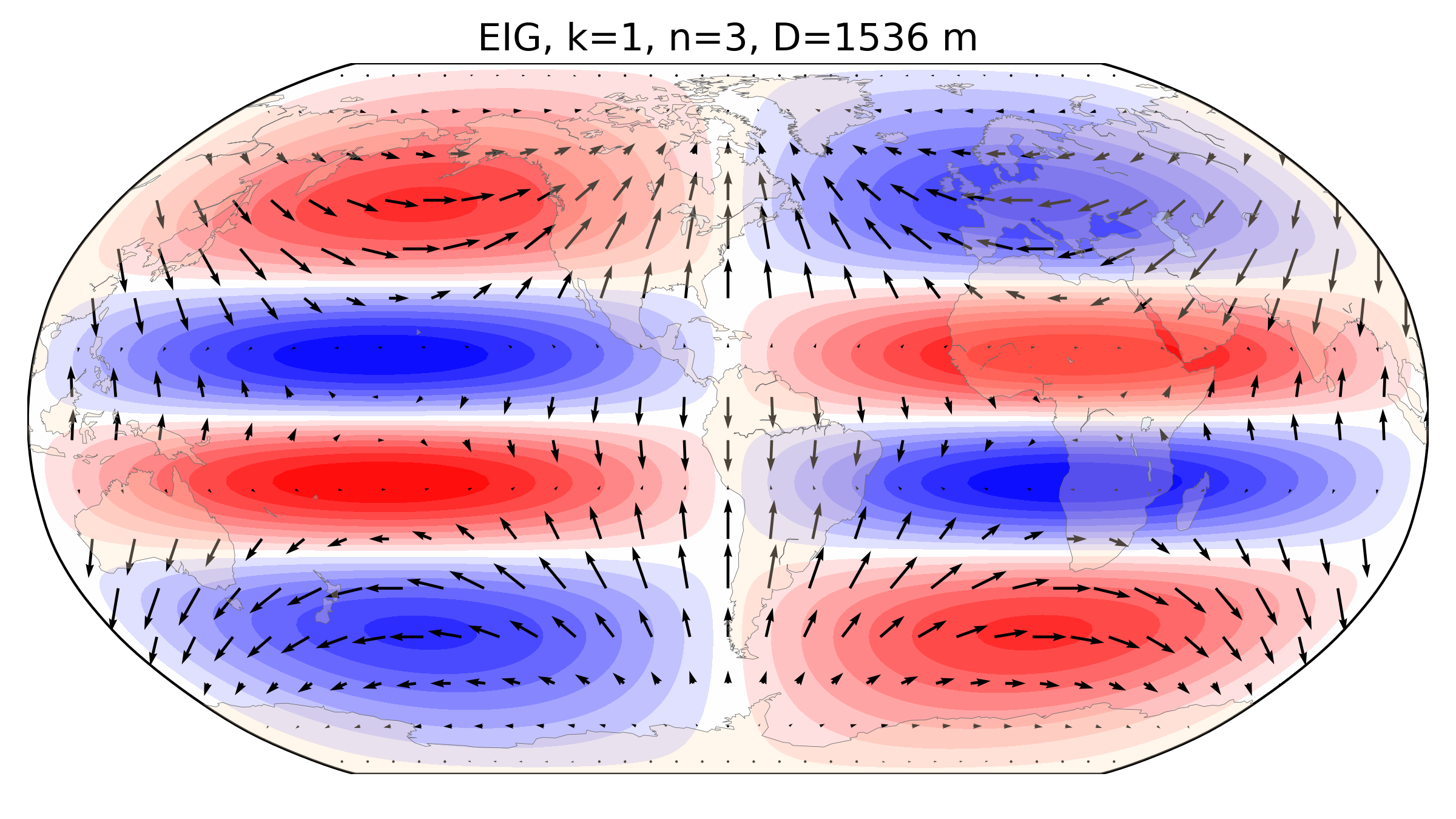
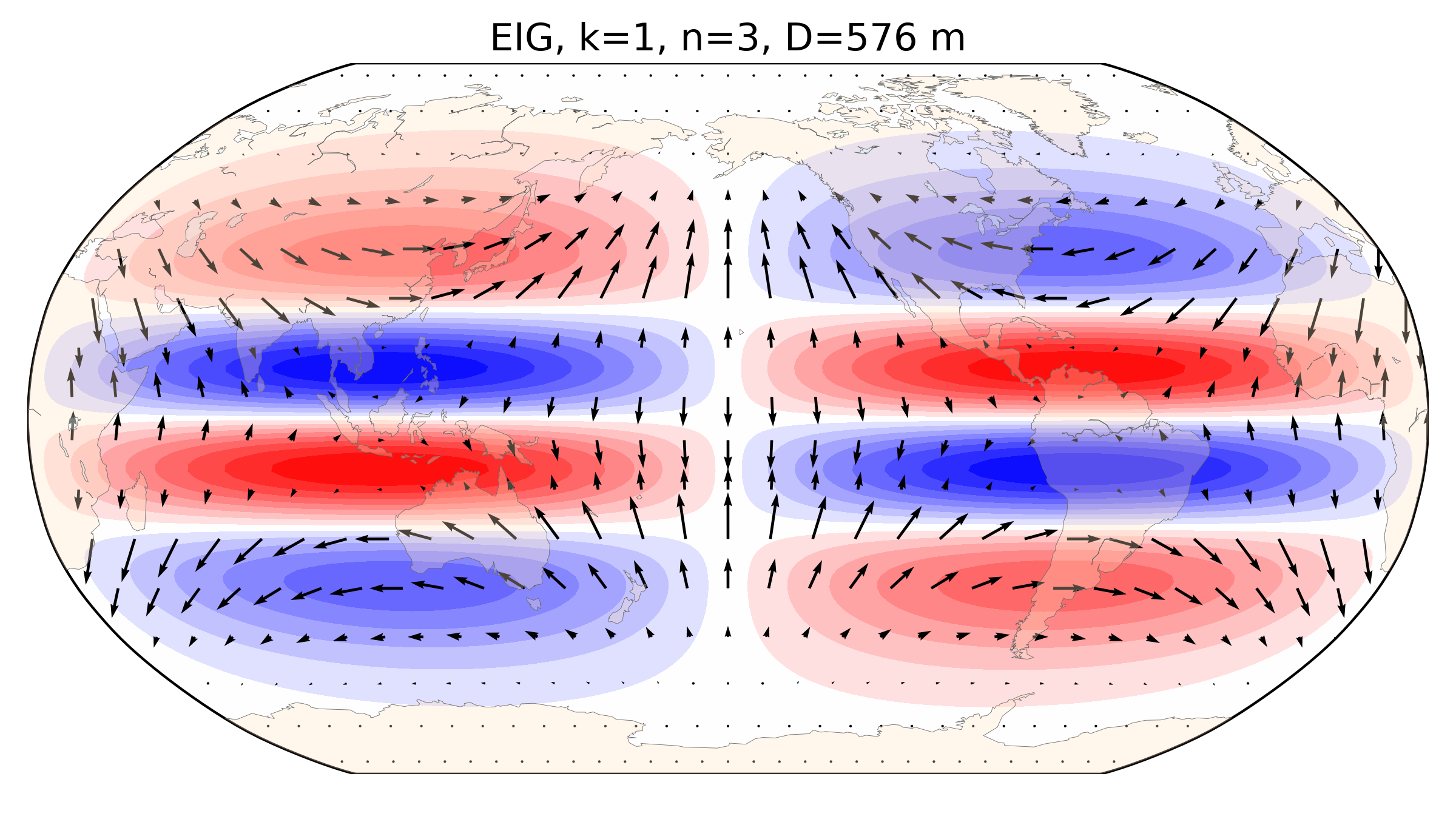
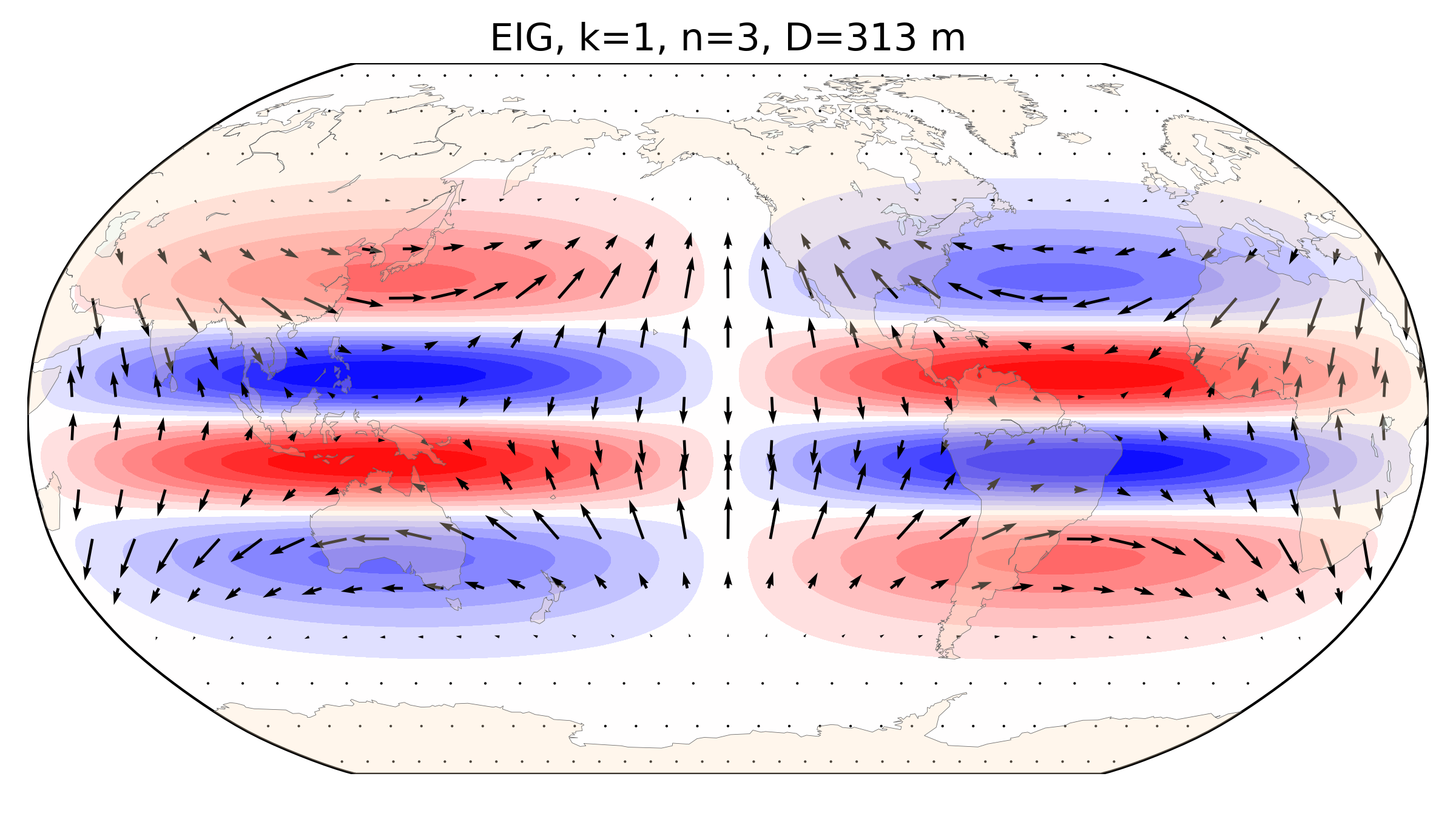
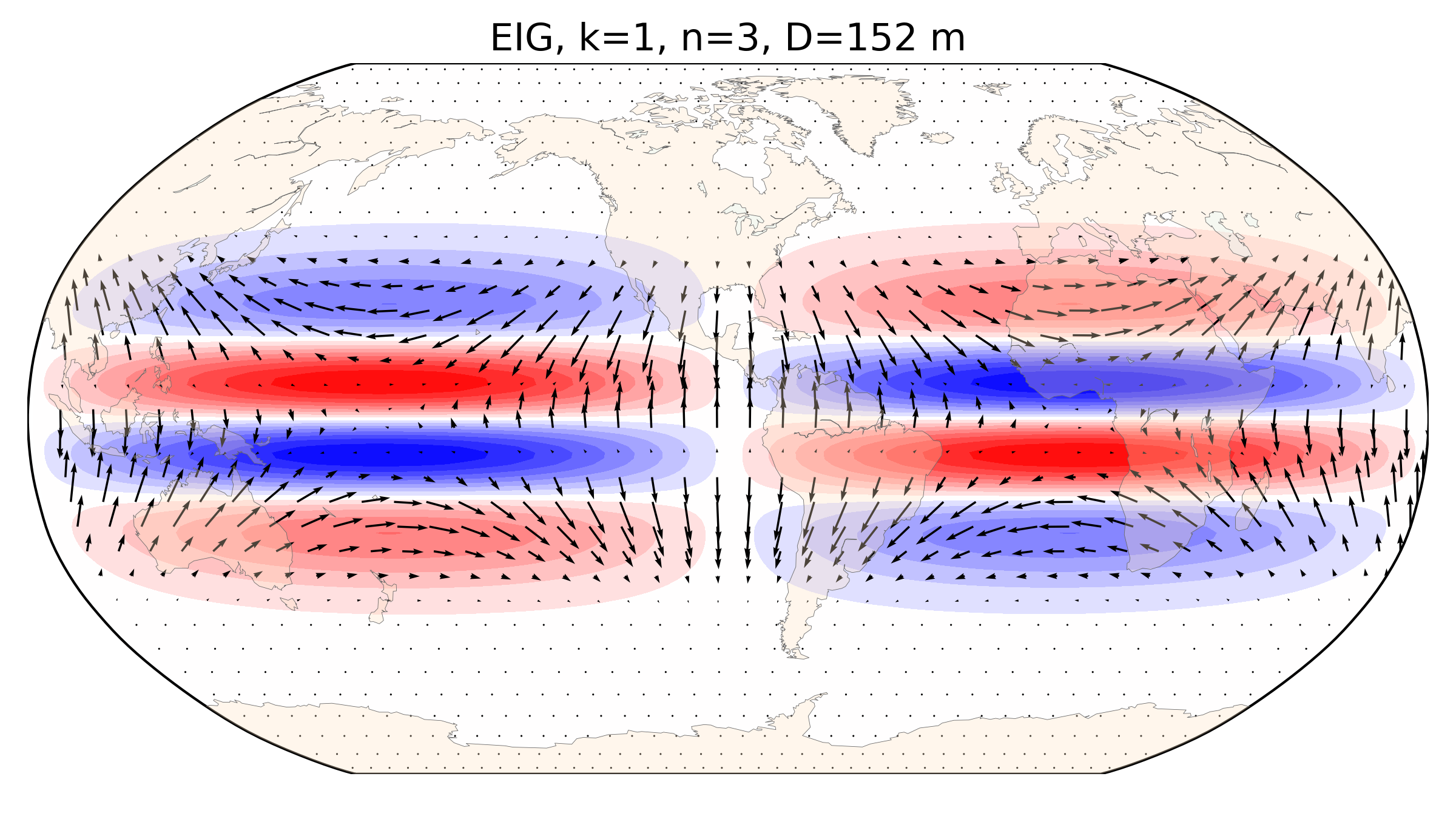
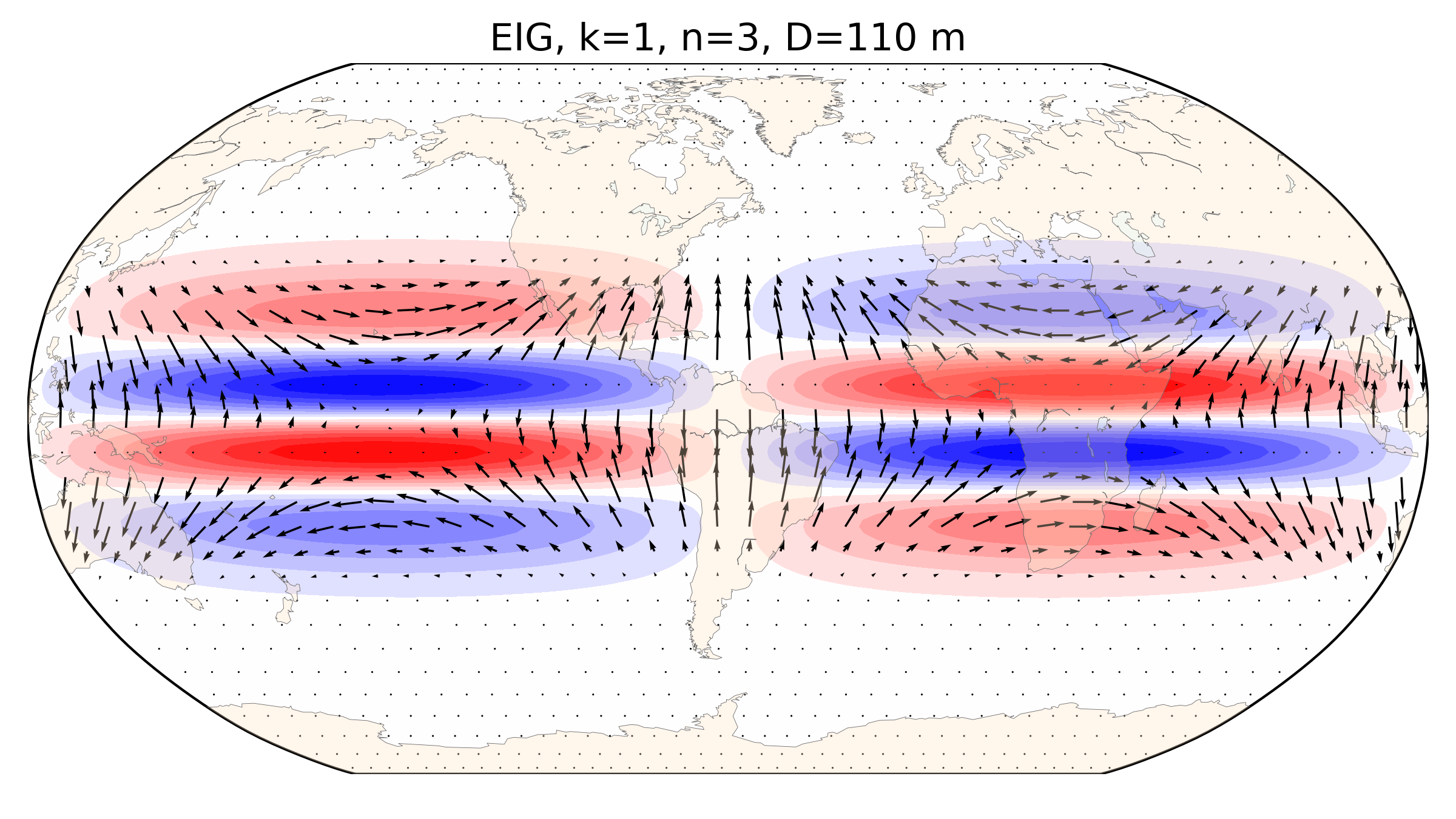
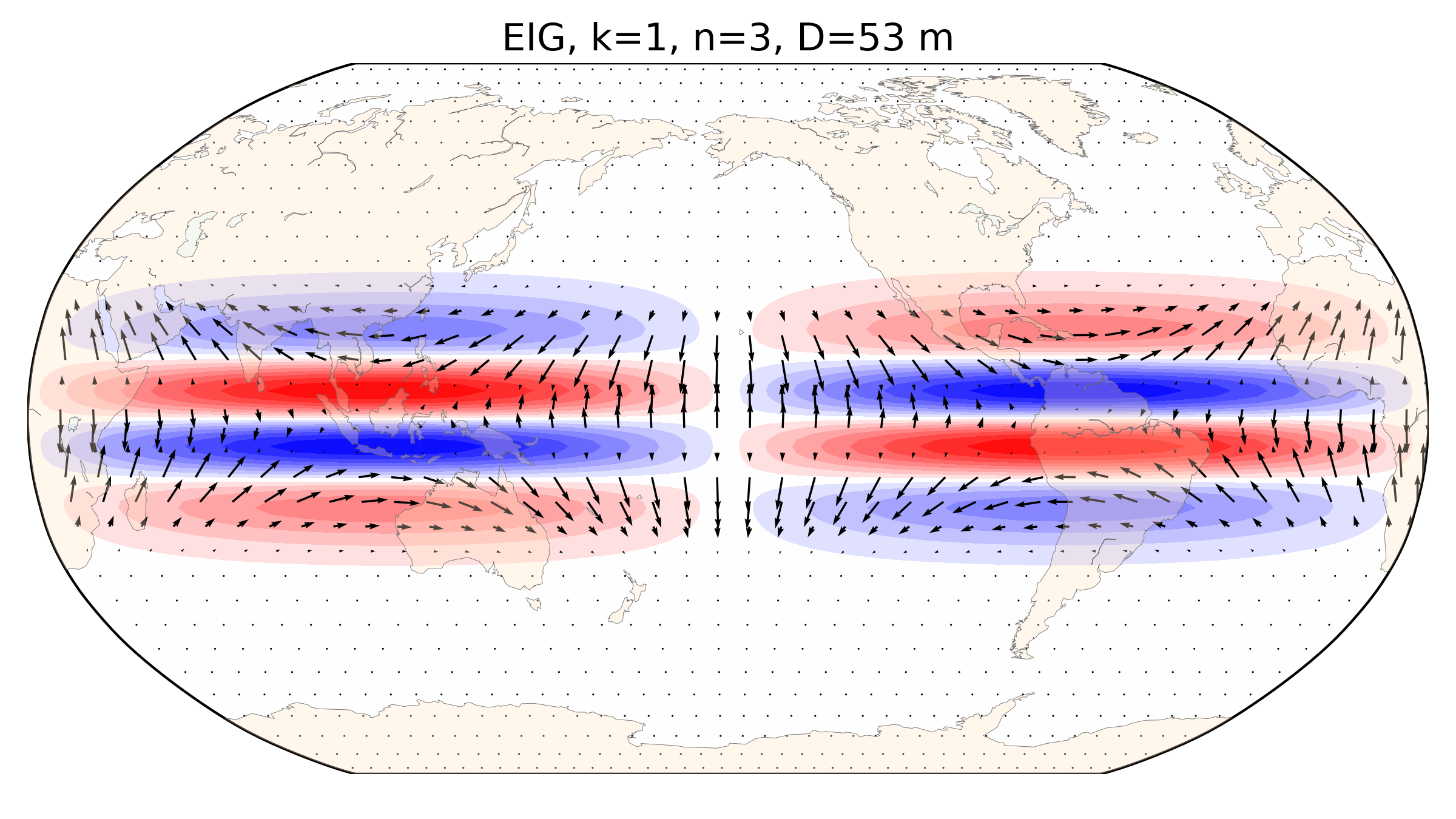
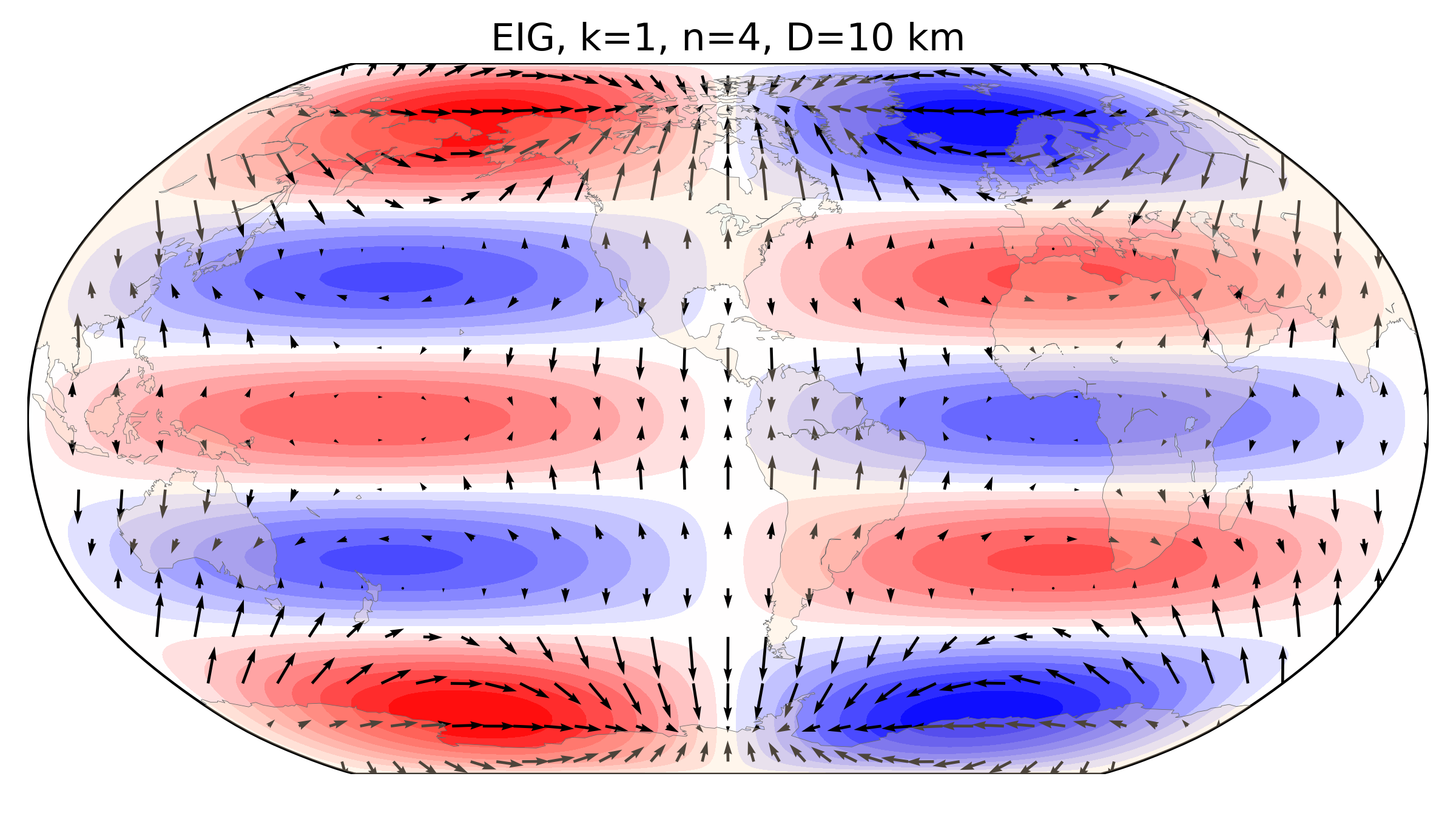
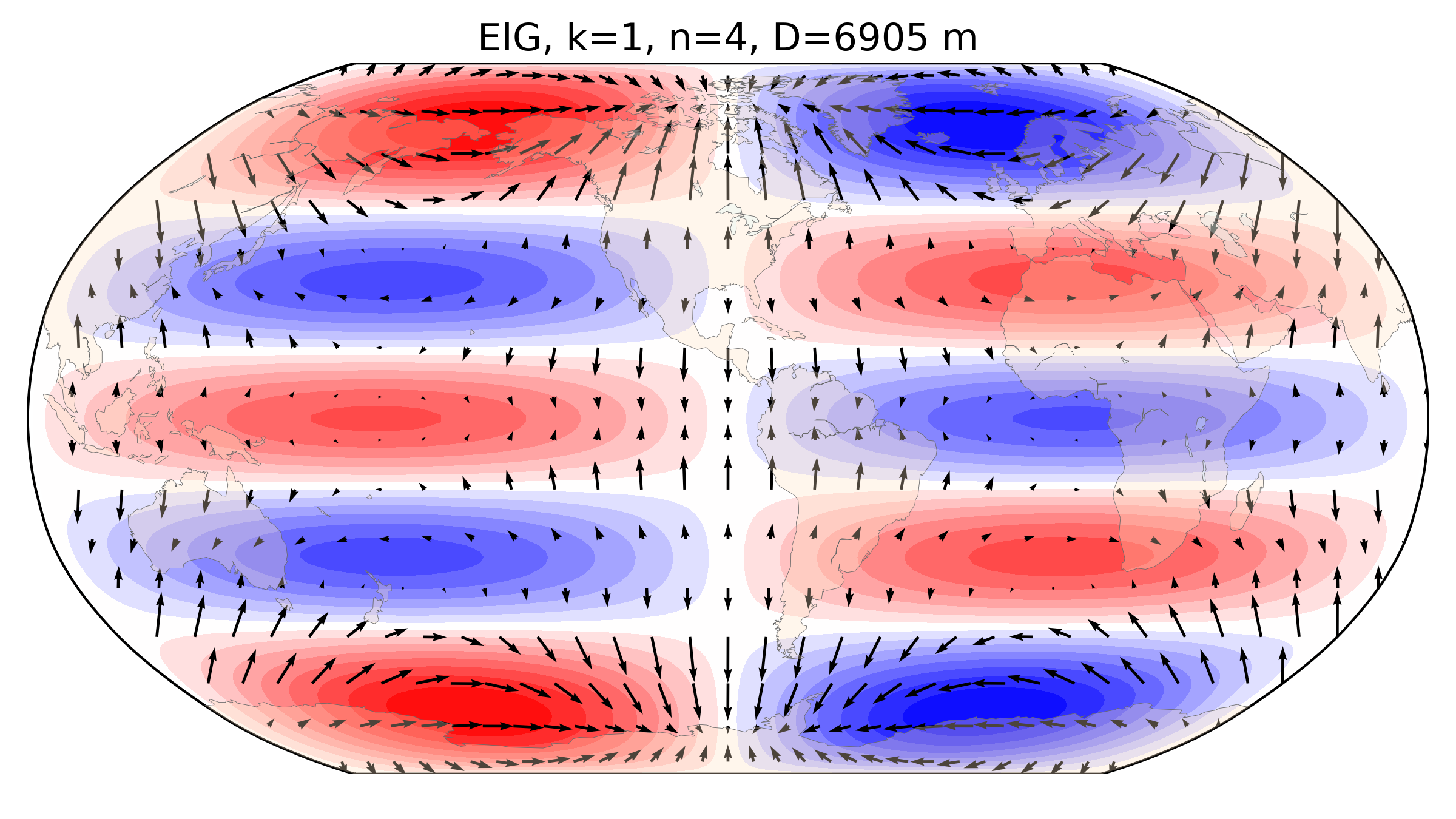
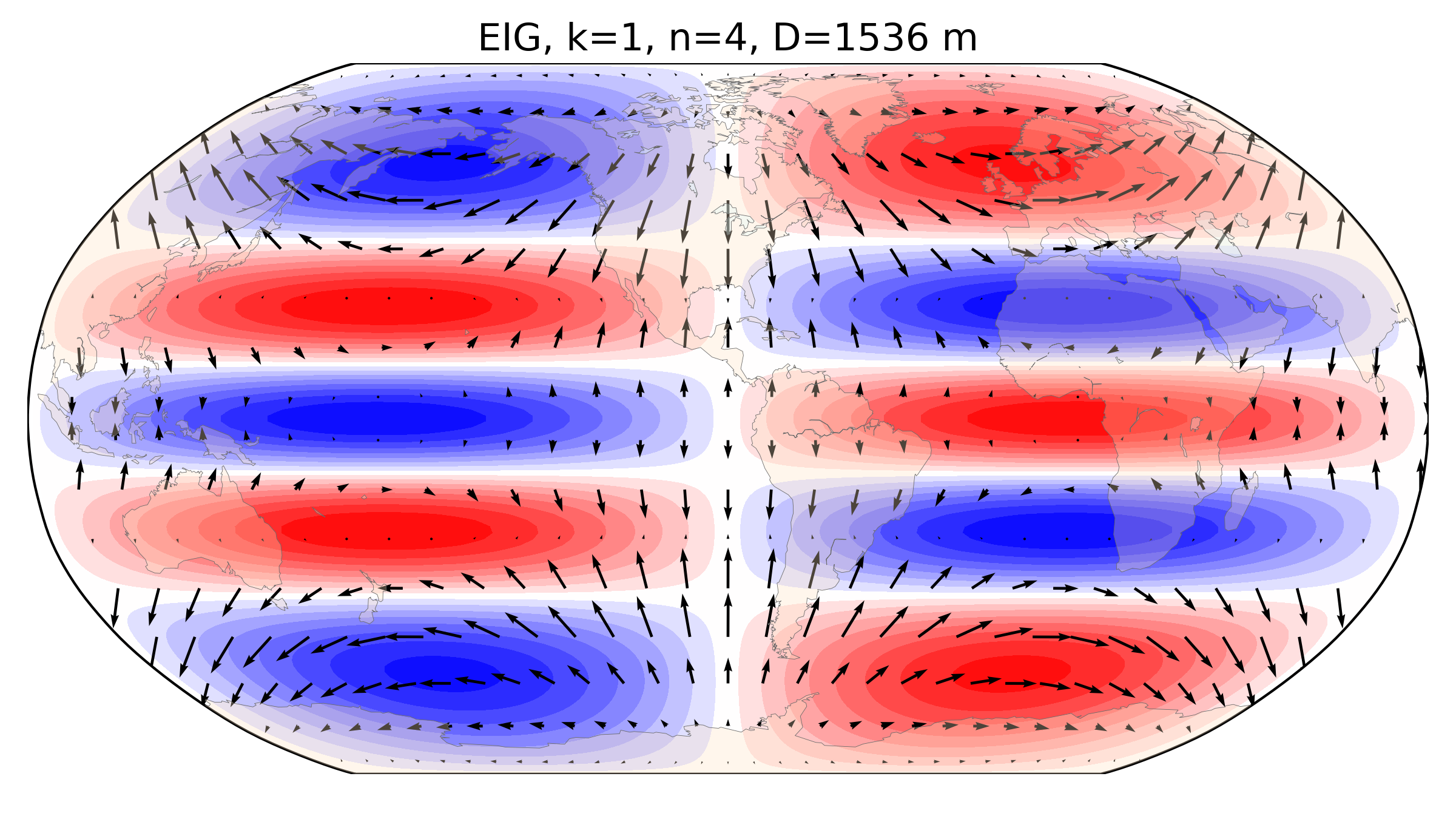
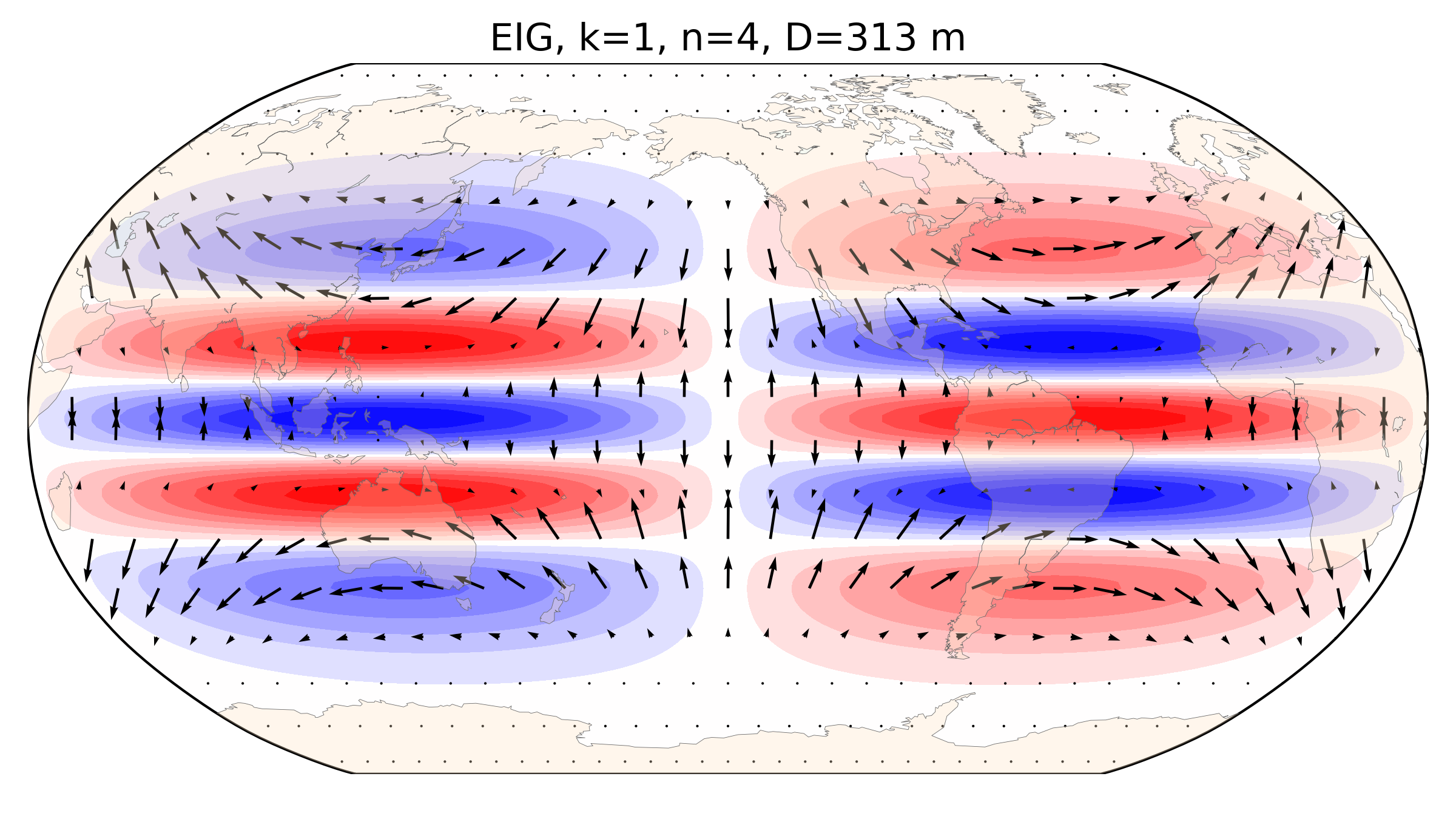
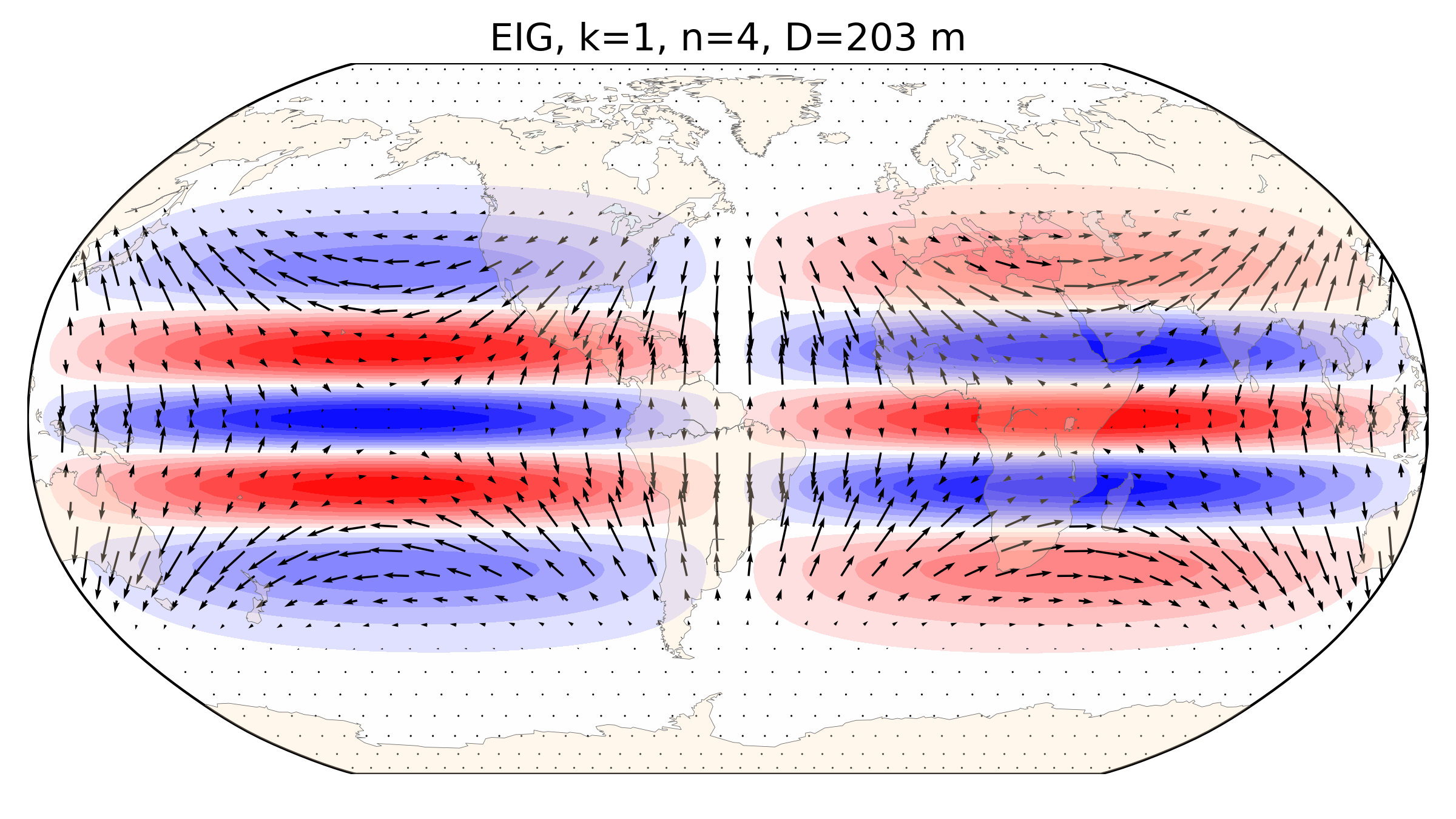
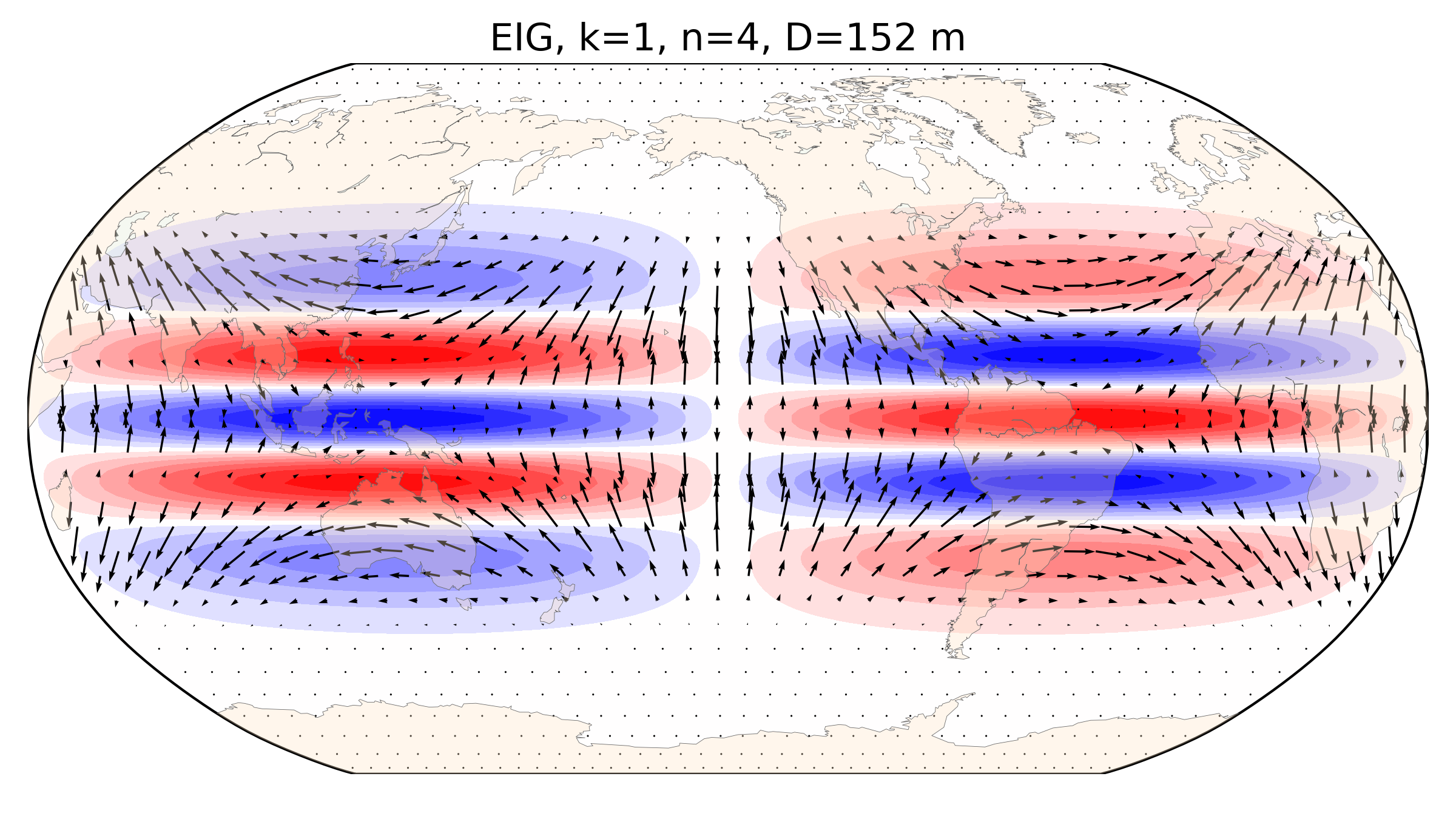
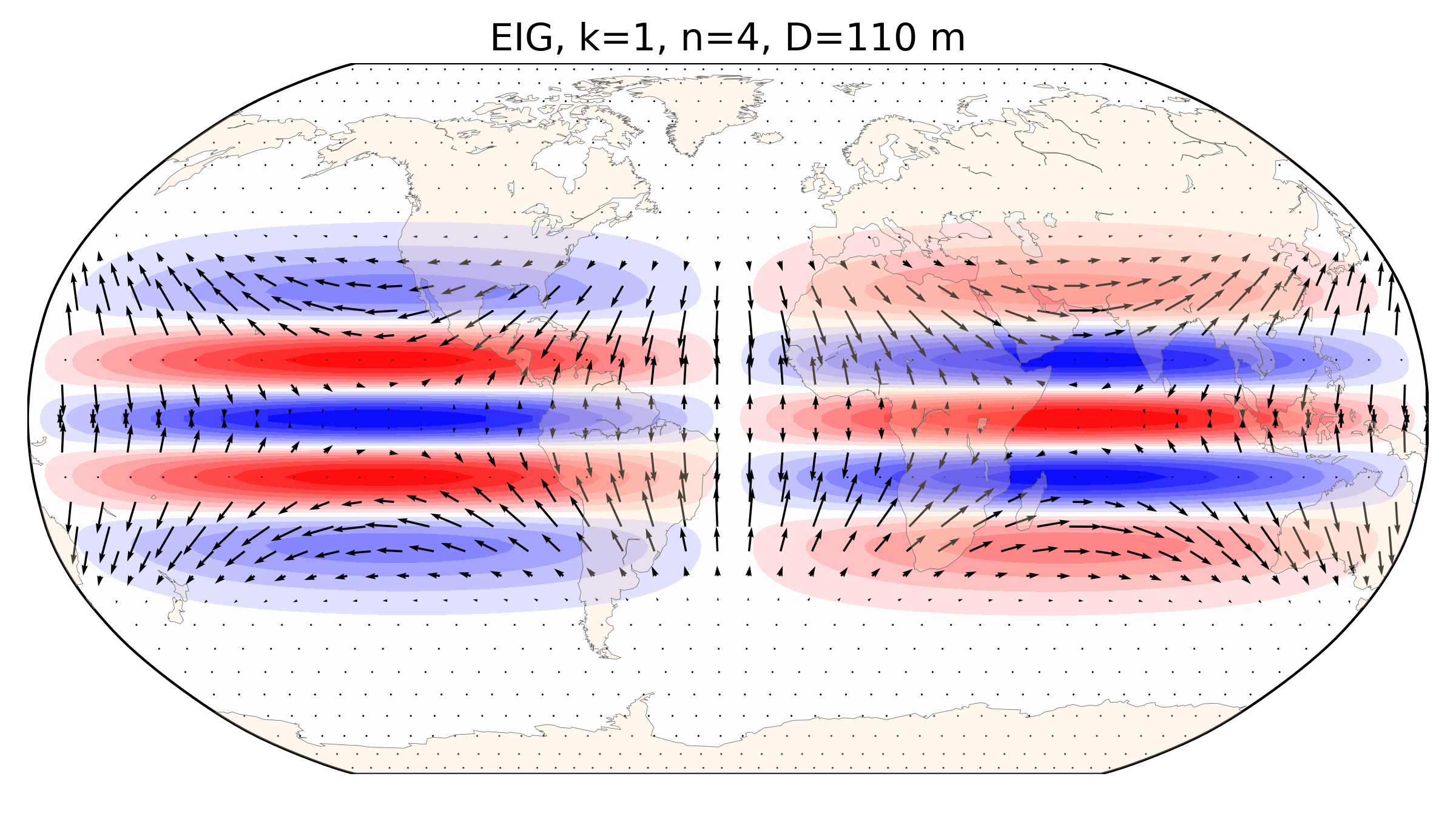
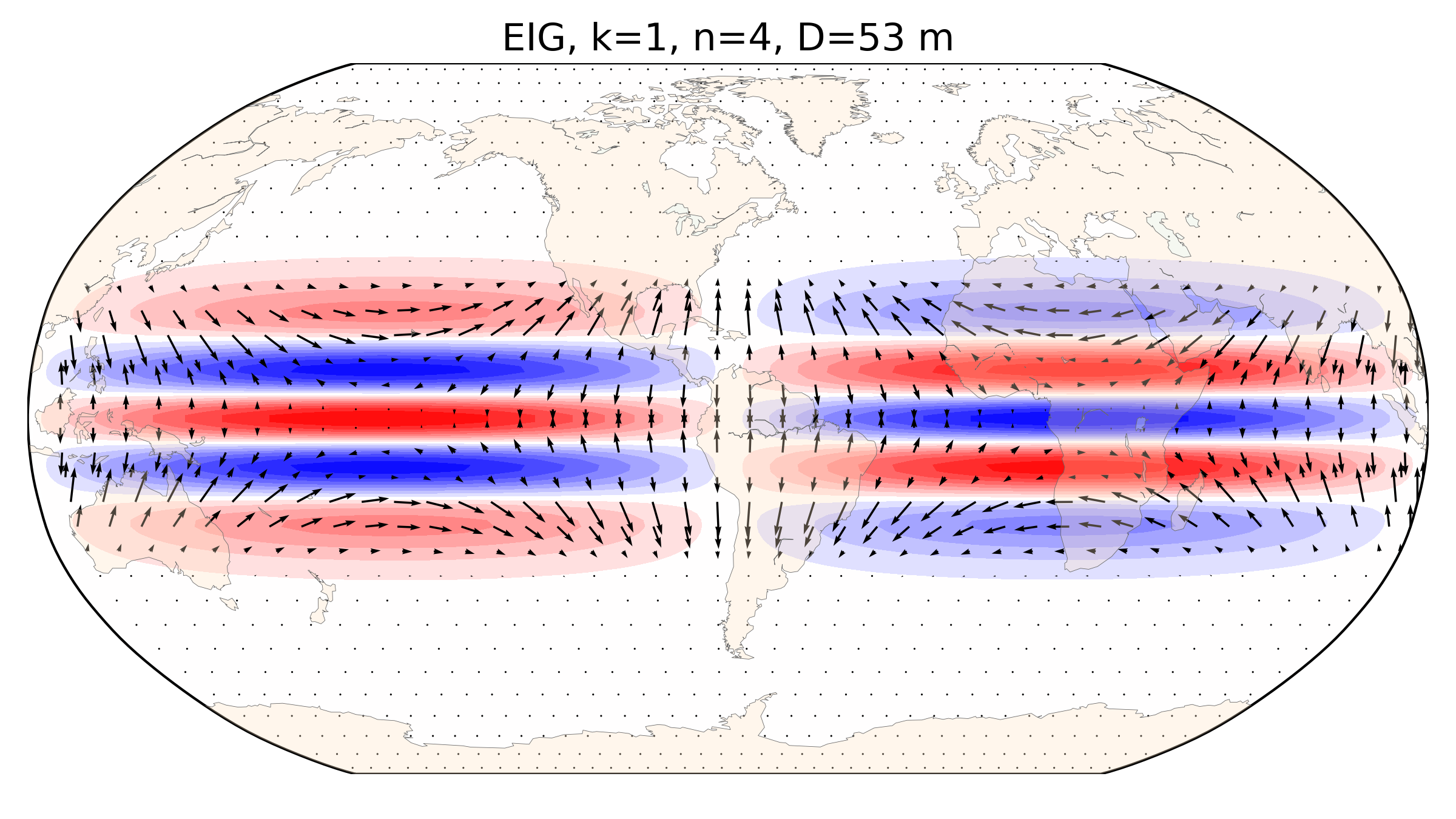
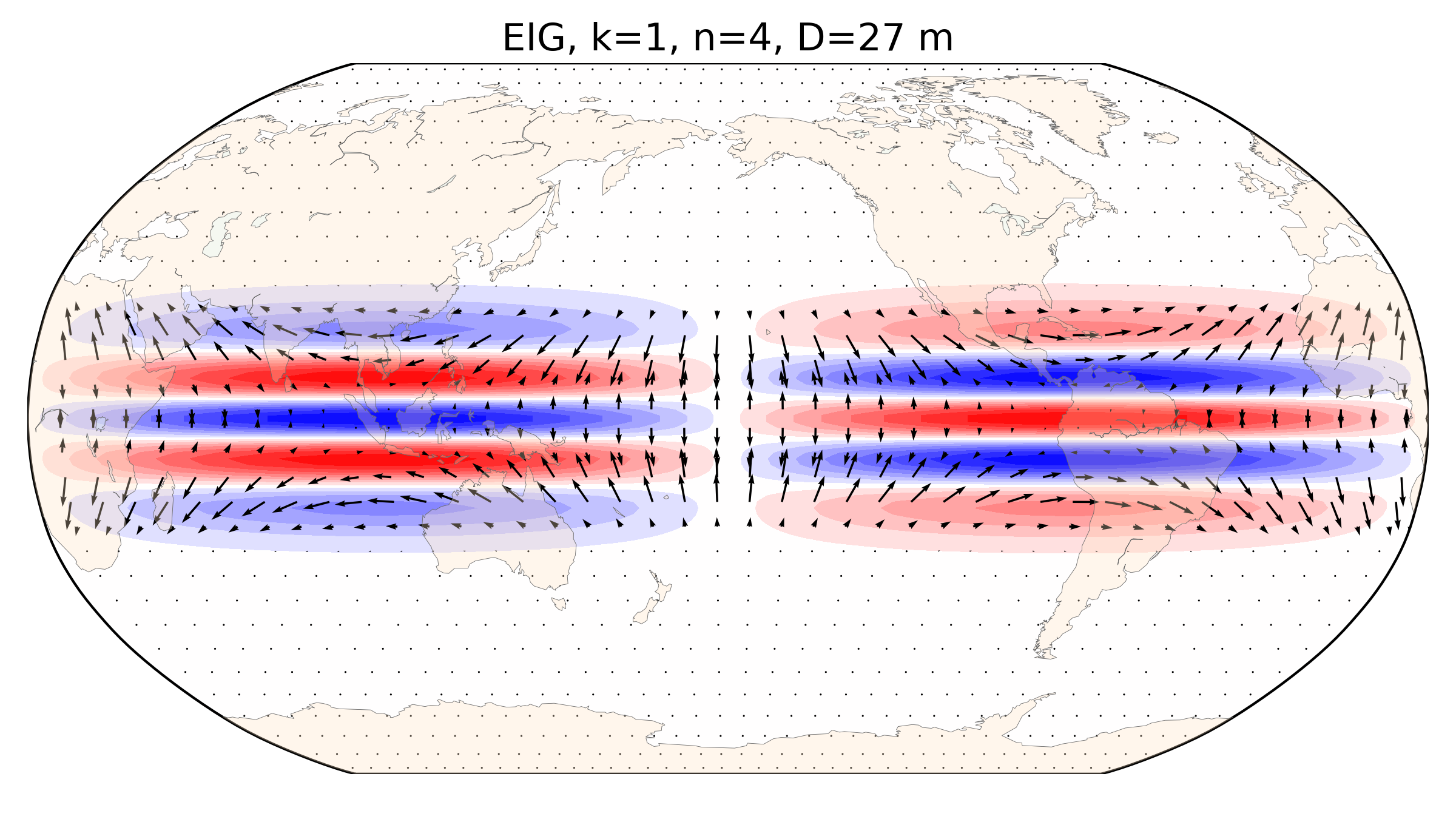
(c) Eastward-propagating Inertia-gravity Waves
Horizontal structure of the slowest eastward-propagating inertia-gravity (EIG) mode on the sphere, n=0 EIG wave. Geopotential height is scaled by the maximal height. Colours vary between -1 and +1 with positive/negative perturbations in red/blue. Wind components are scaled by the maximal wind speed.
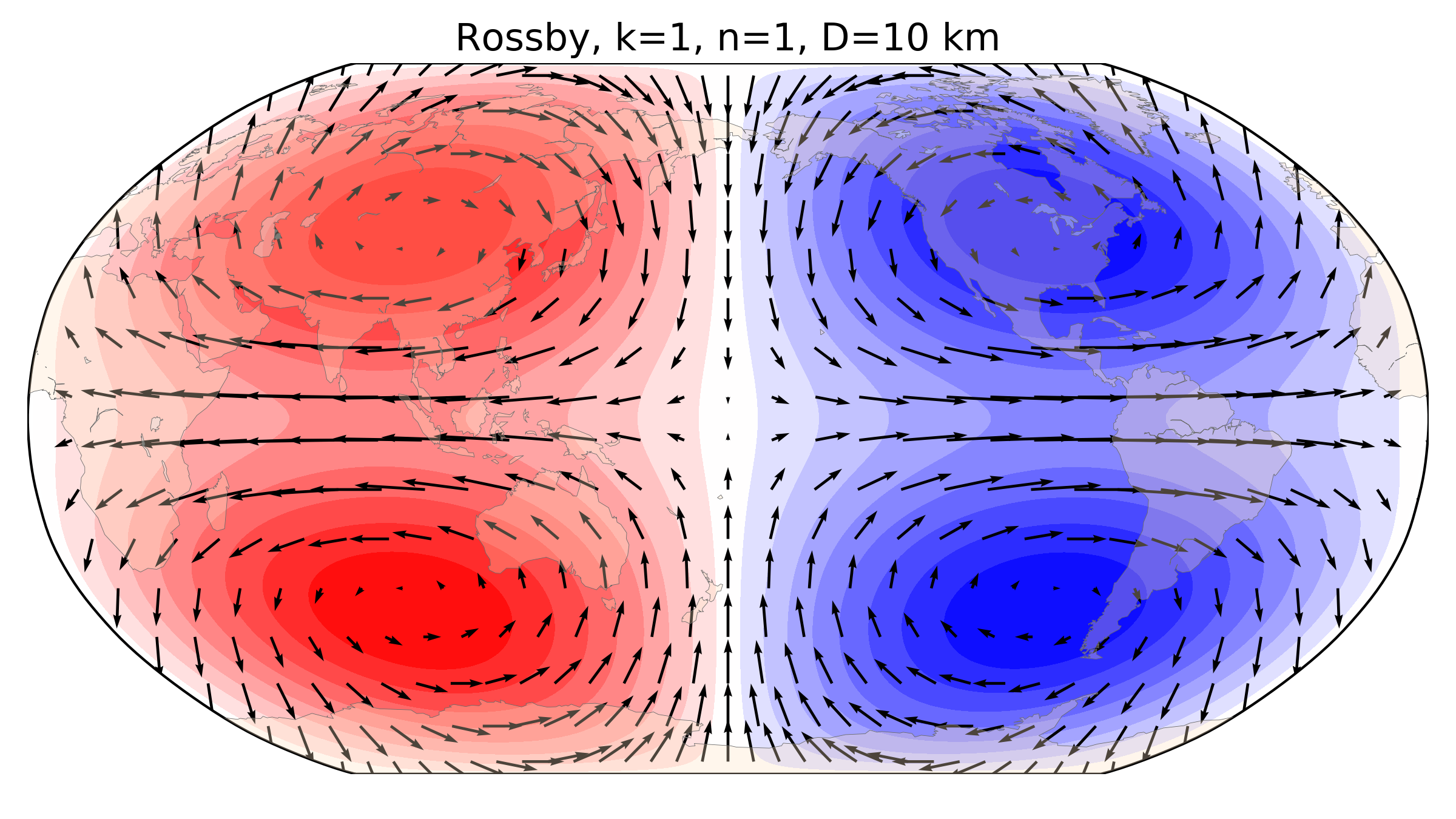
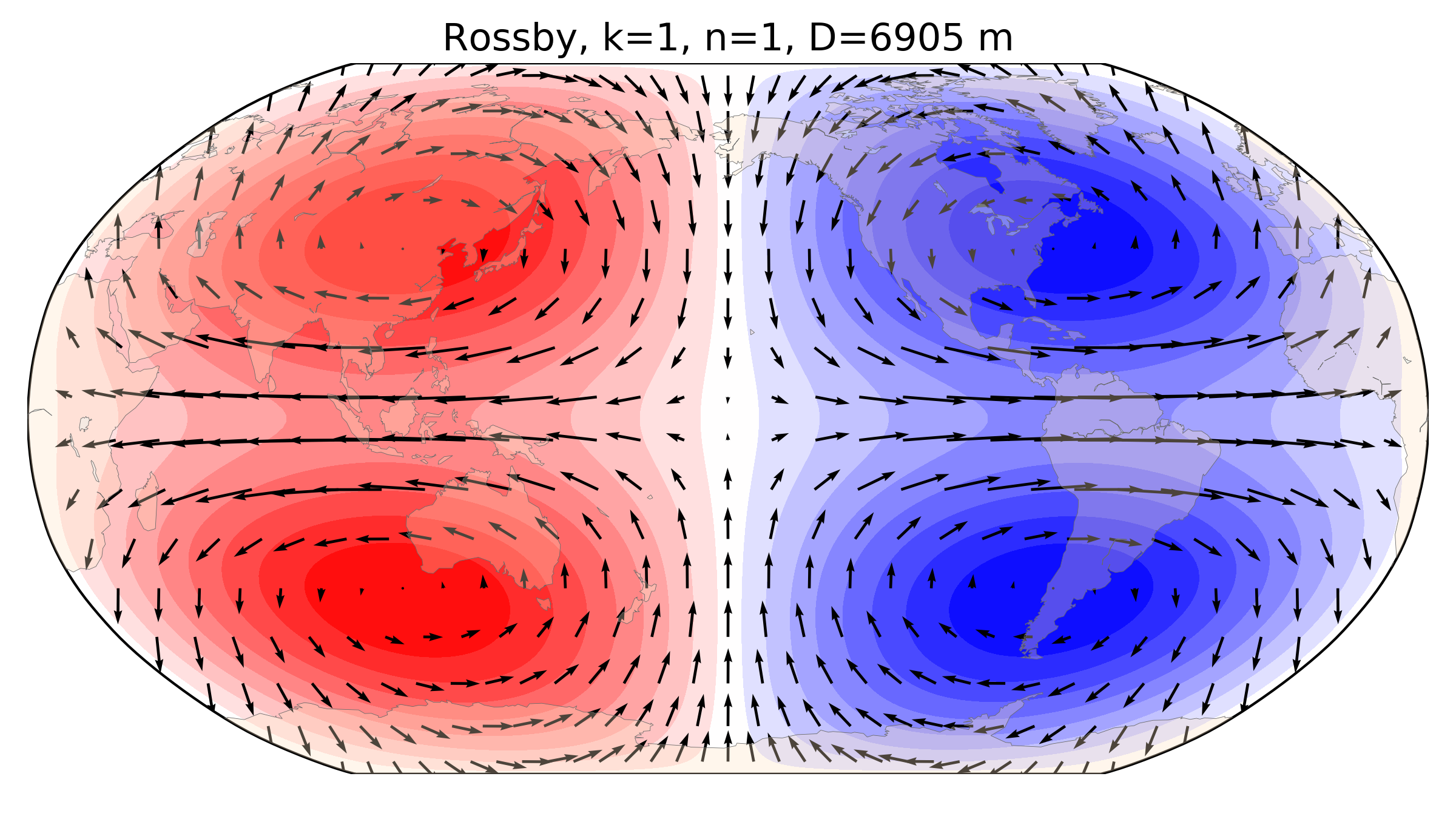
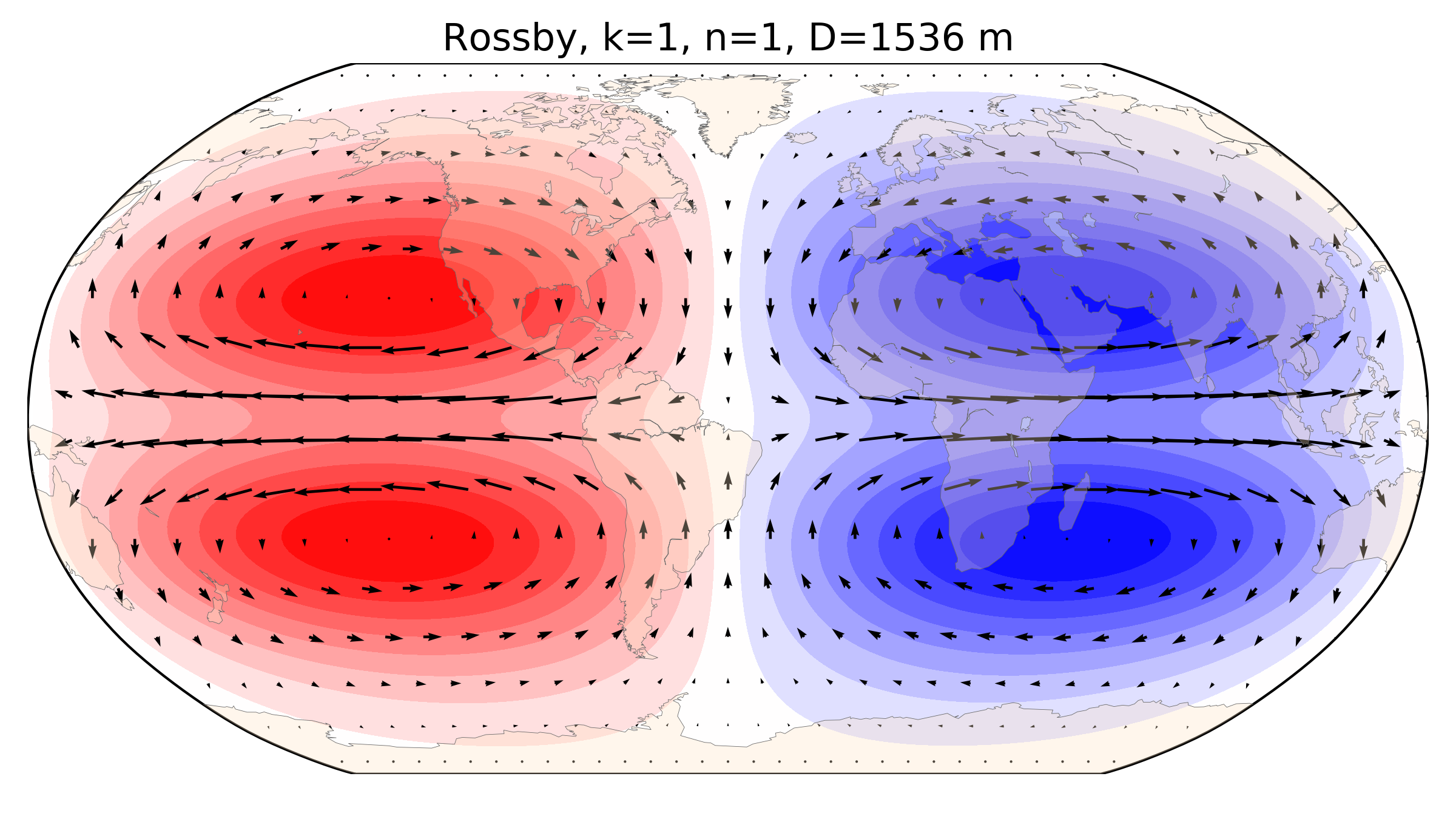
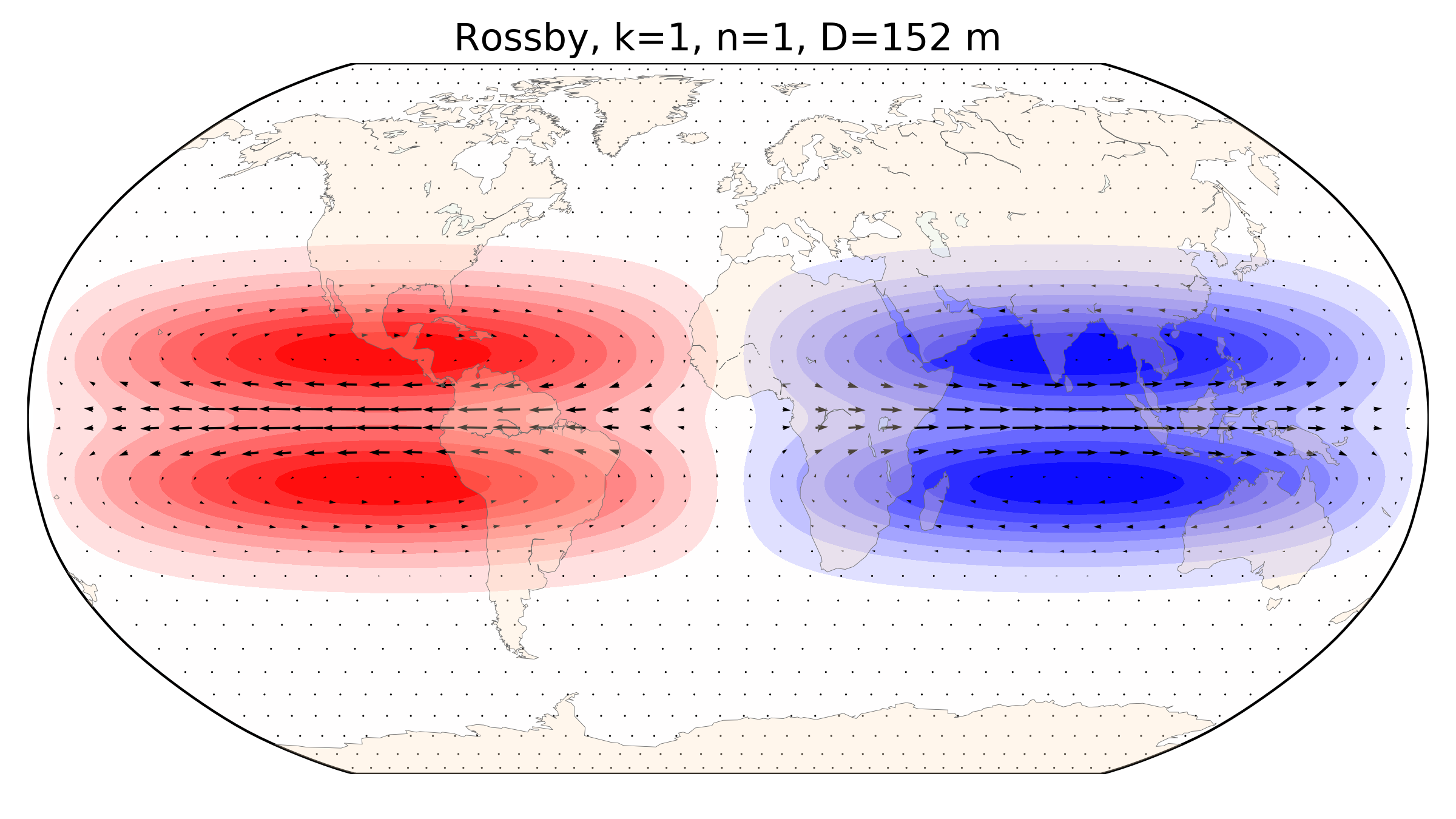
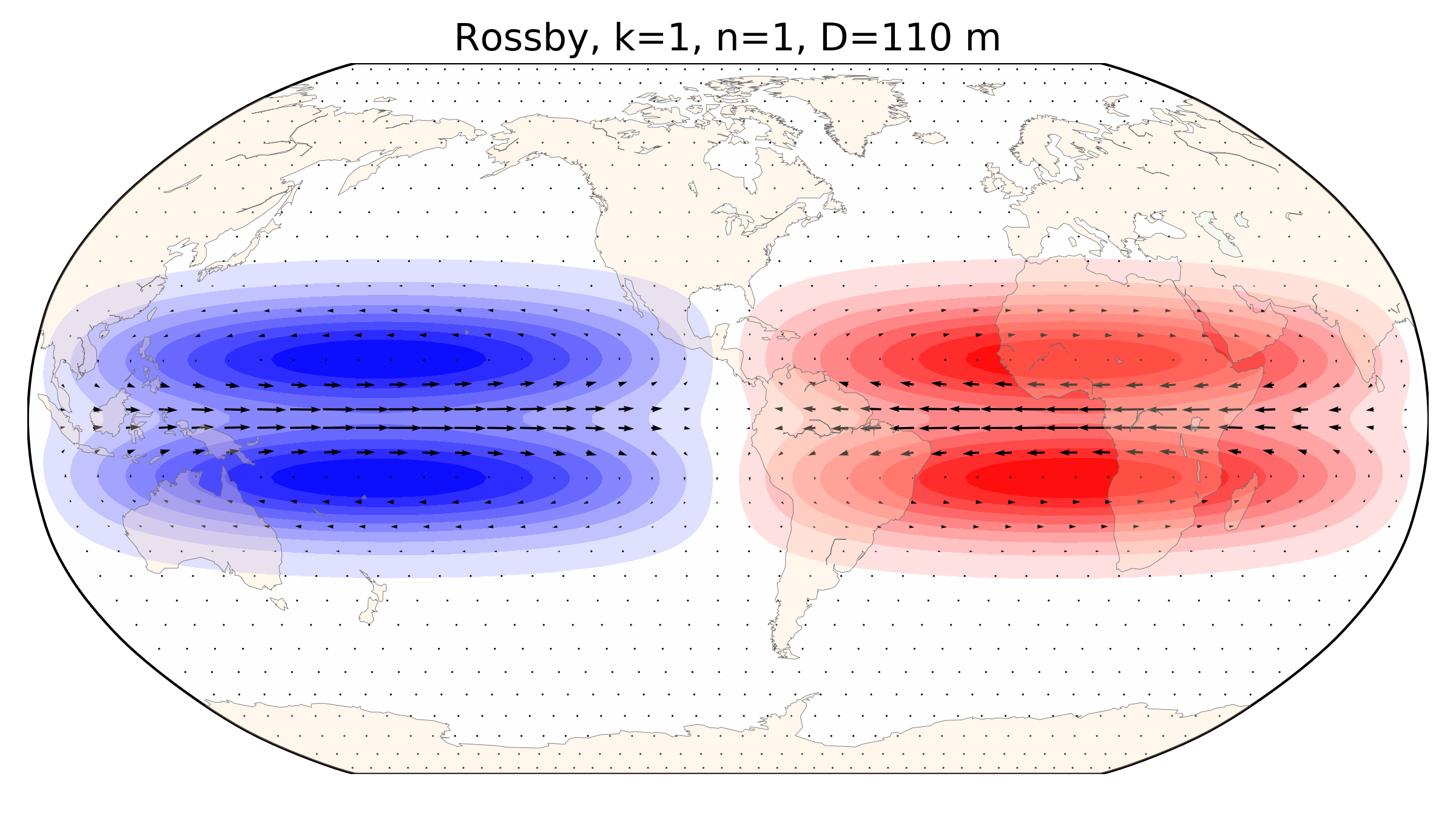
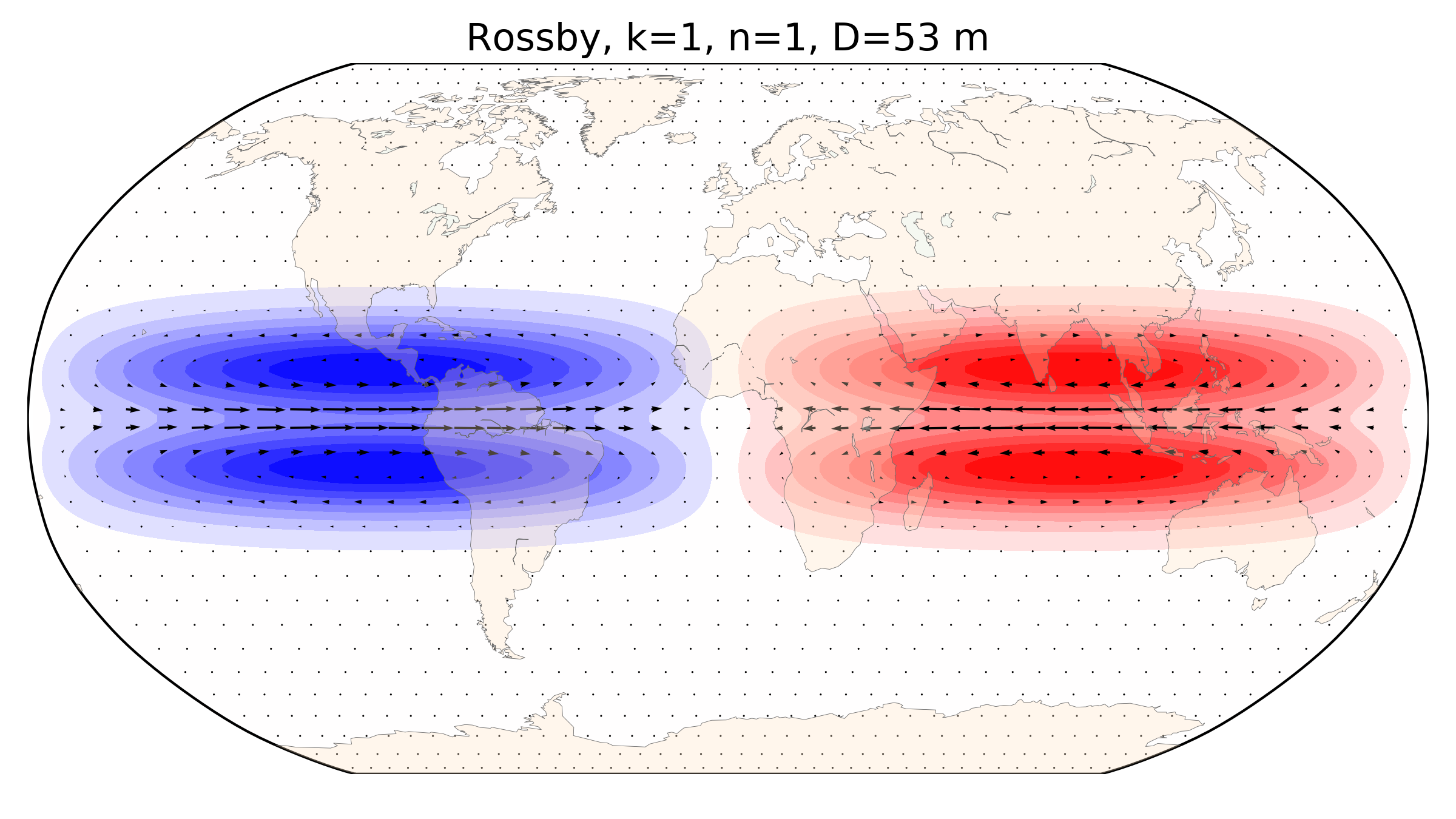
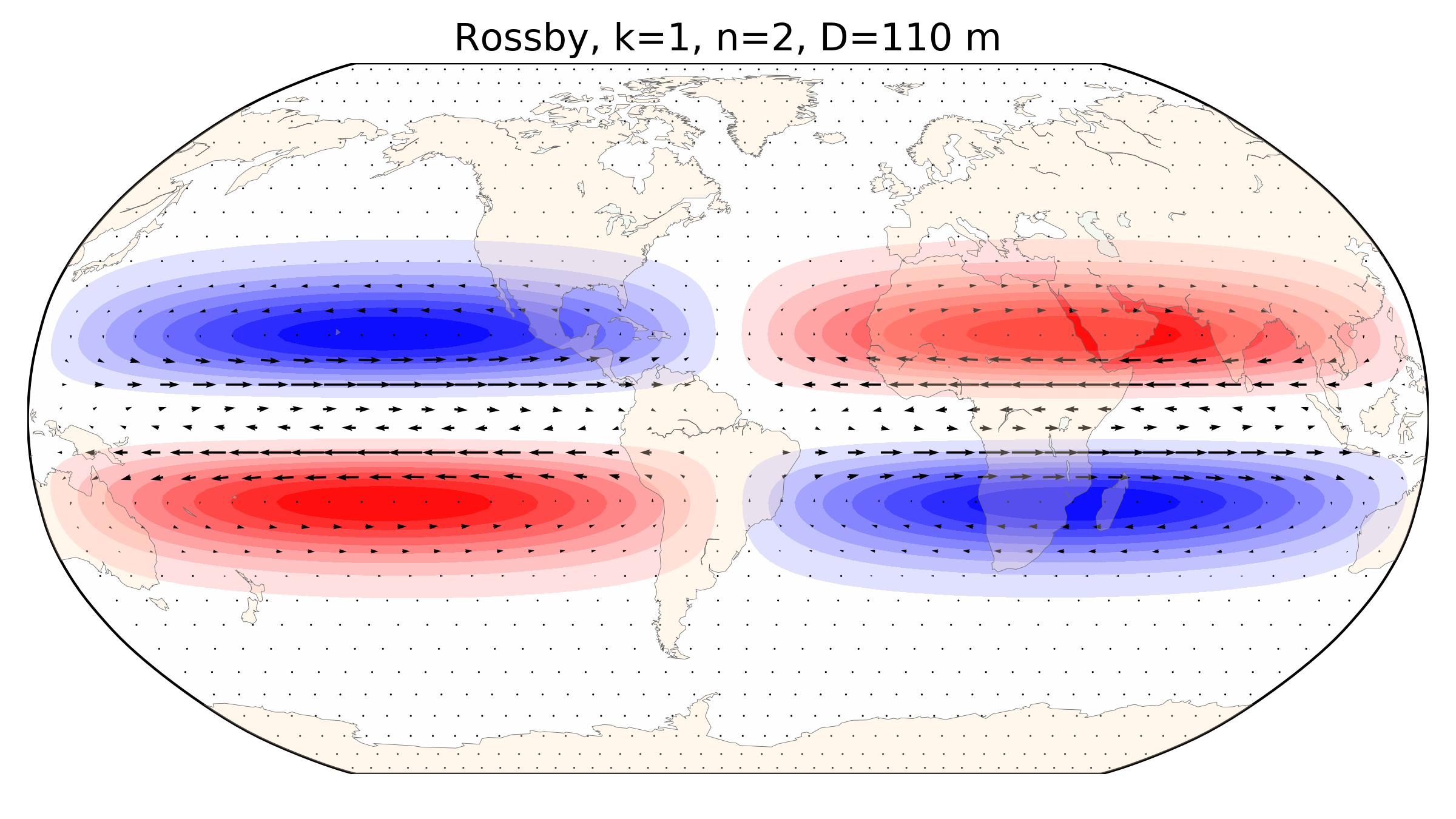
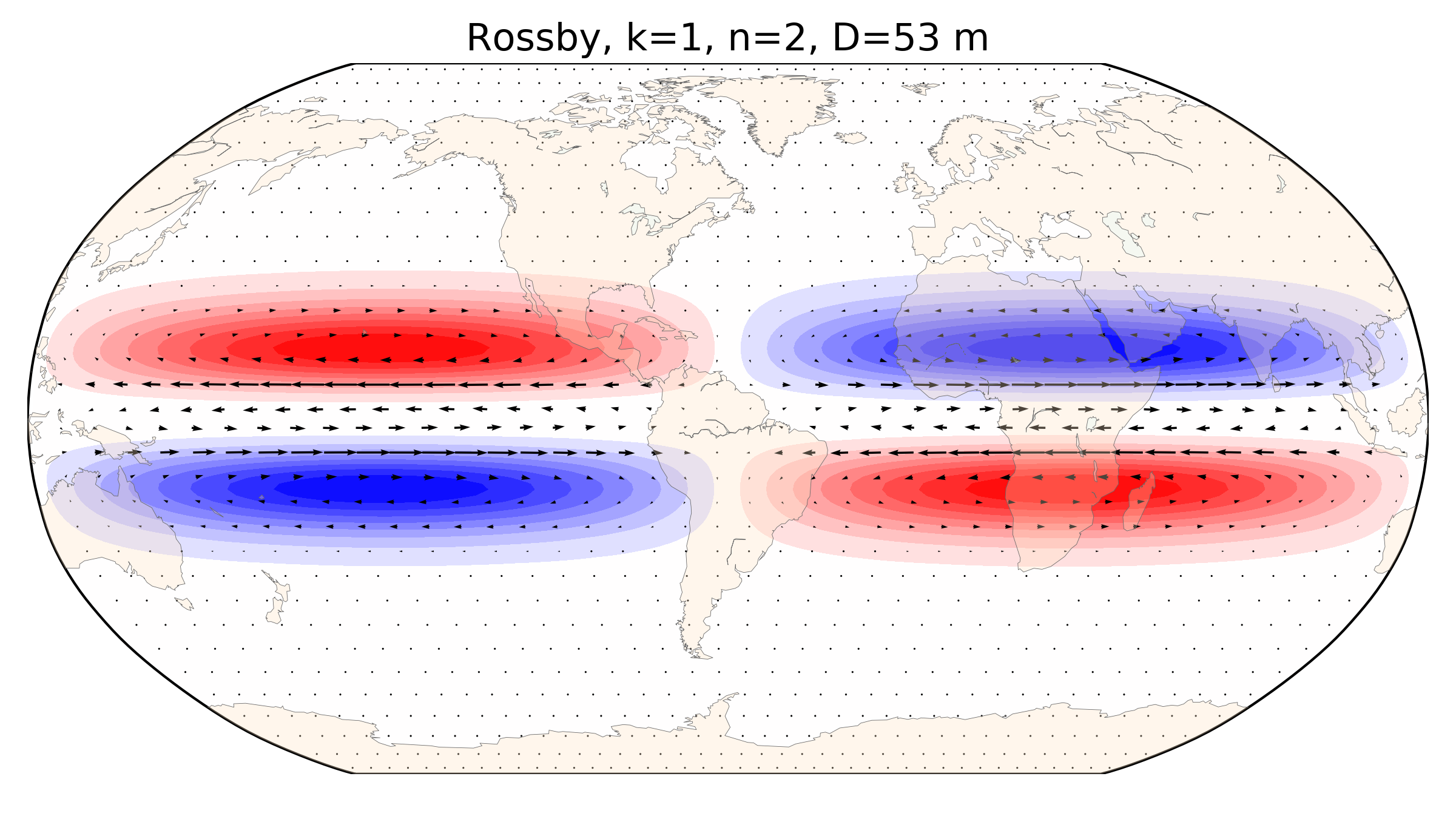
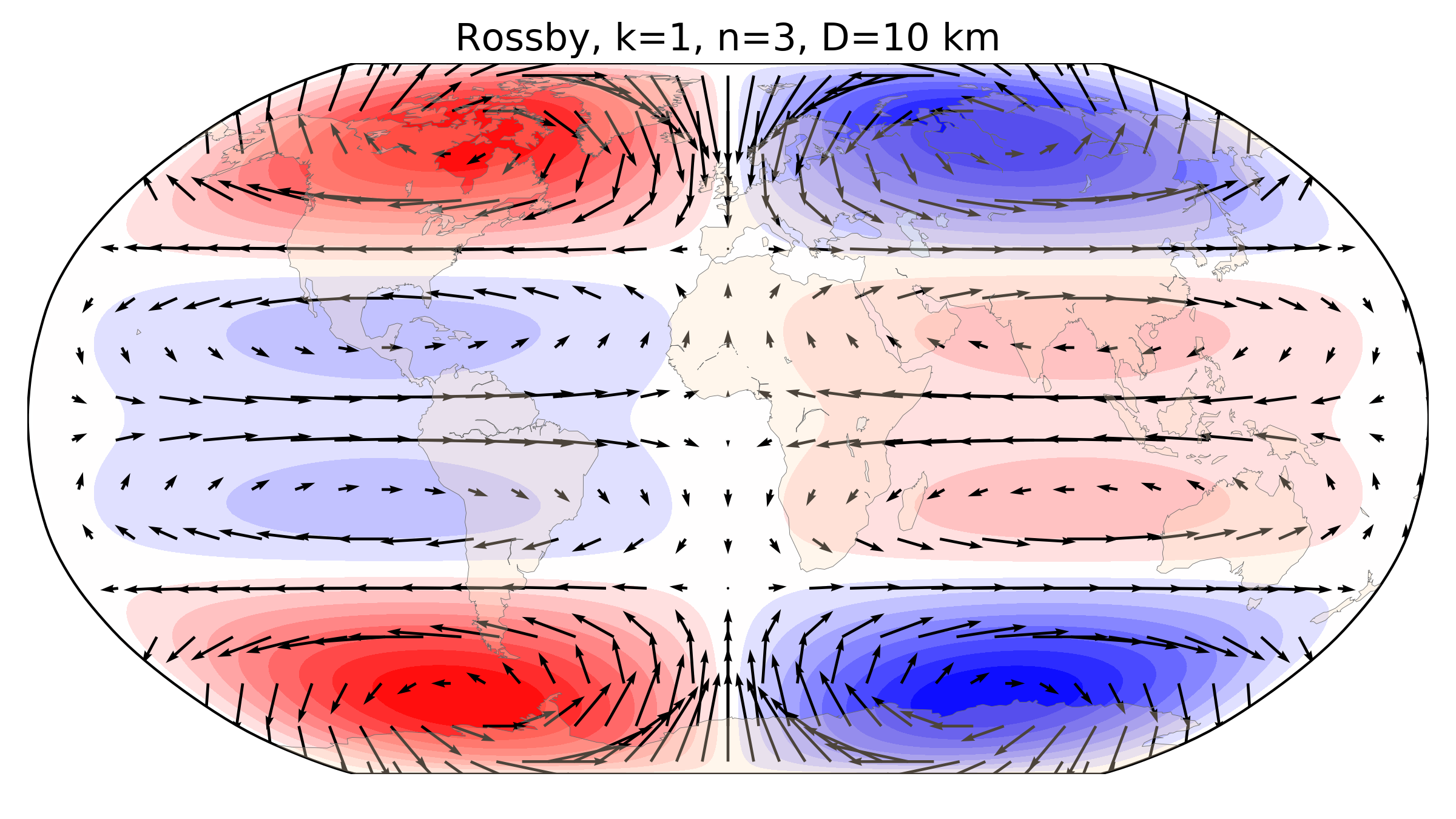
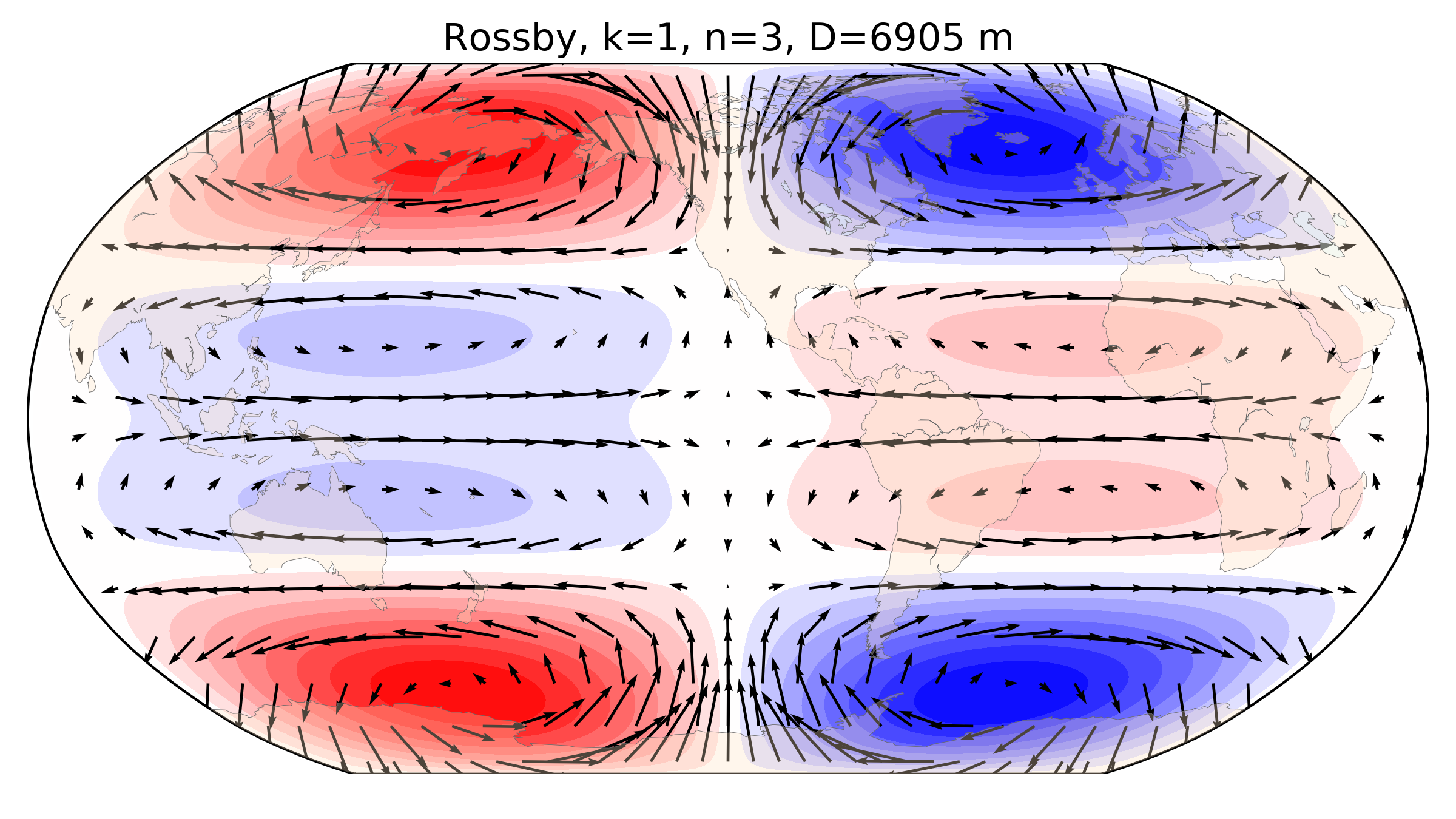
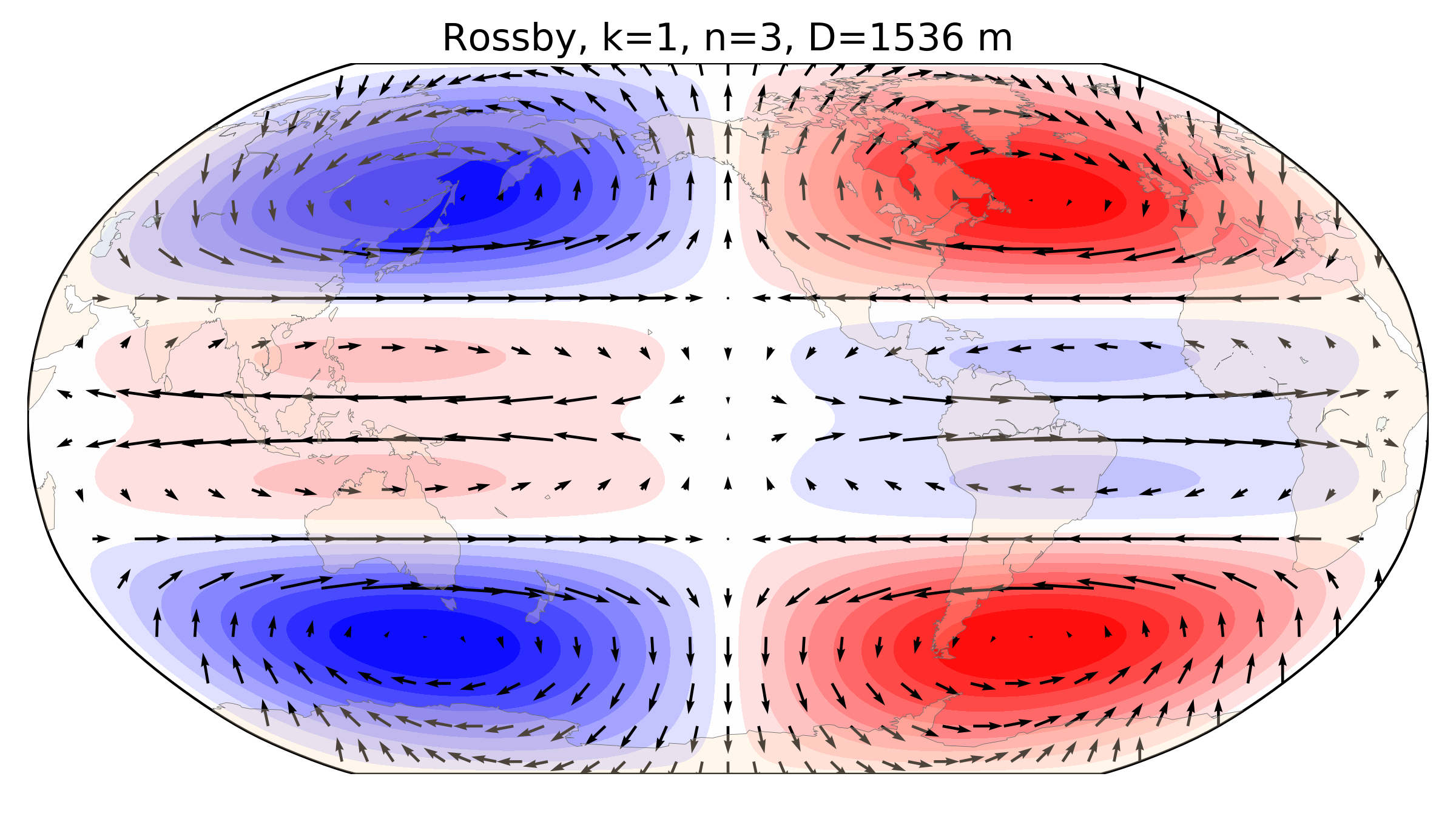
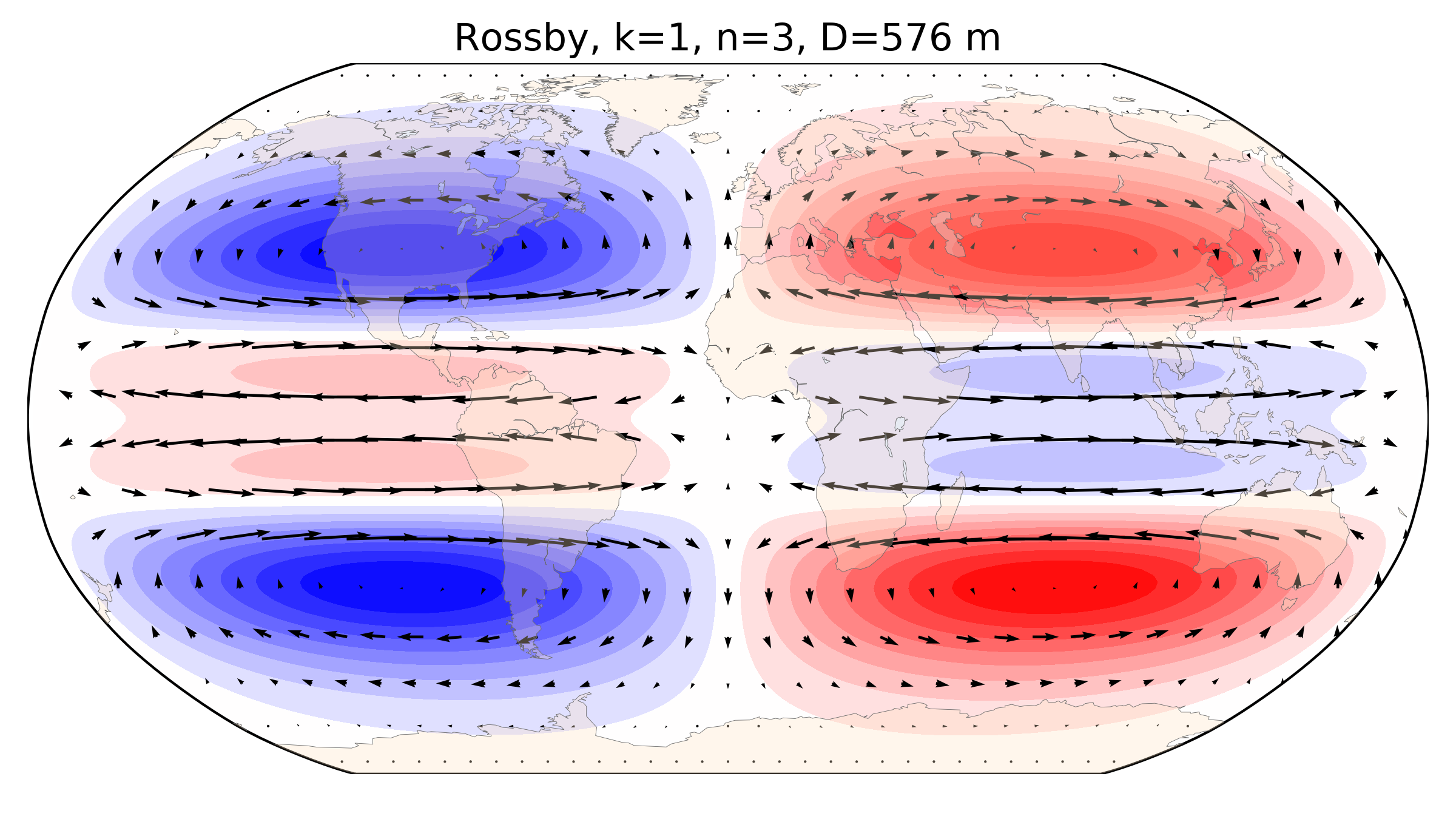
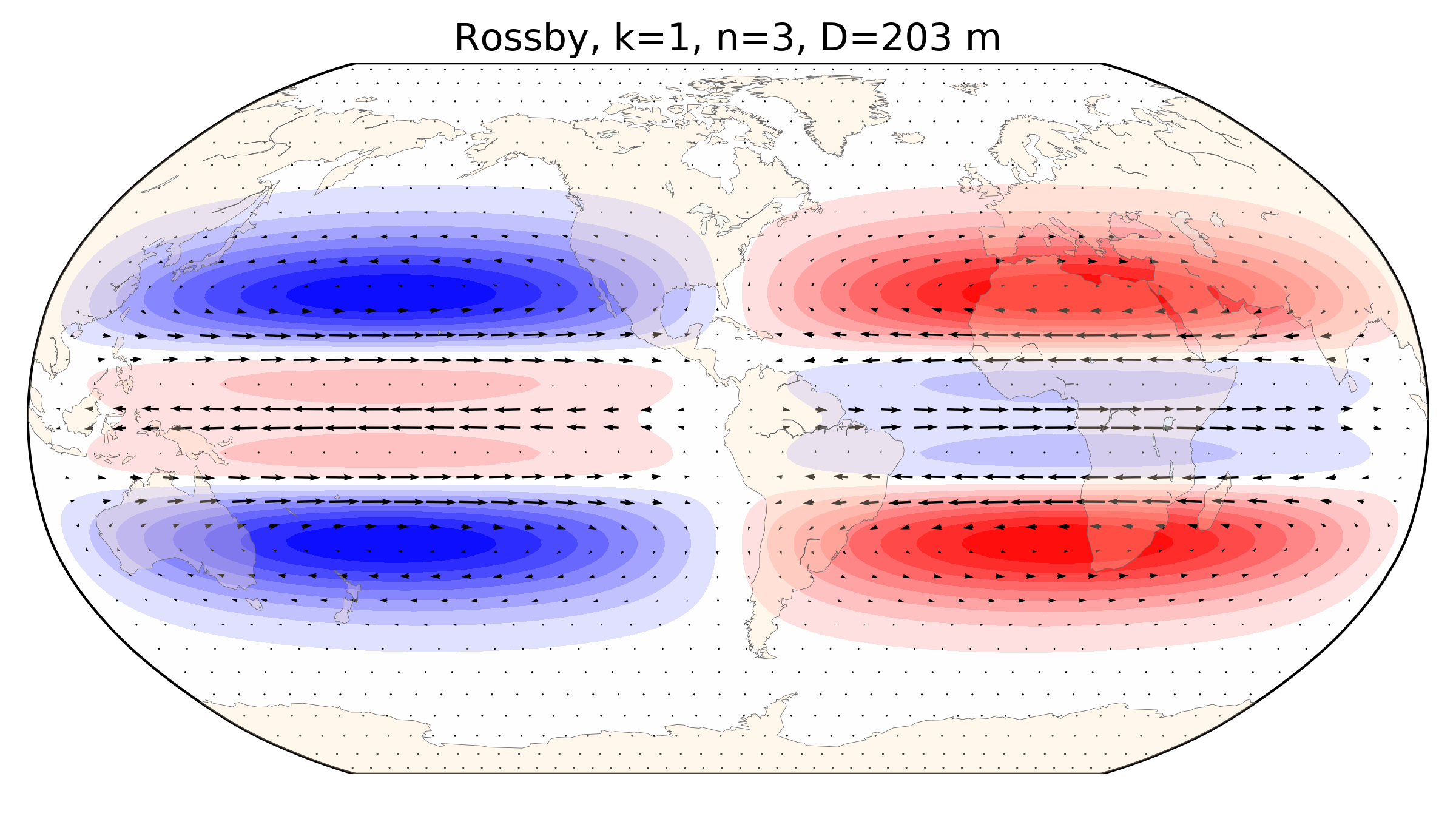
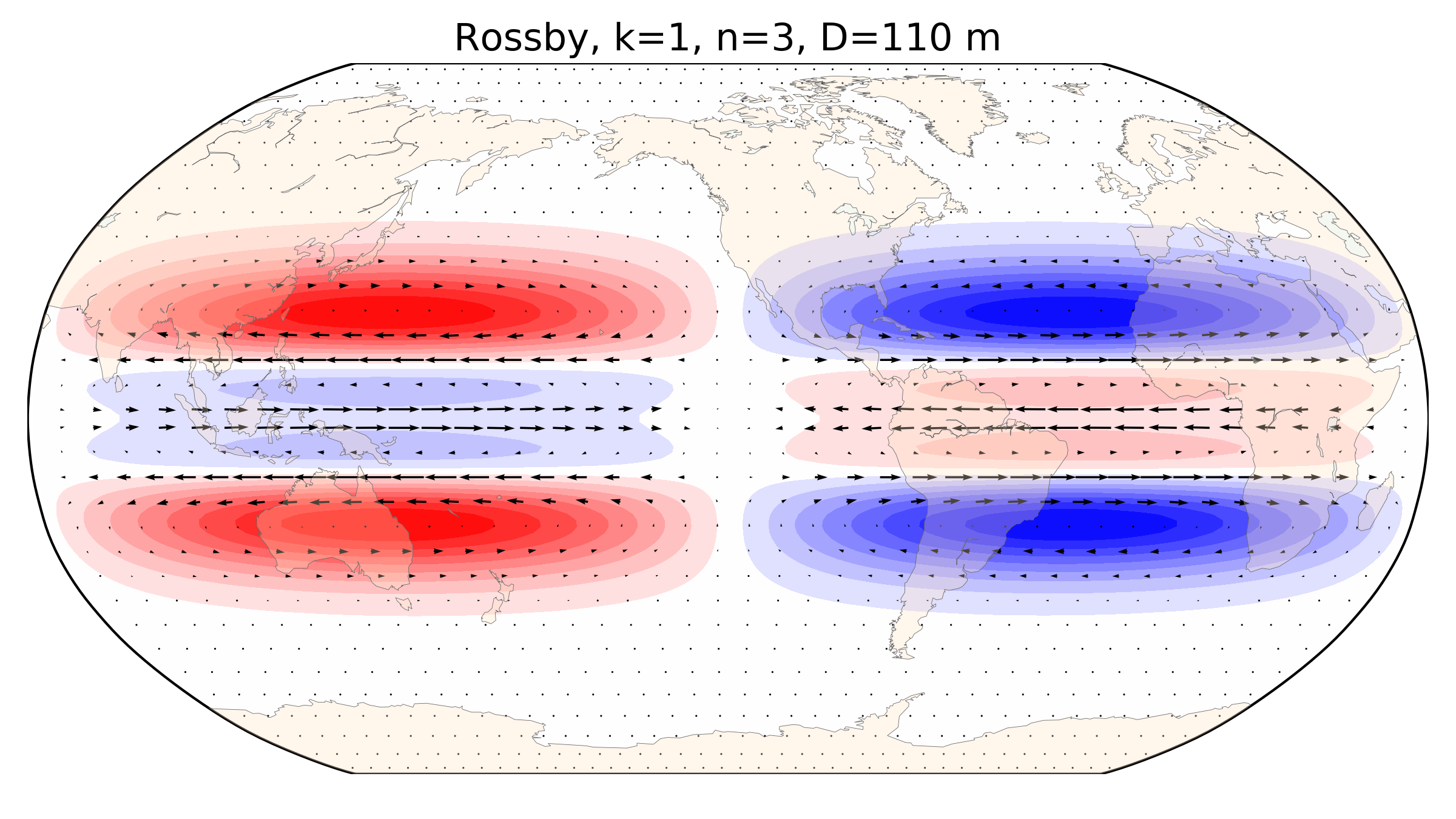
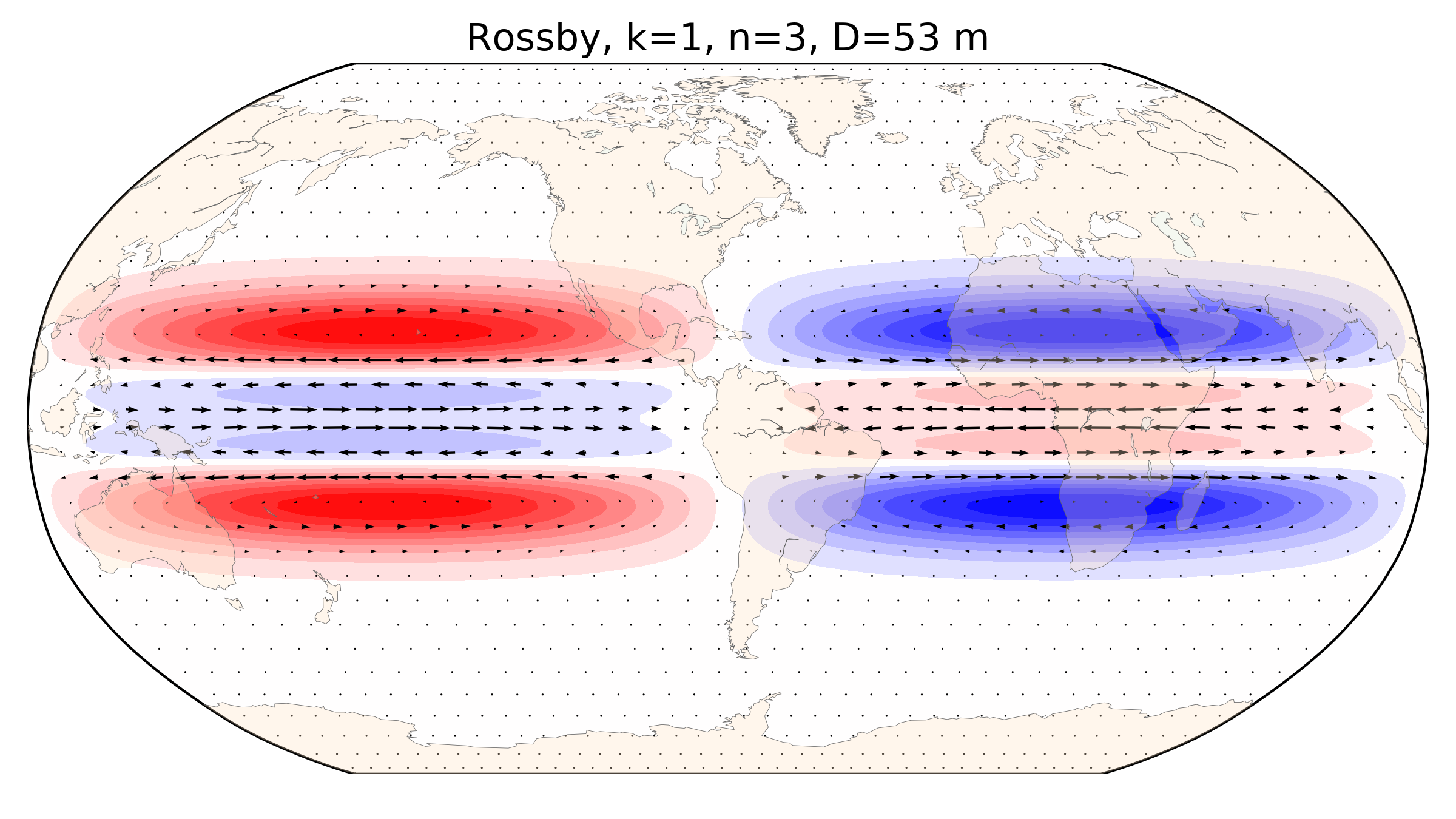
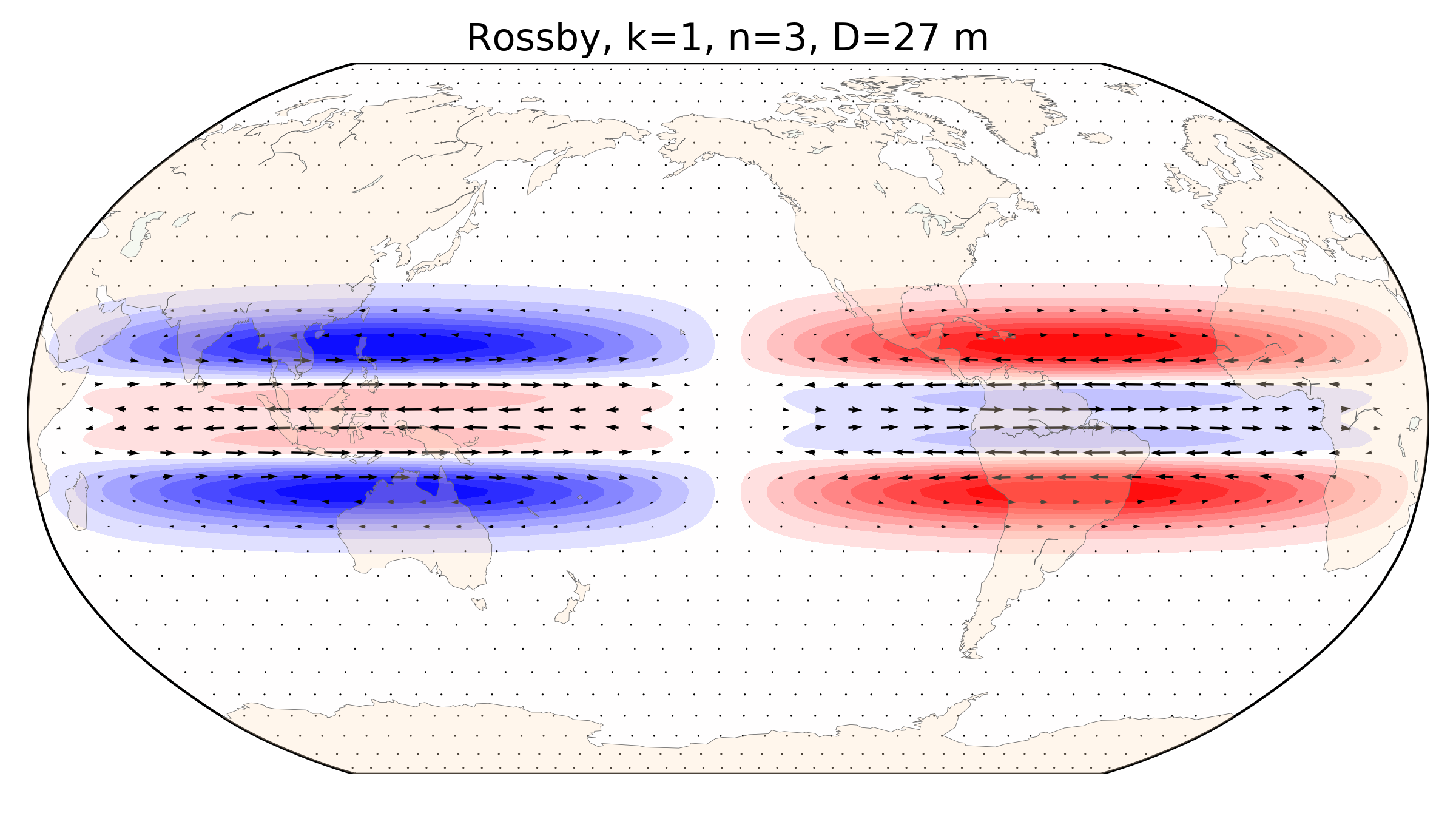
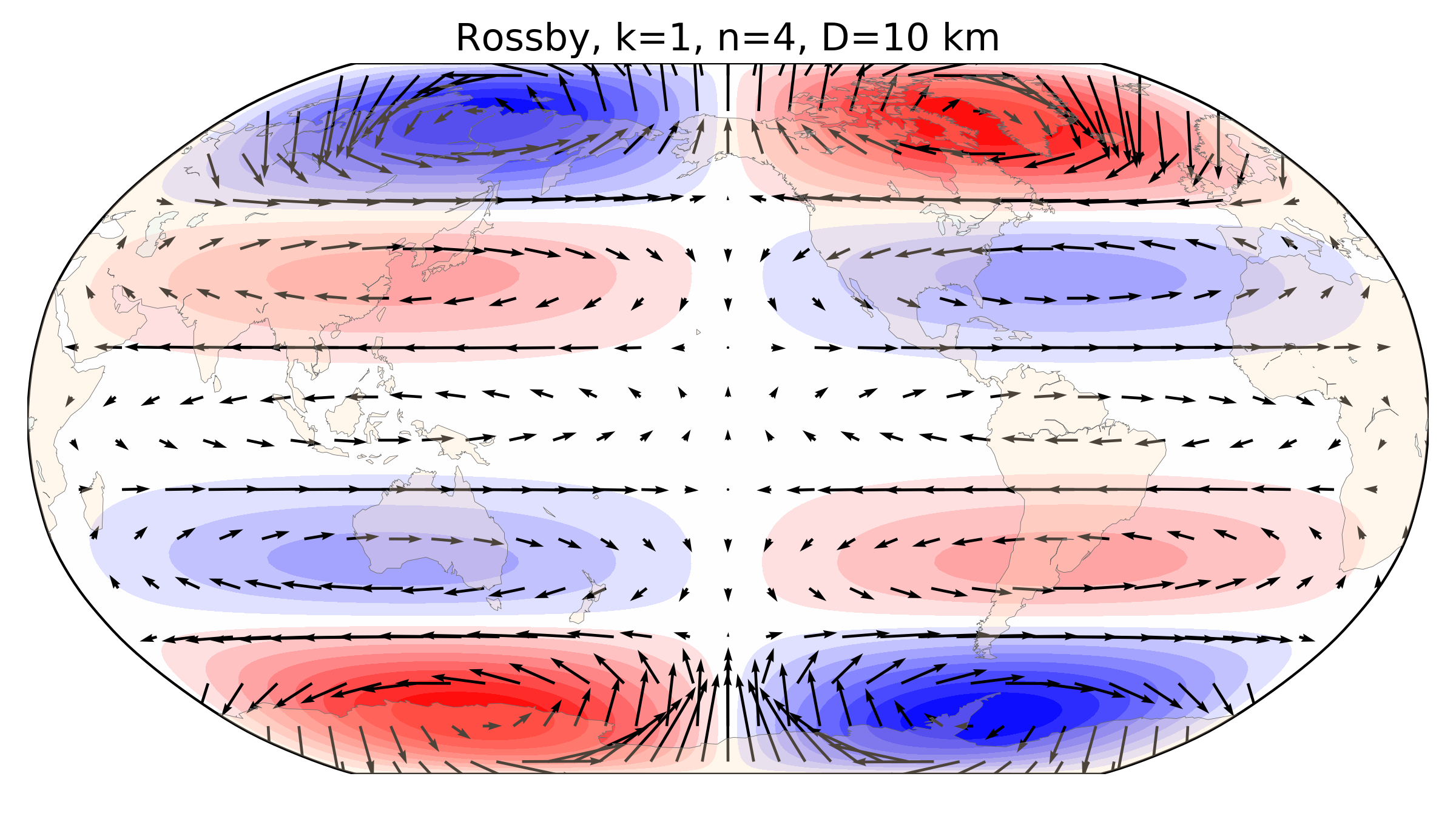
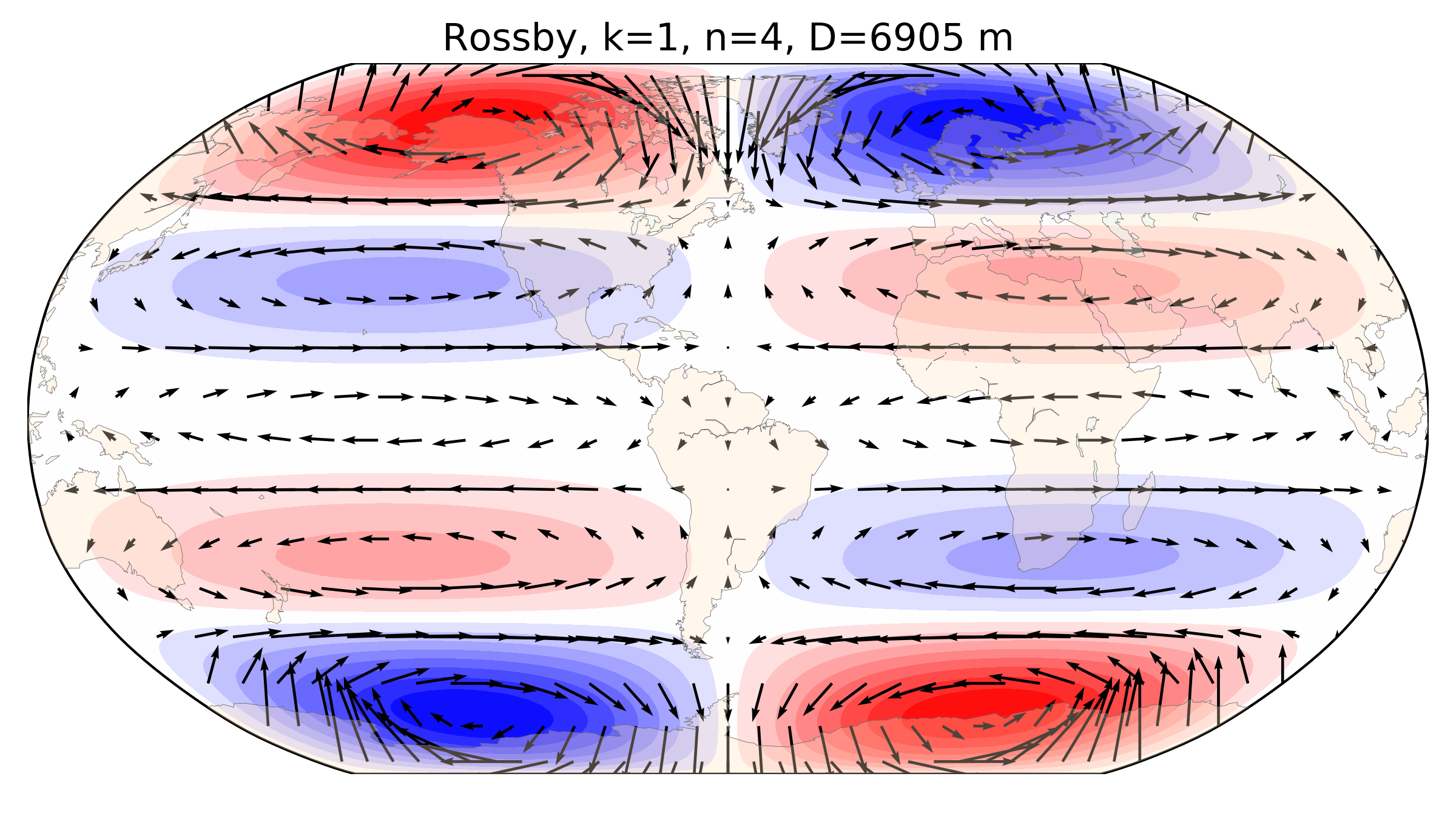
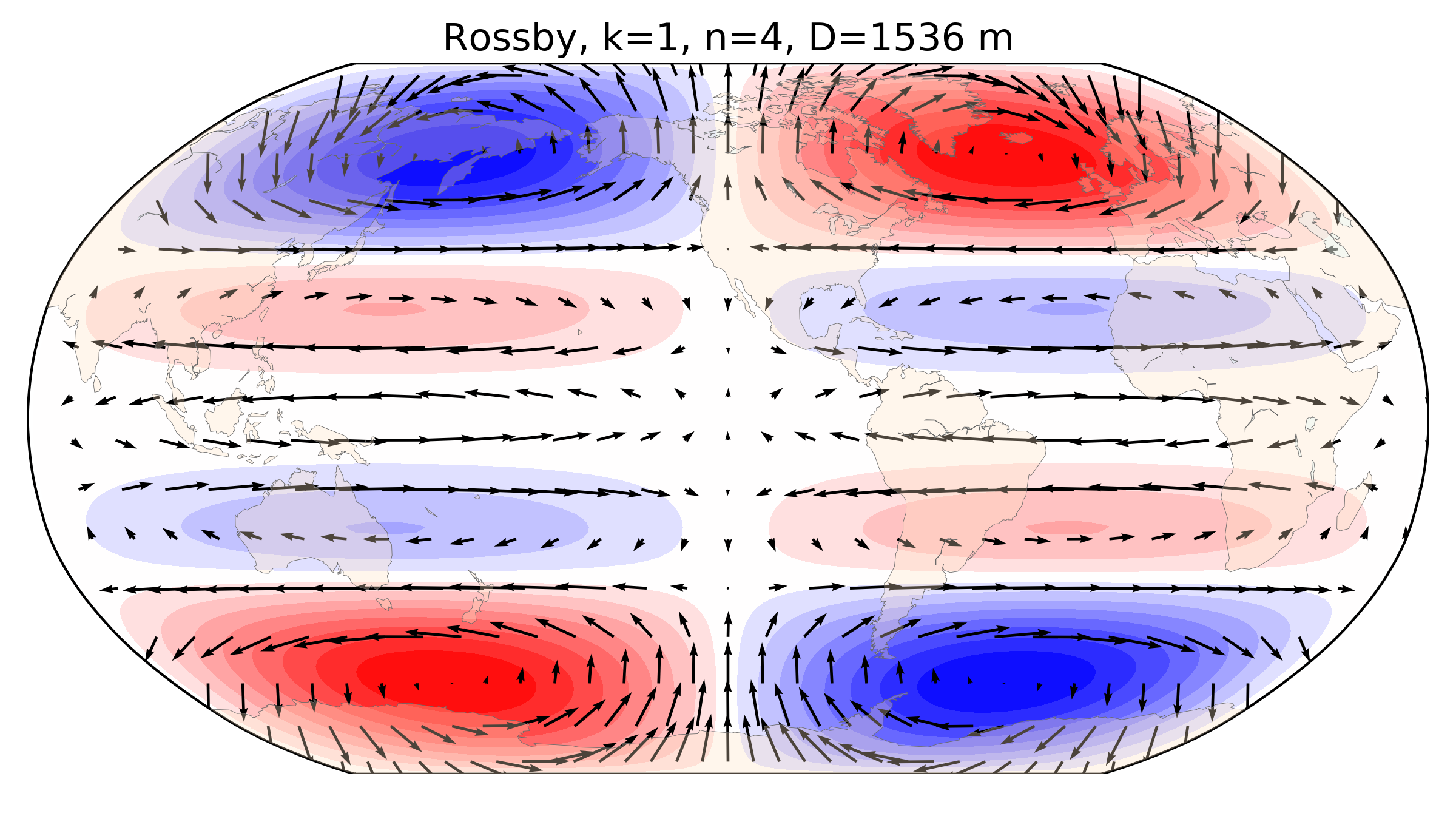
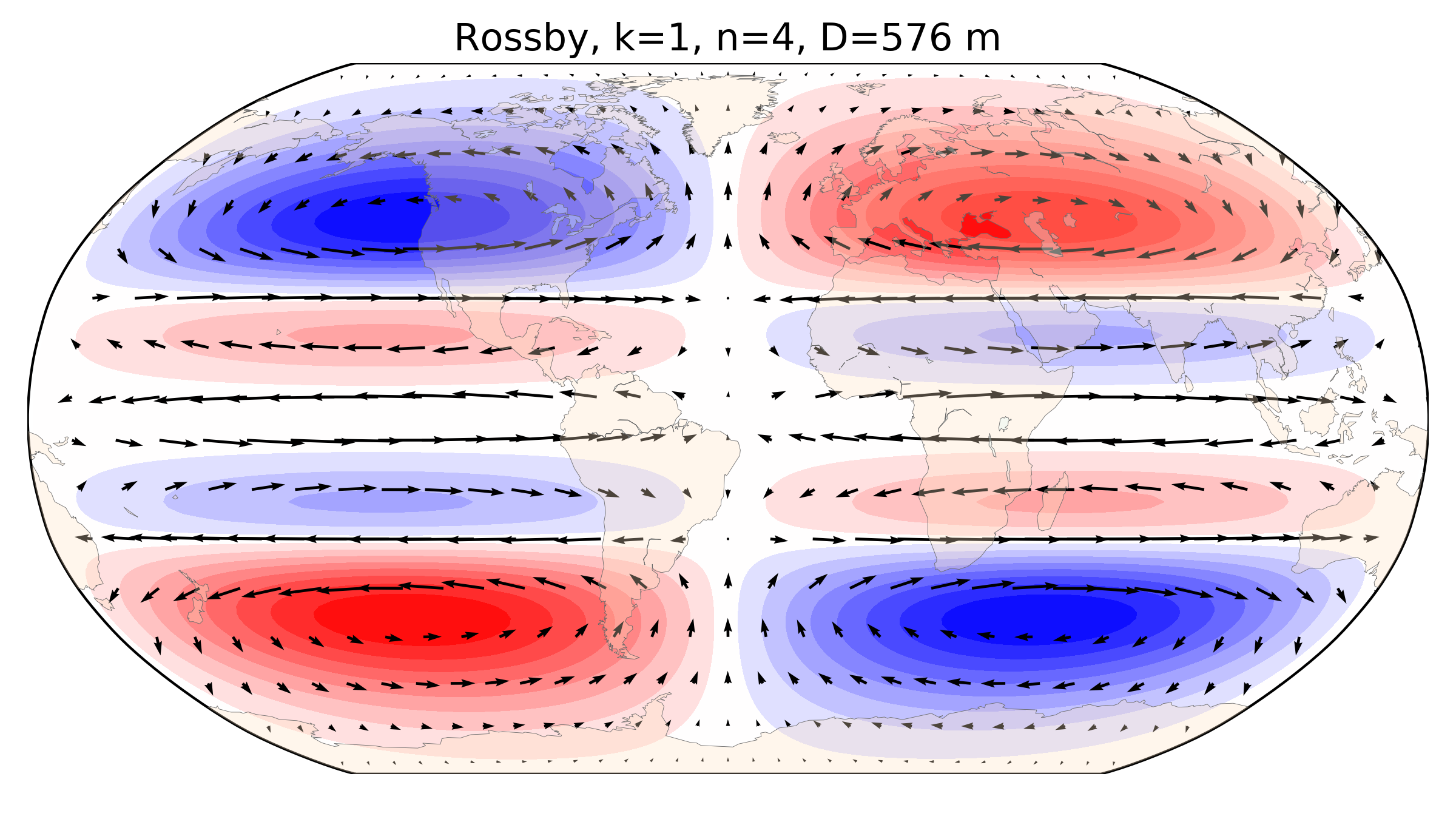
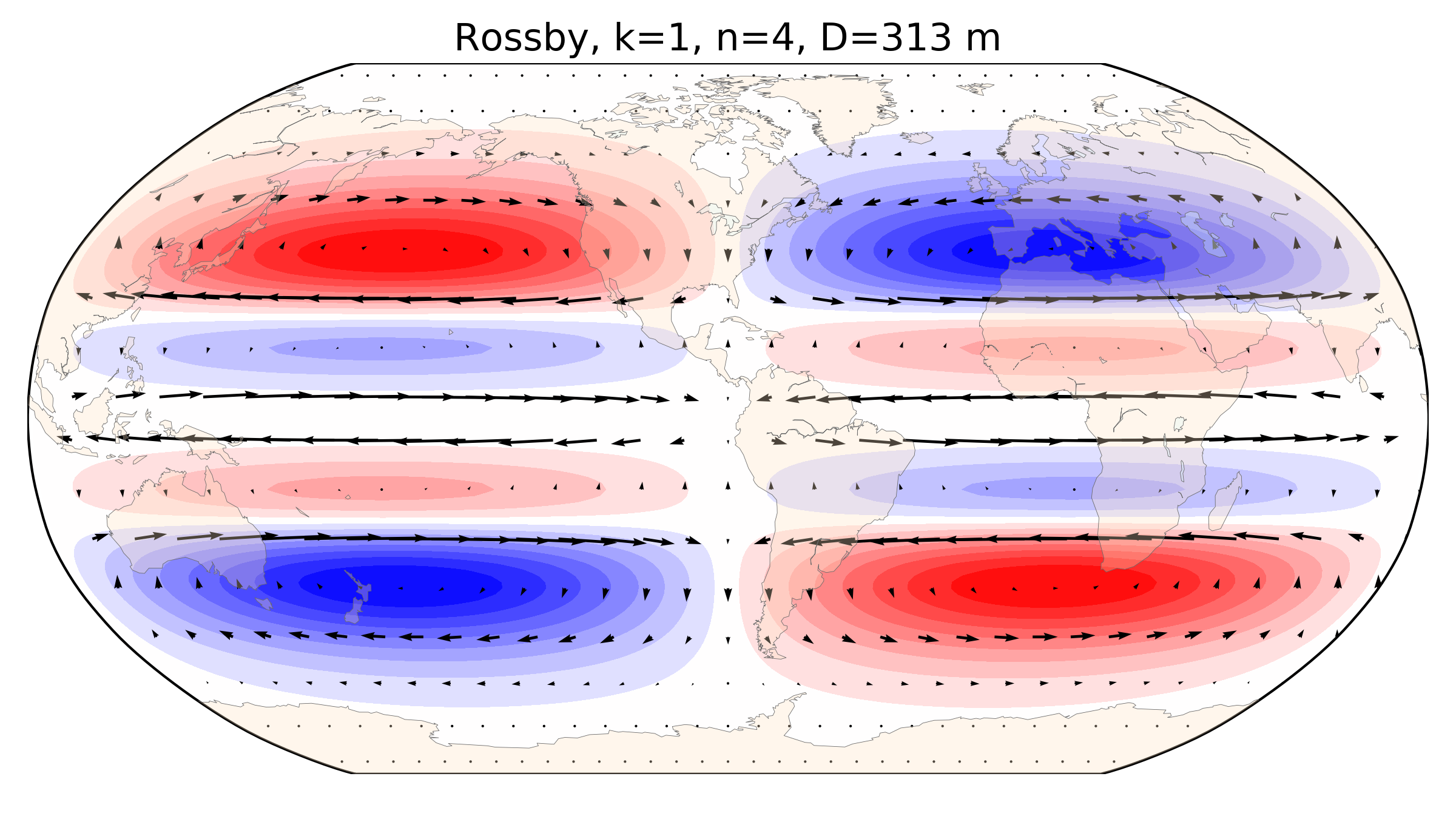
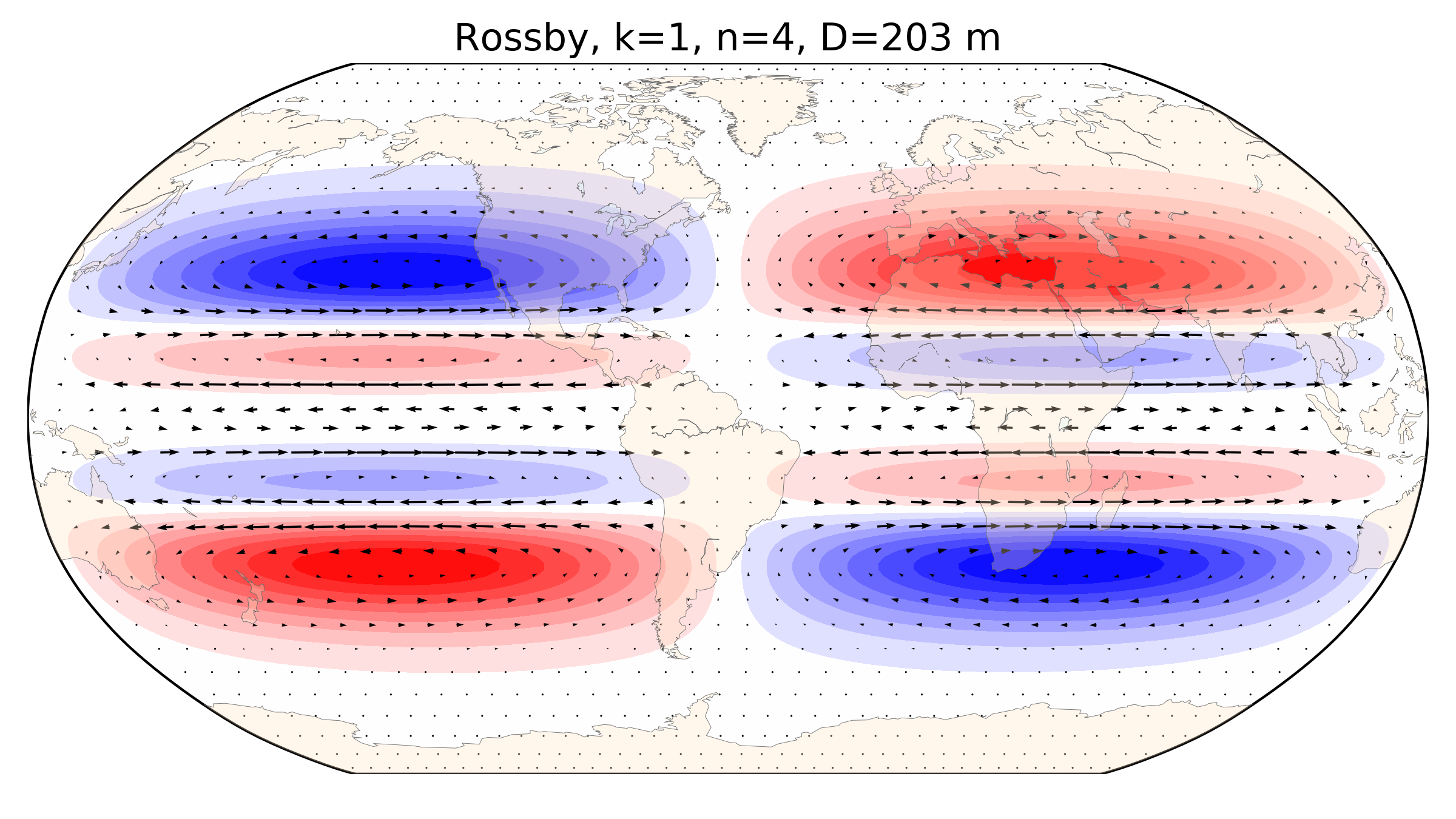
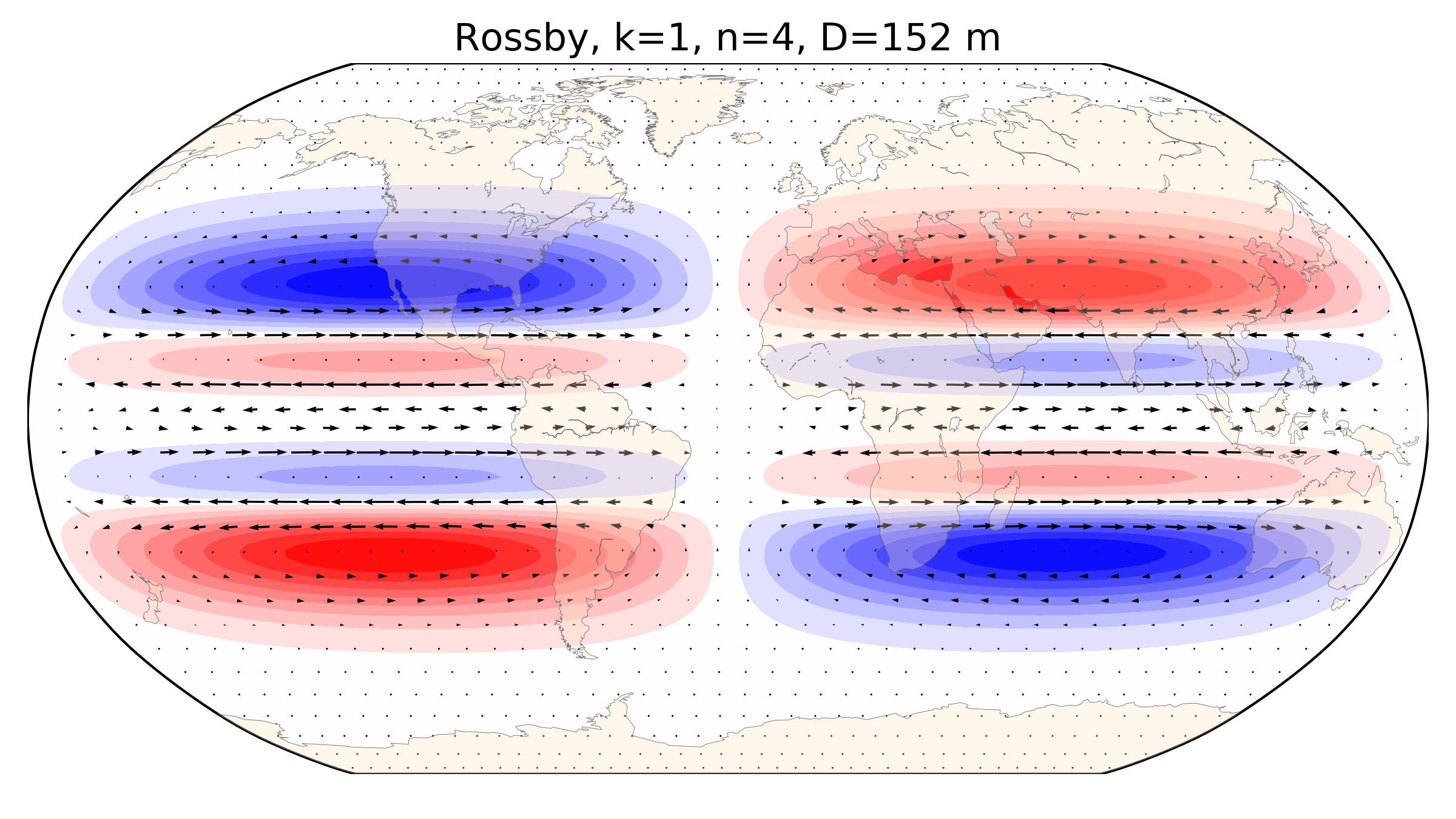
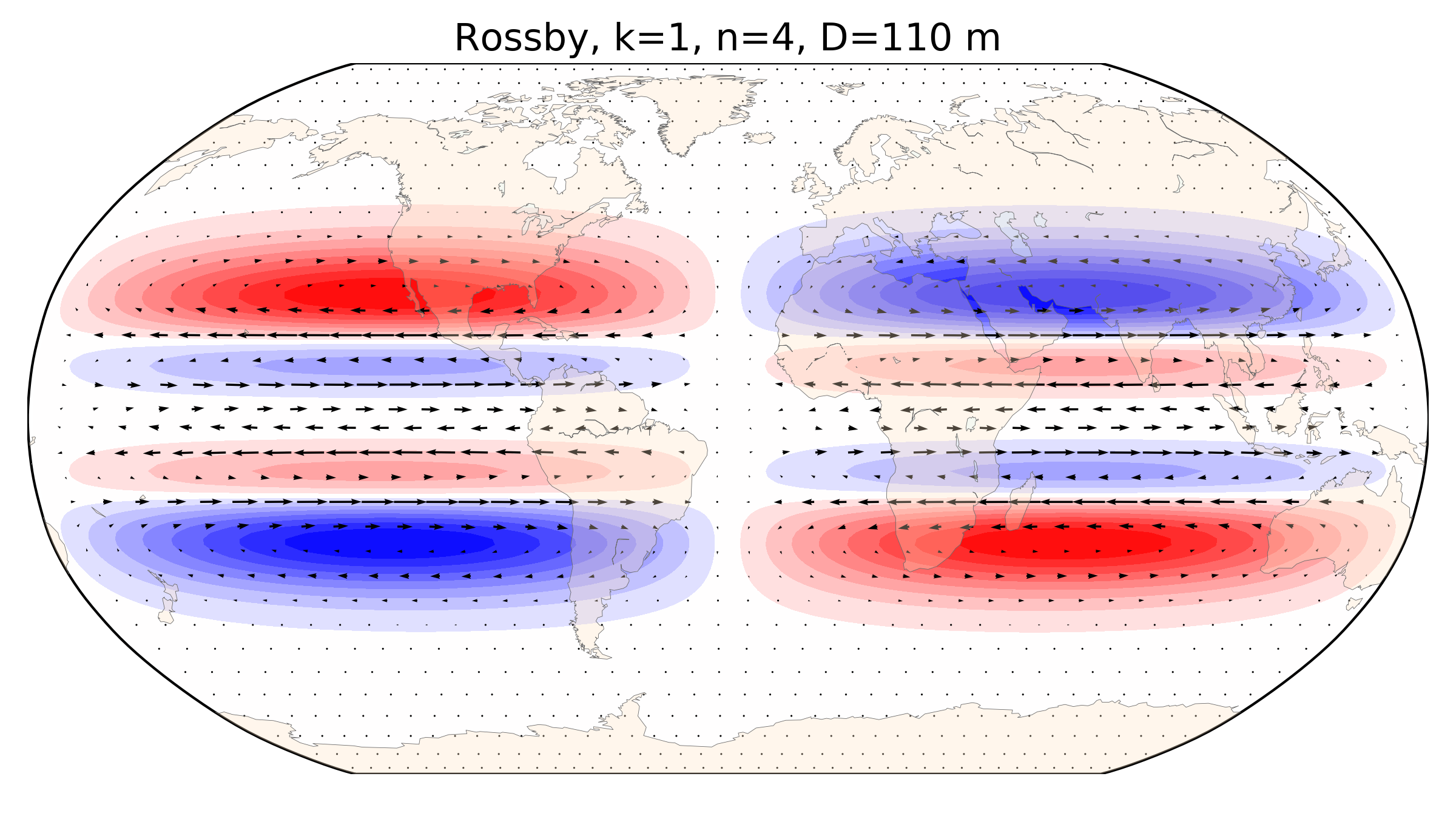
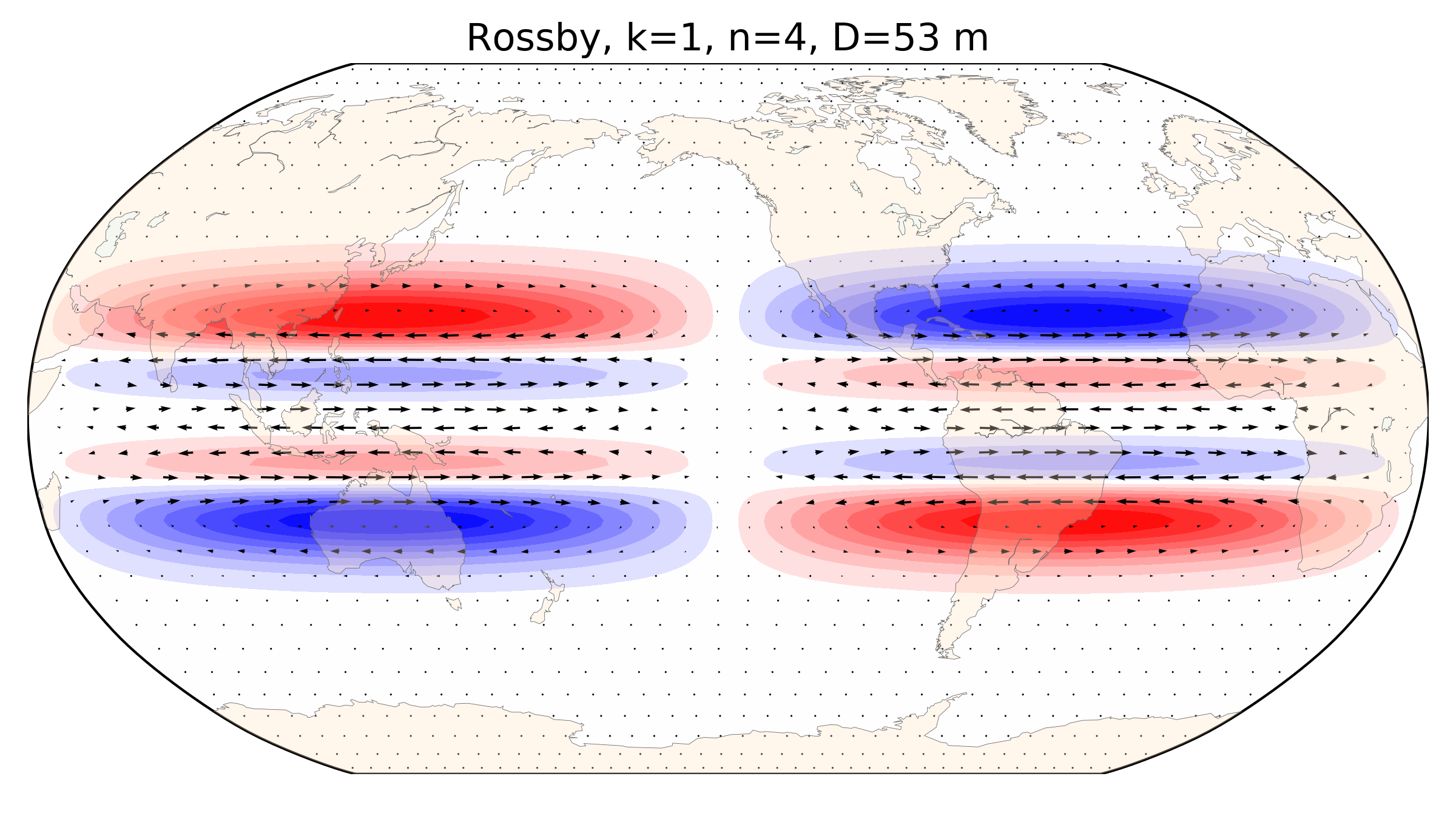
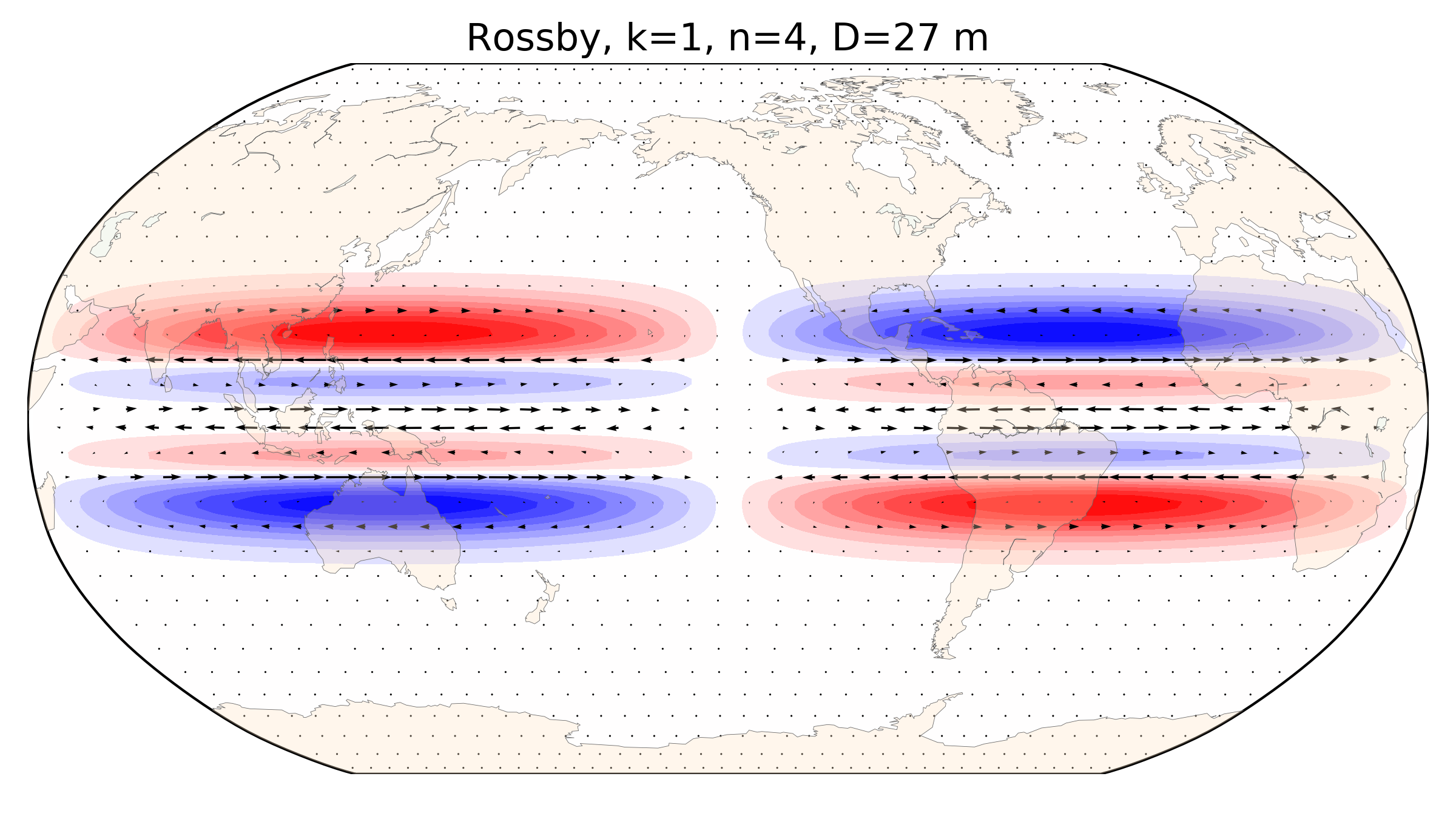
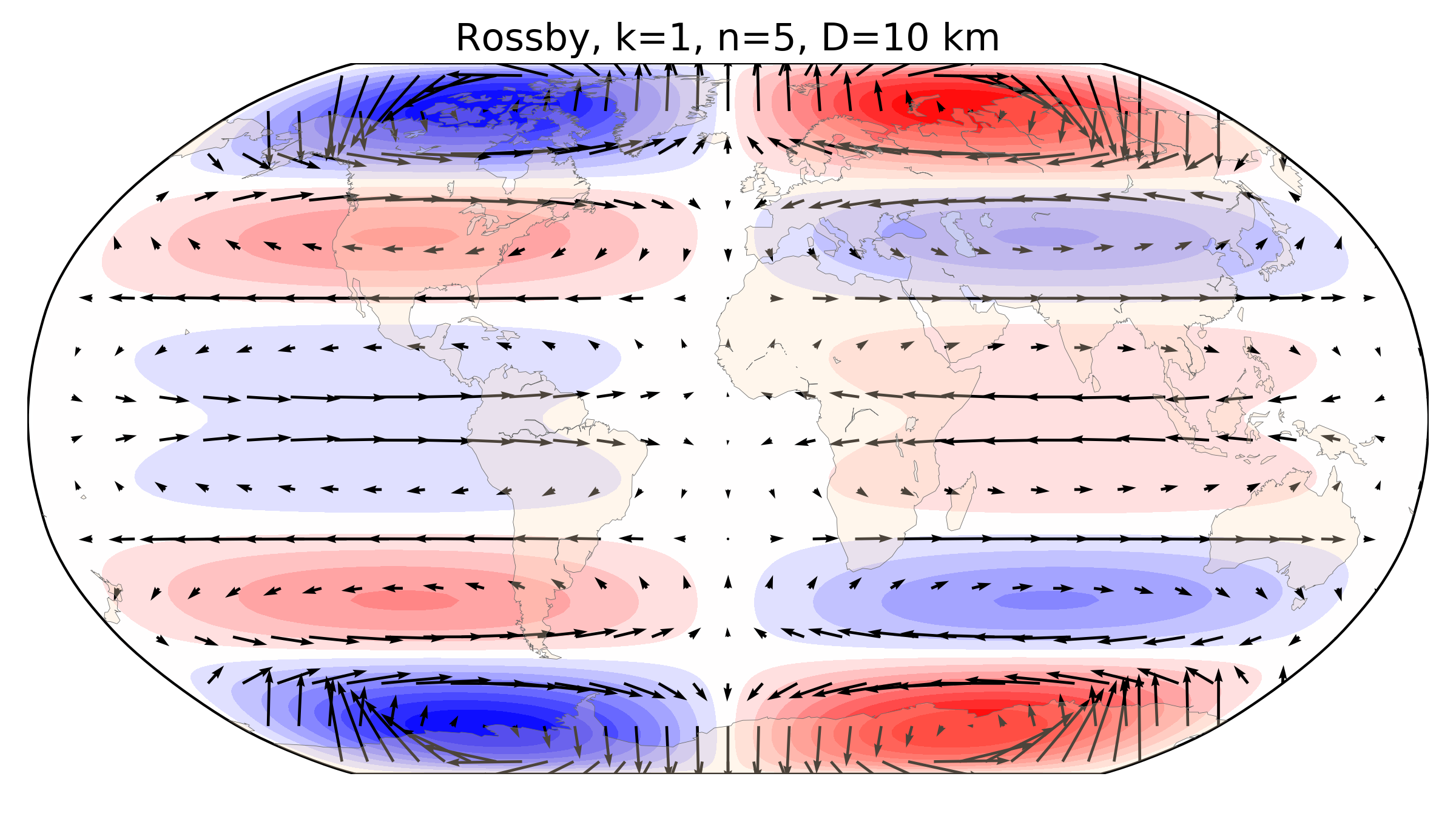
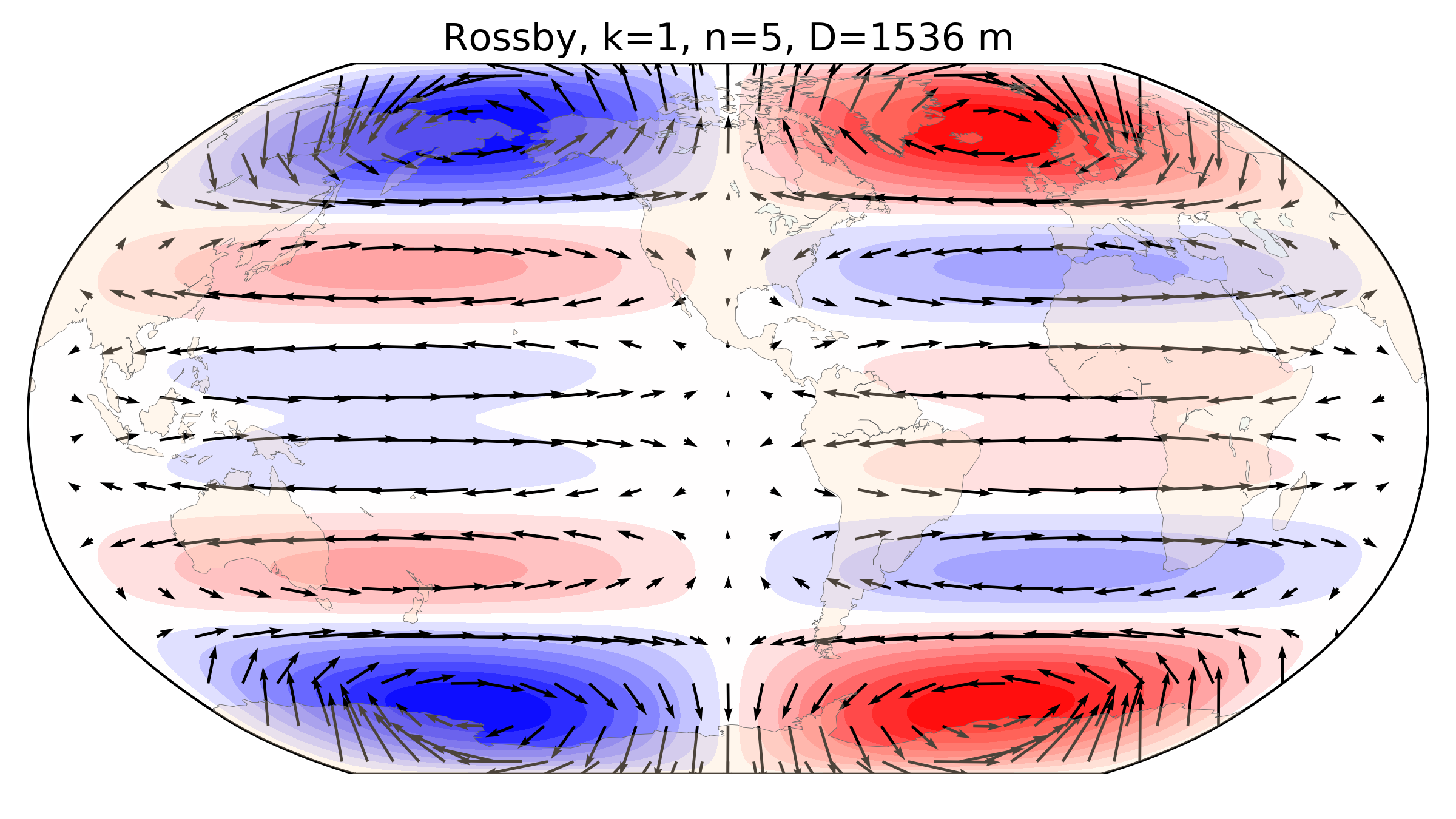
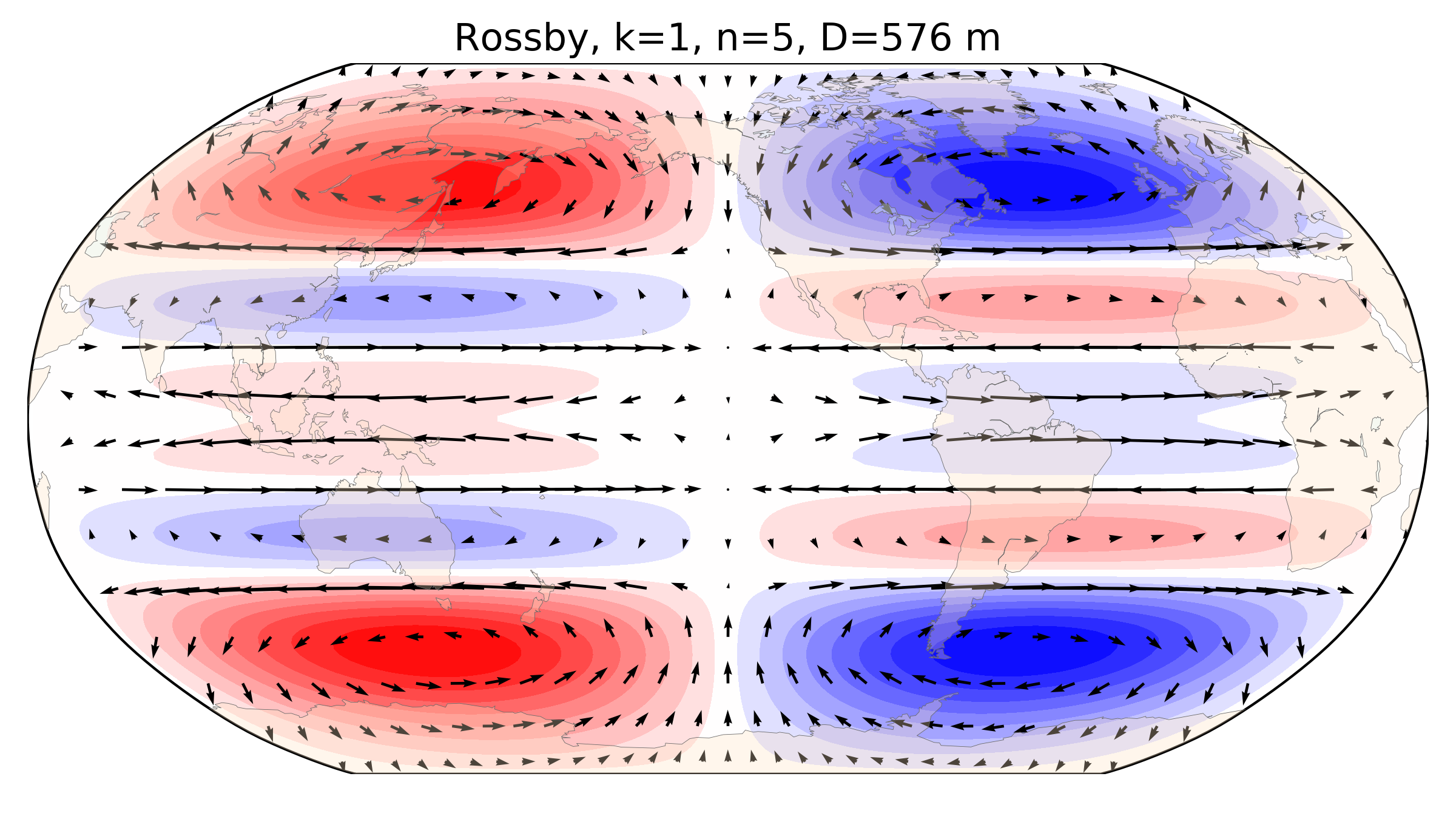
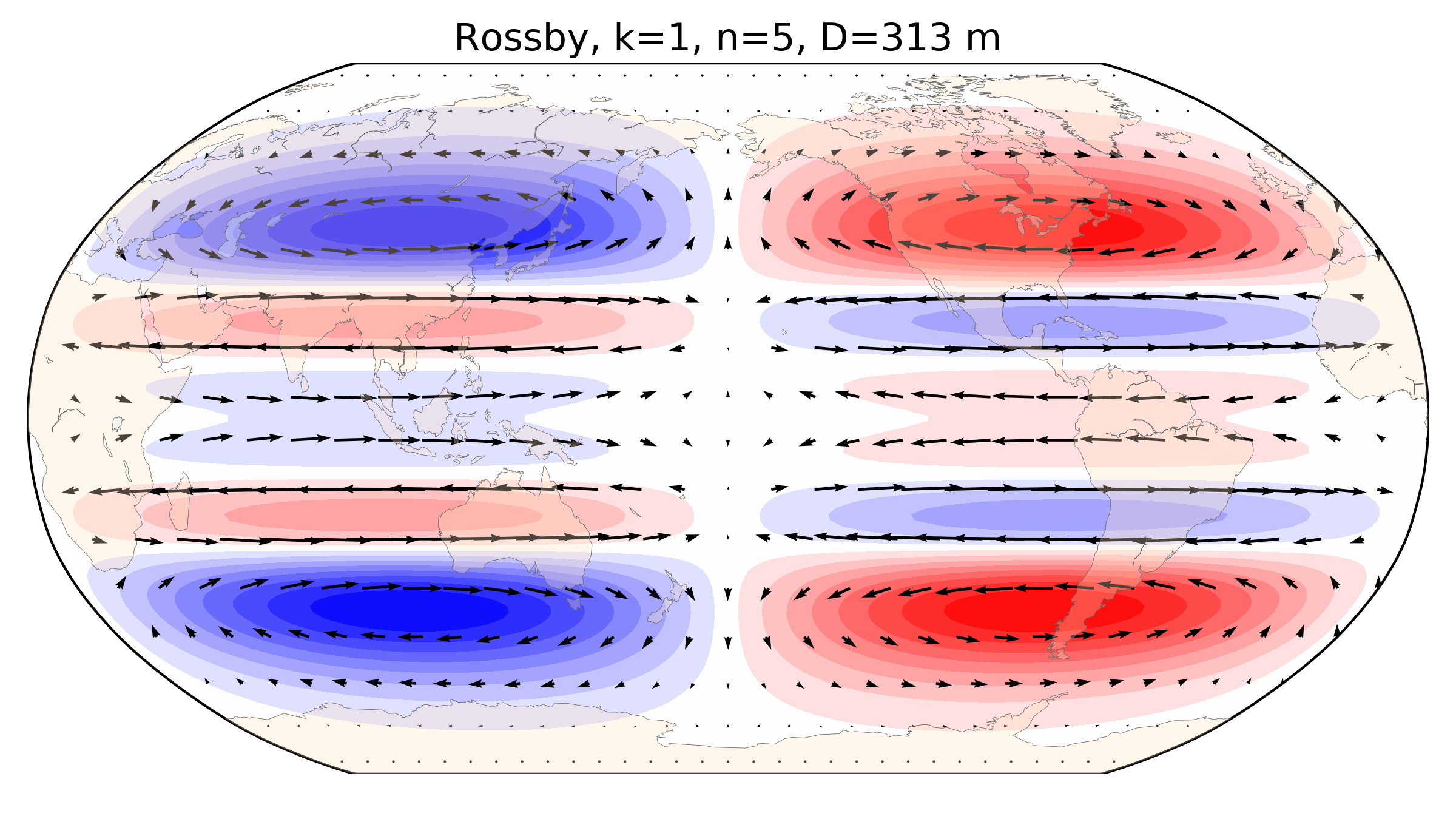
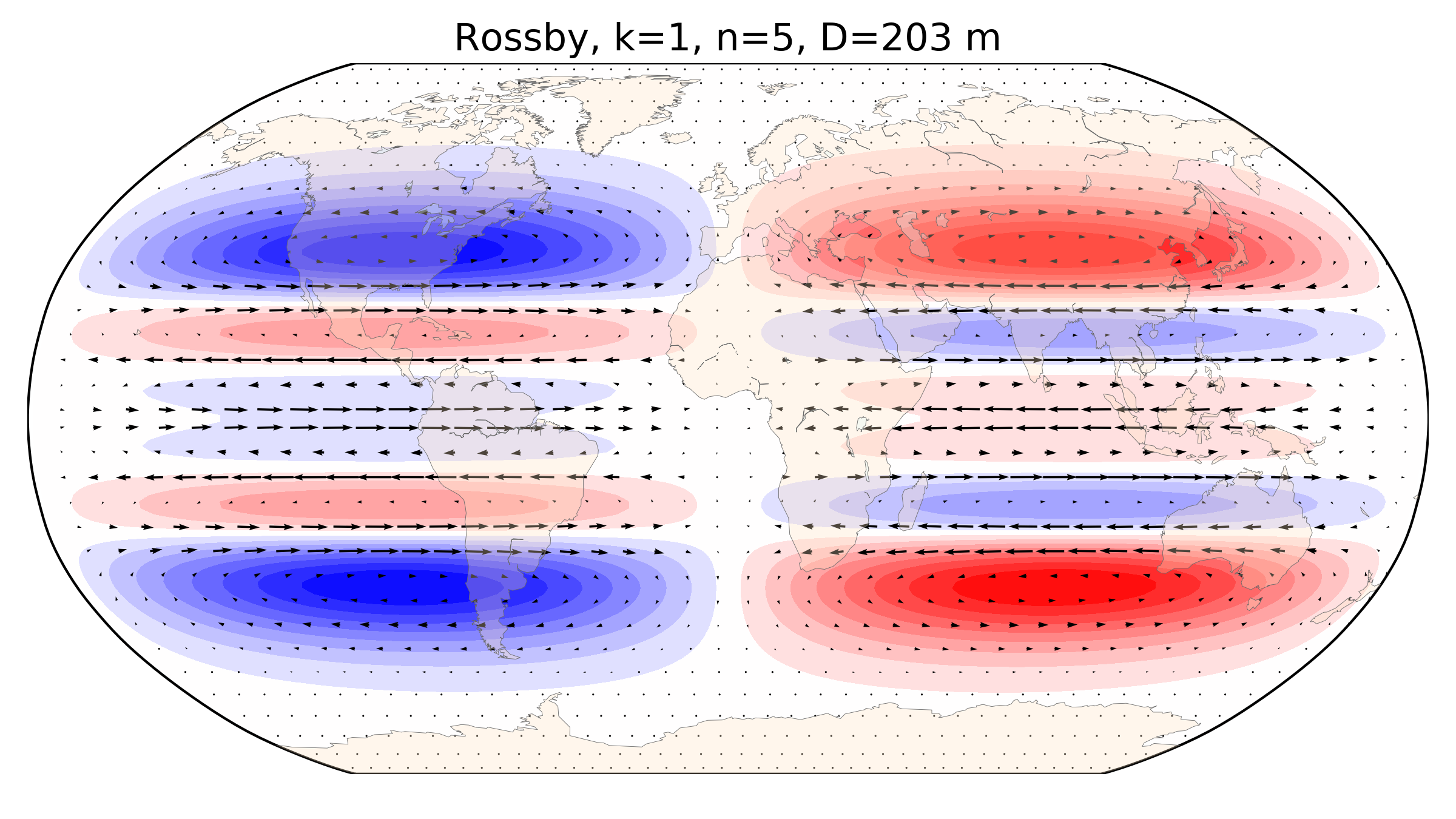
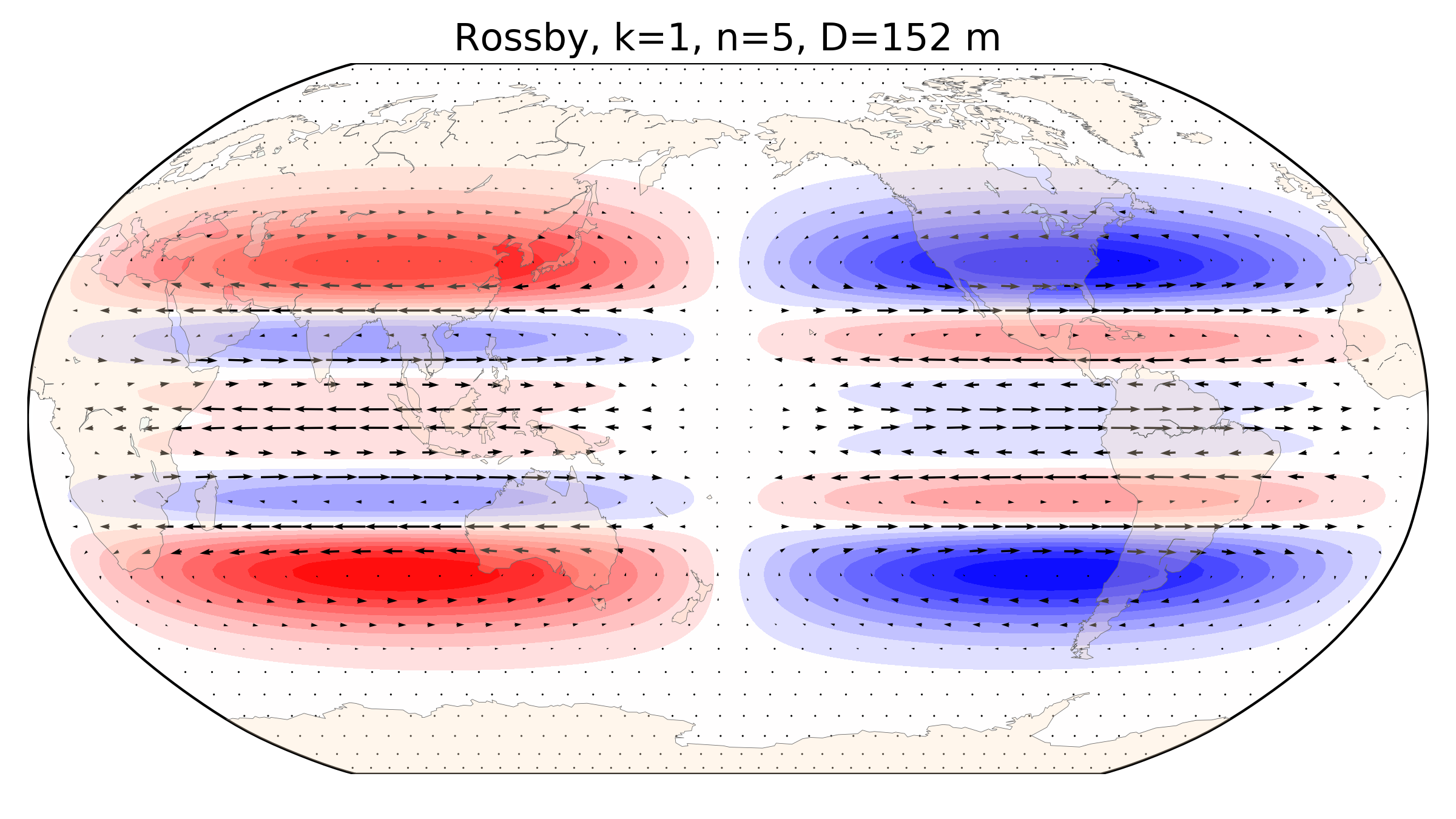
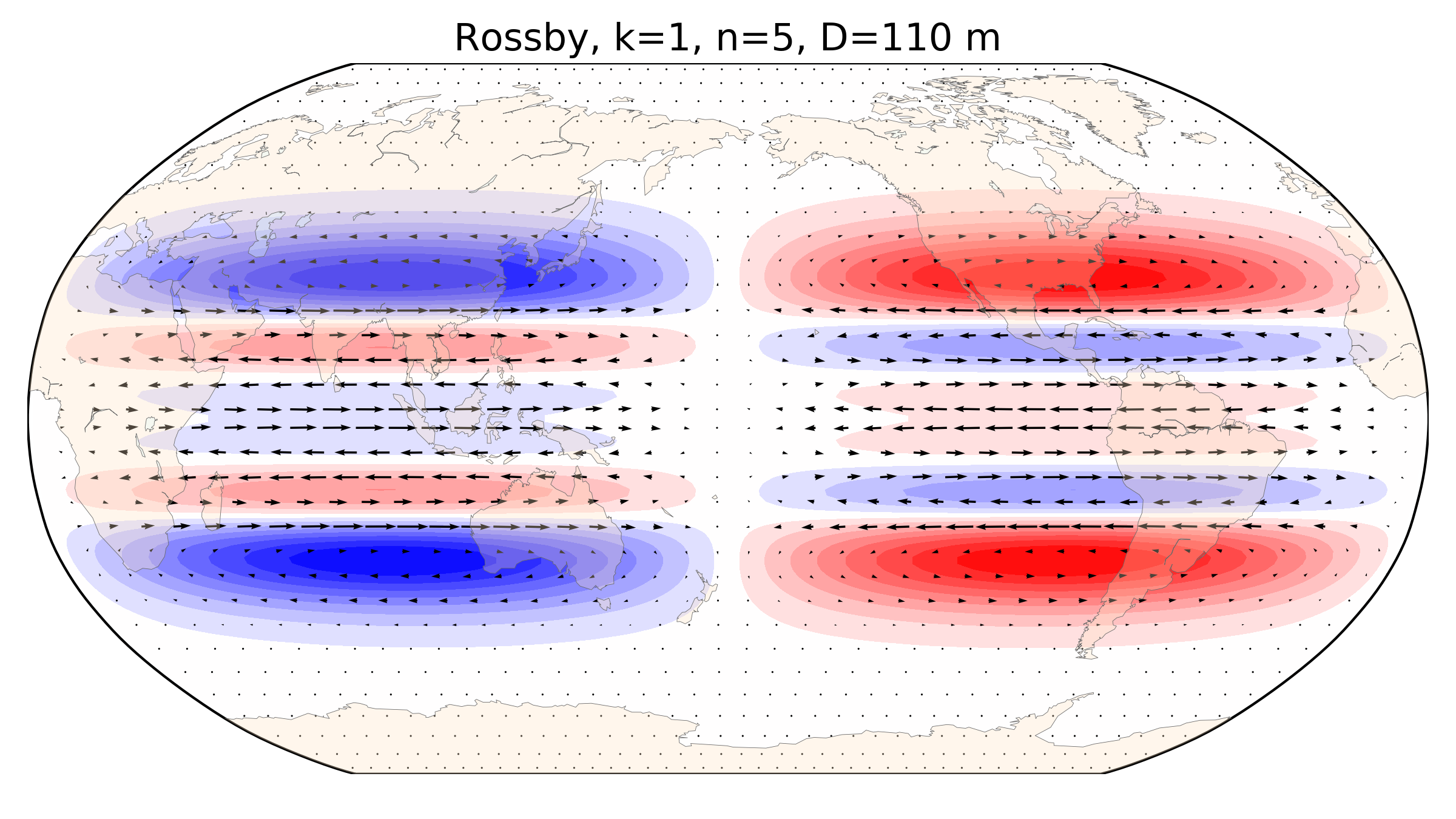
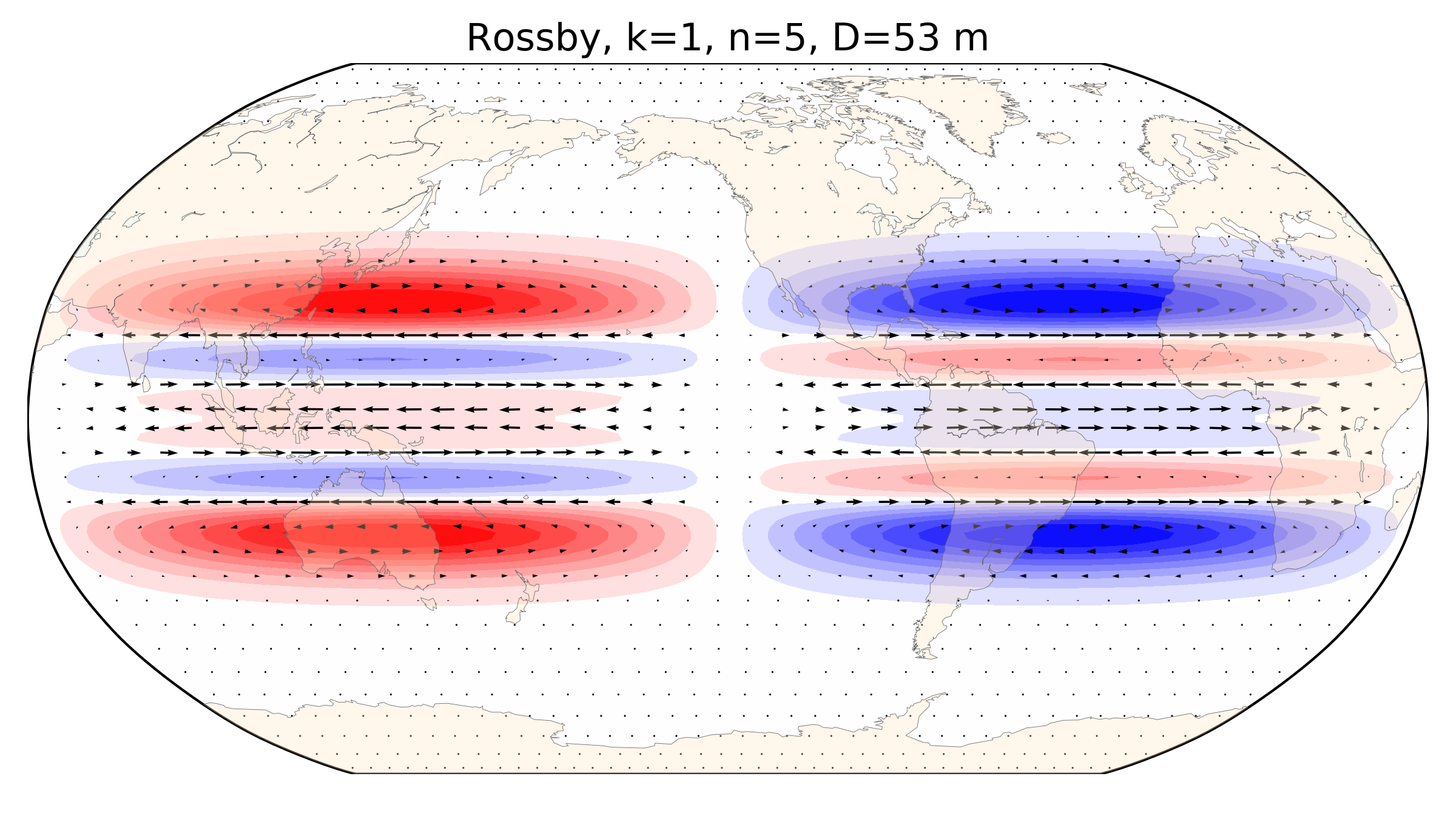
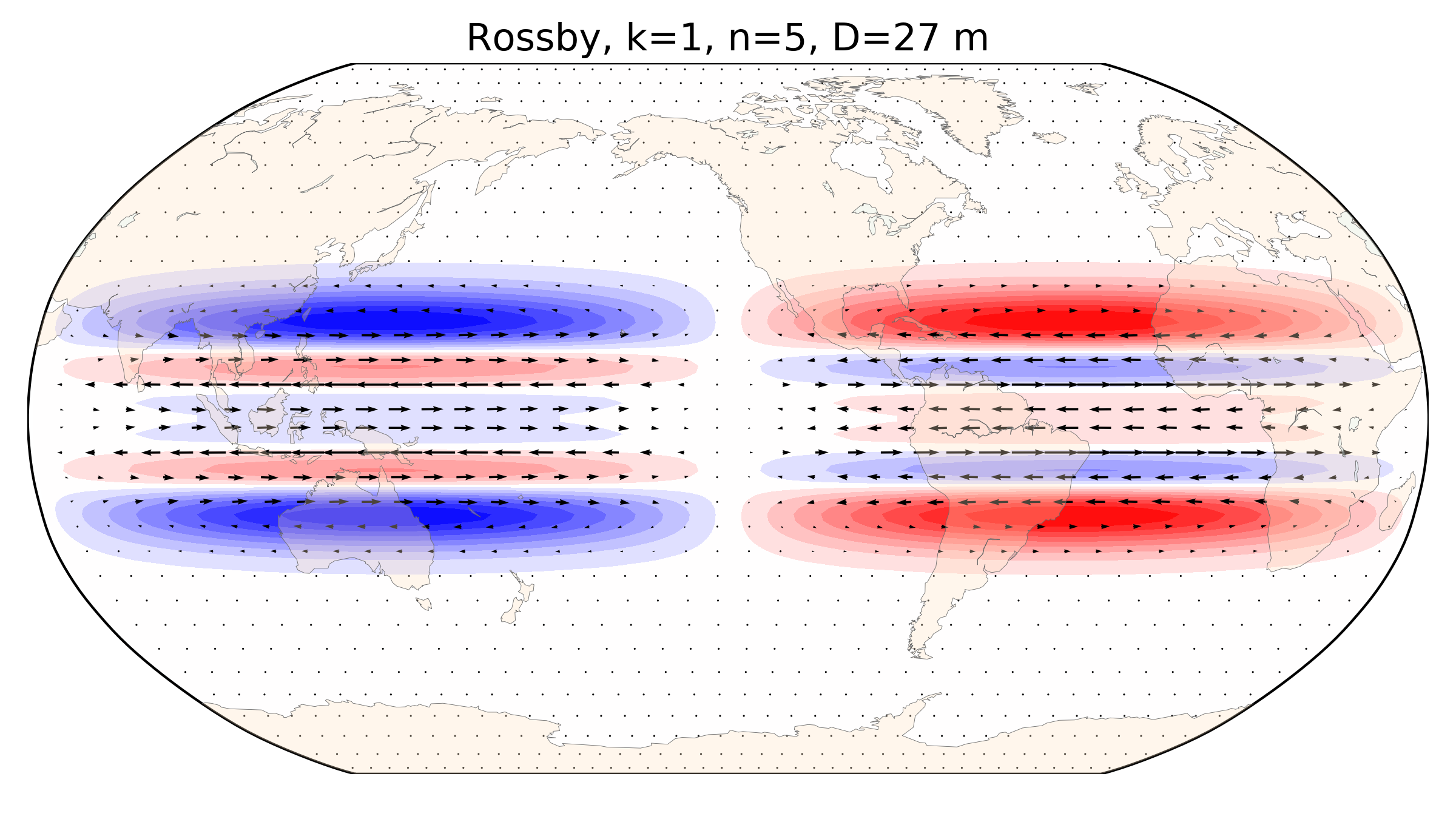
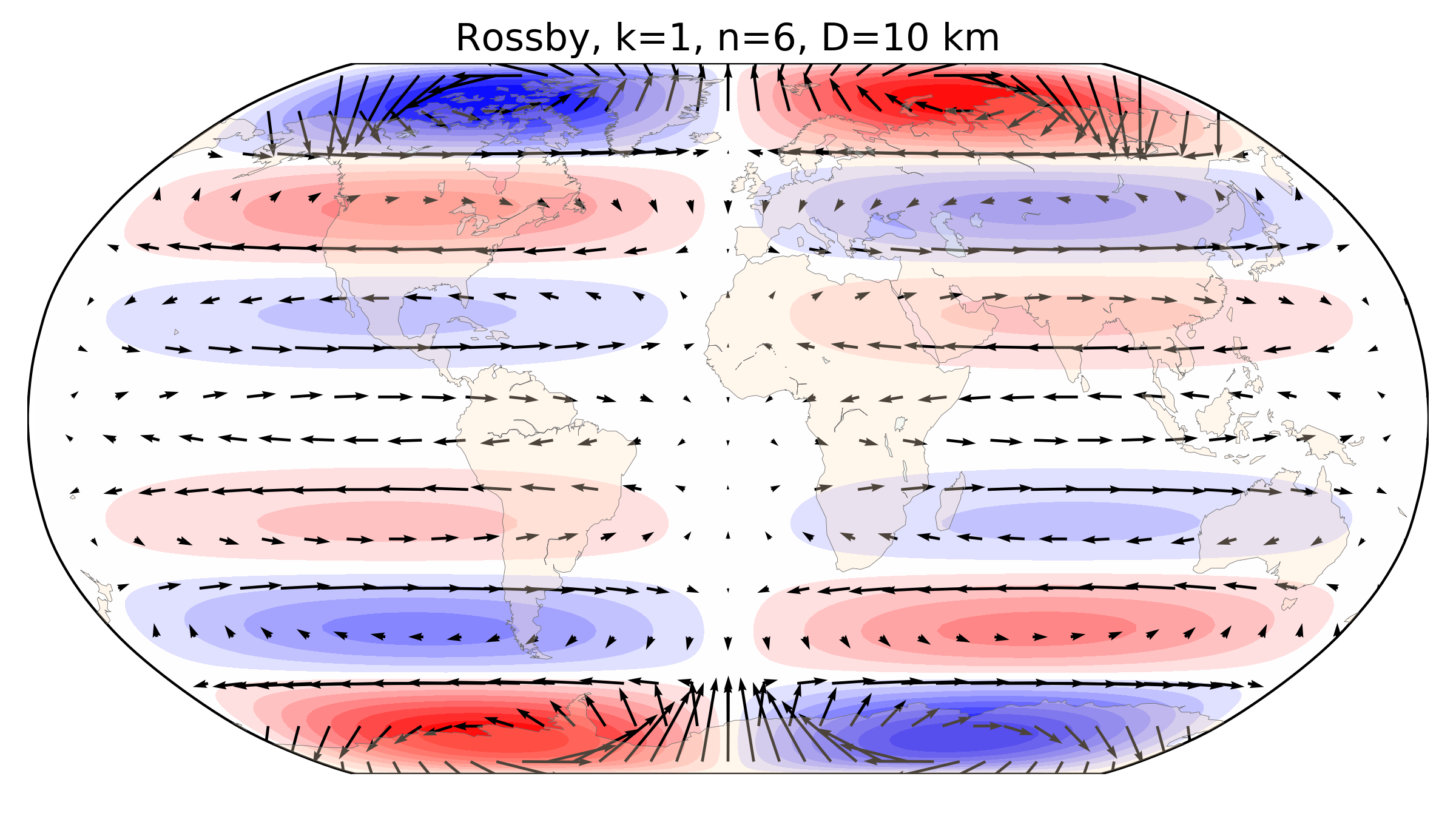
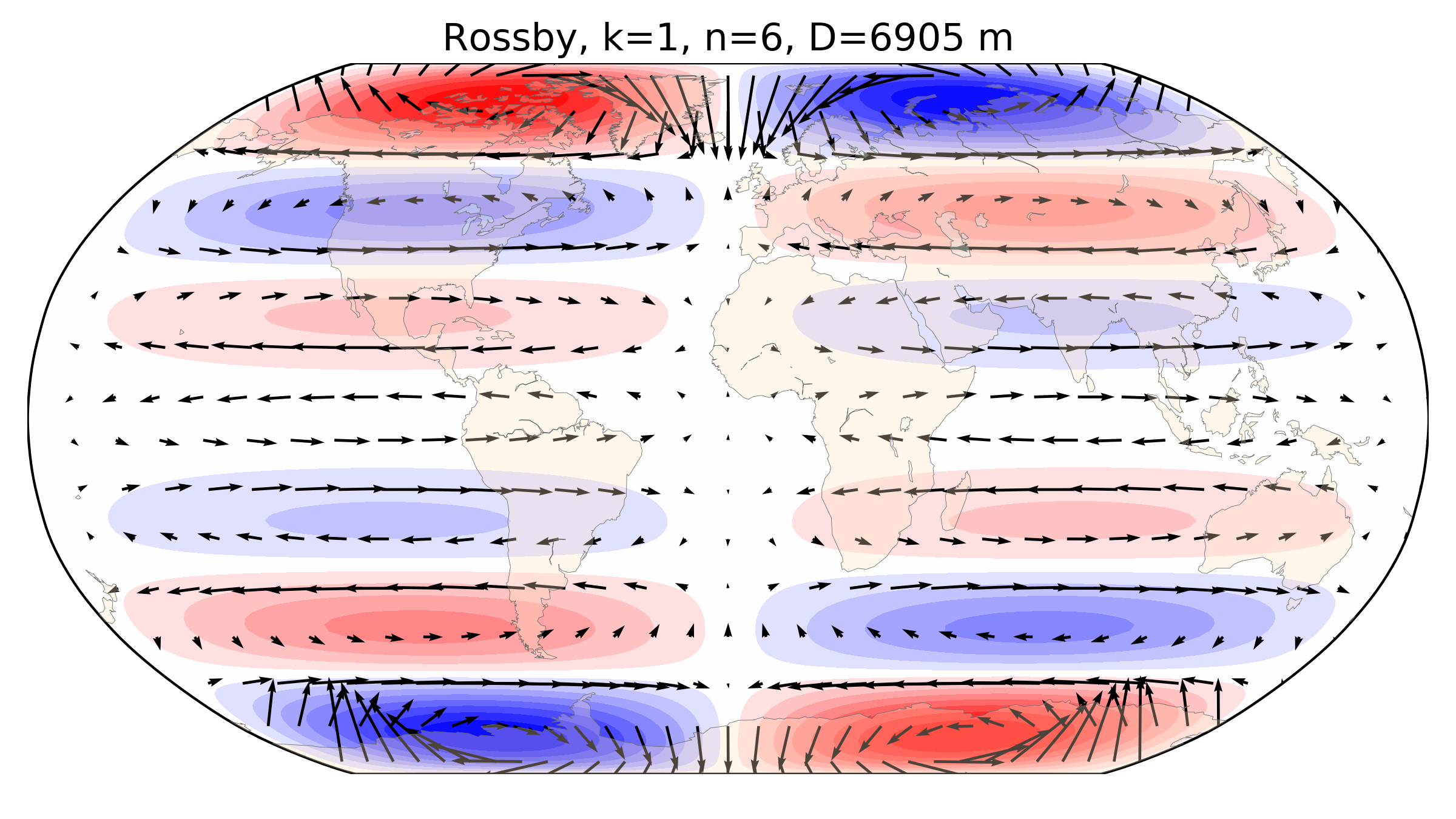
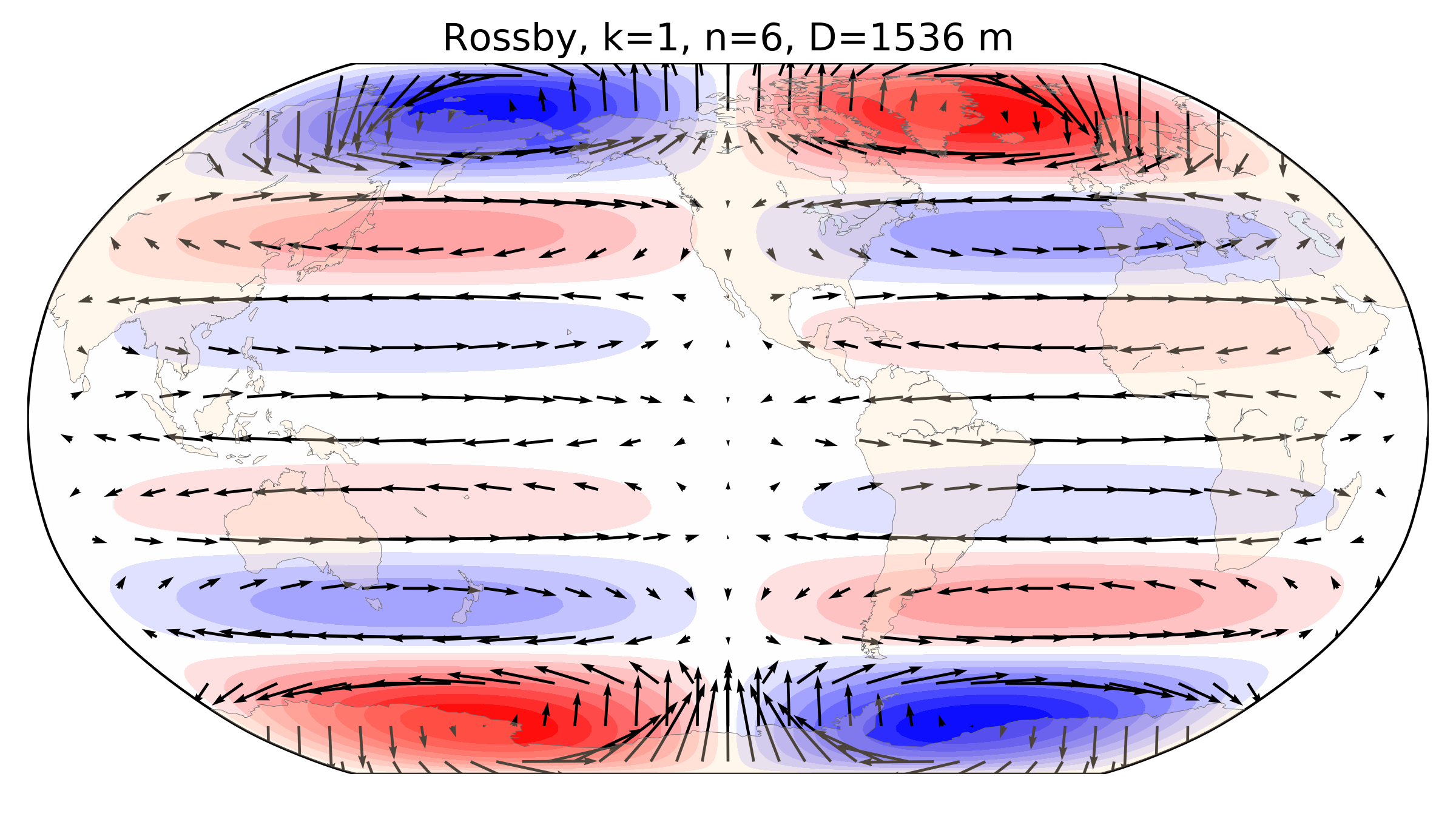
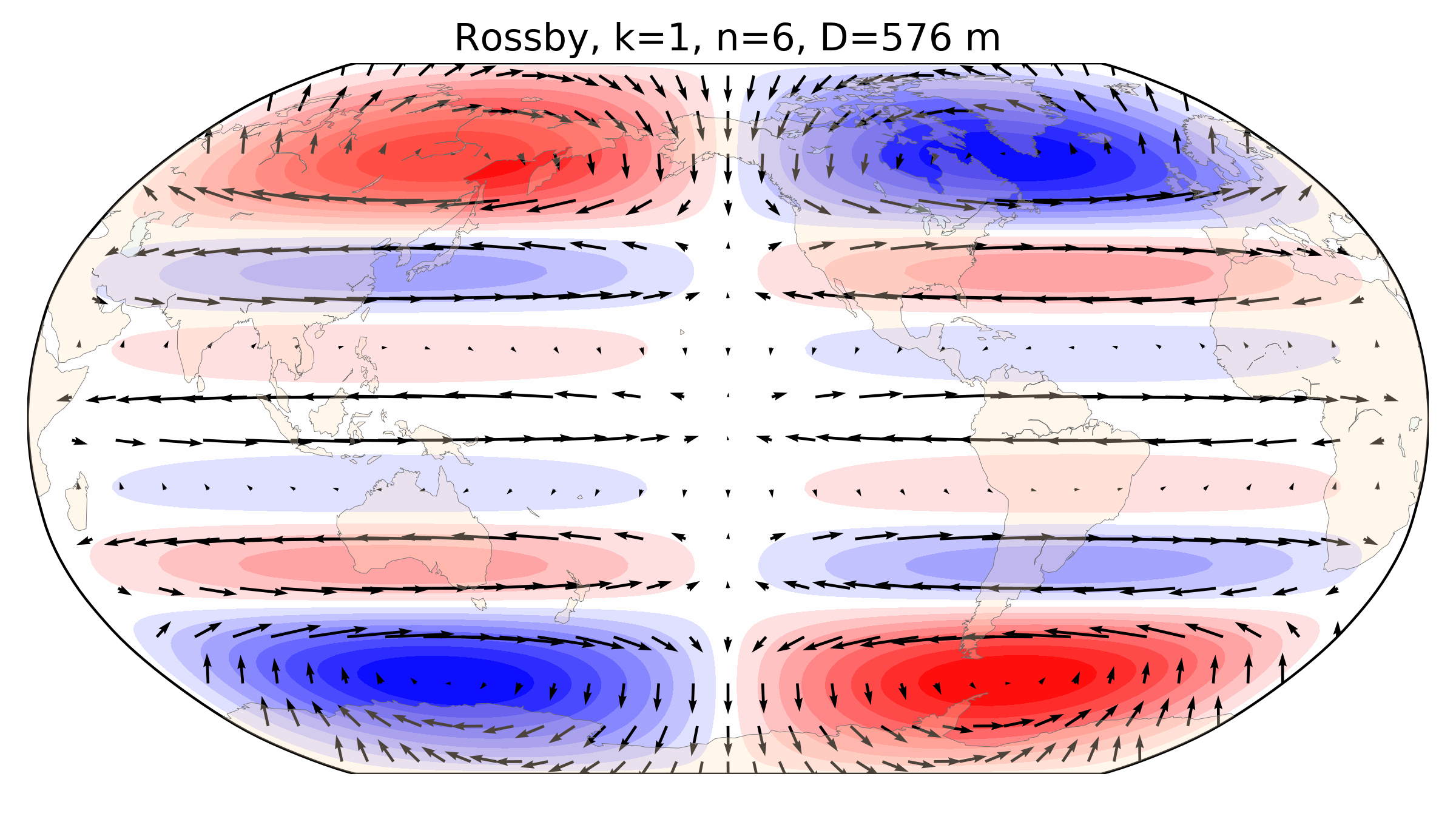
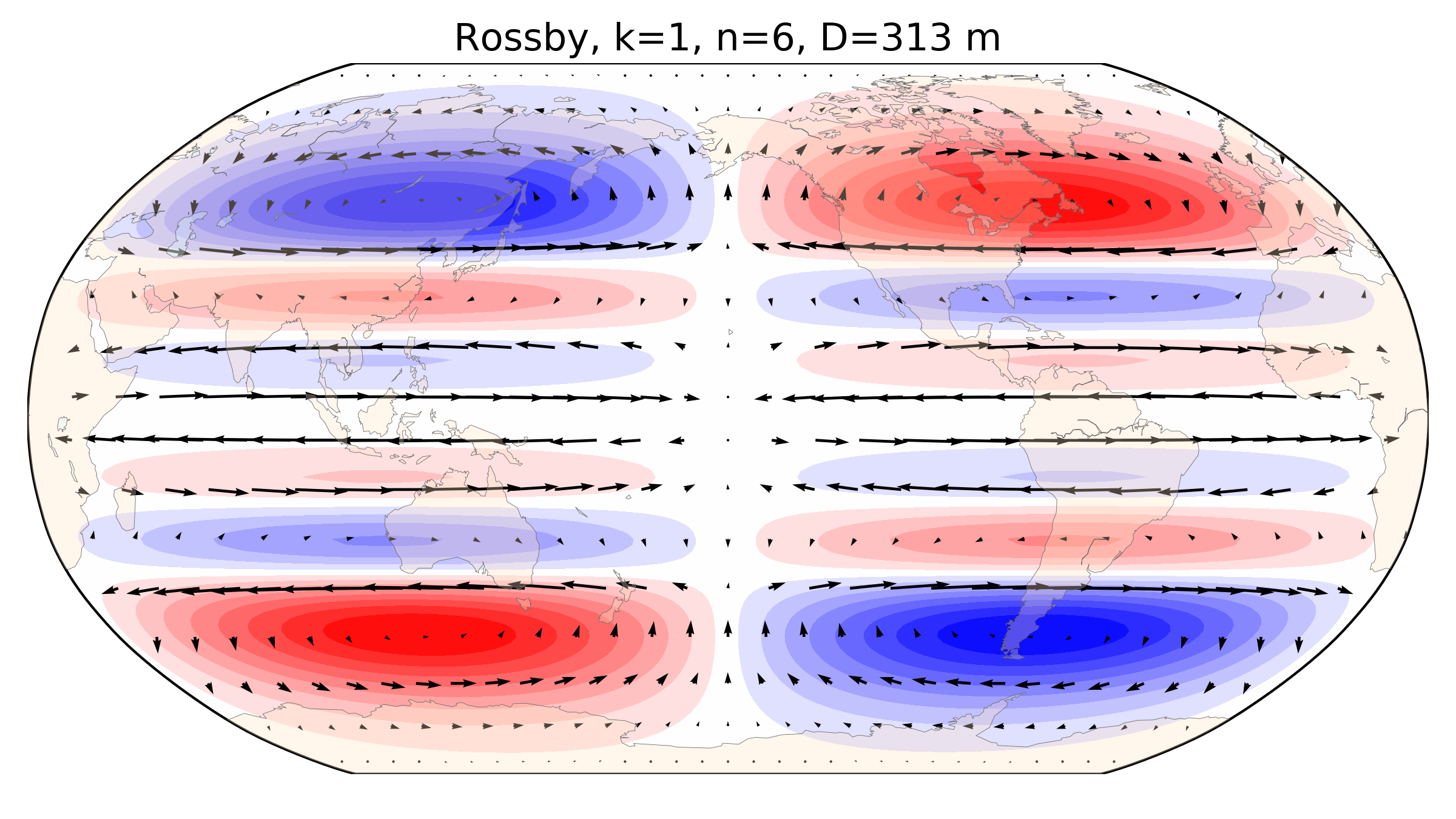
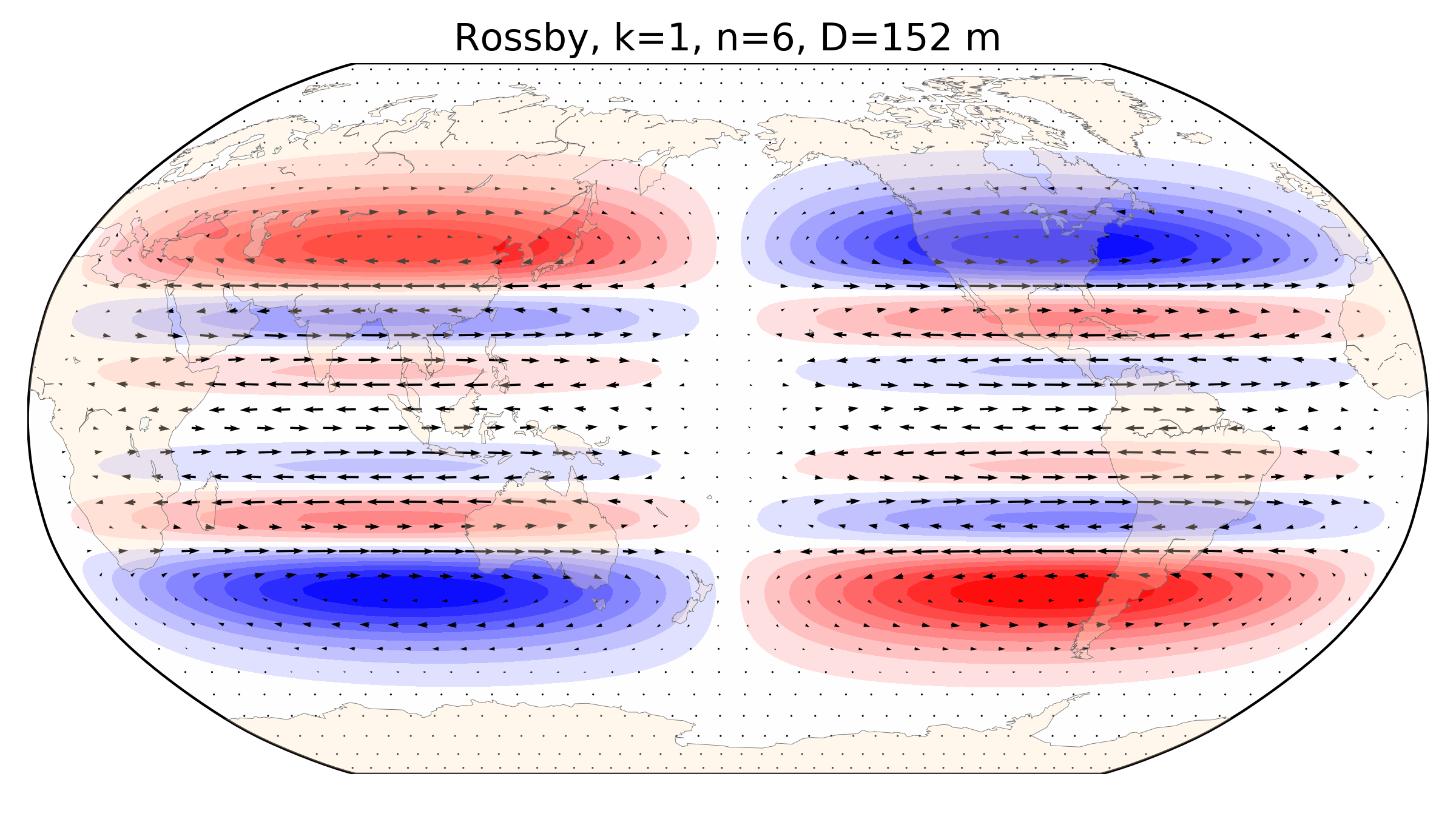
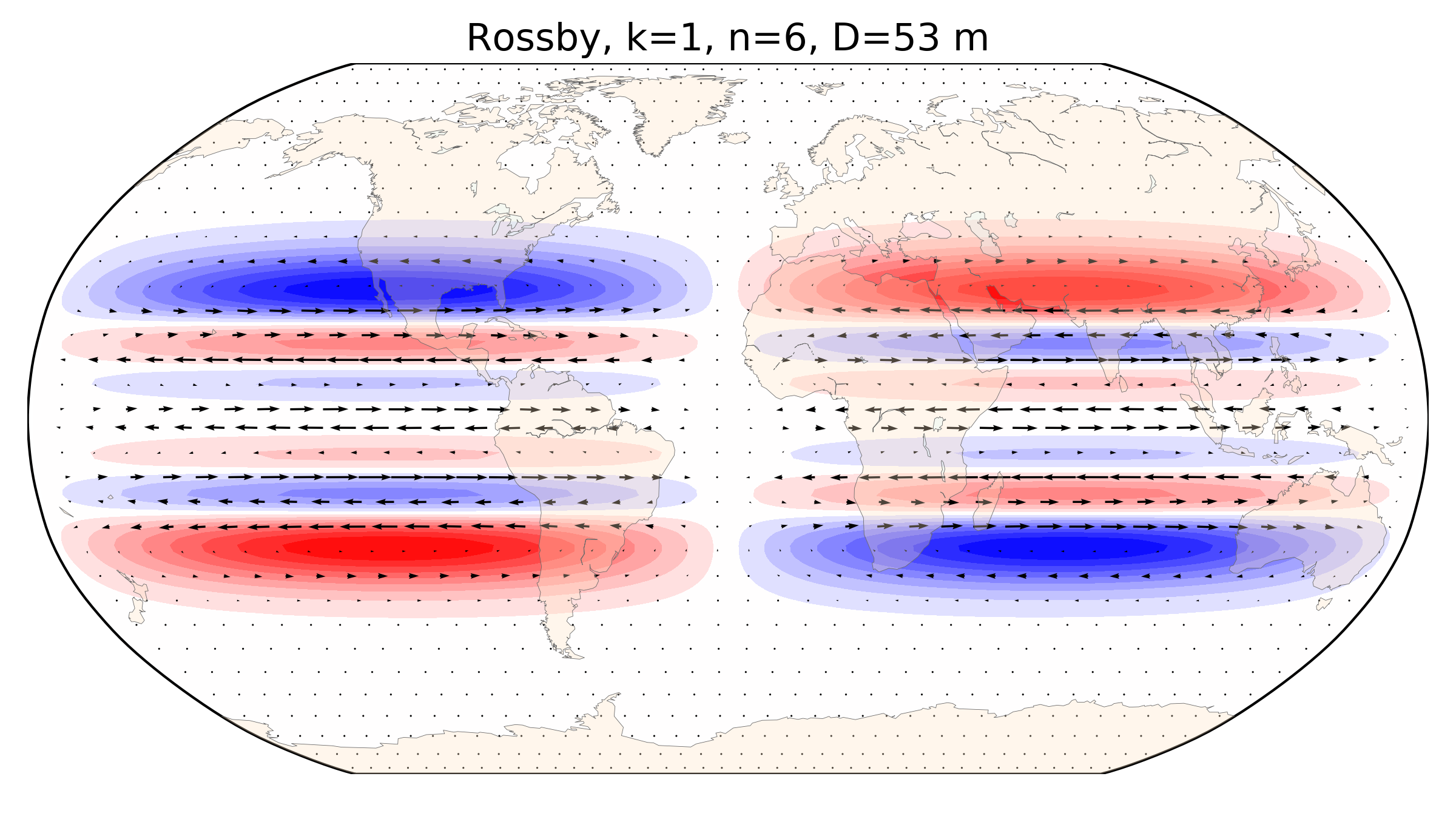
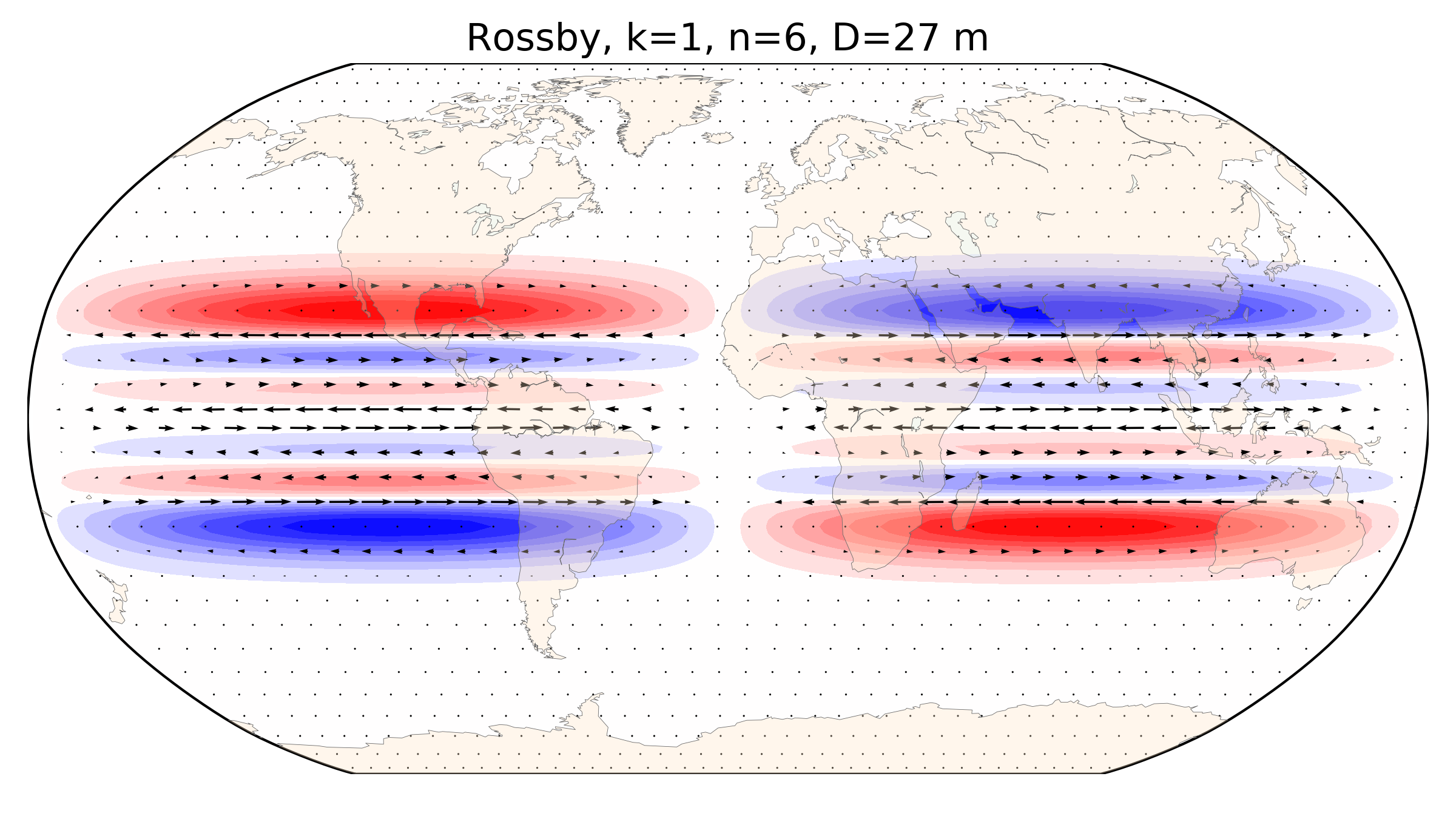
(d) Rossby Waves
Horizontal structure of the fastest Rossby mode on the sphere, n=1 Rossby wave.
Geopotential height is scaled by the maximal height. Colours vary between -1 and +1 with positive/negative perturbations in red/blue.
Wind components are scaled by the maximal wind speed. The n=1 Rossby wave, together with the Kelvin wave, provides basic understanding of large-scale circulation in response to tropical heating perturbations, following Gill (1980) and the long-wave approximation.
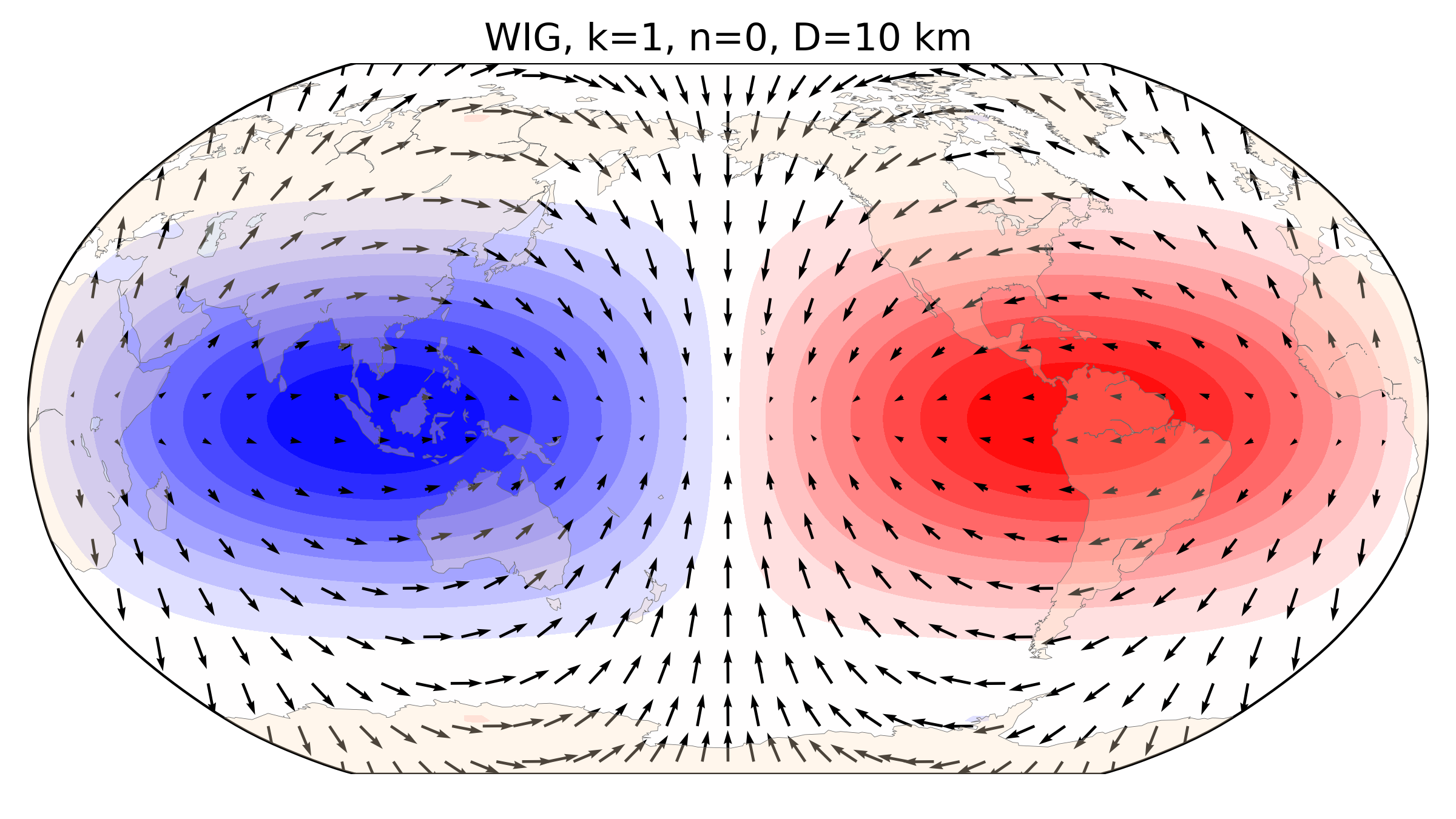
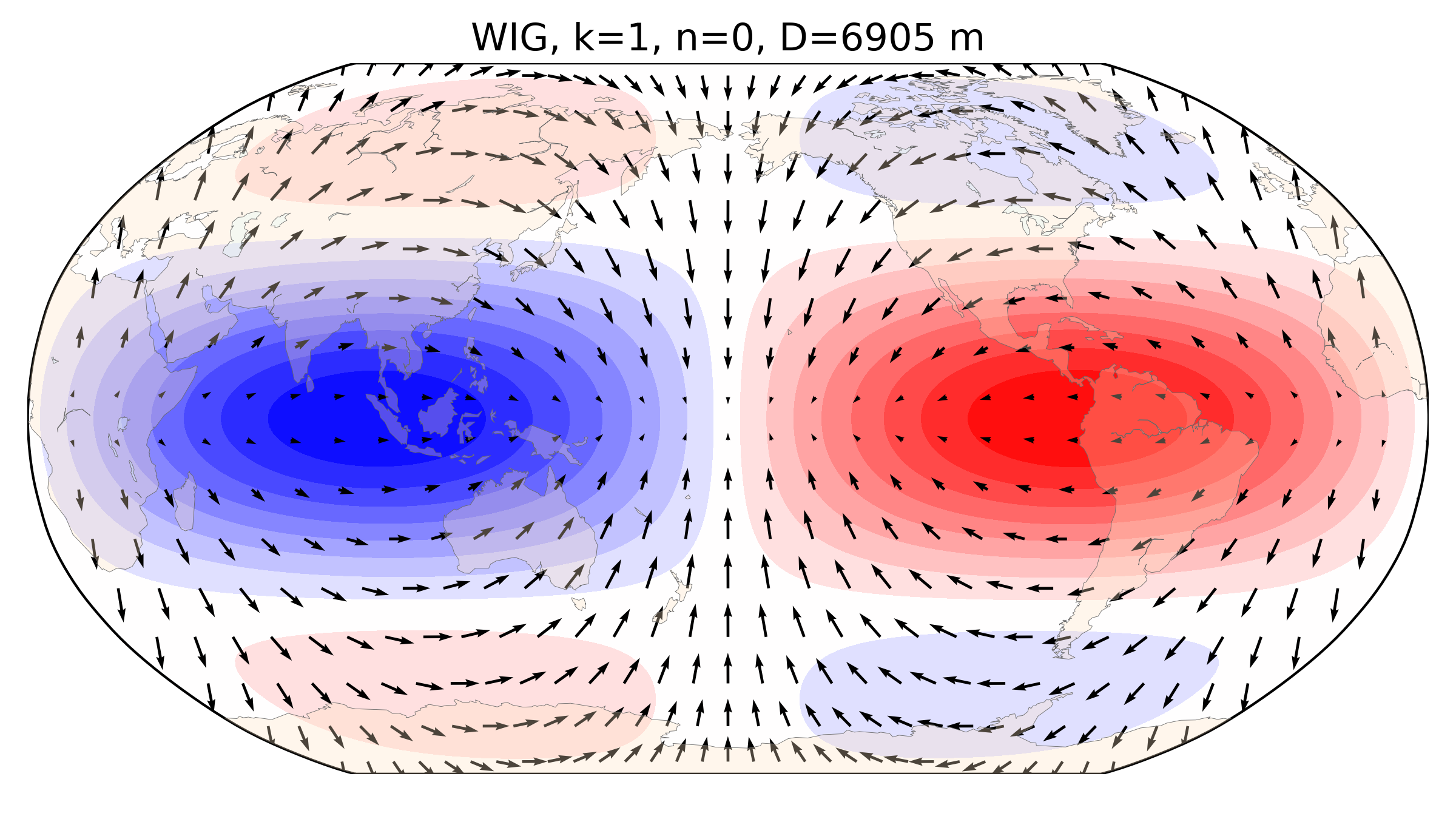
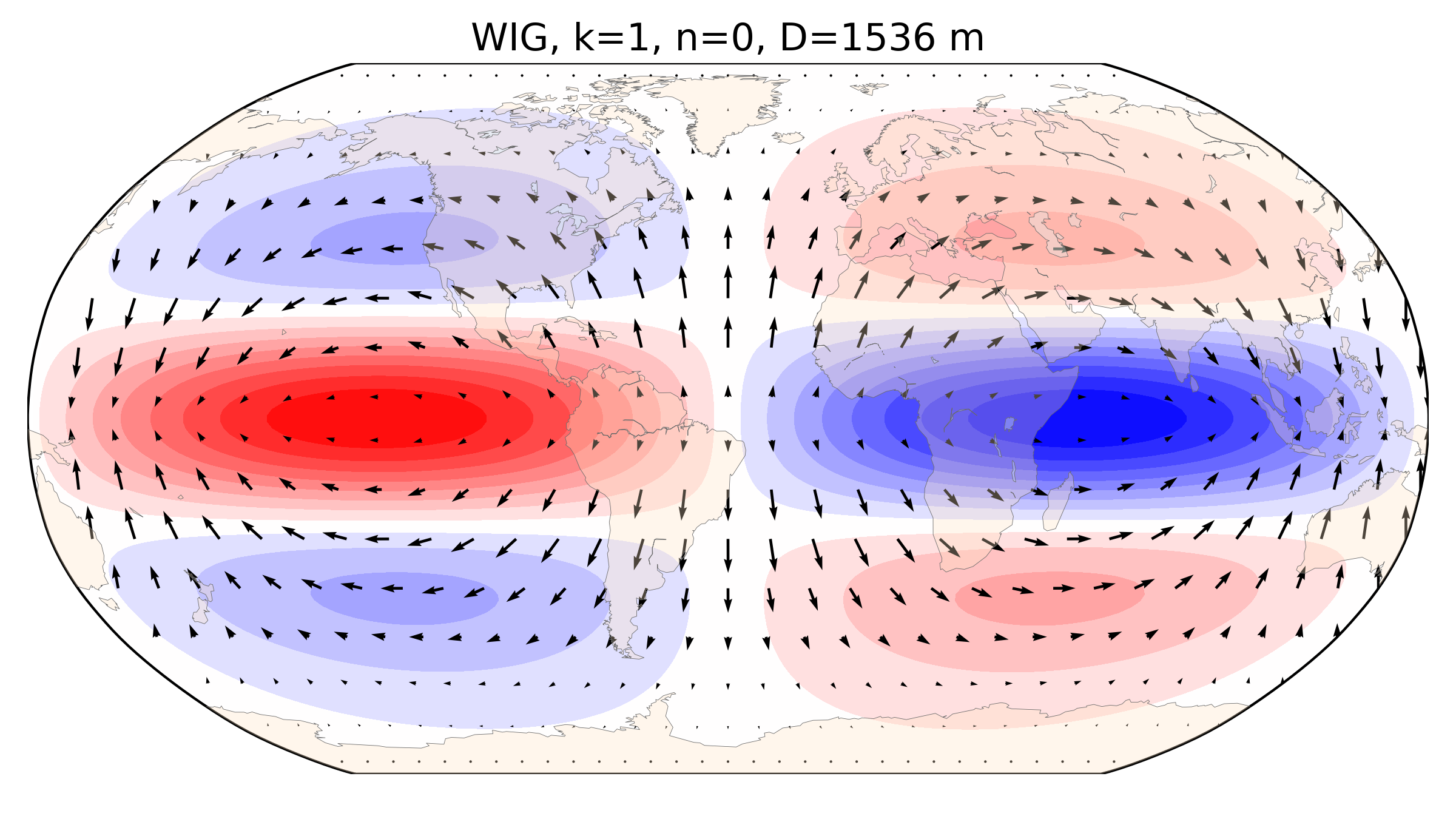
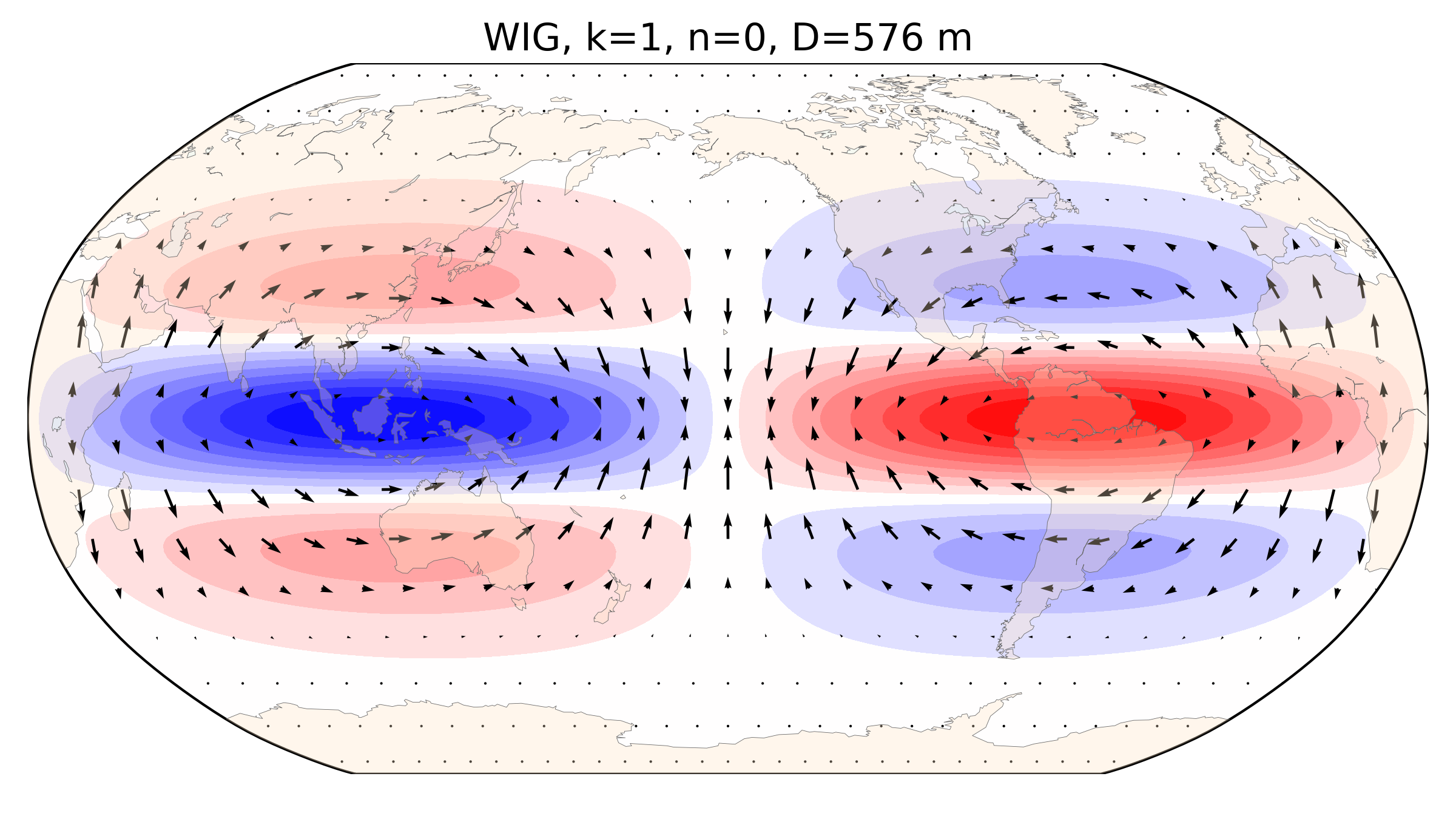
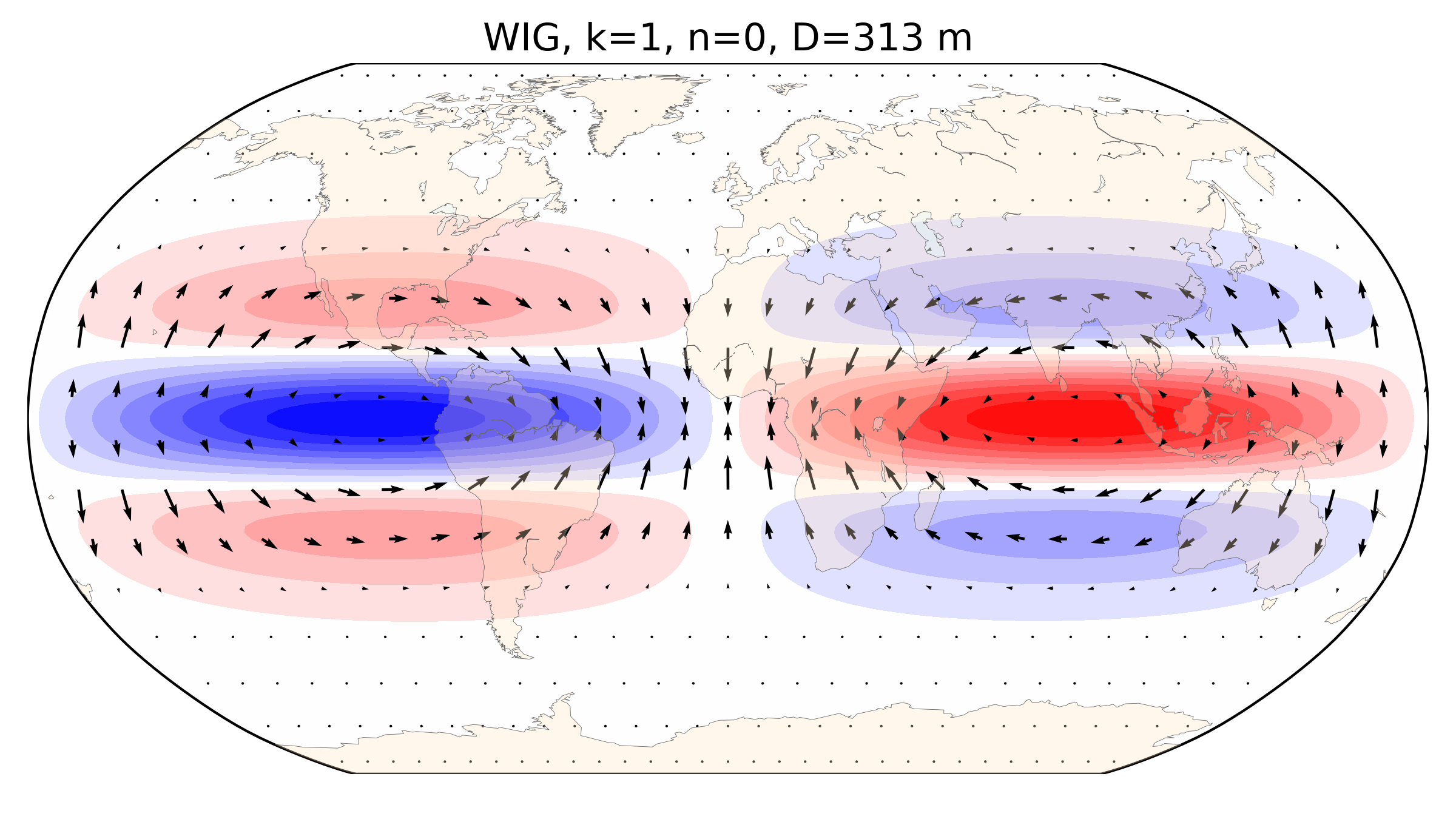
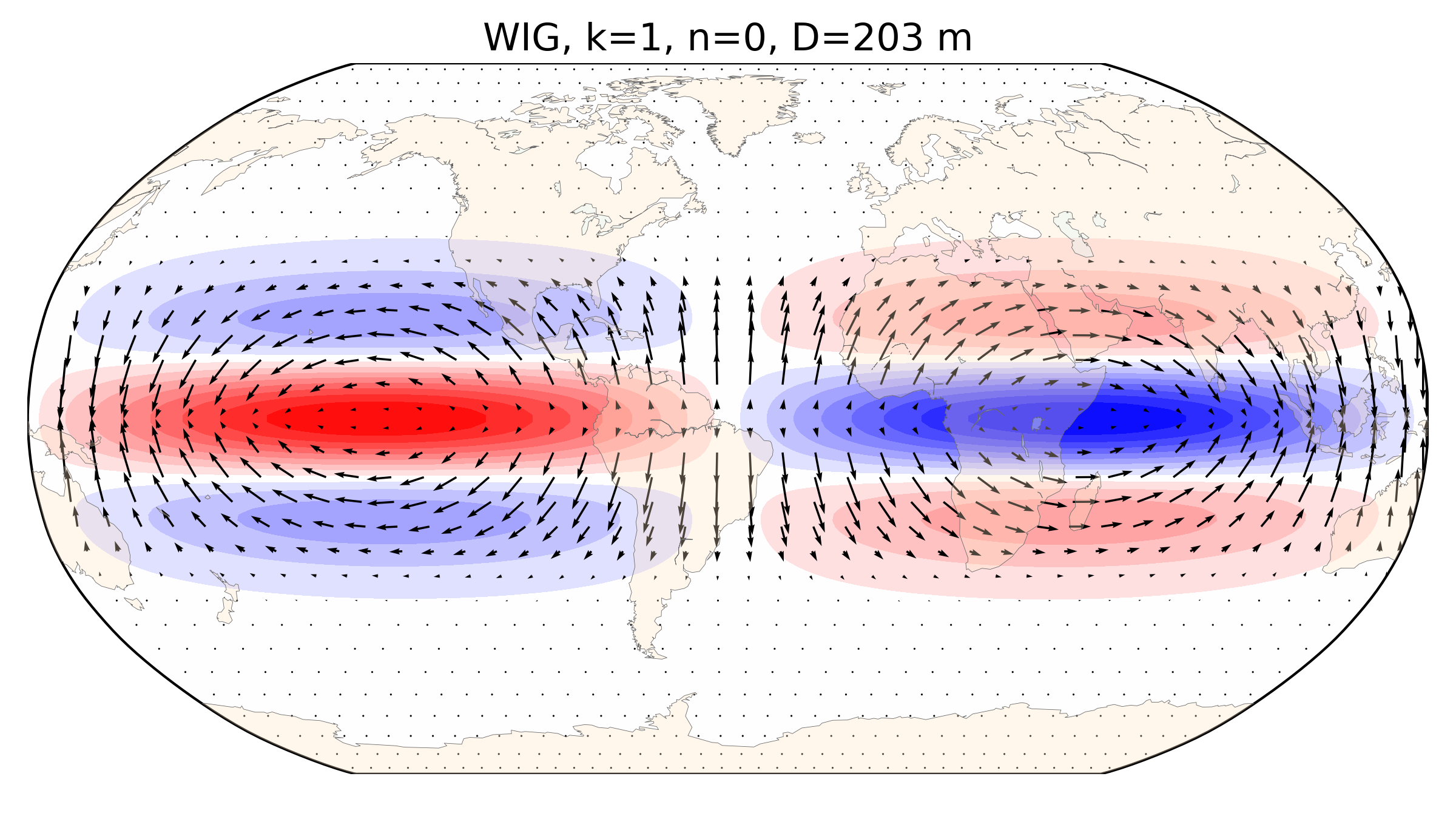
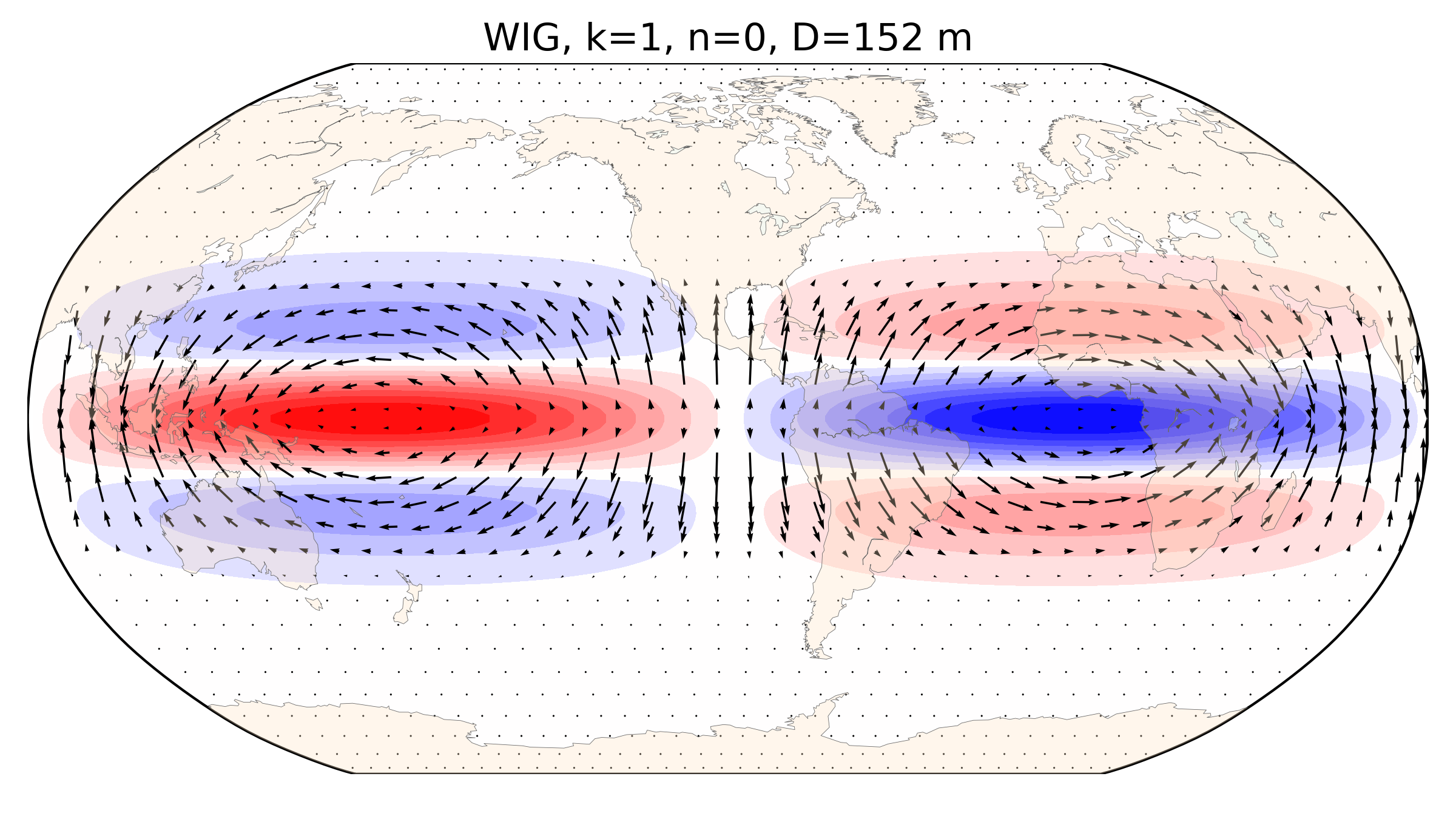
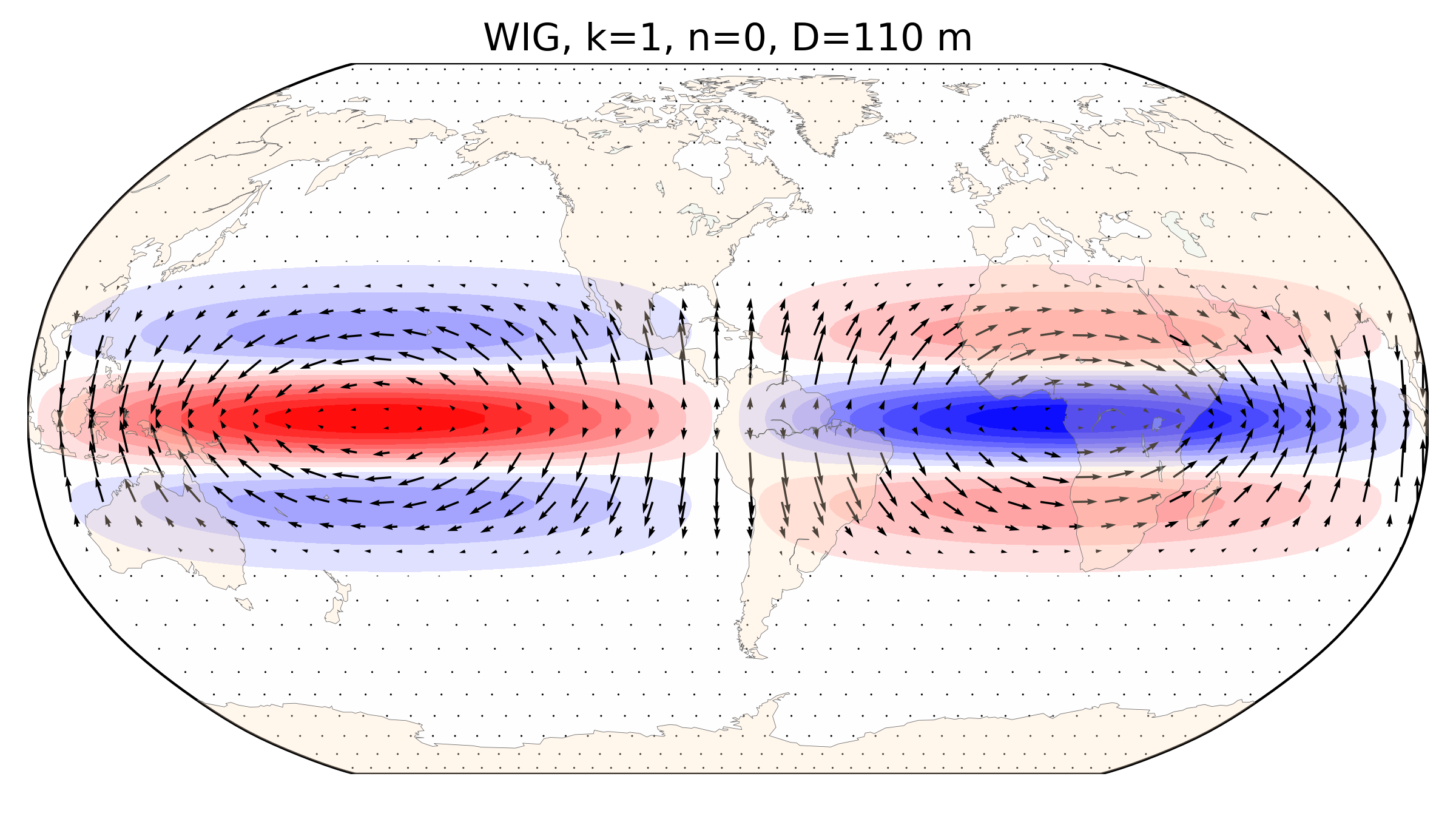
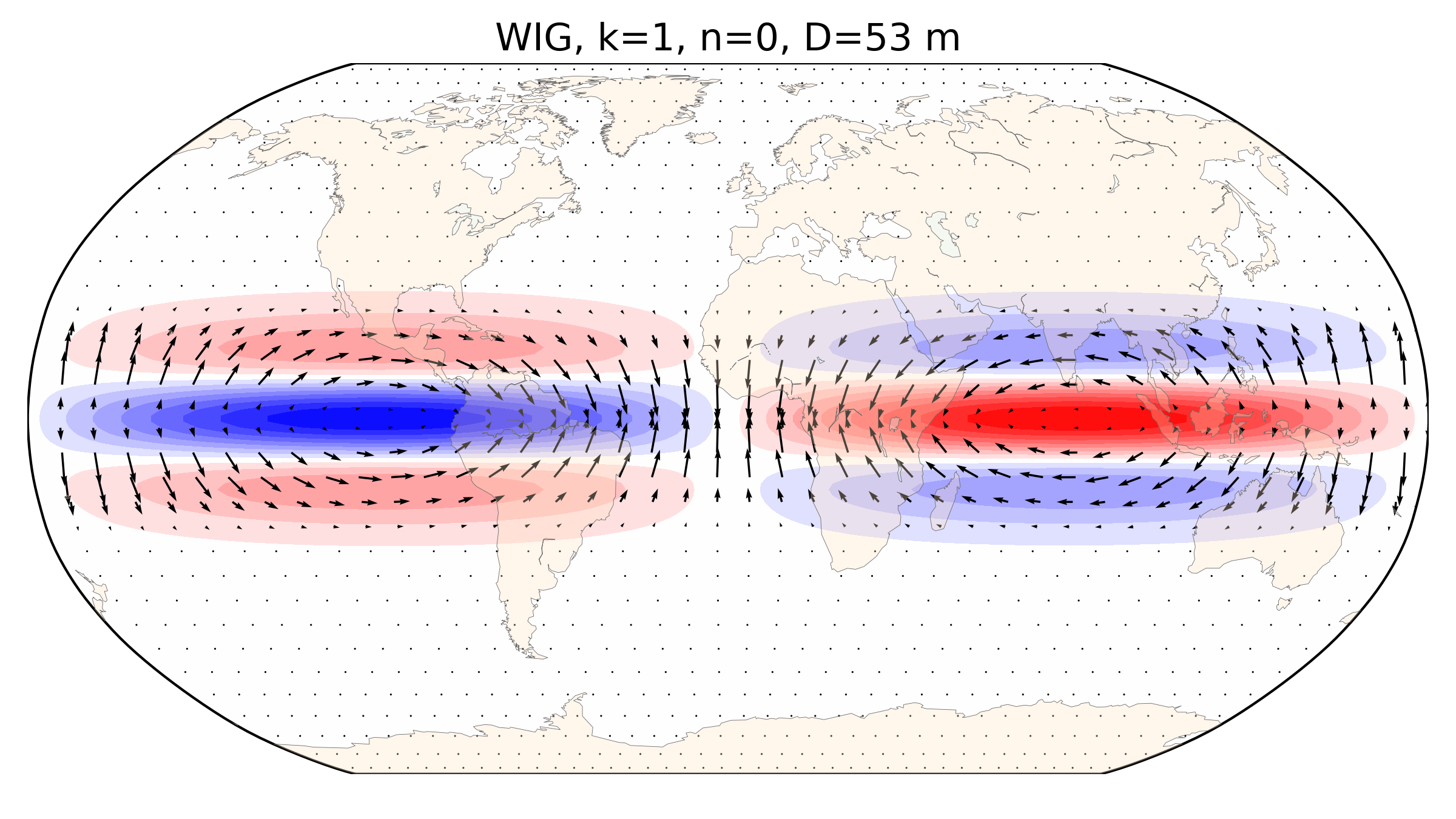
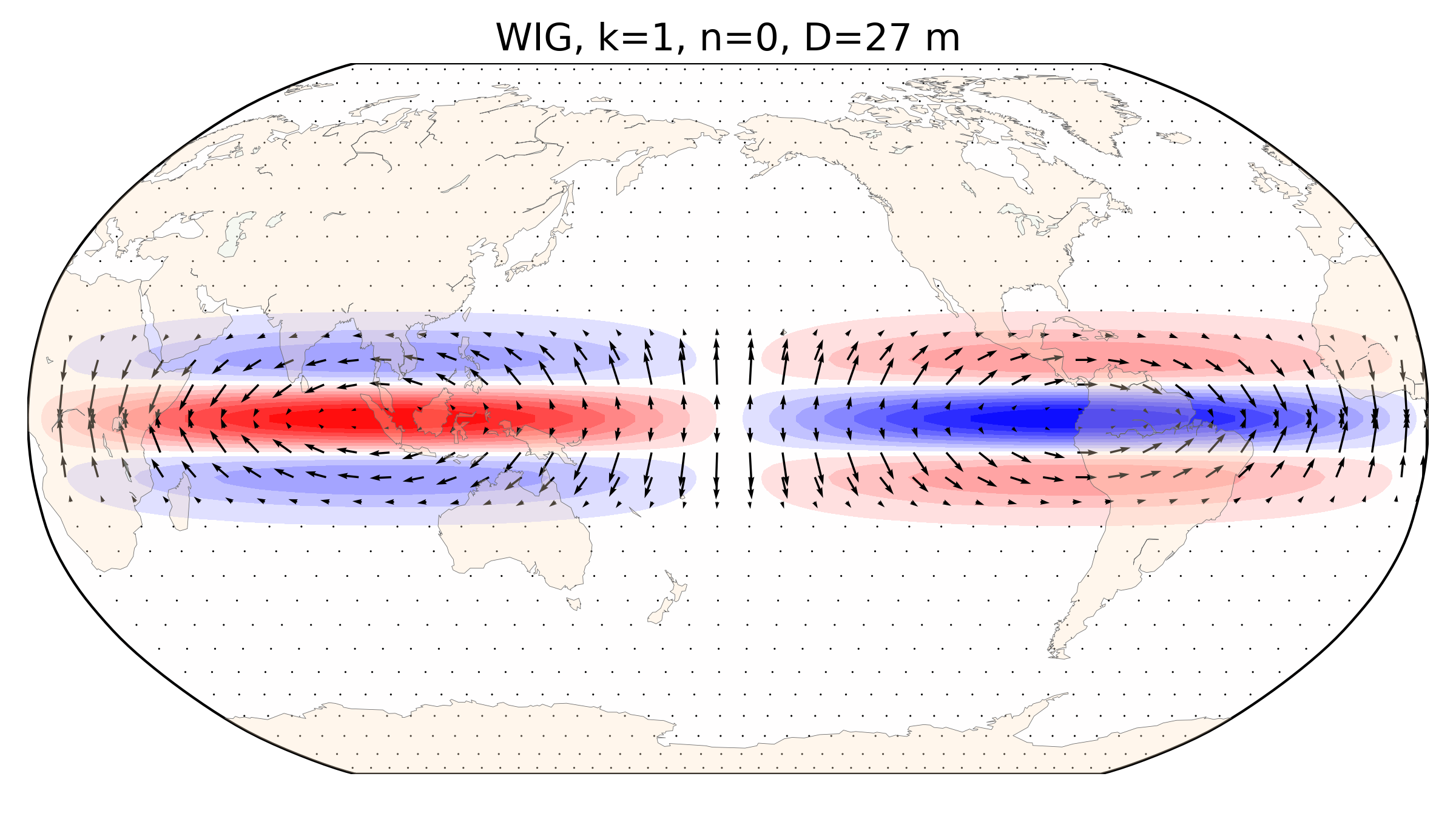
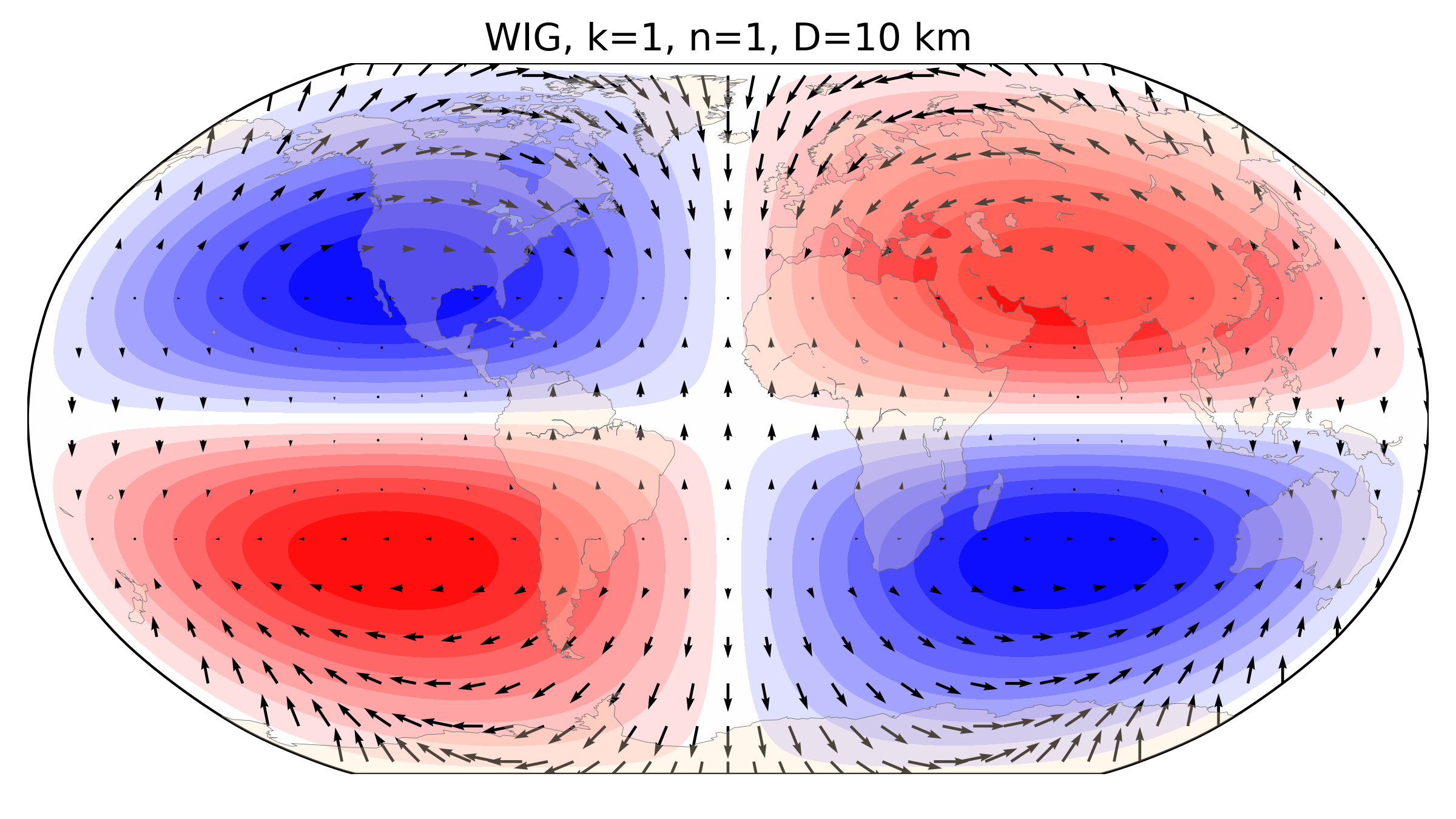
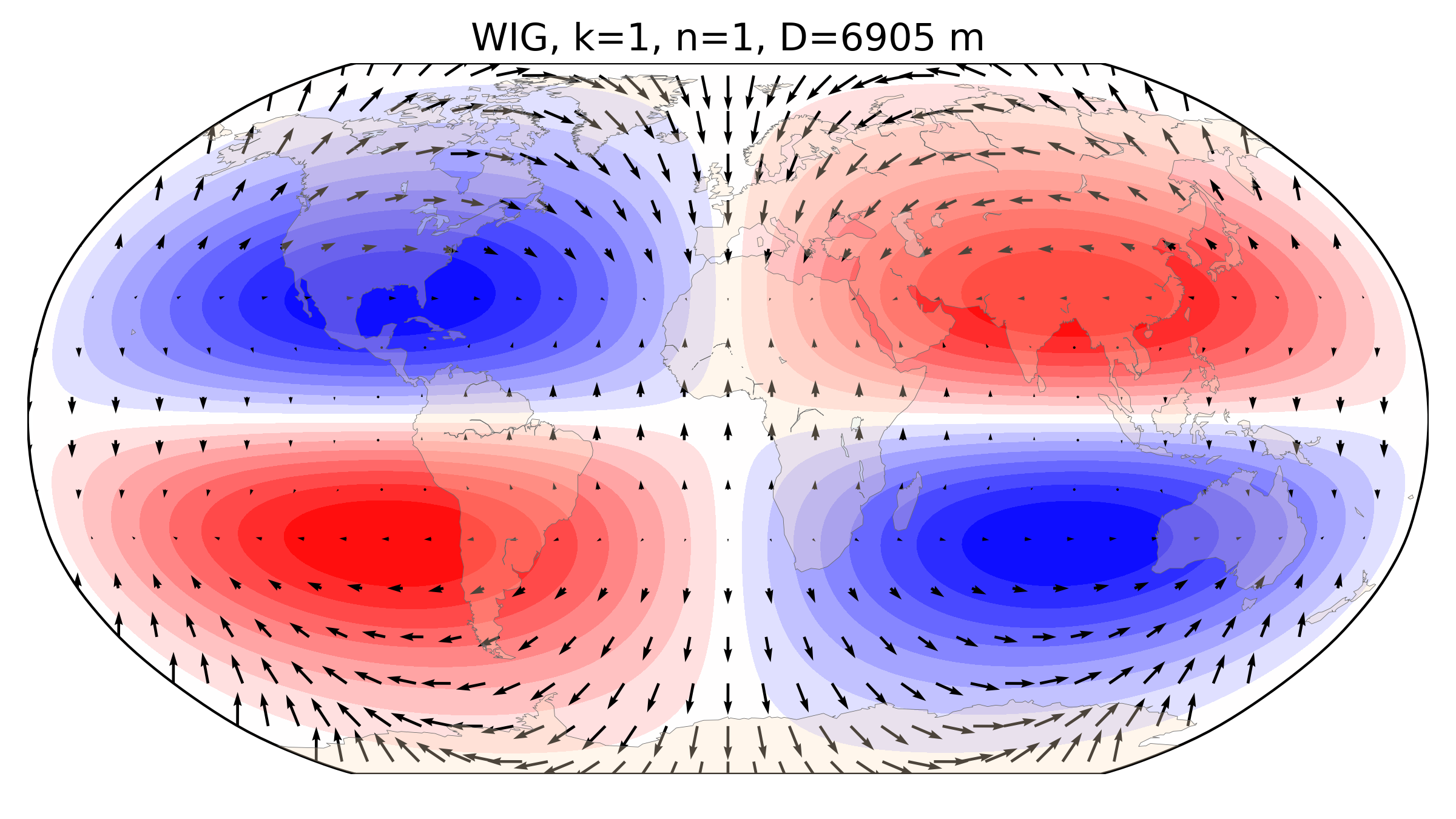
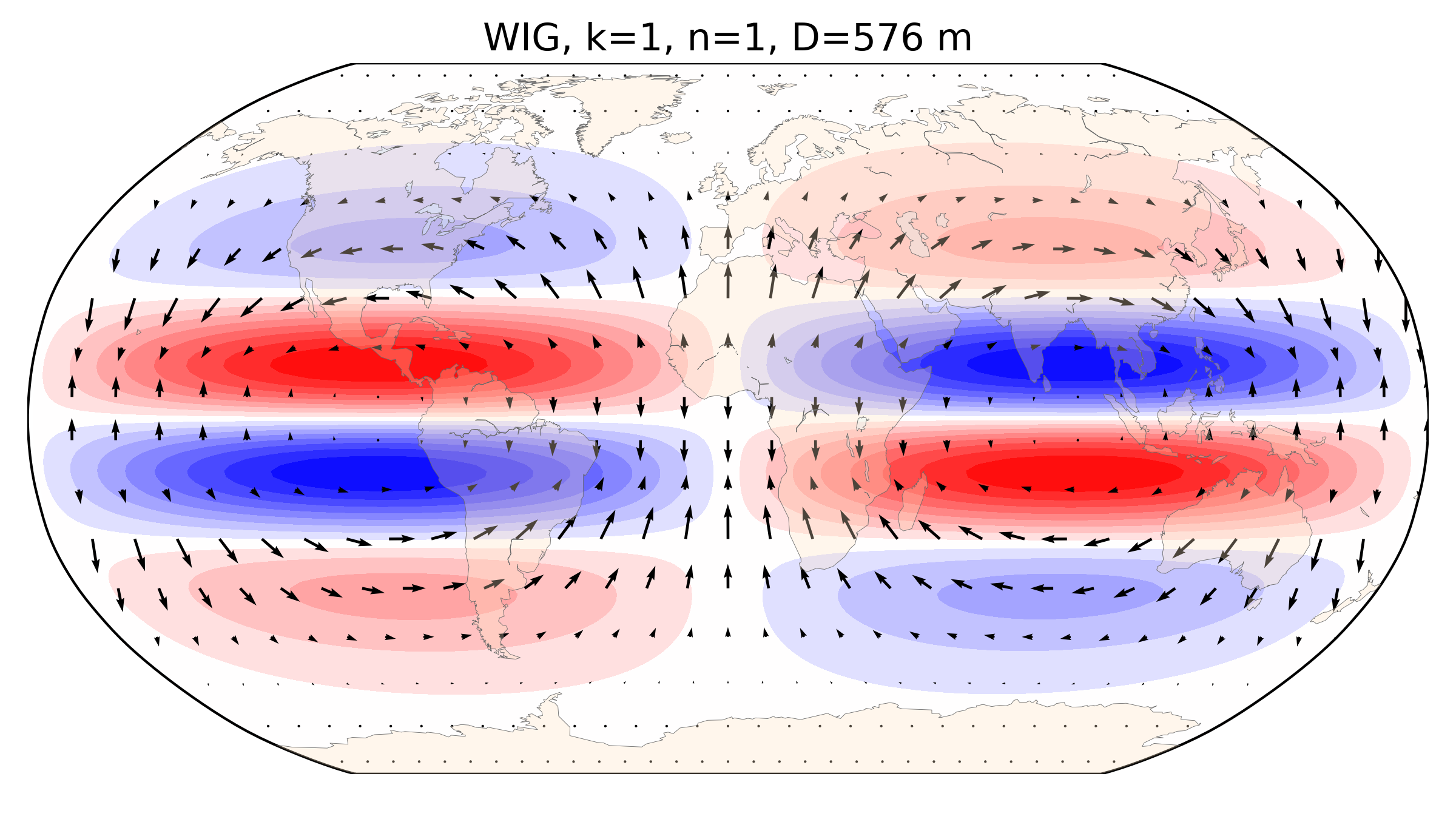
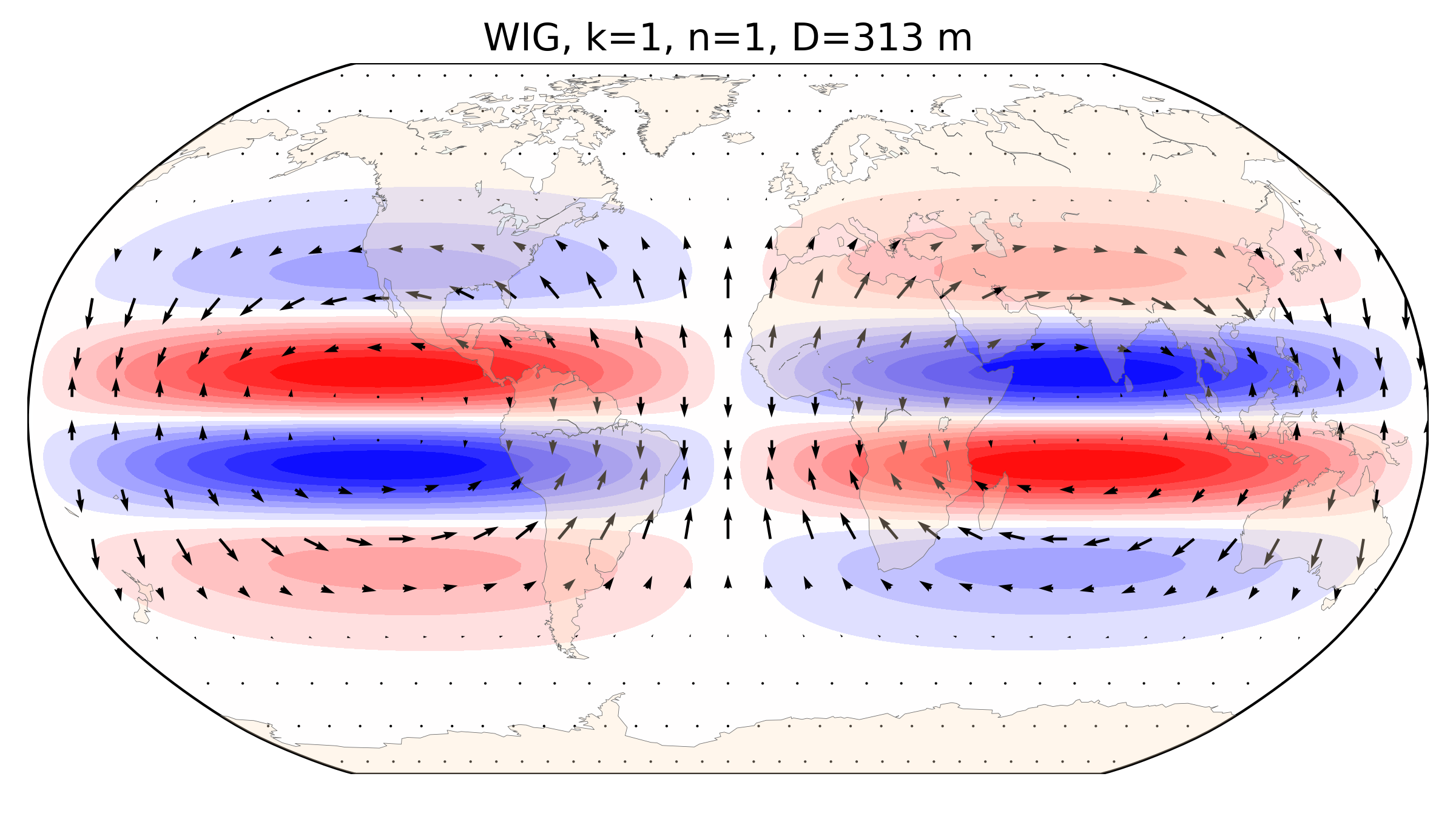
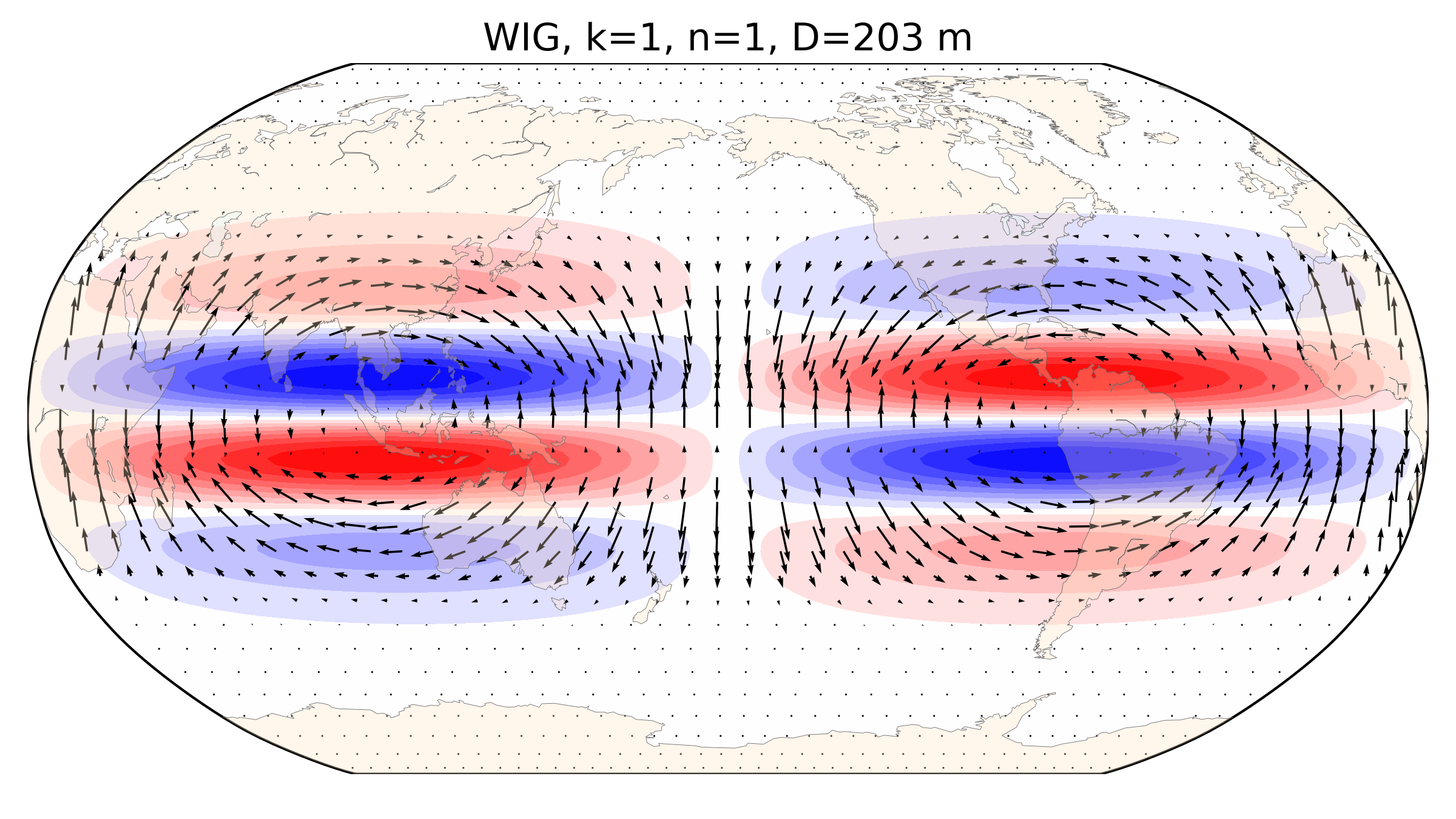
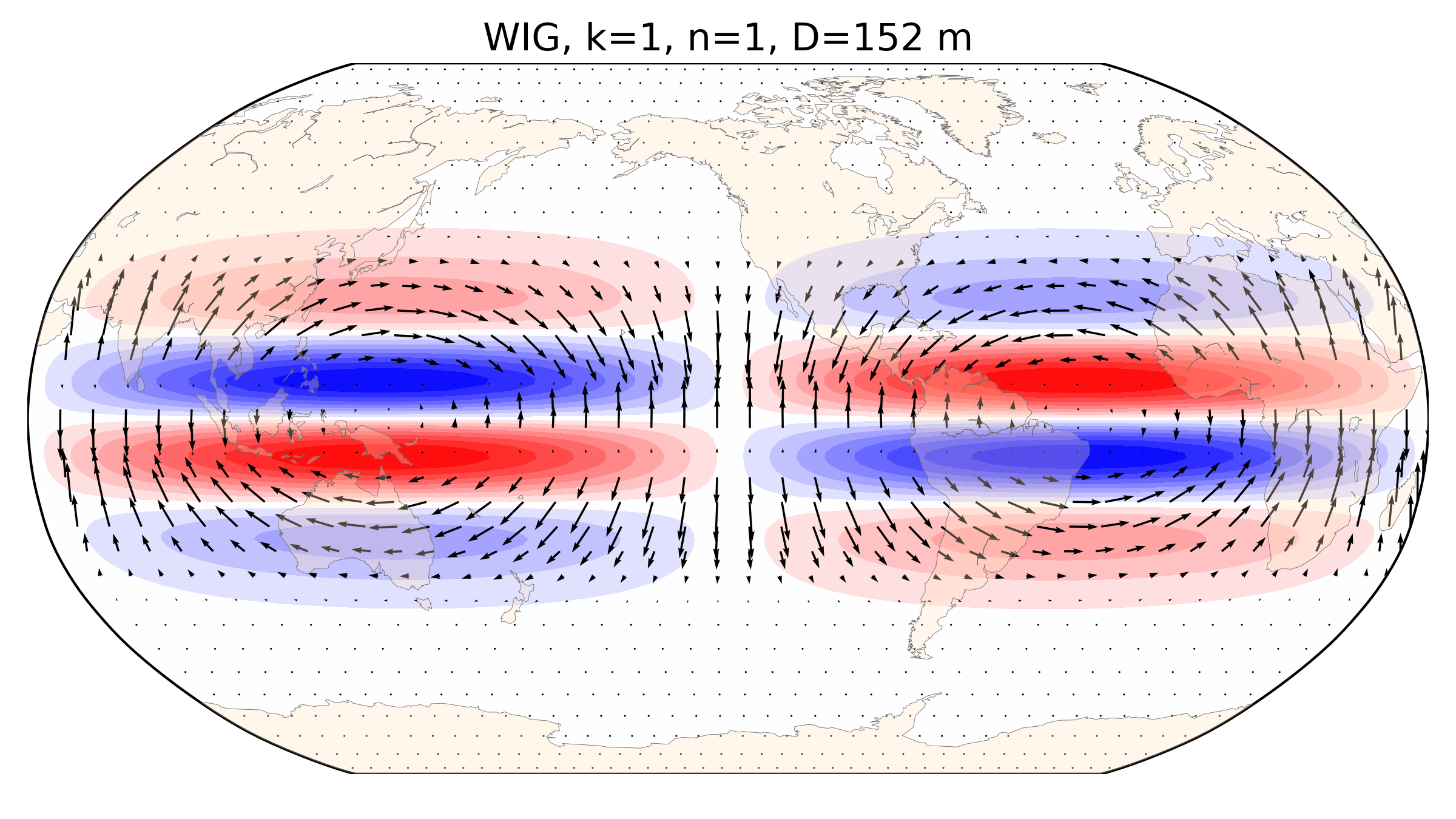
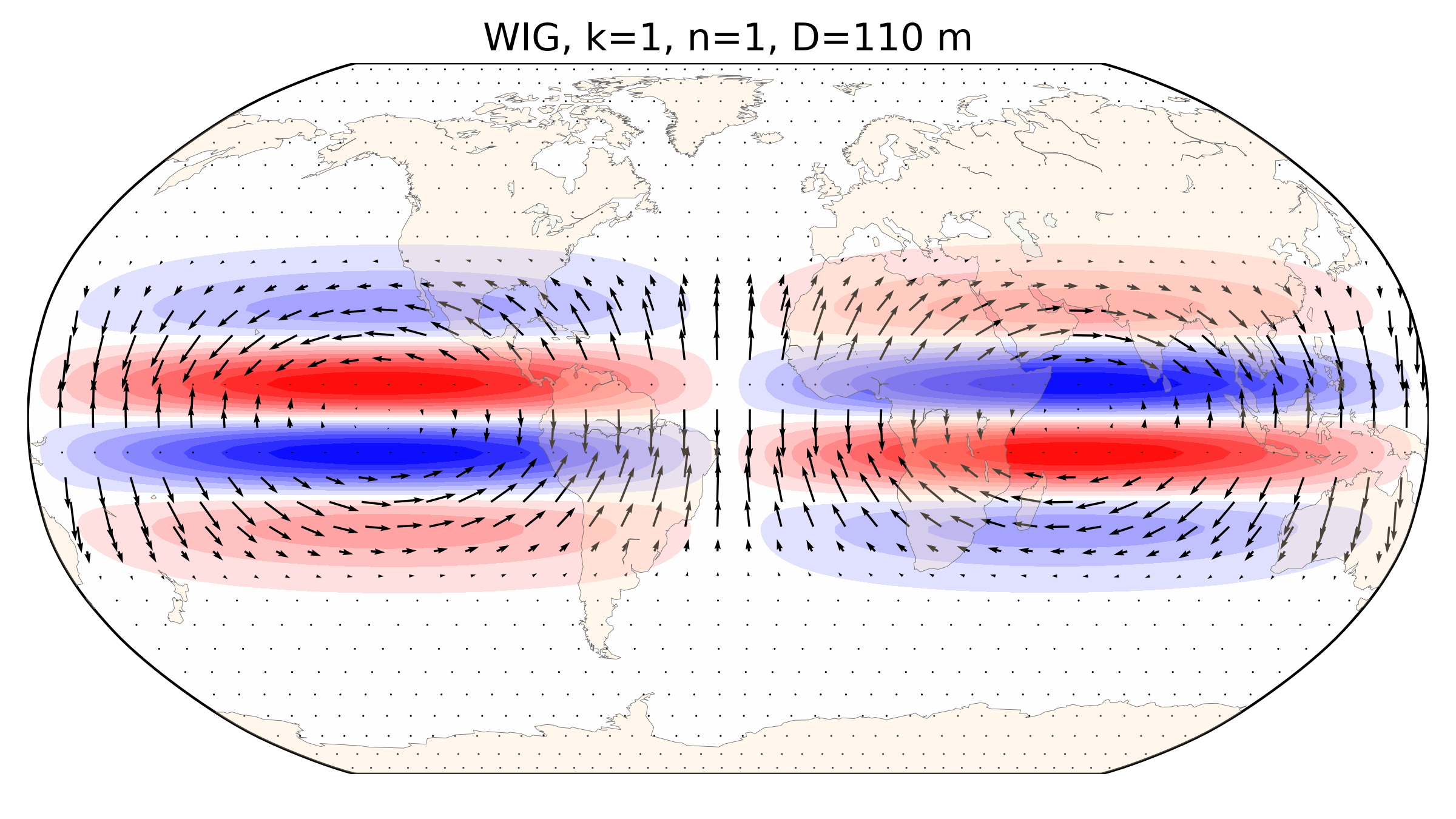
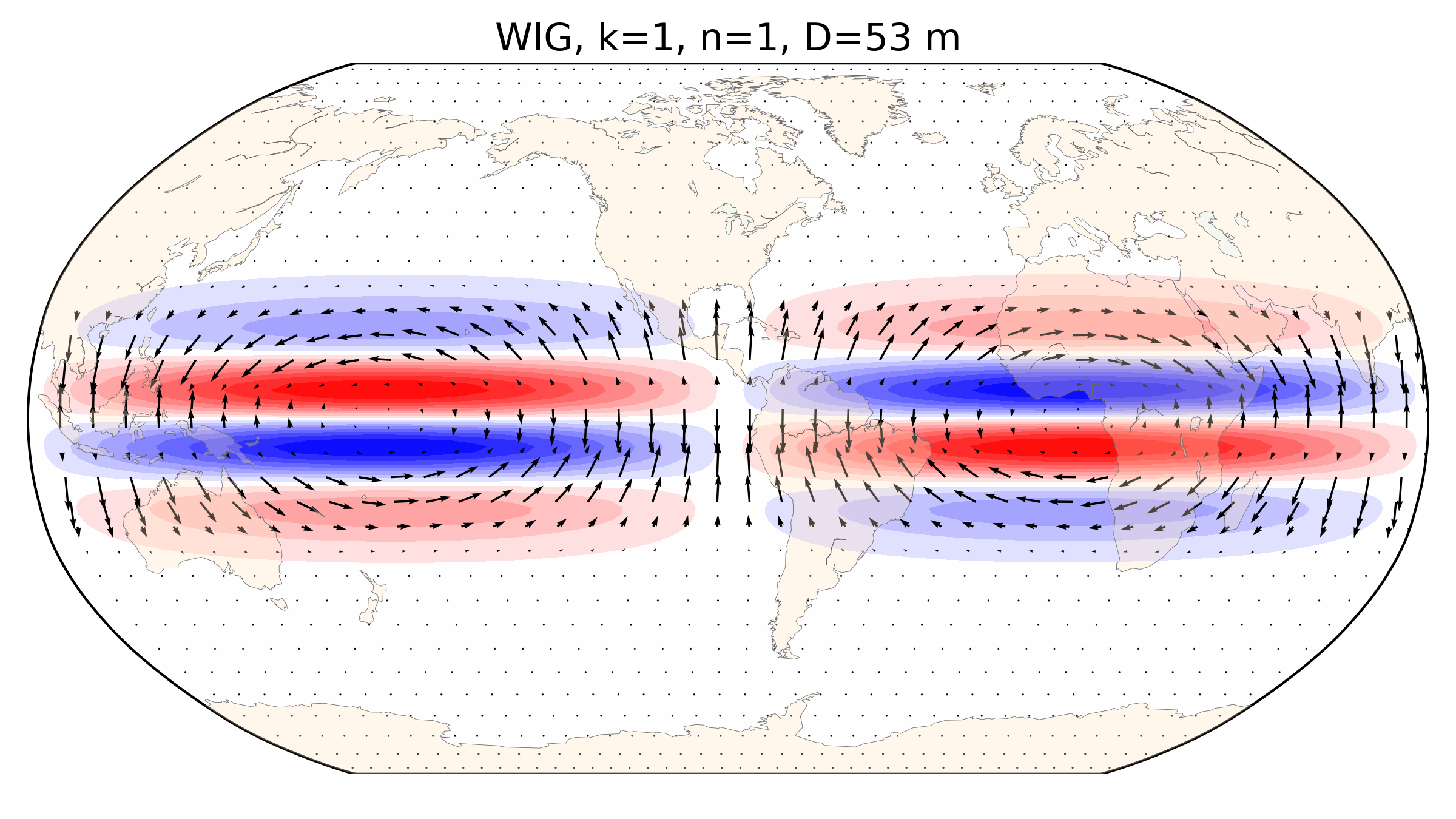
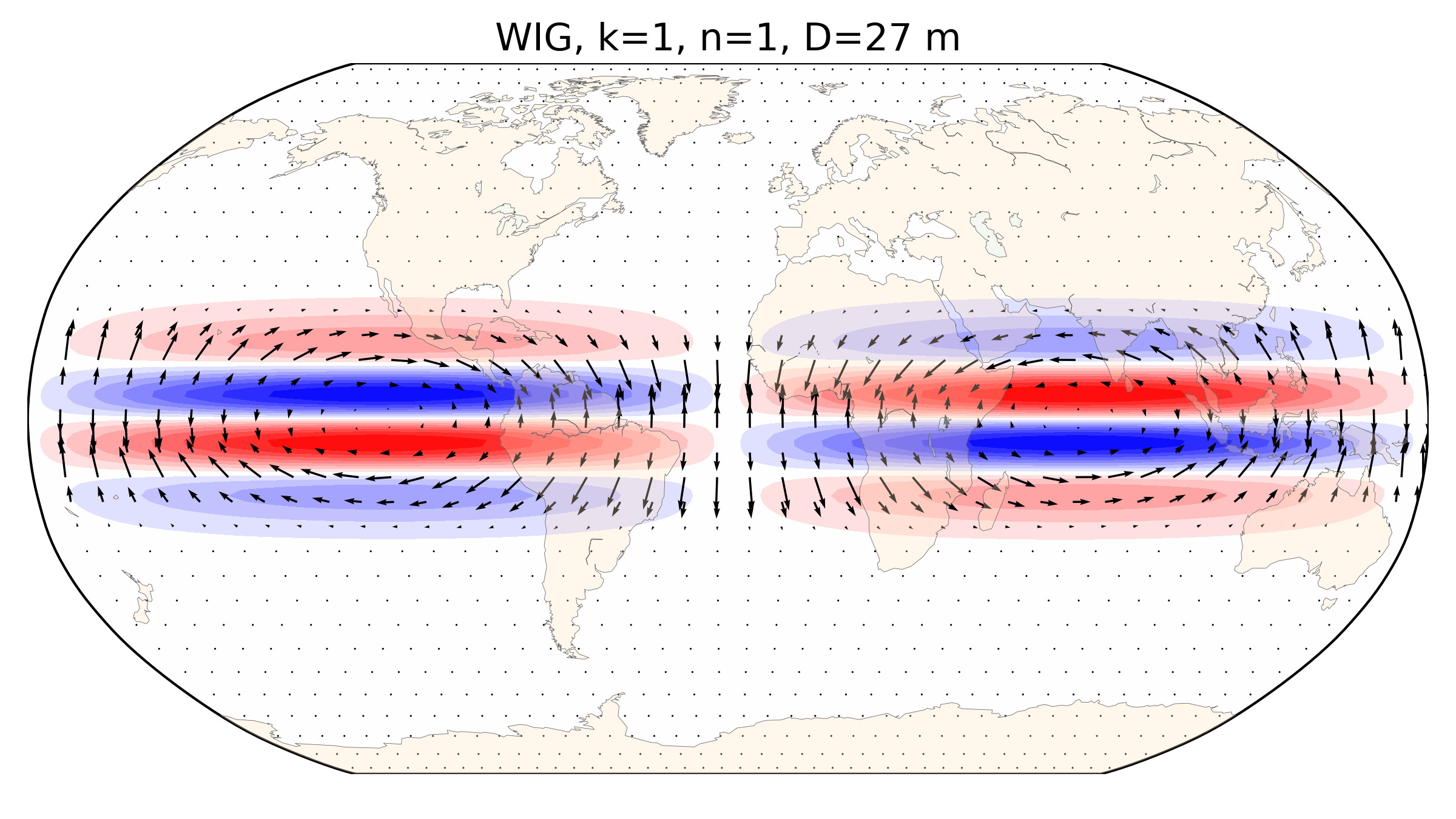
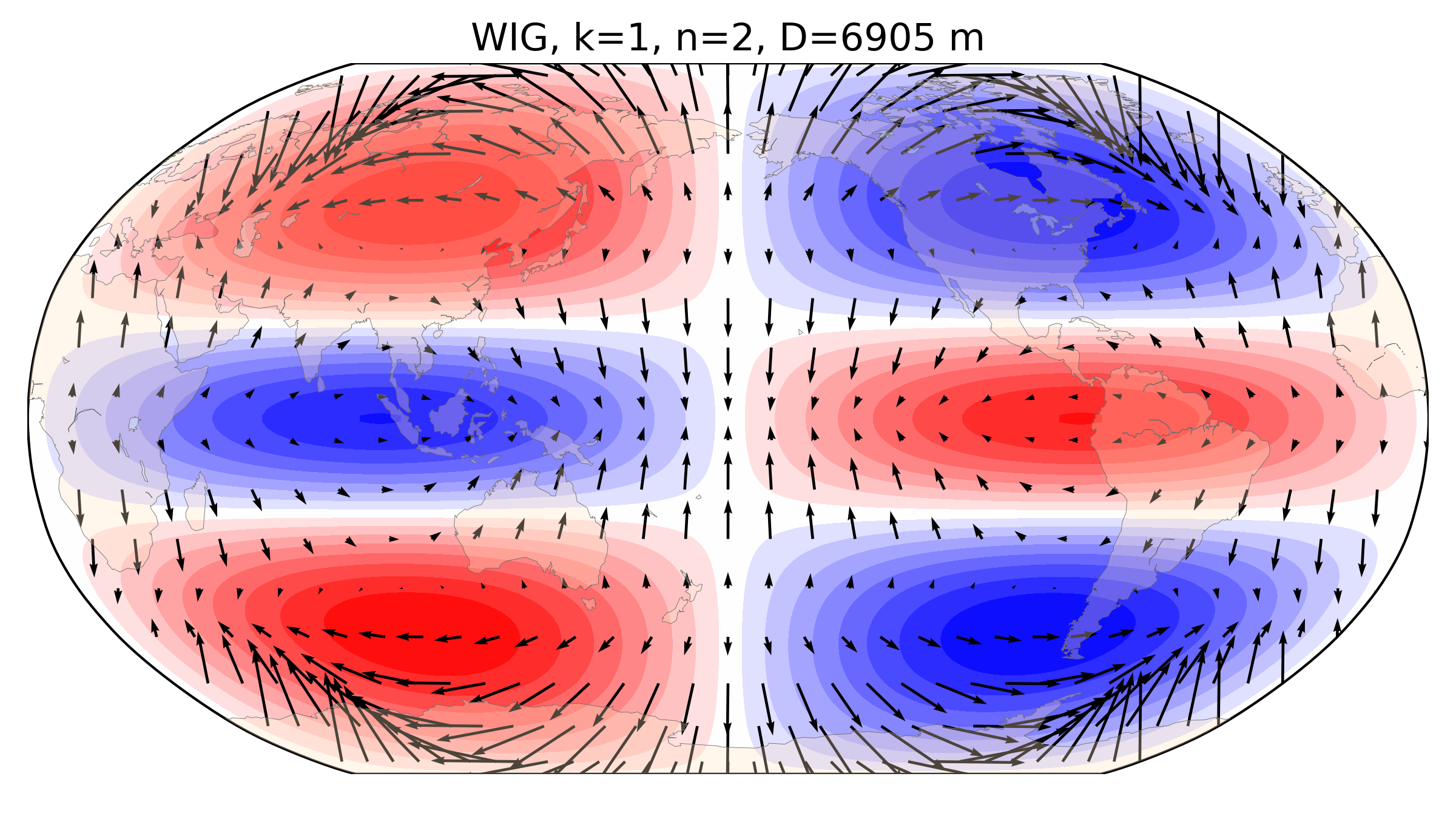
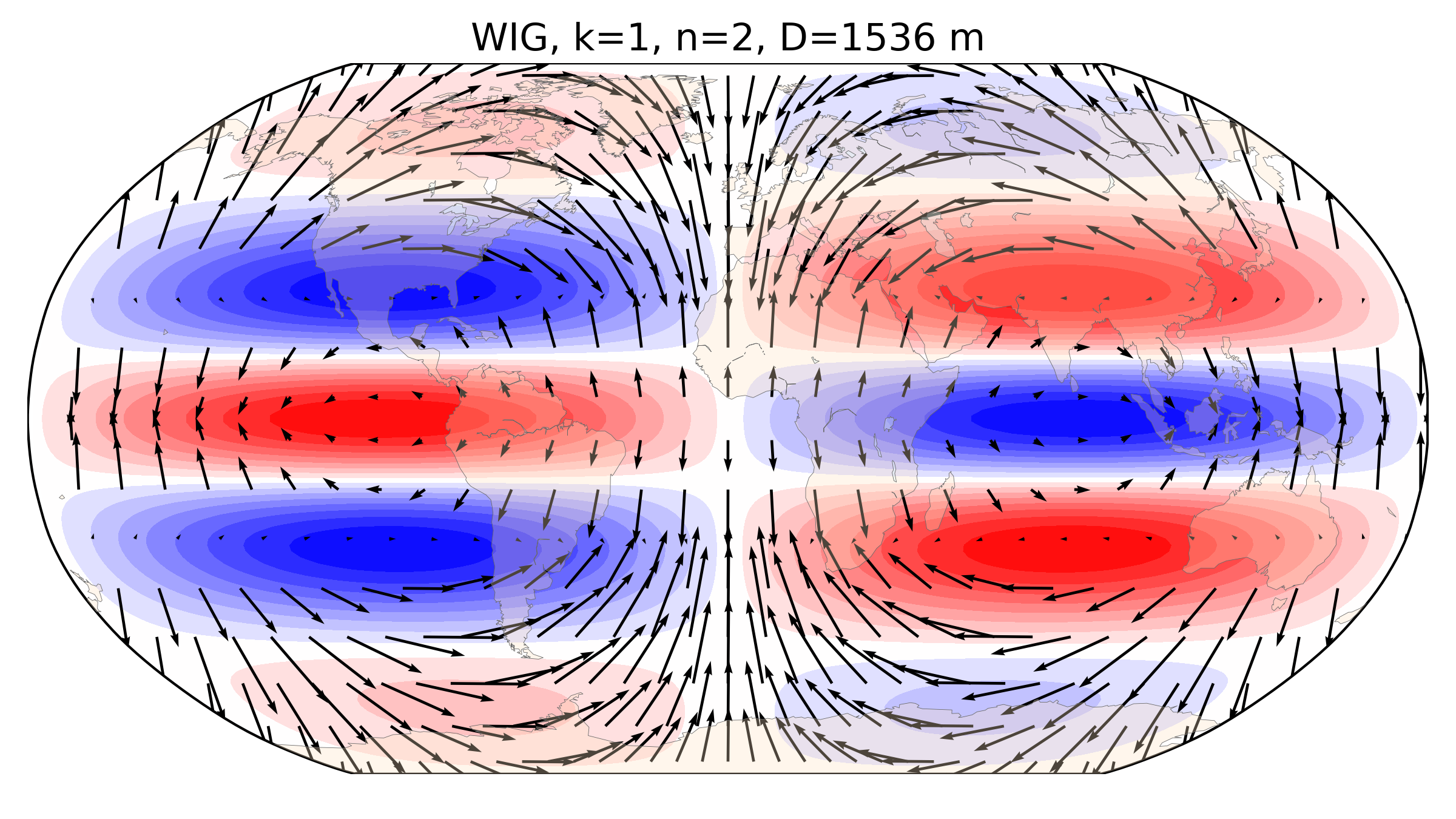
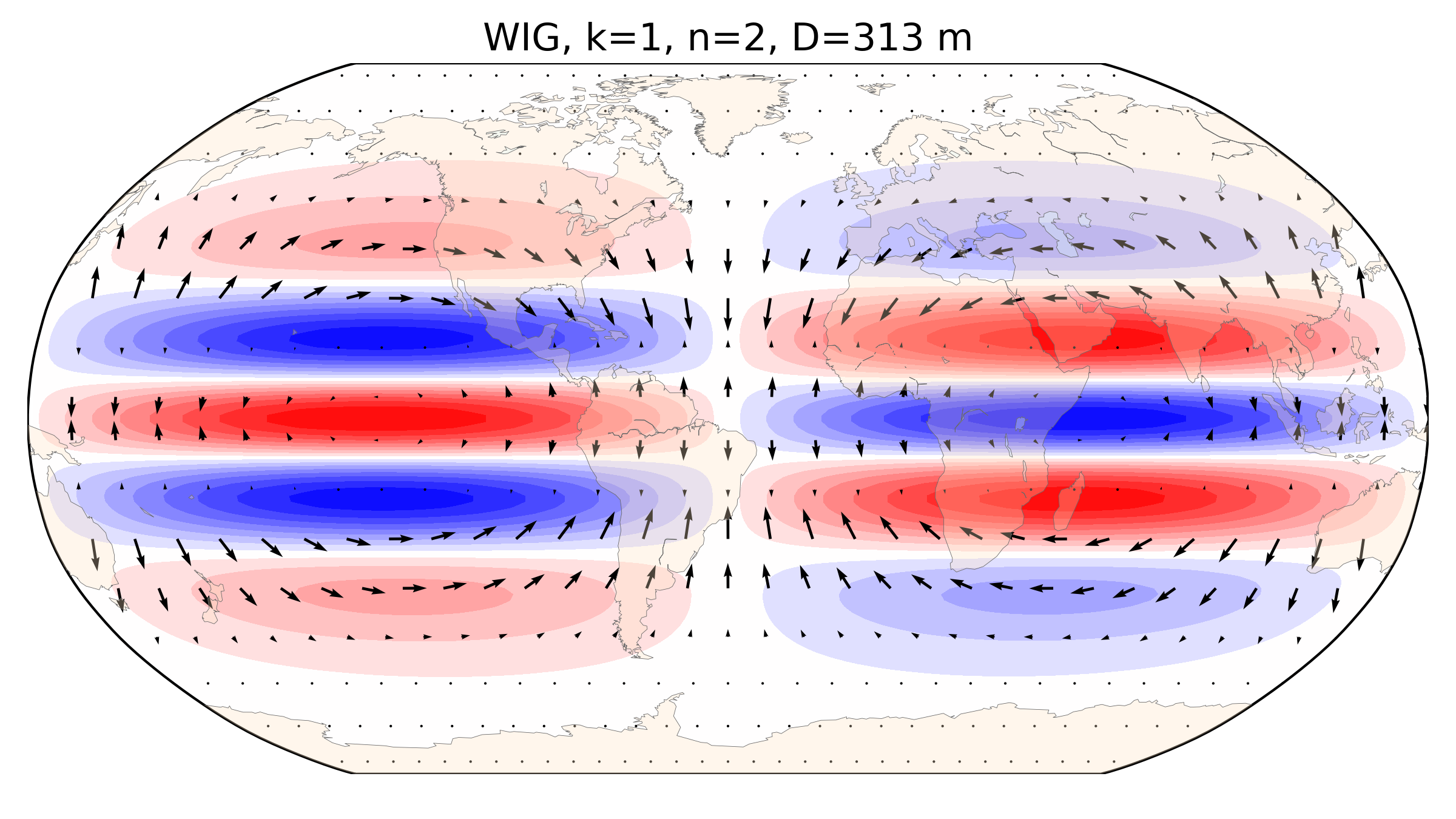
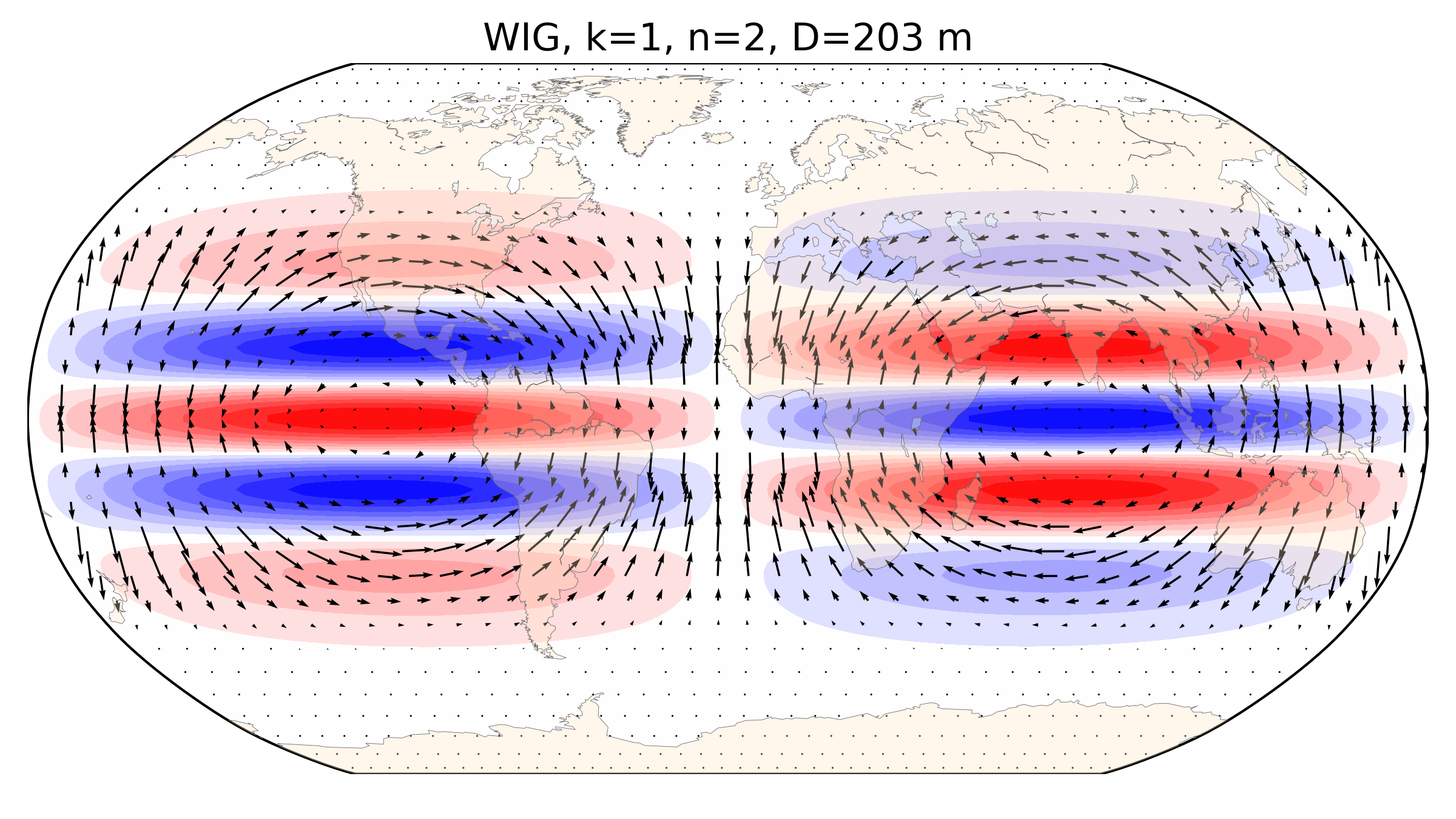
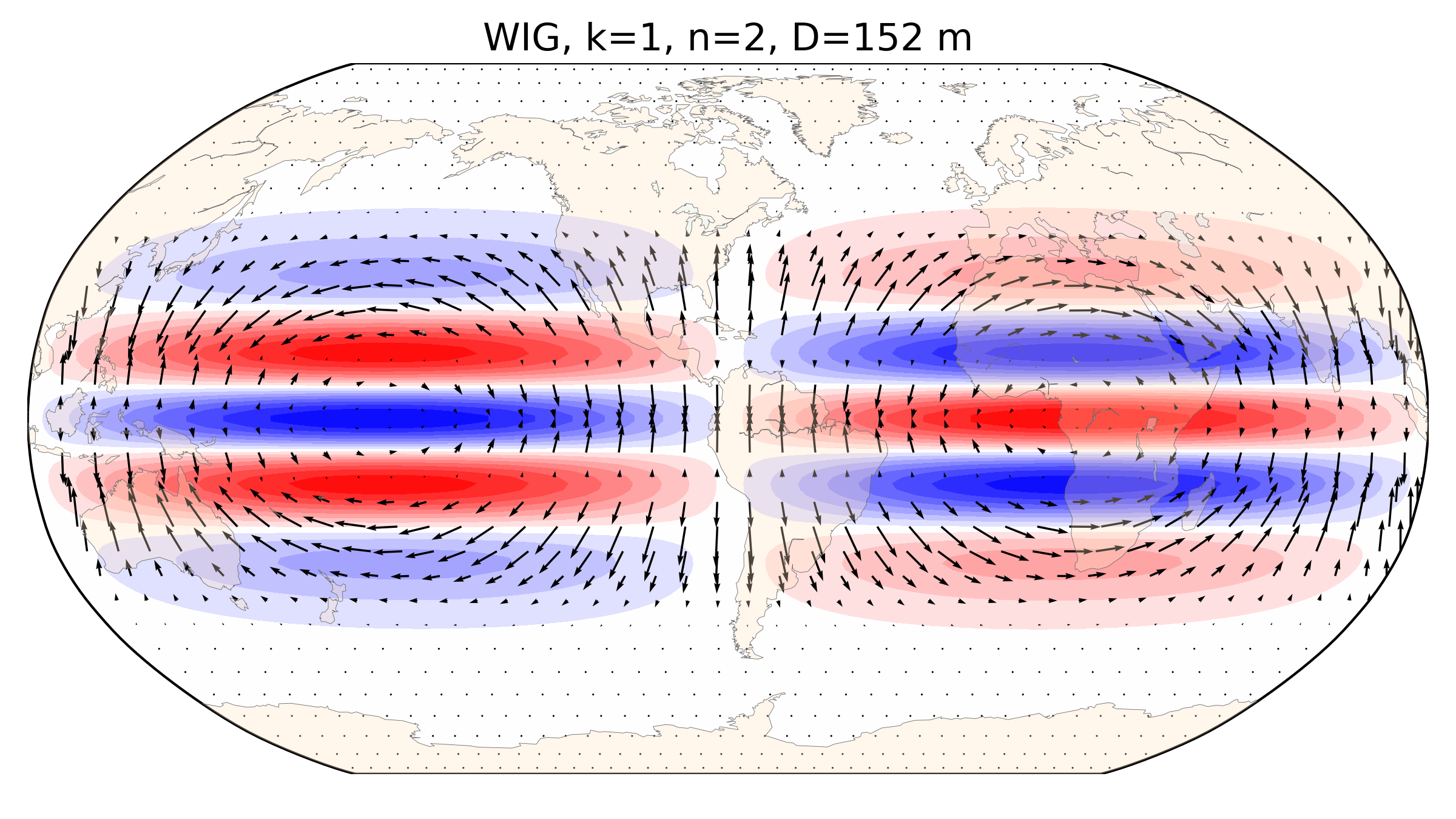
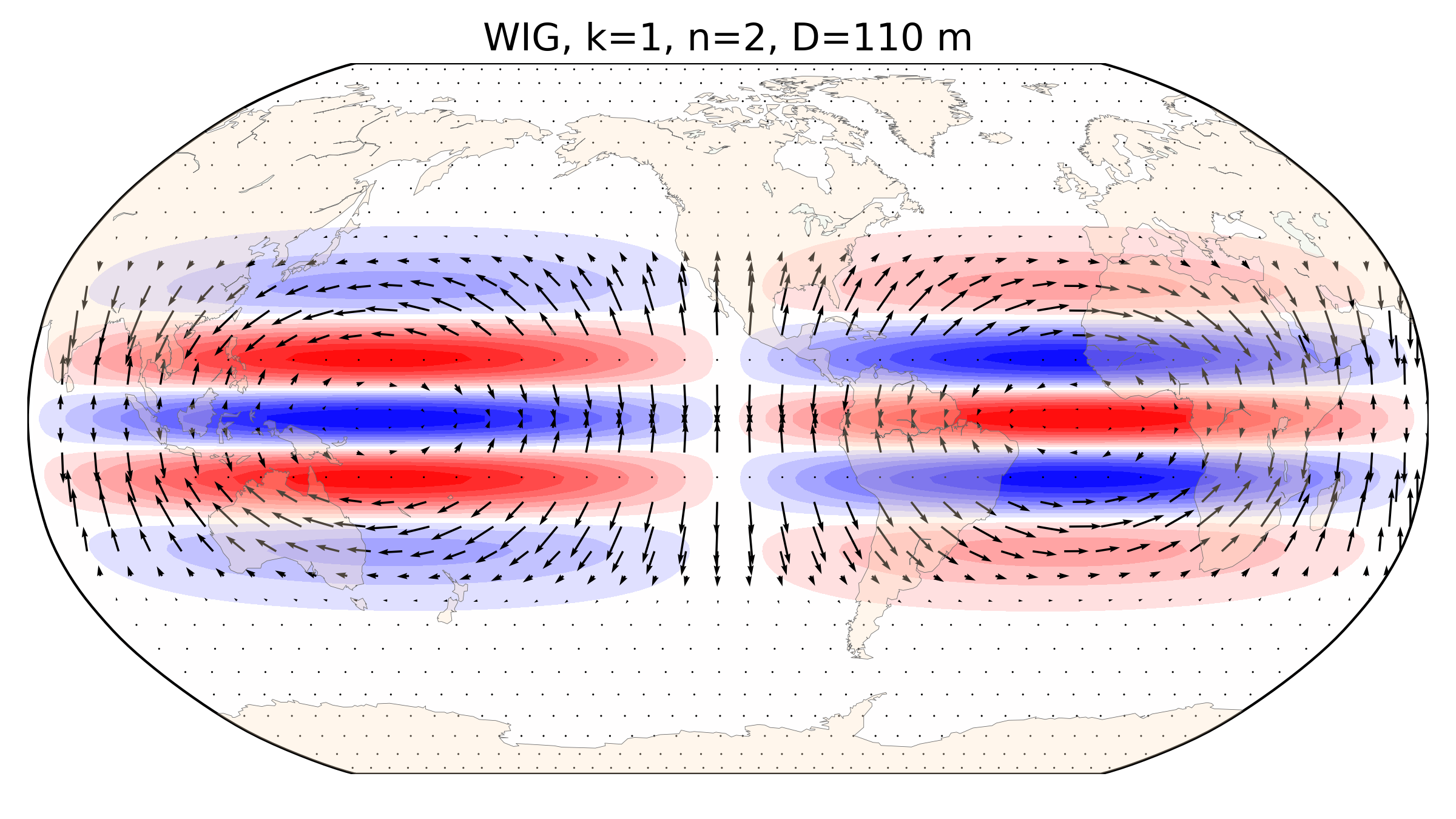
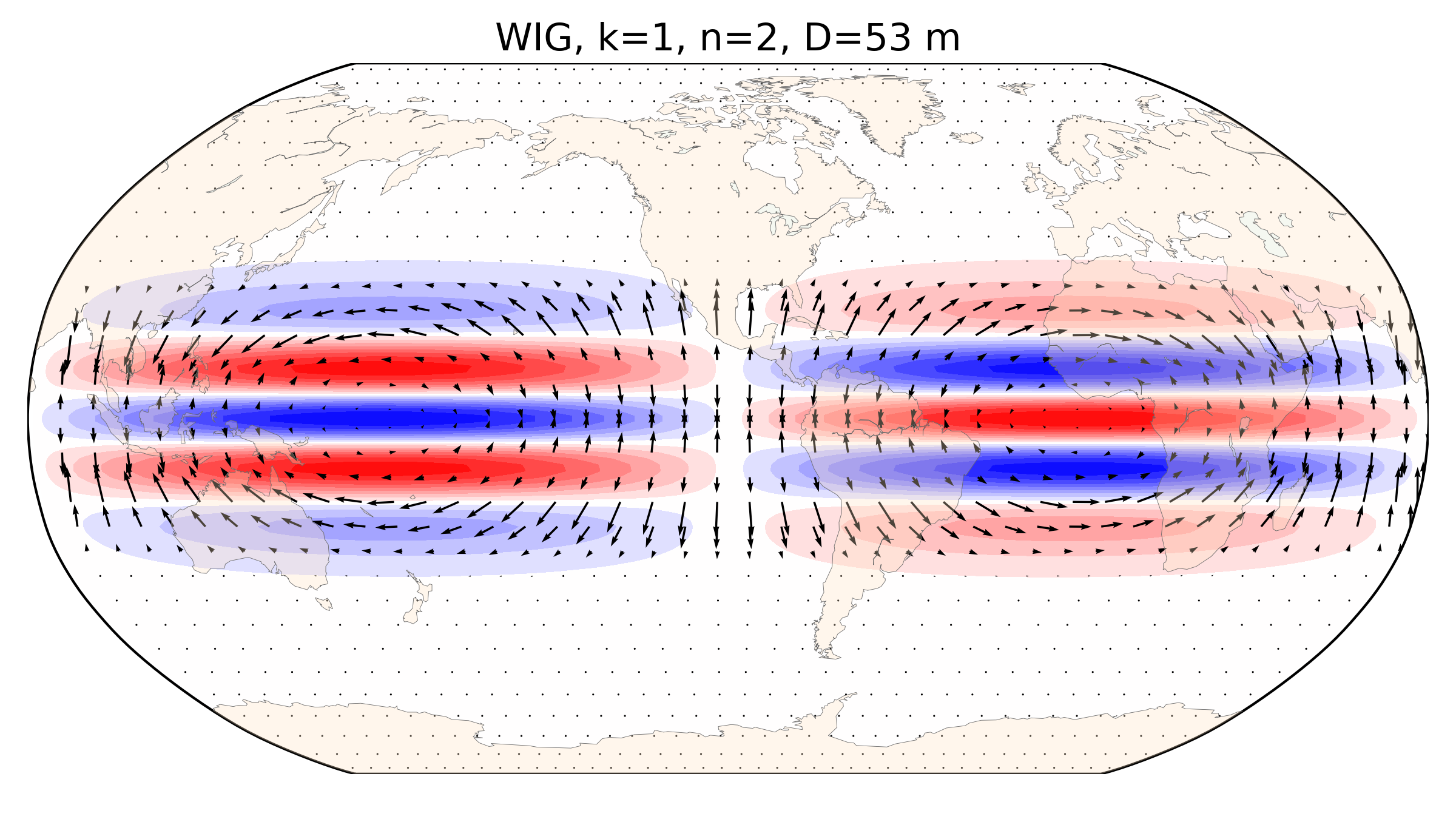
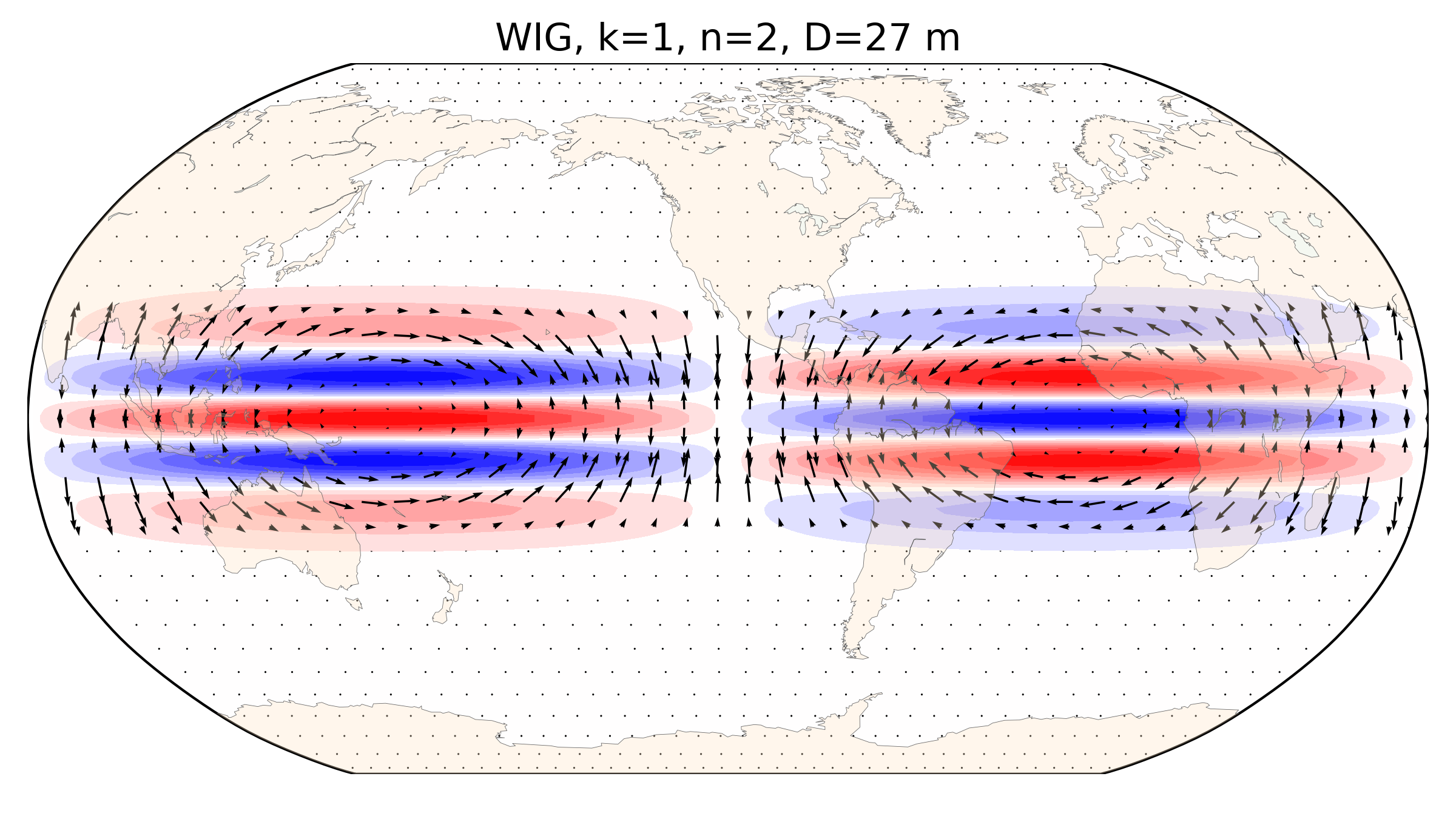
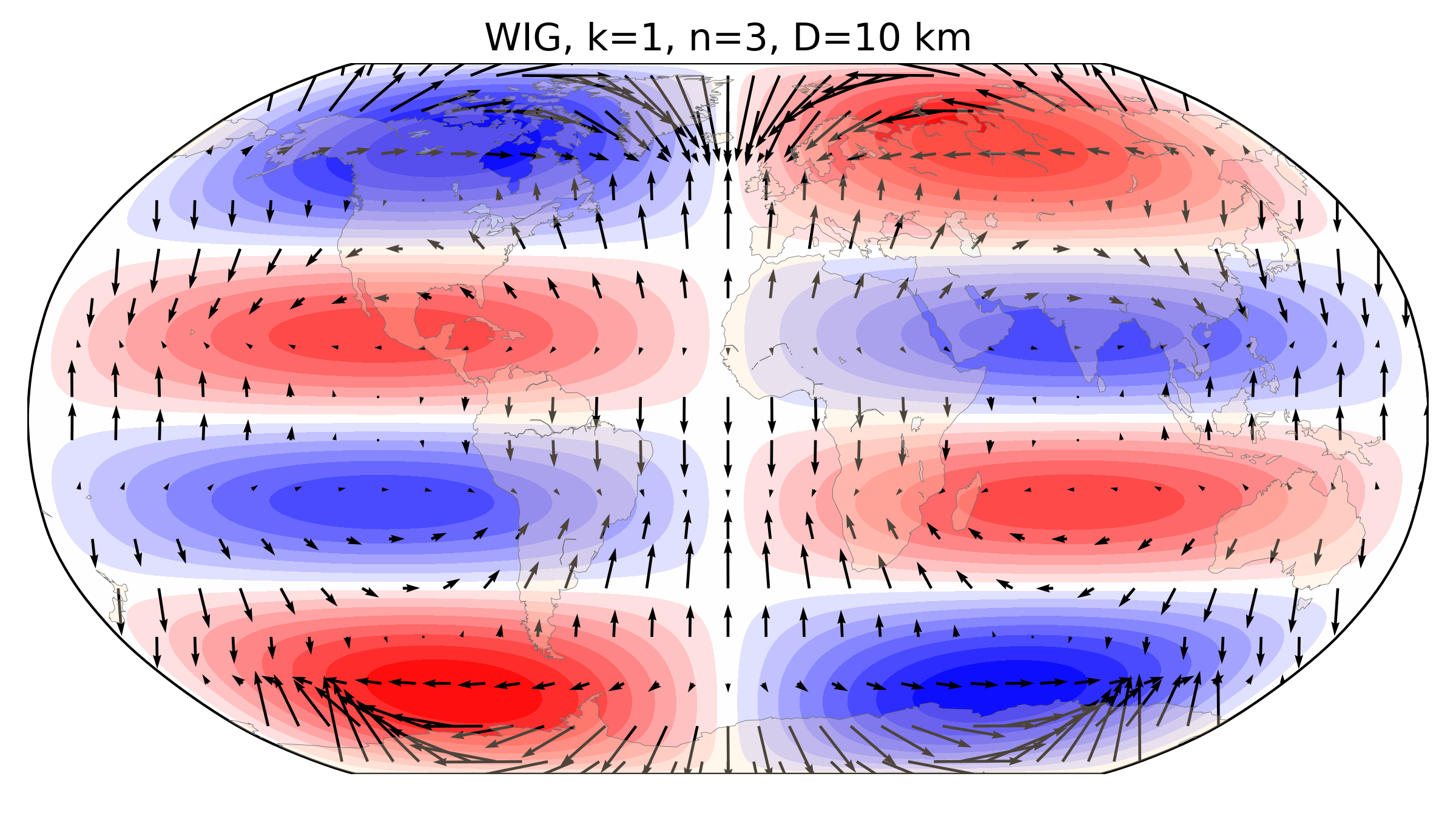
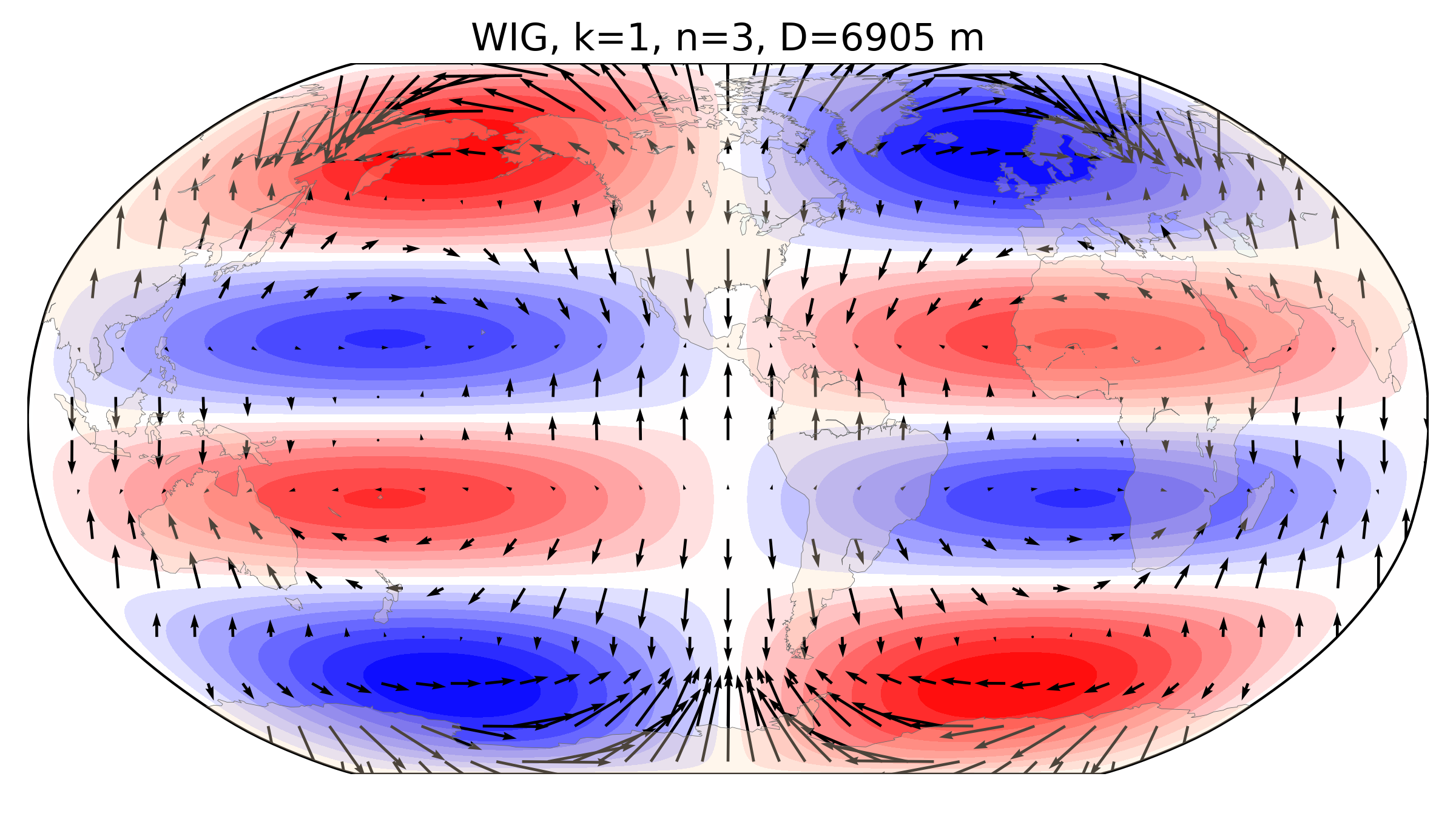
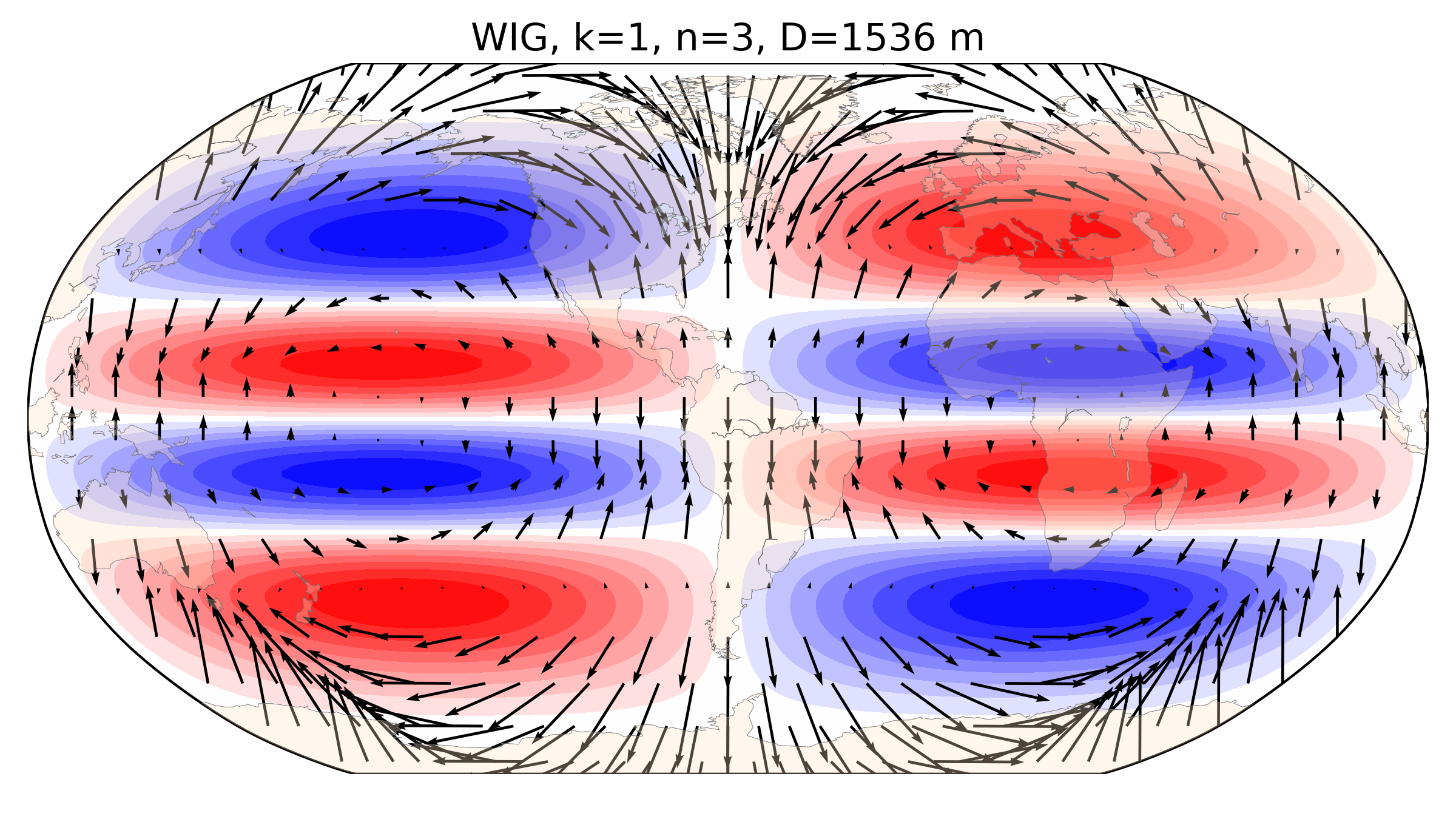
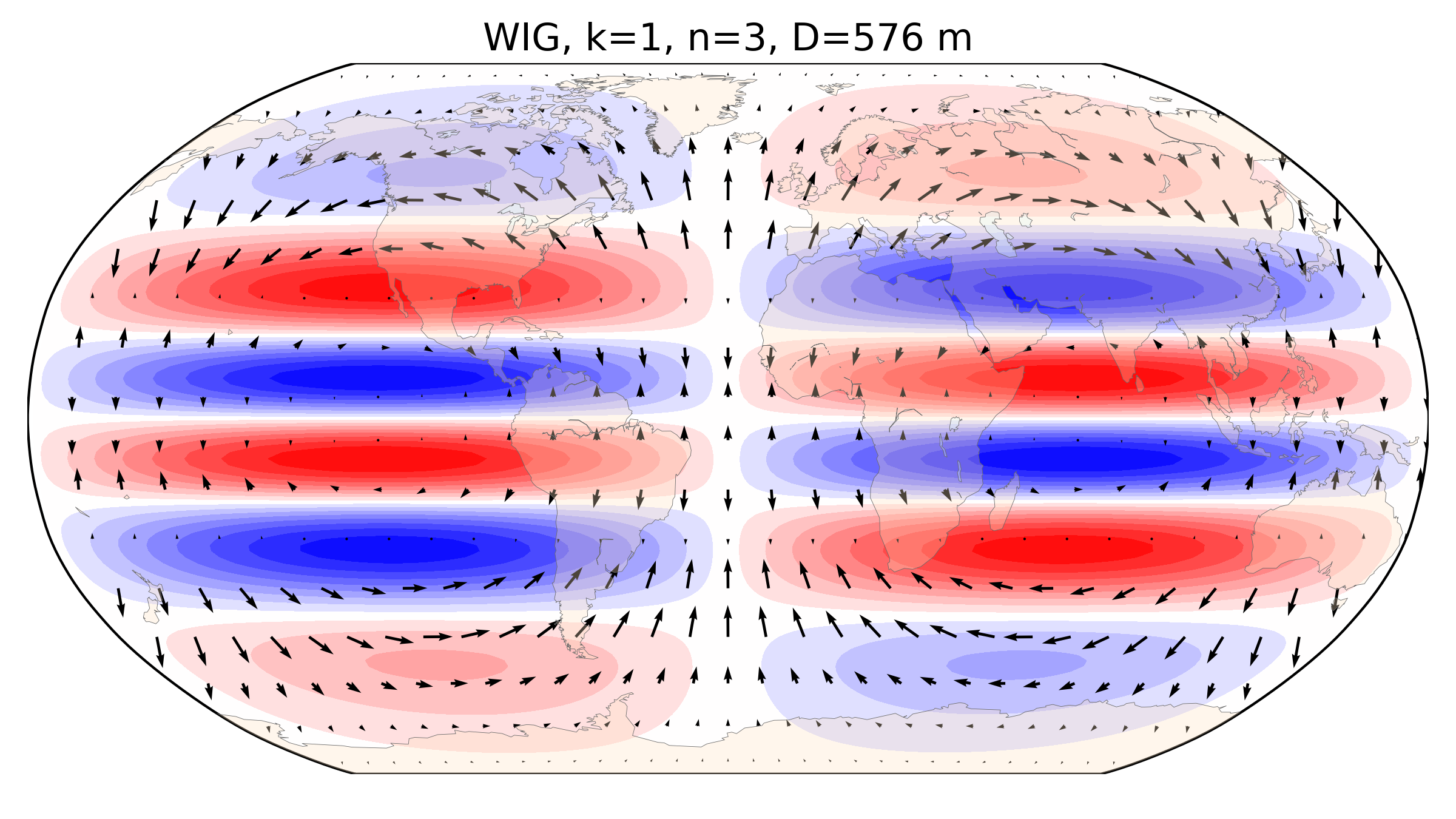
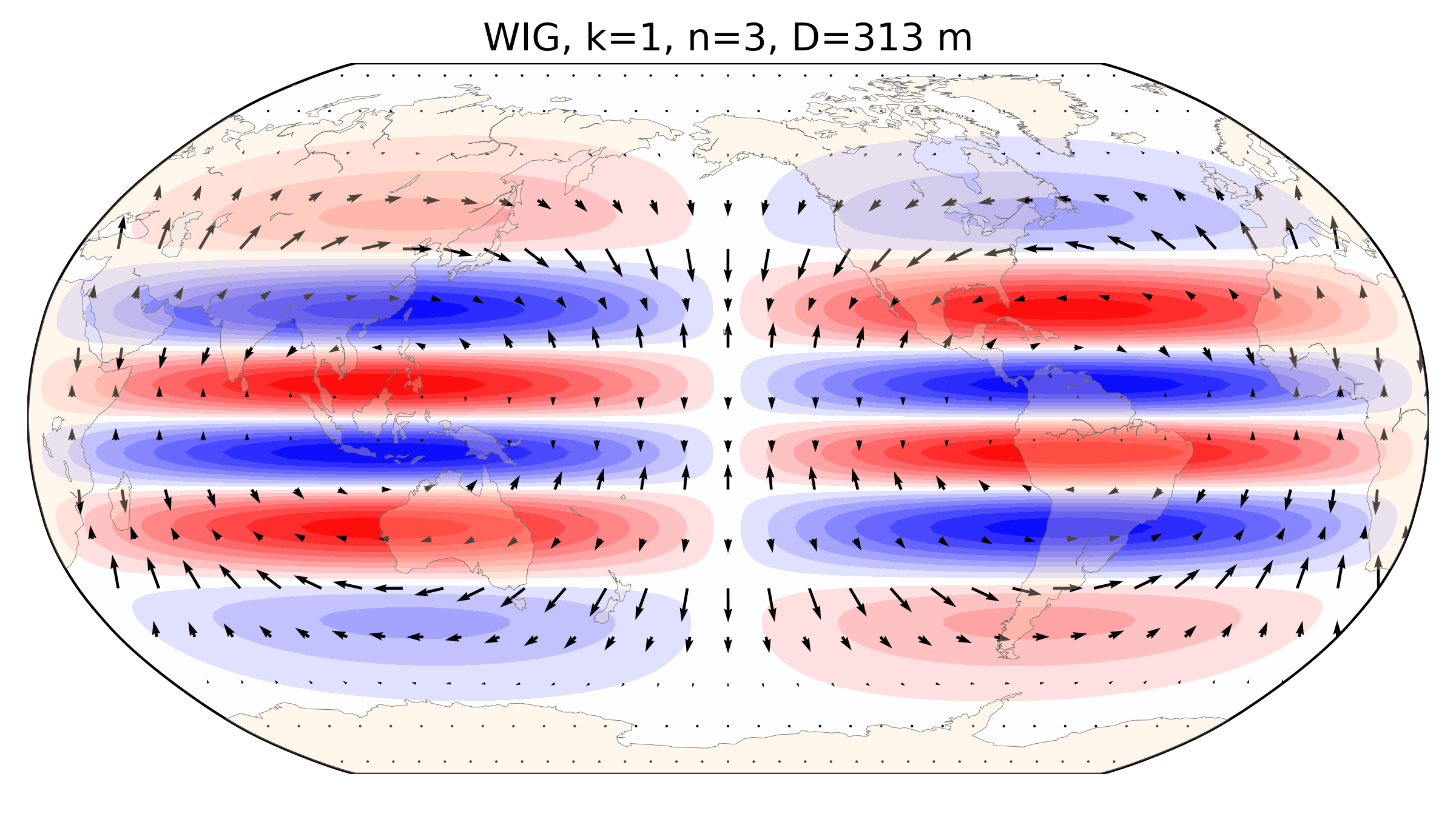
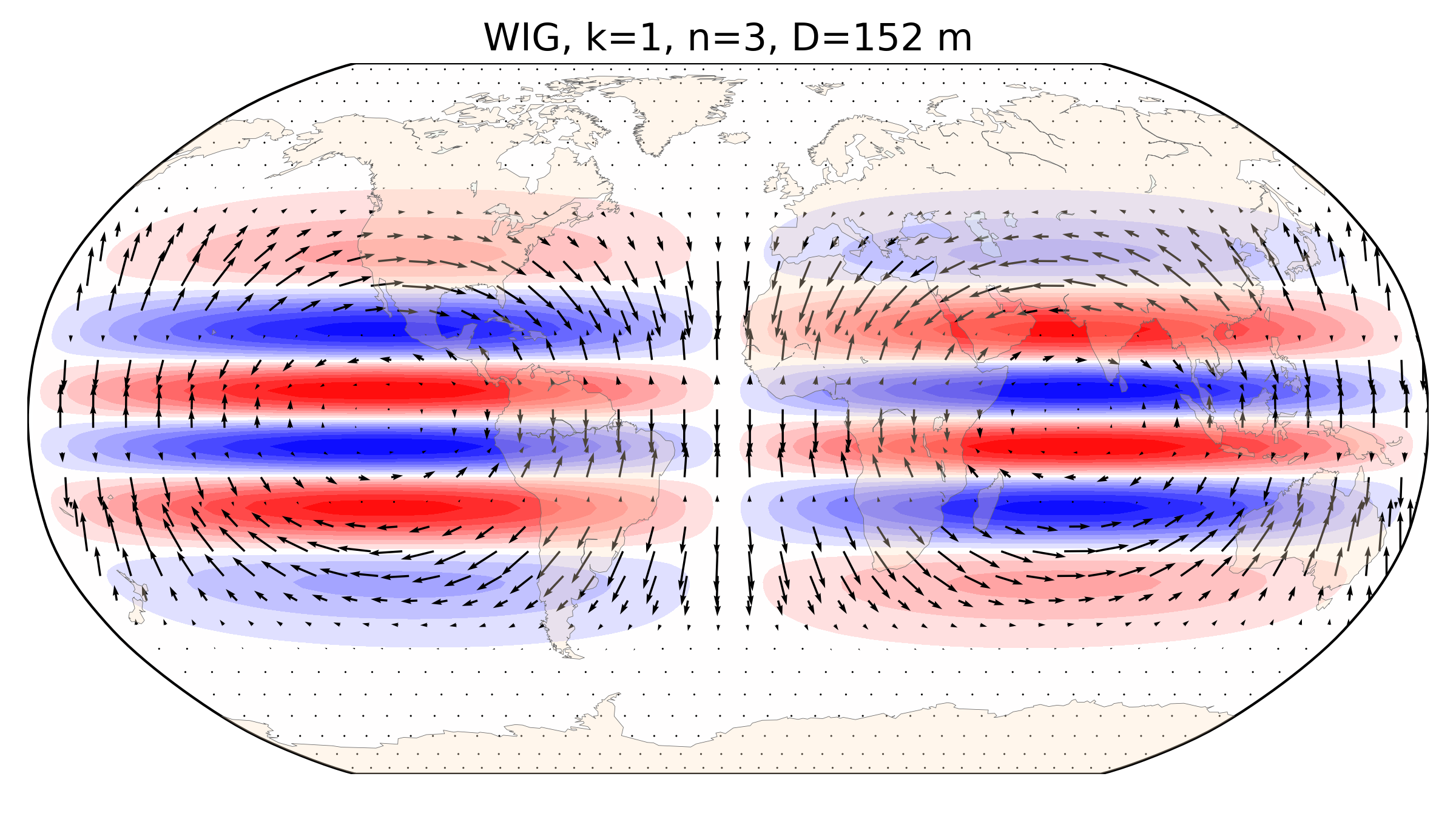
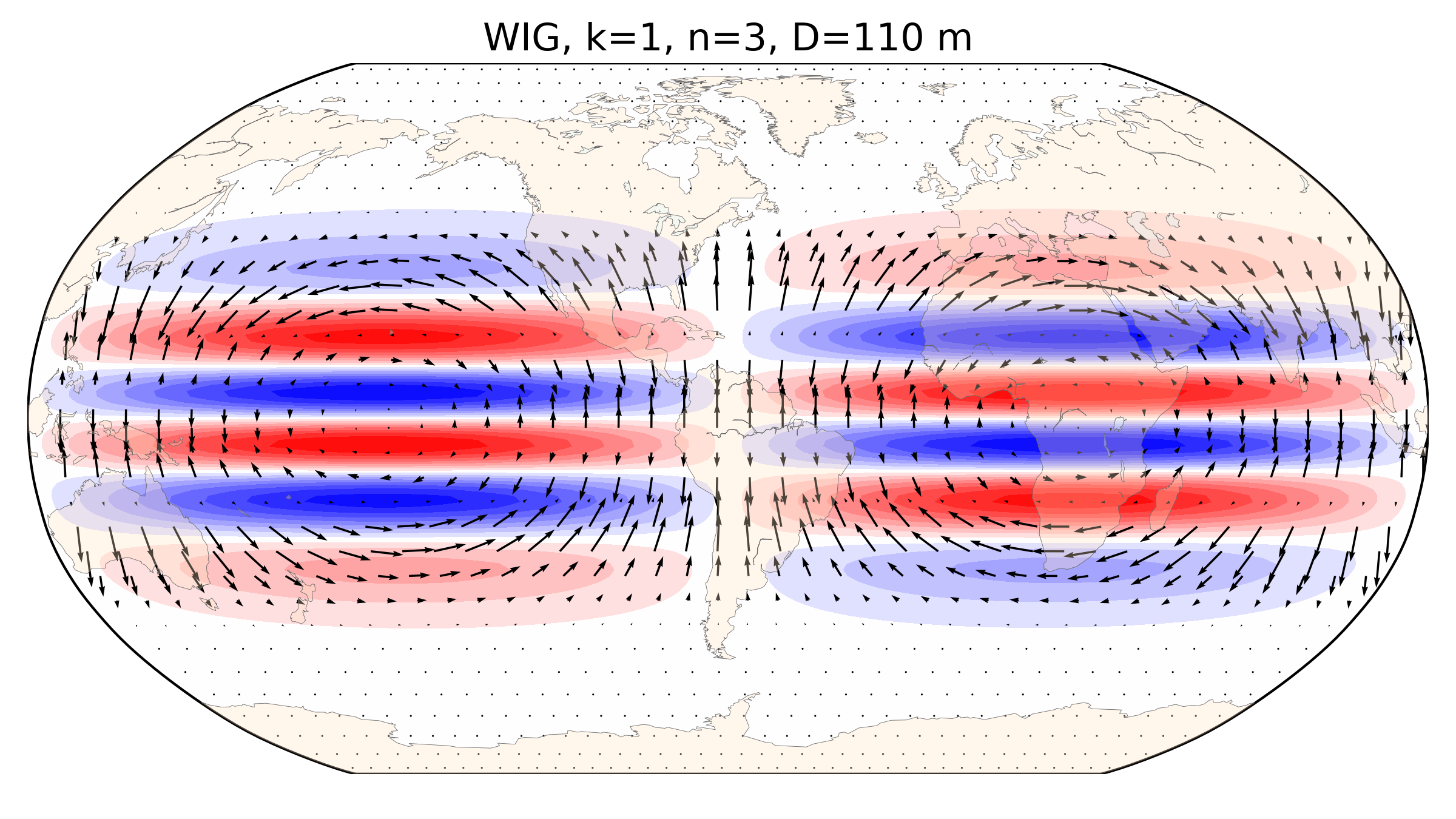
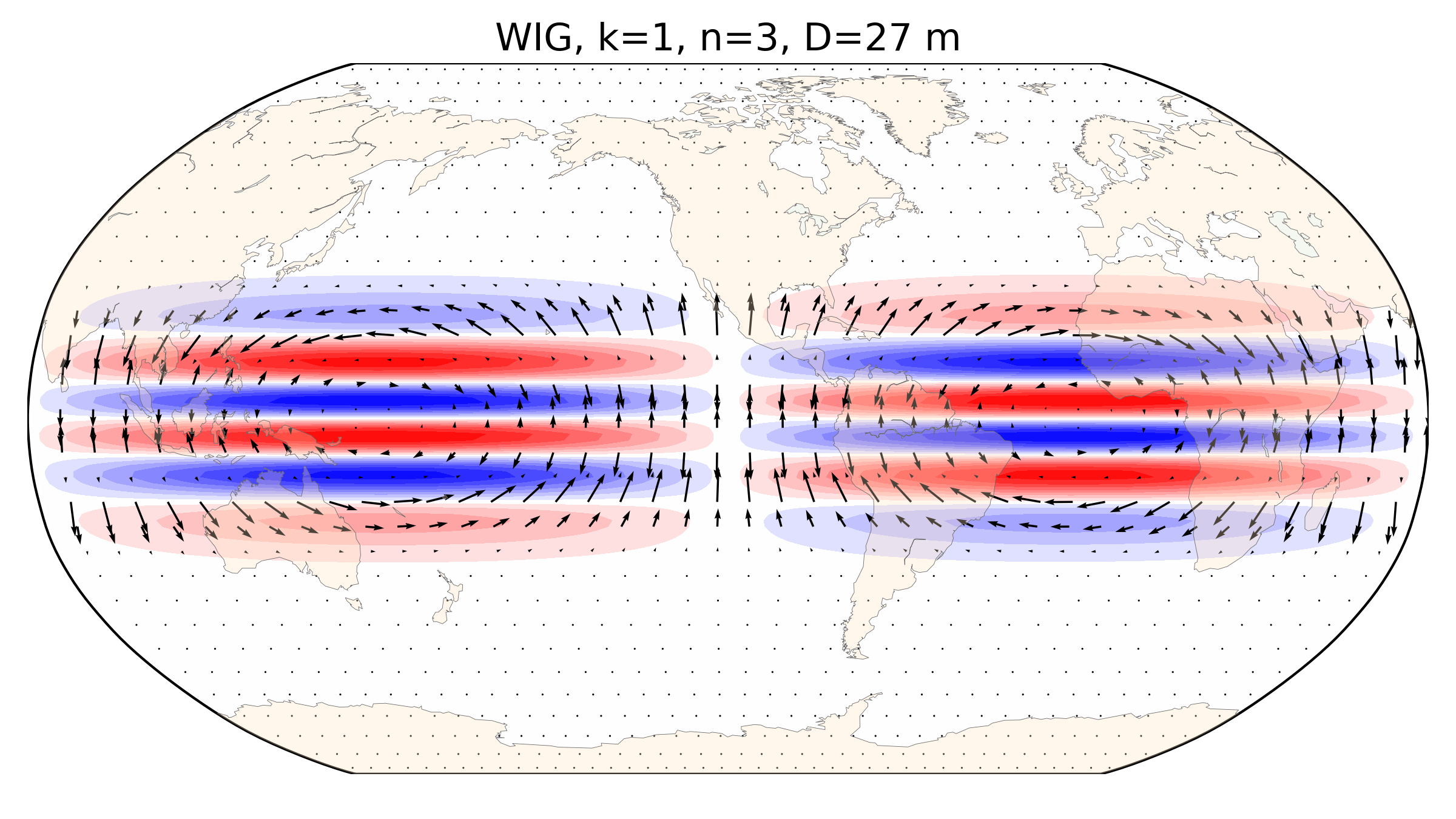
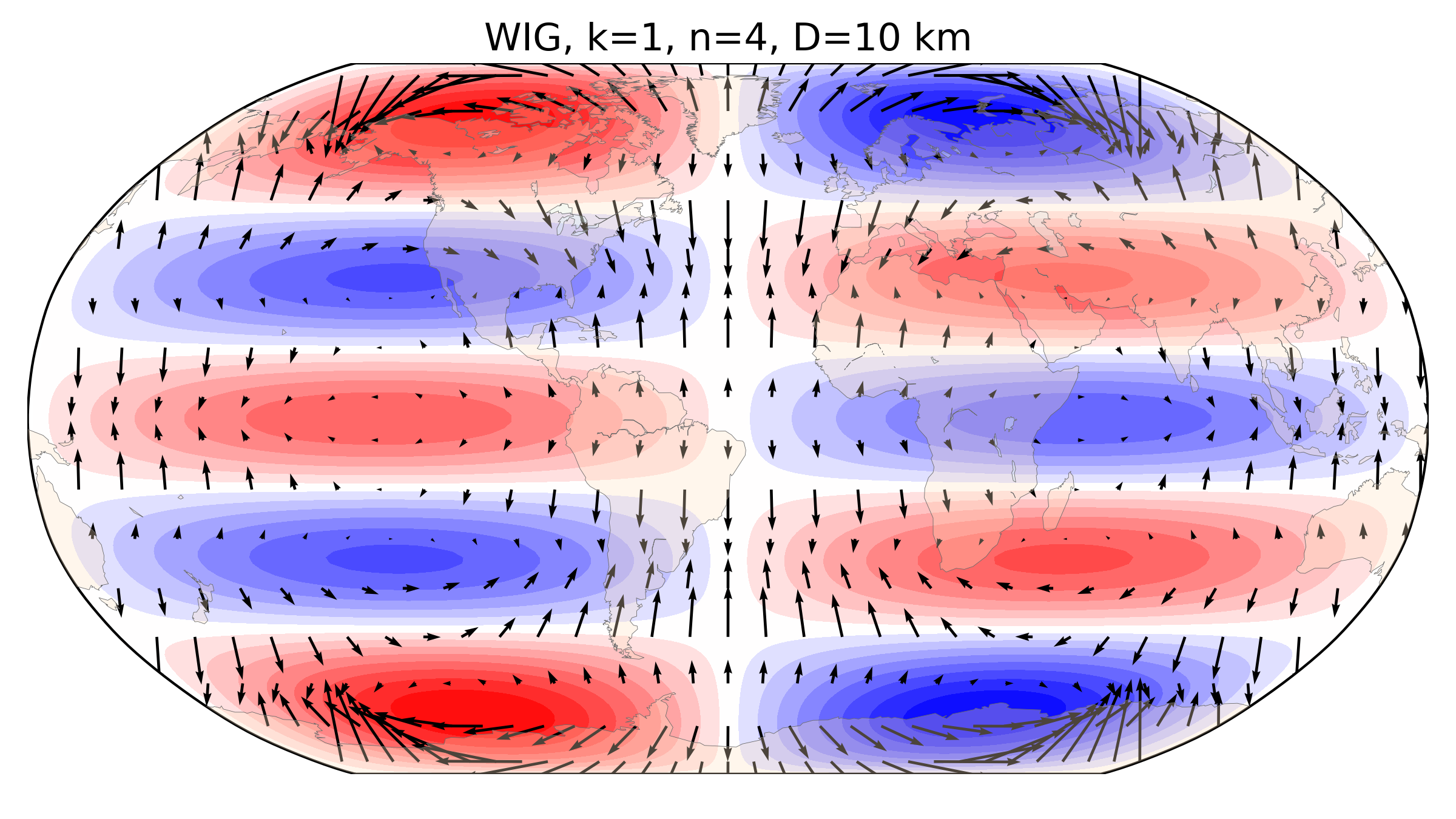
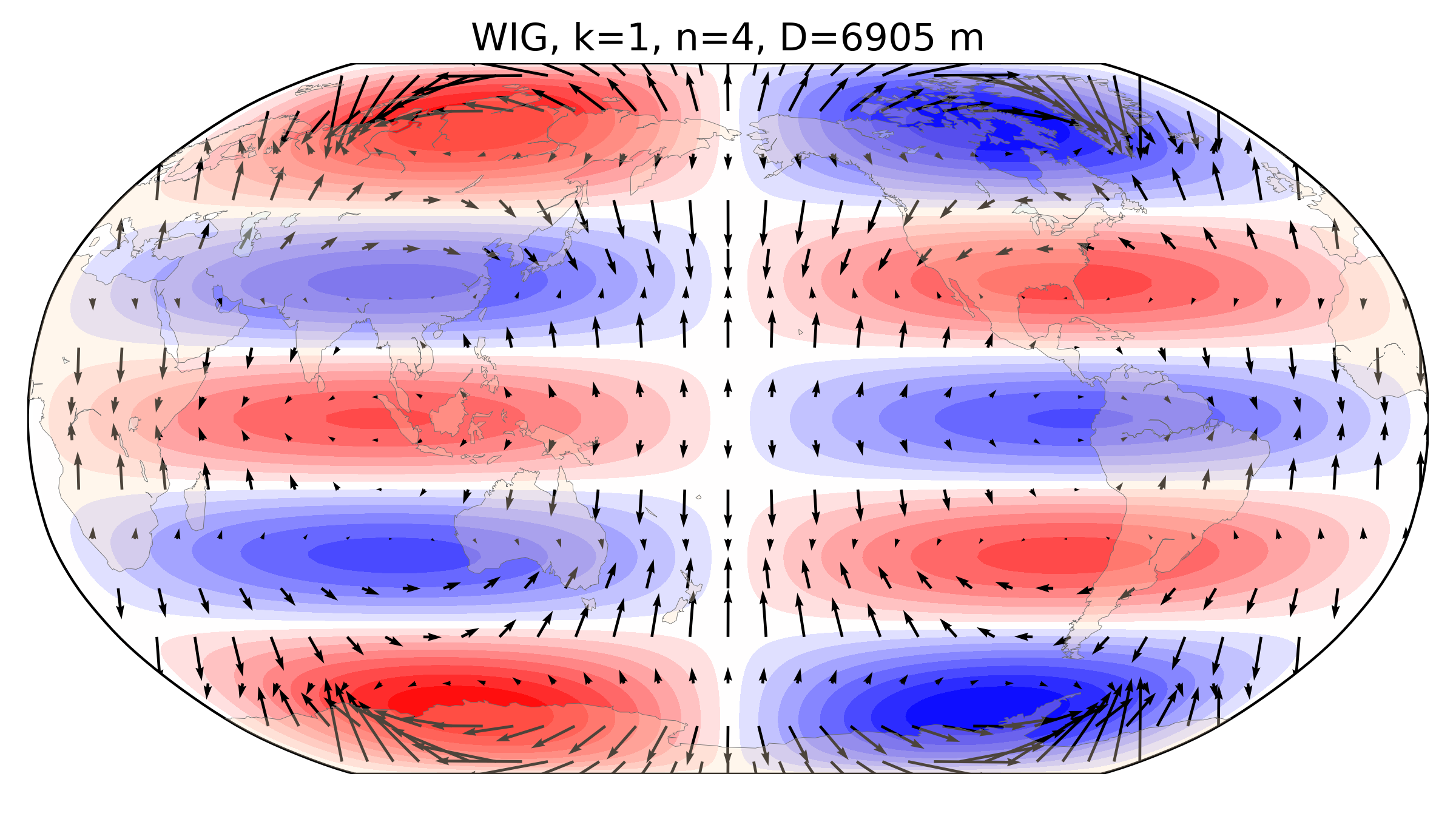
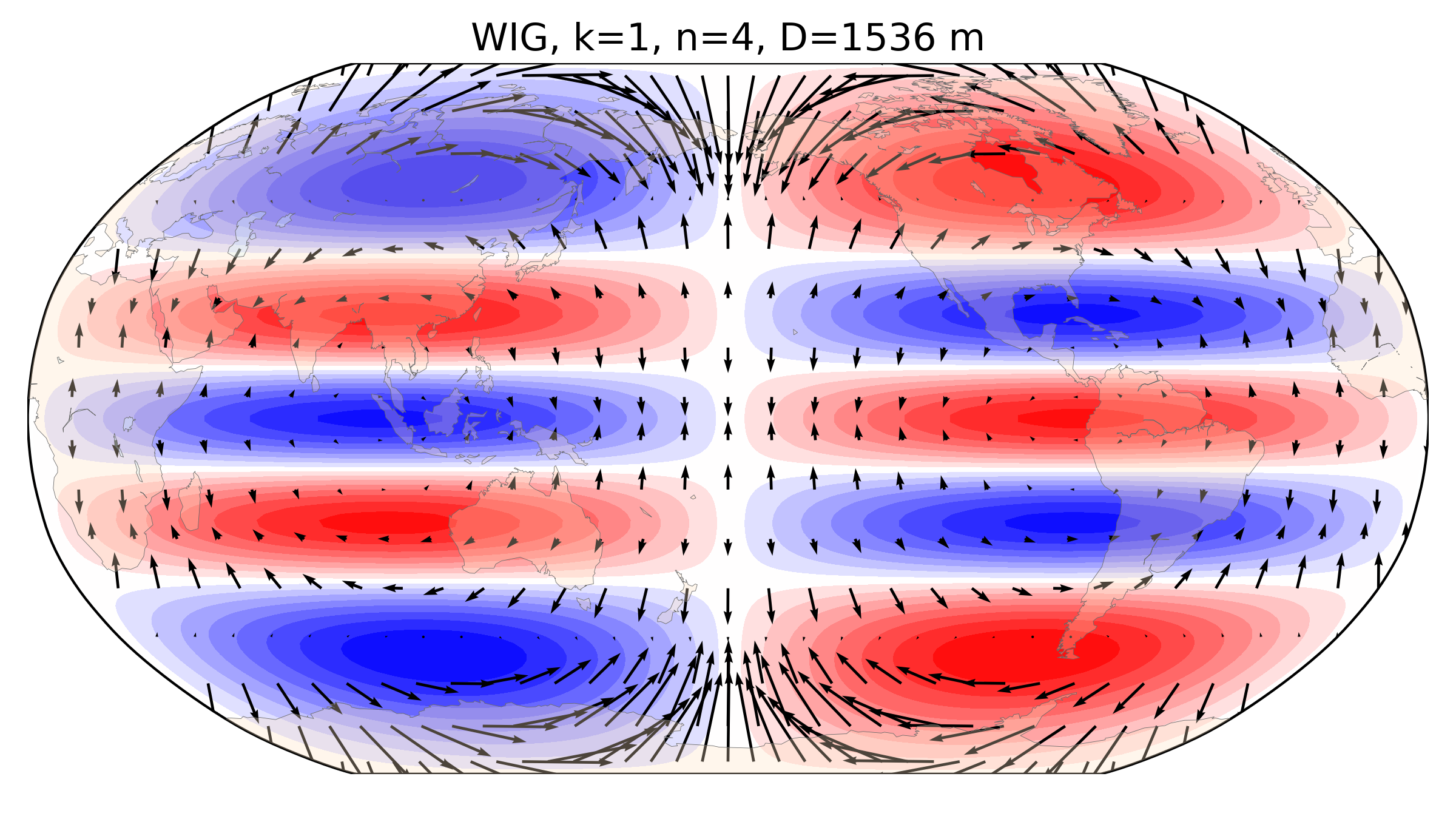
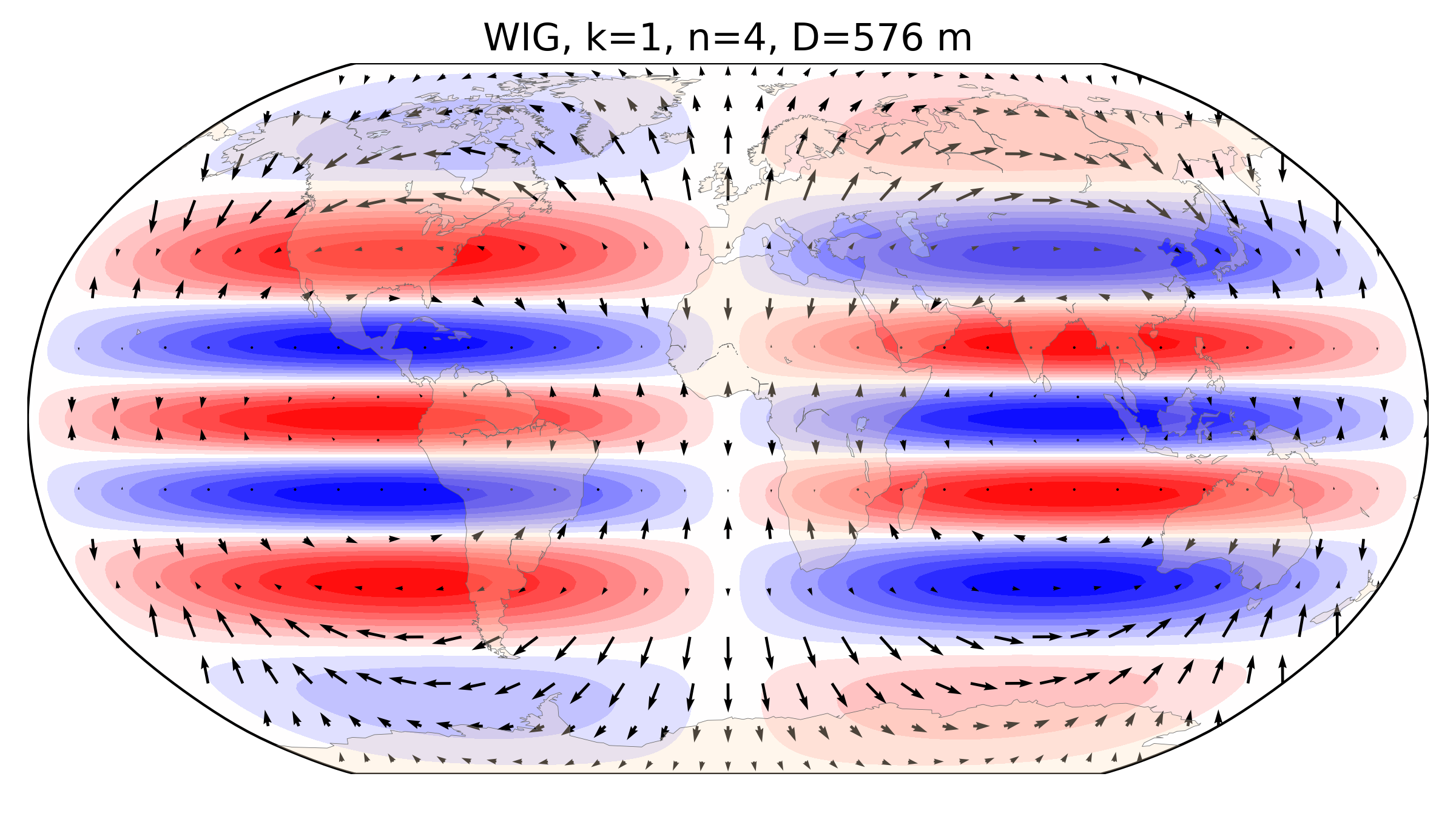
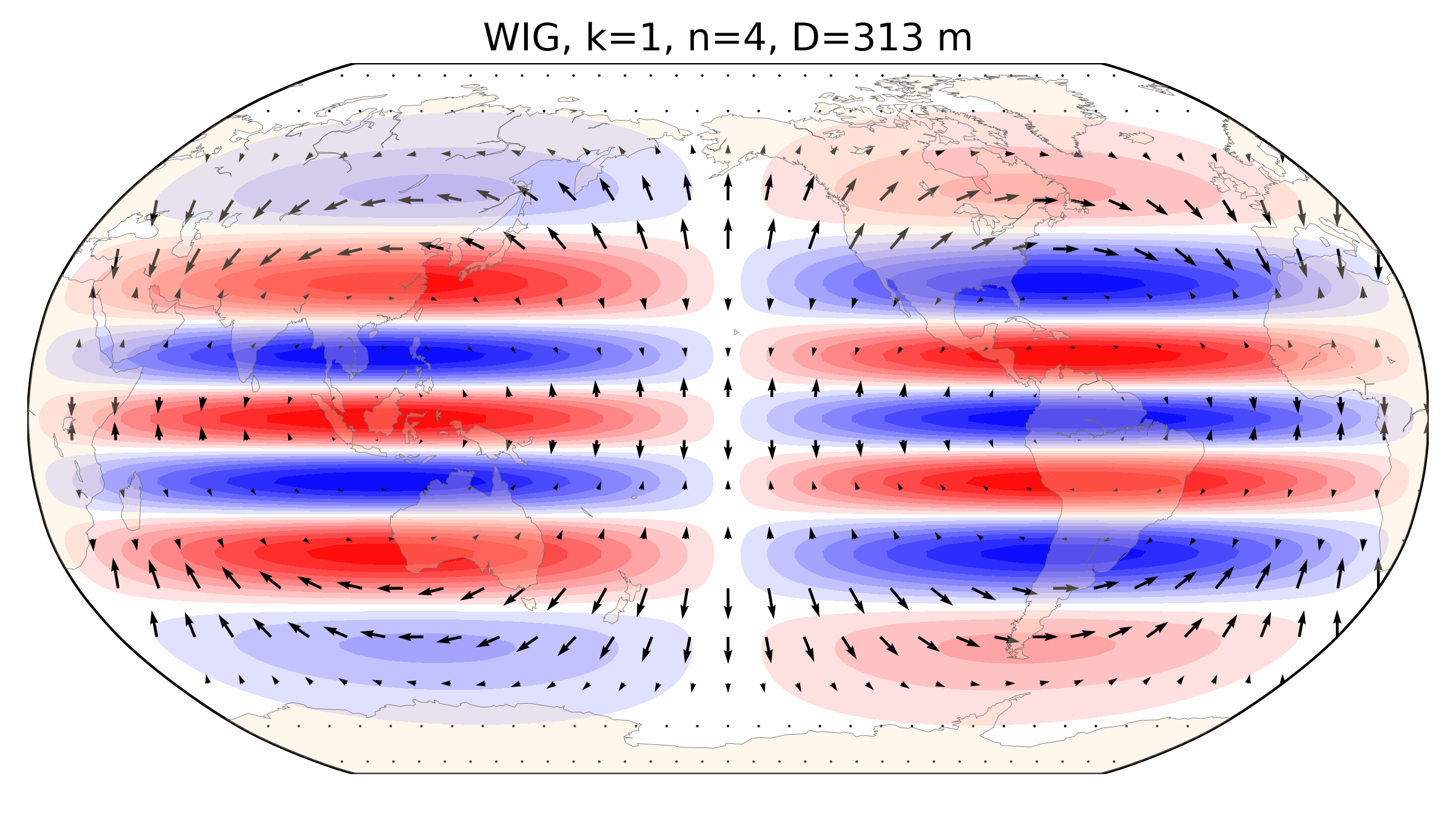
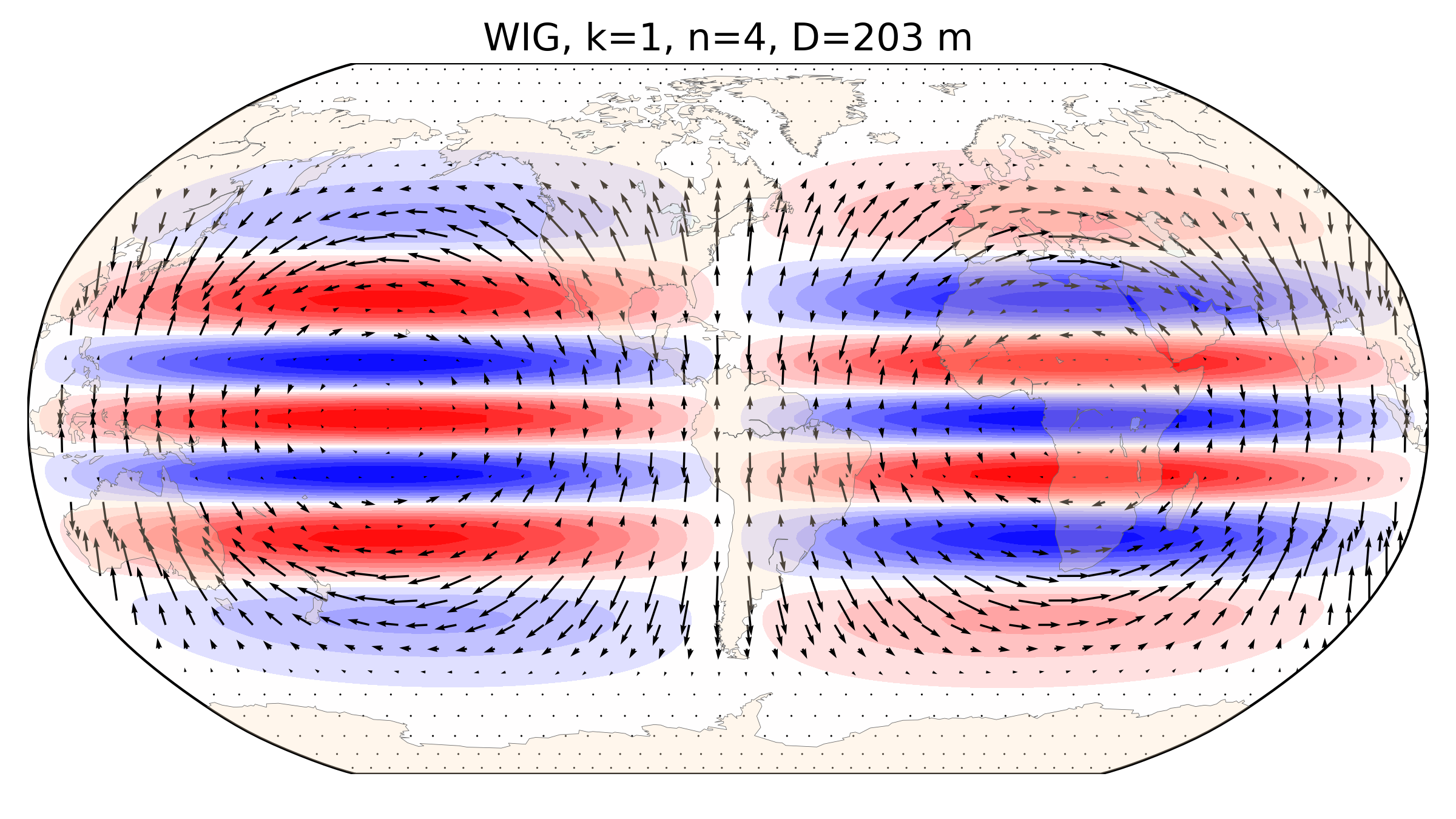
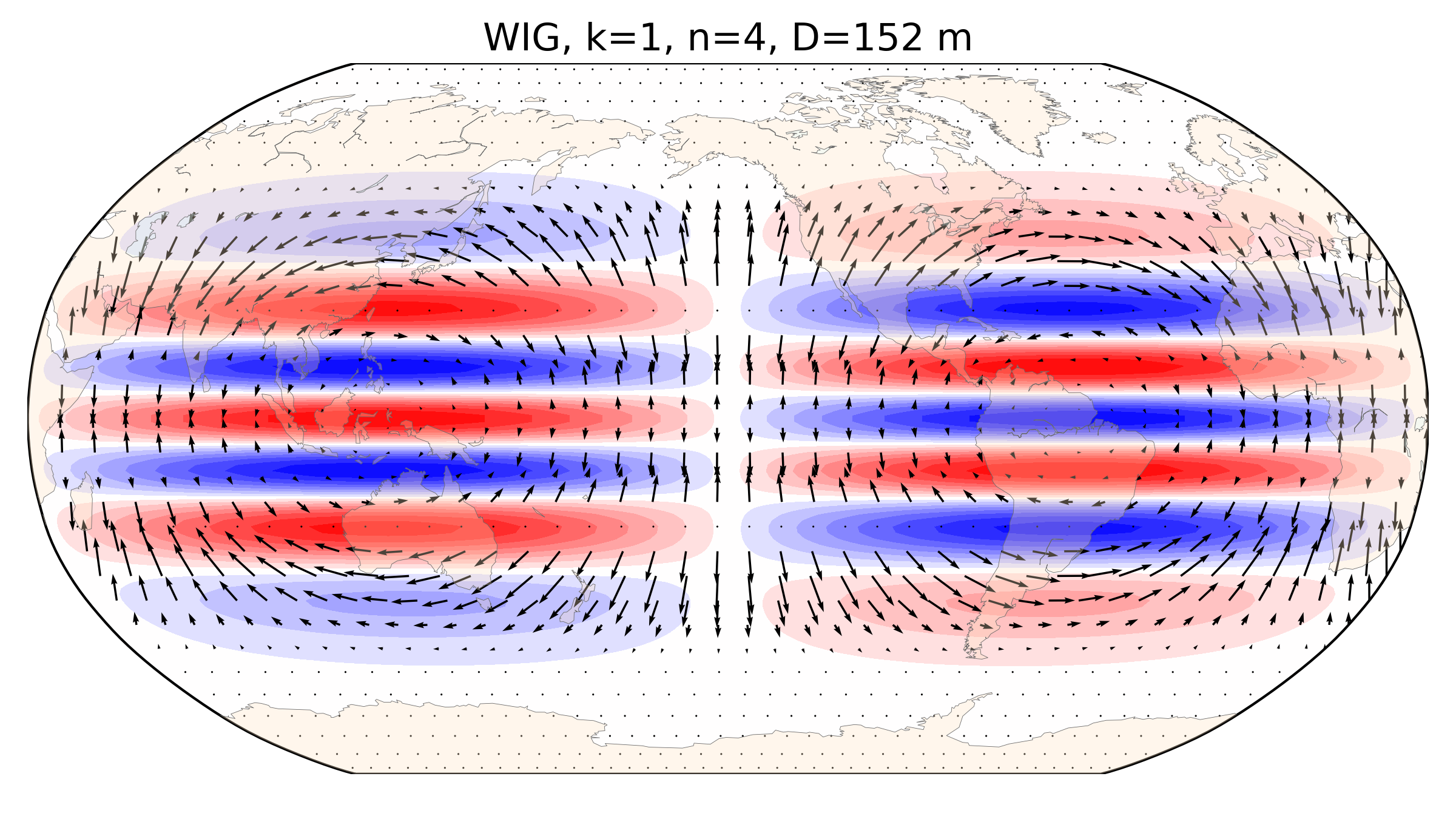
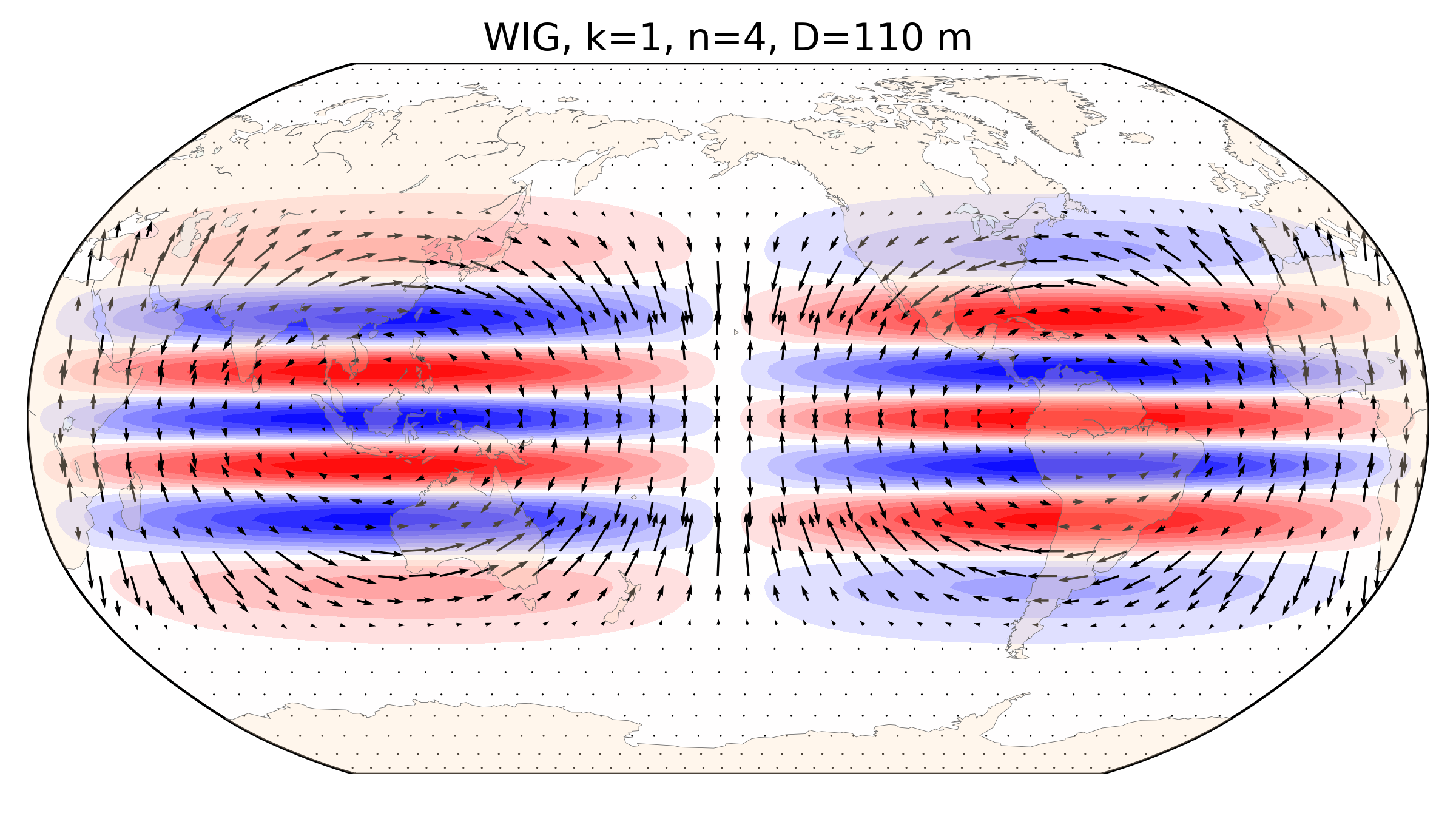
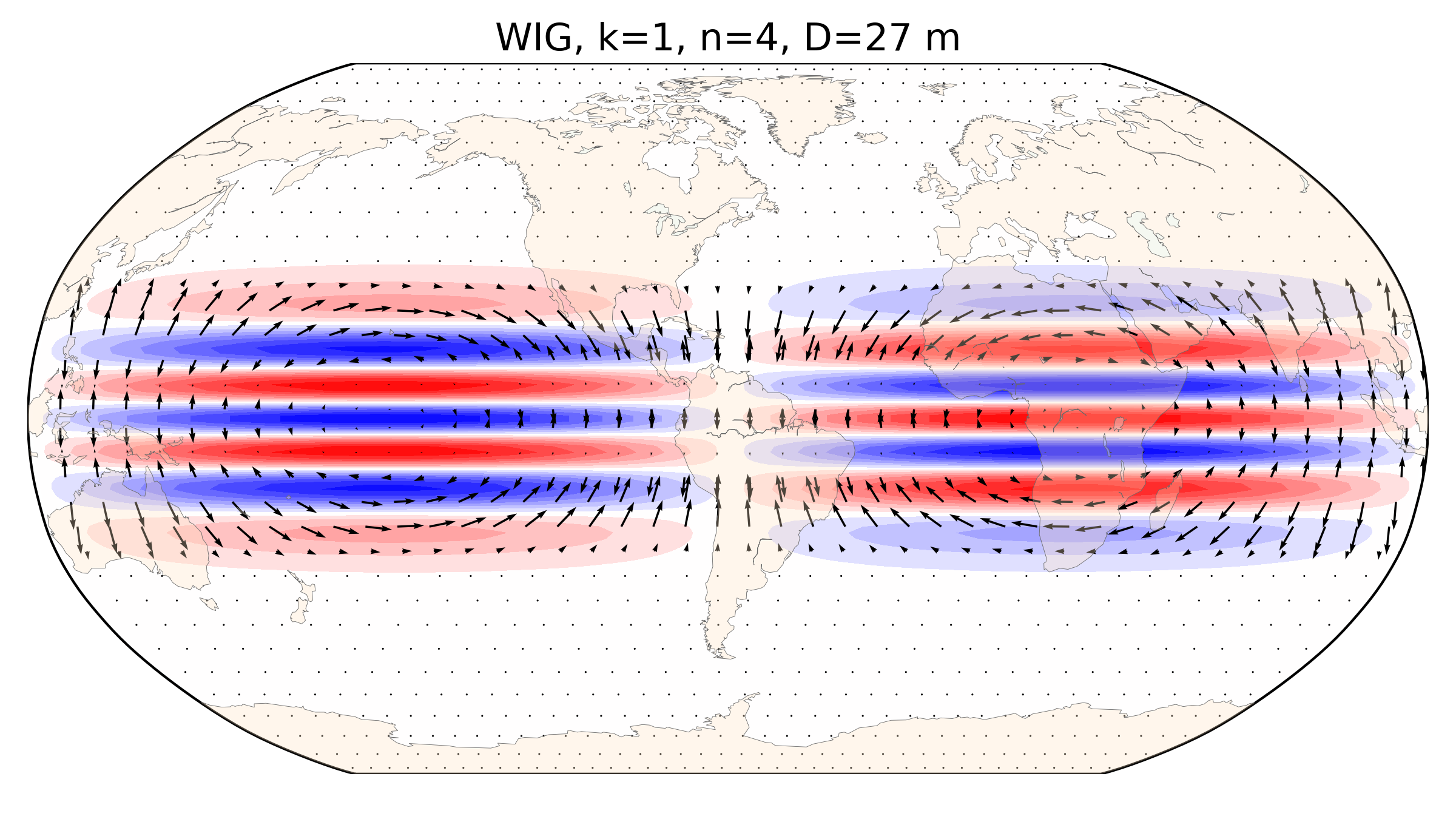
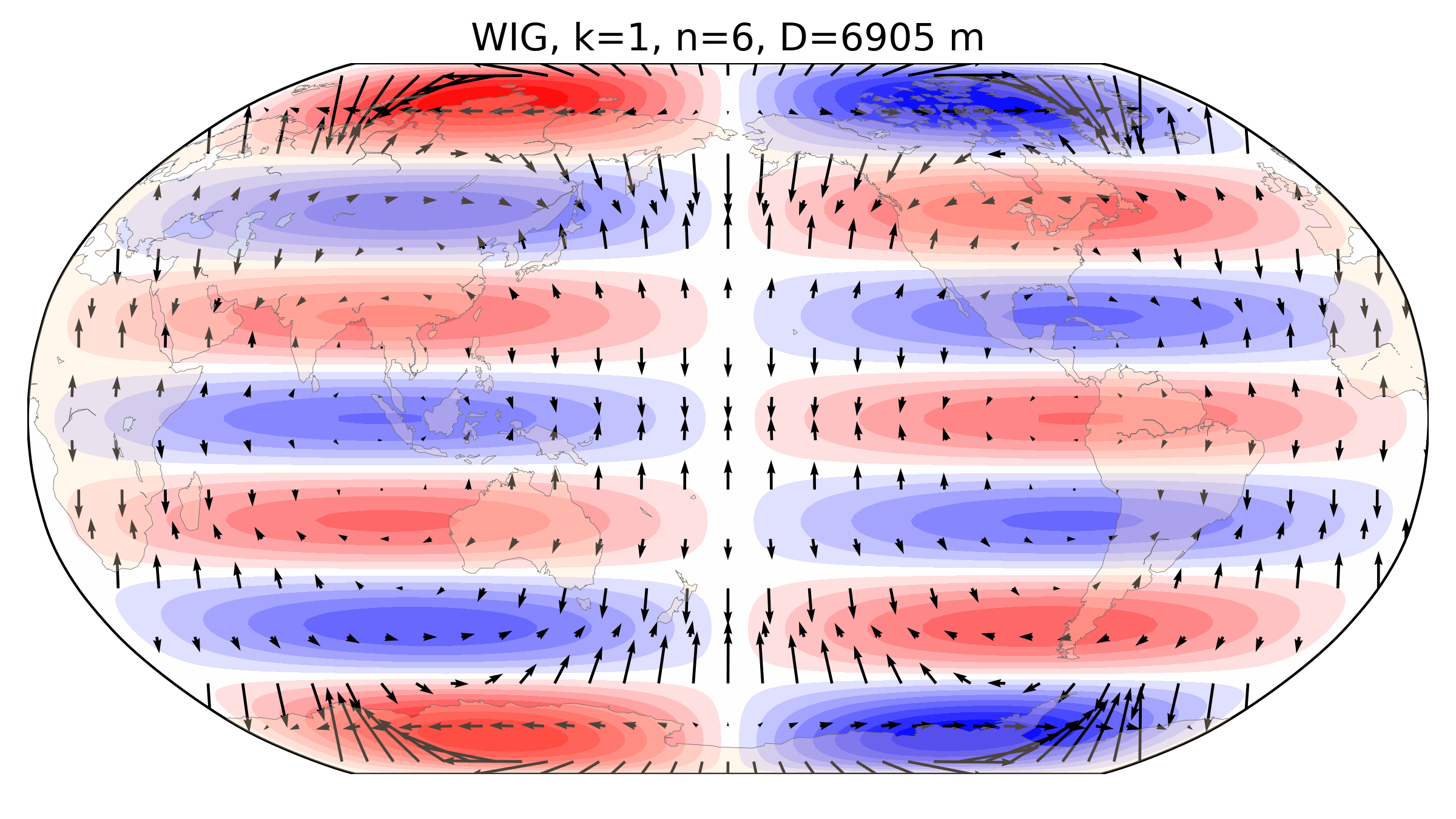
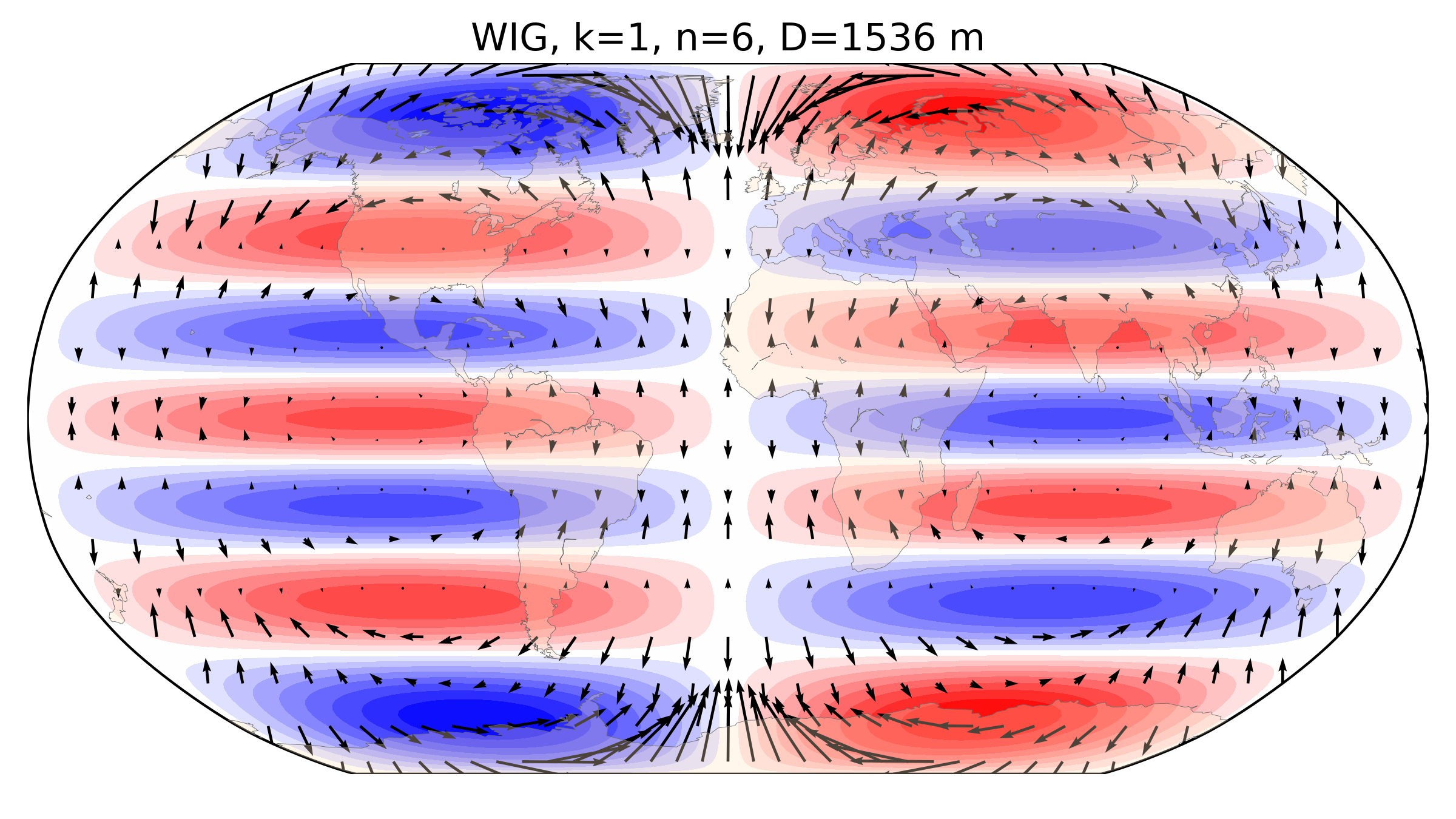
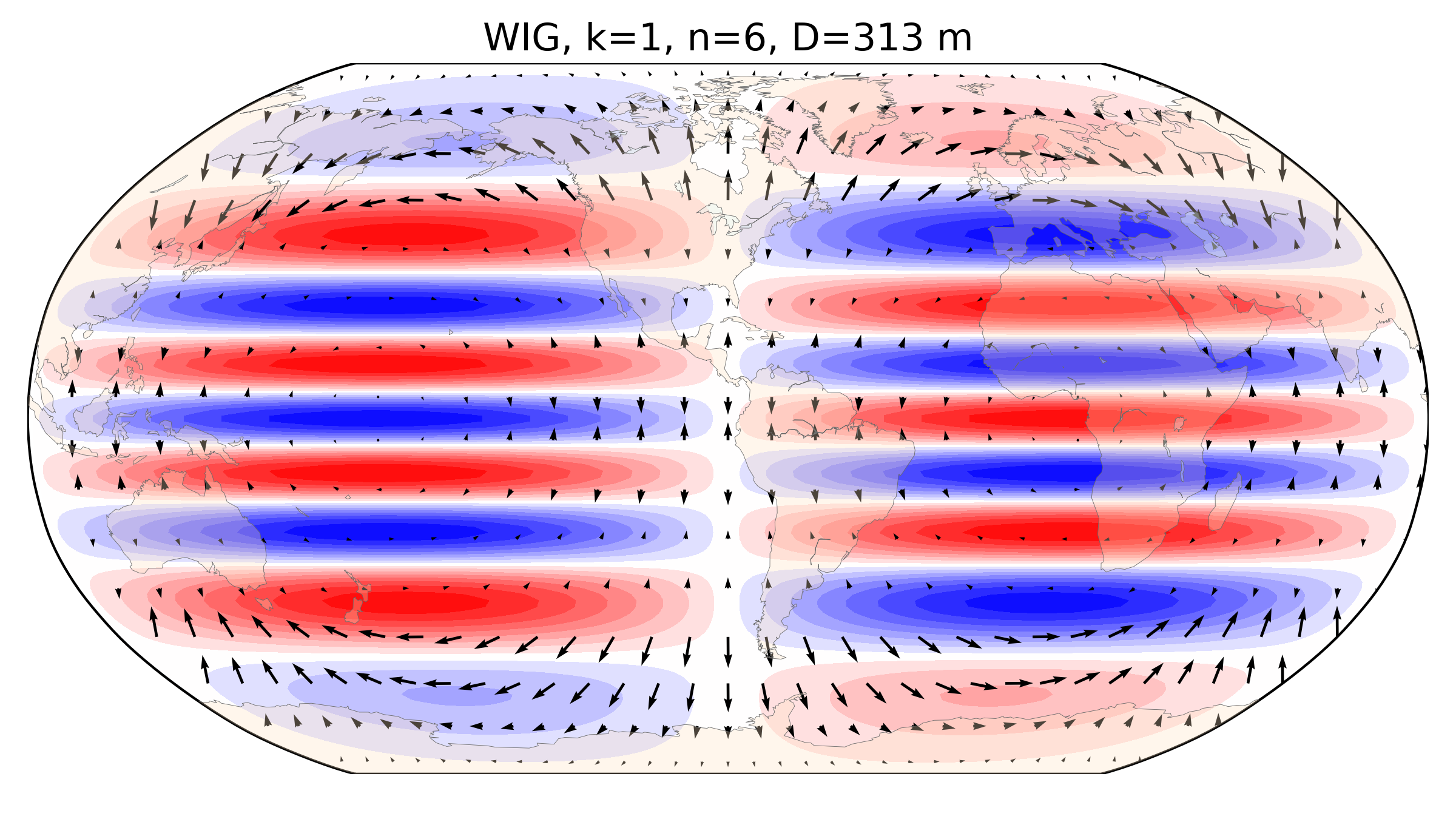
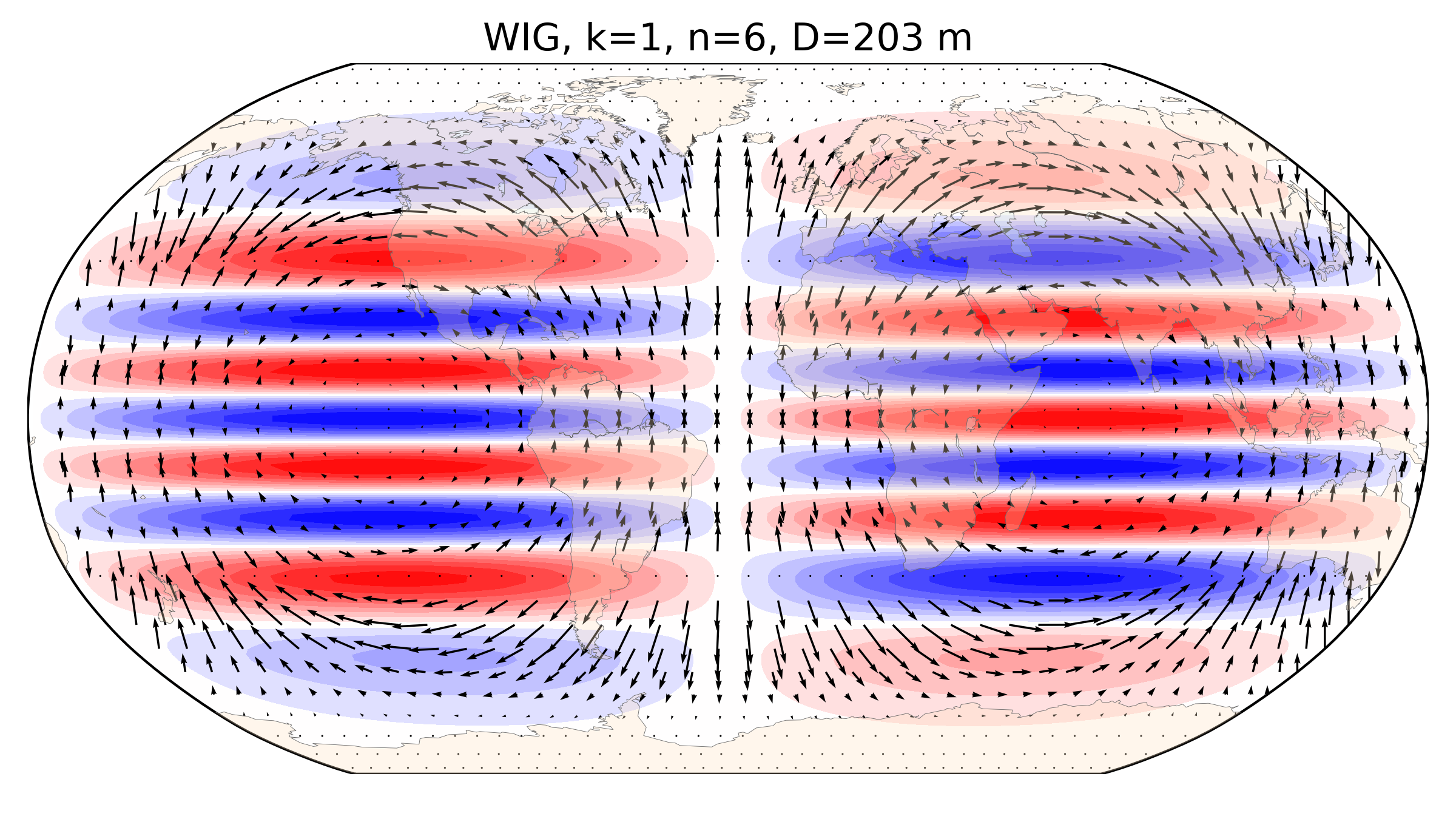
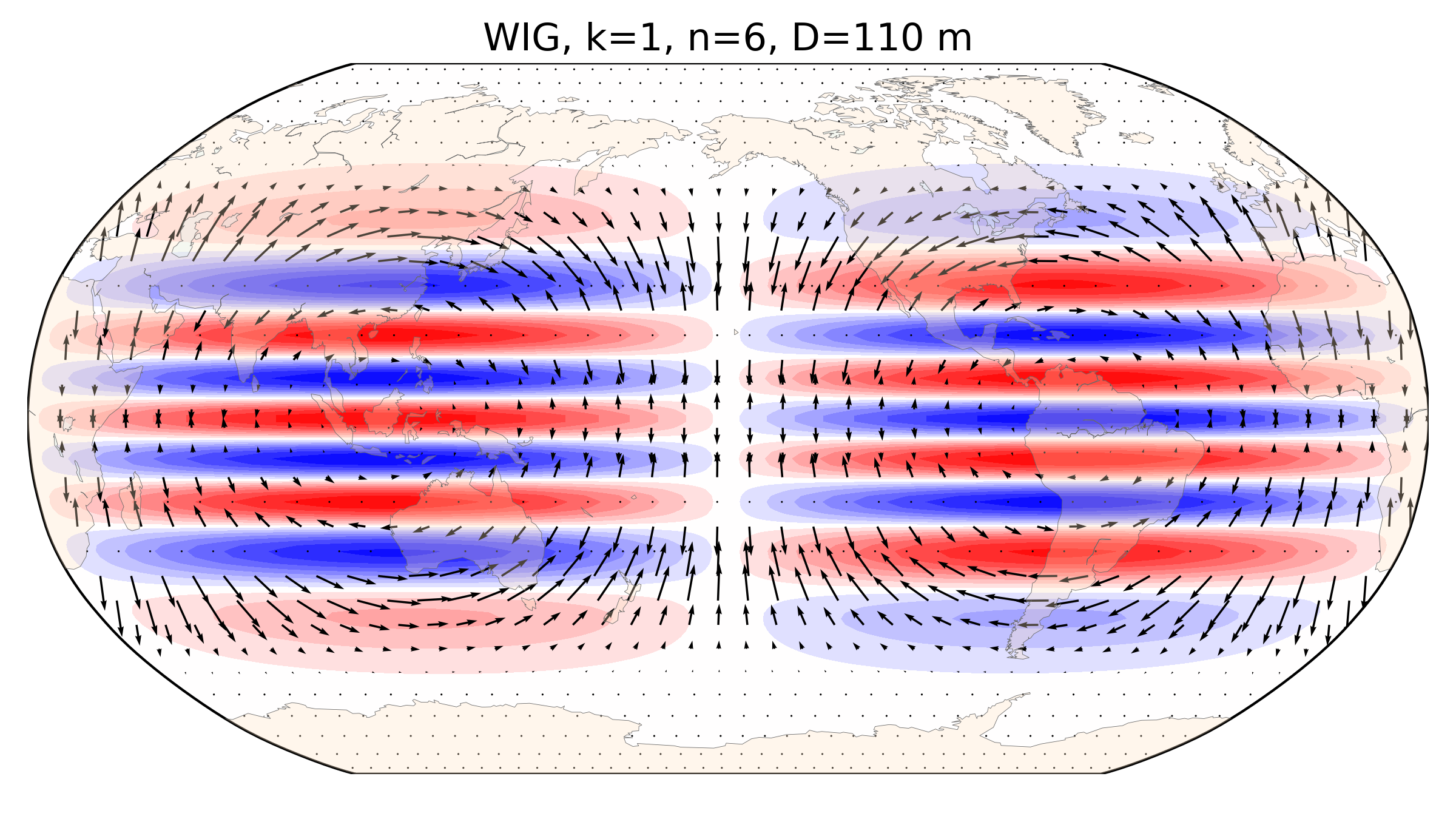
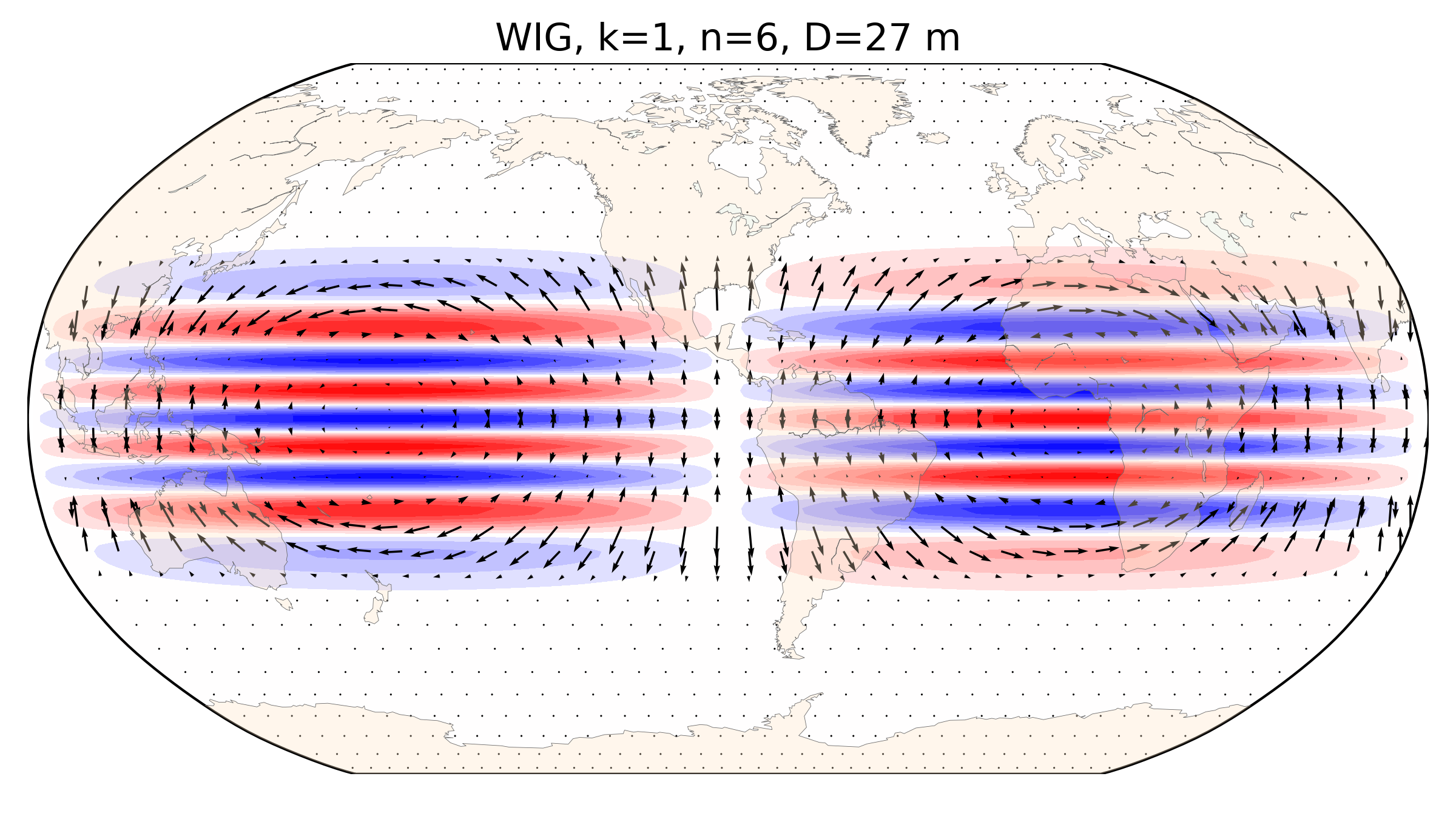
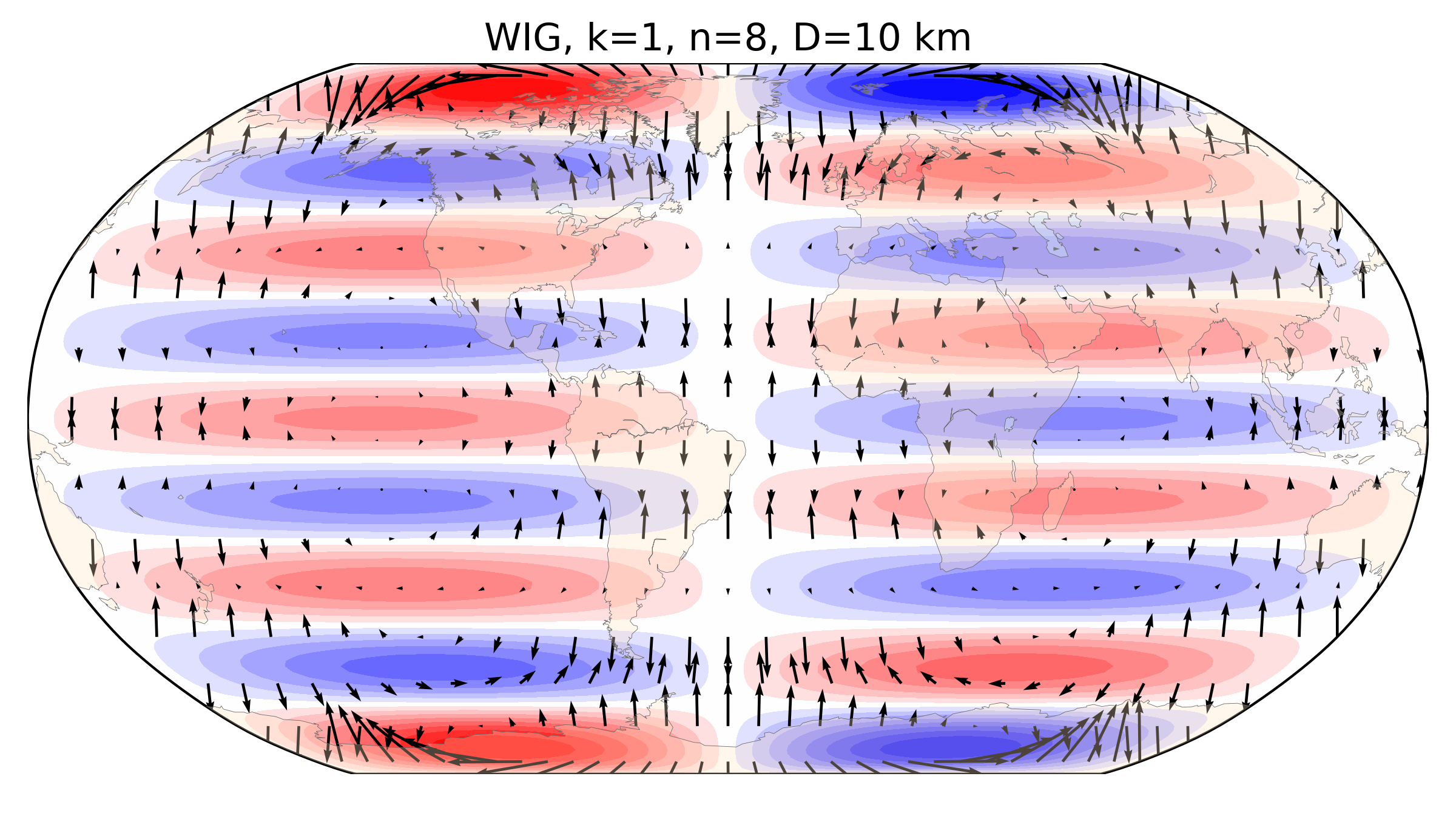
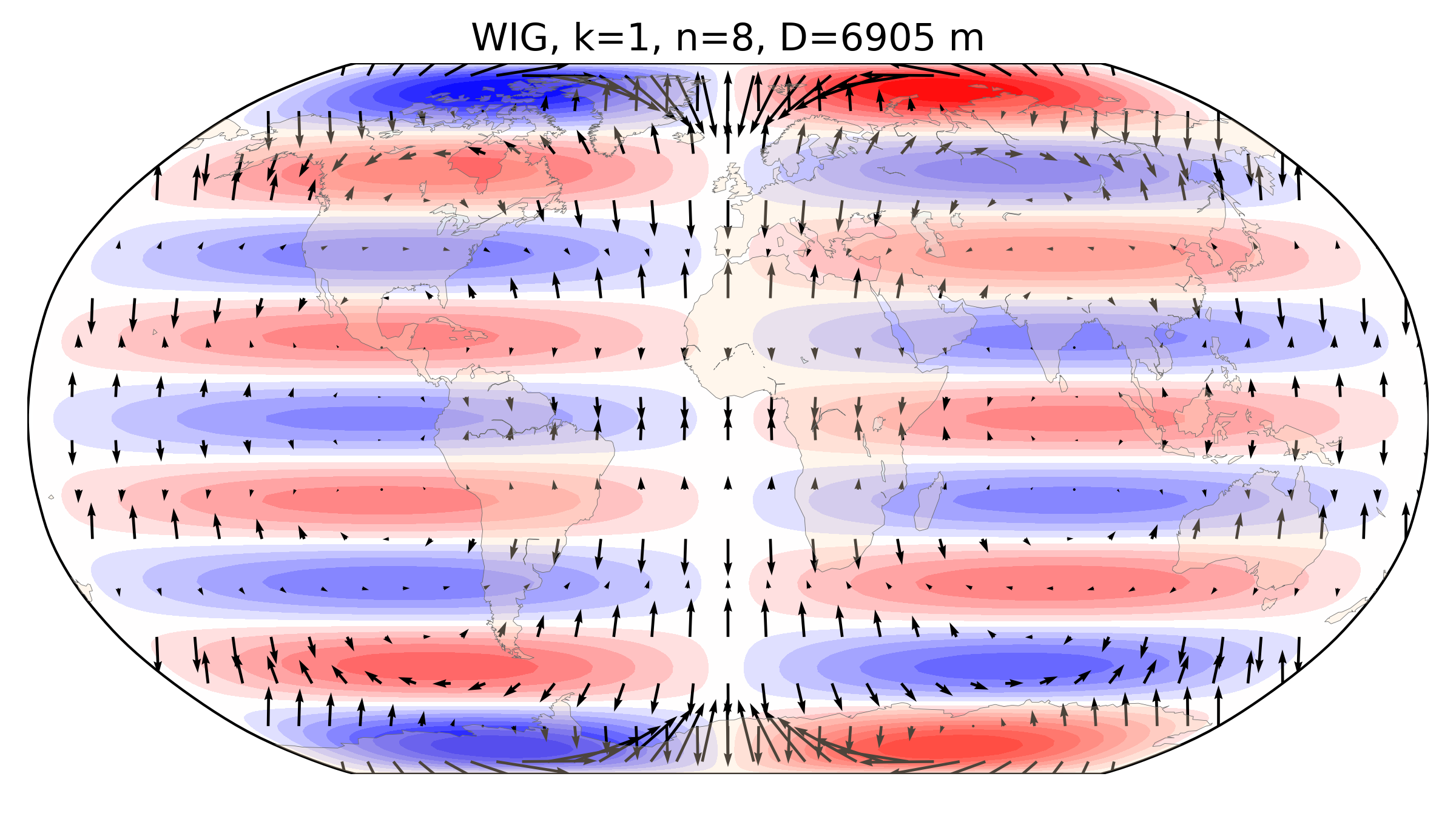
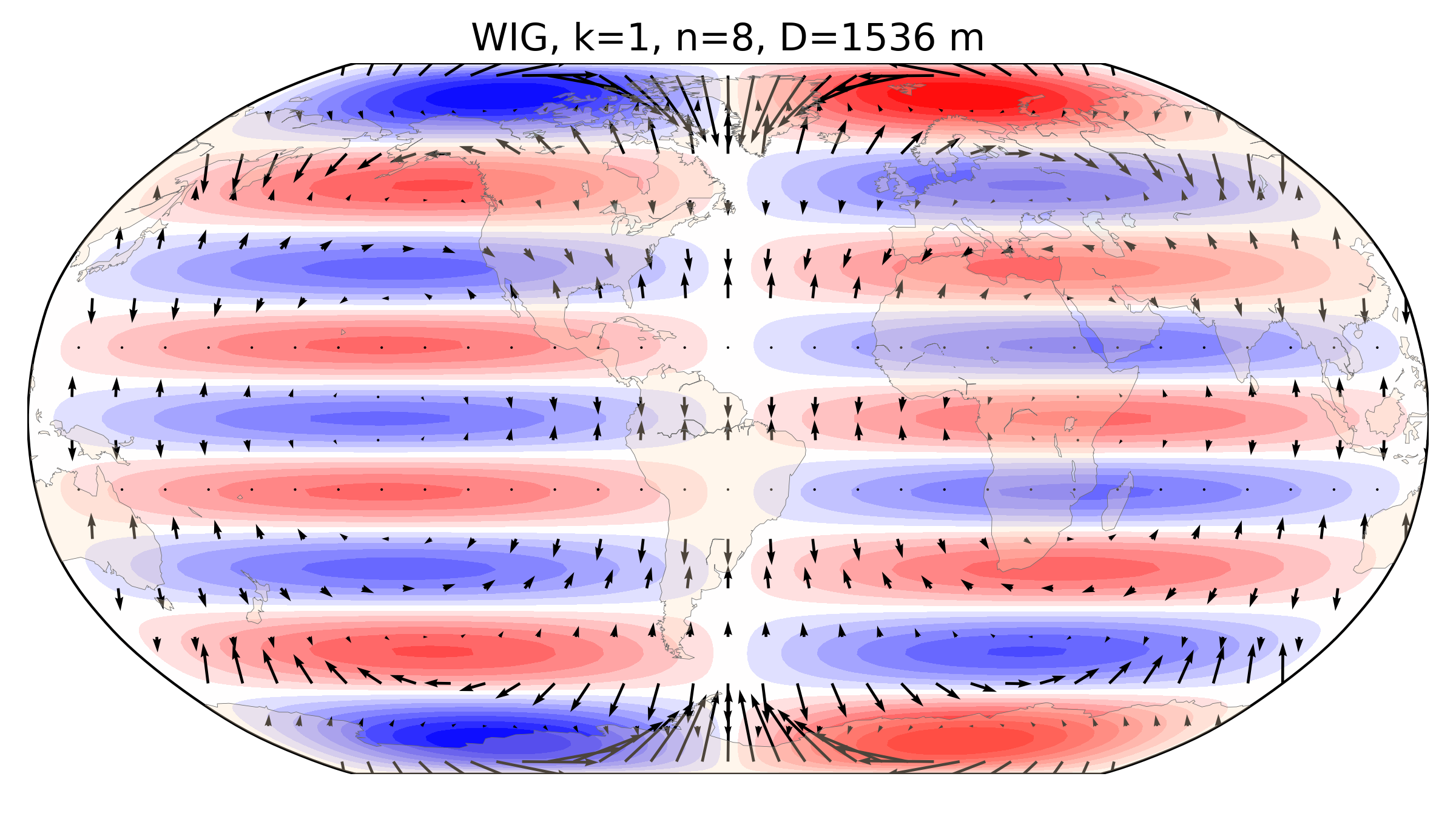
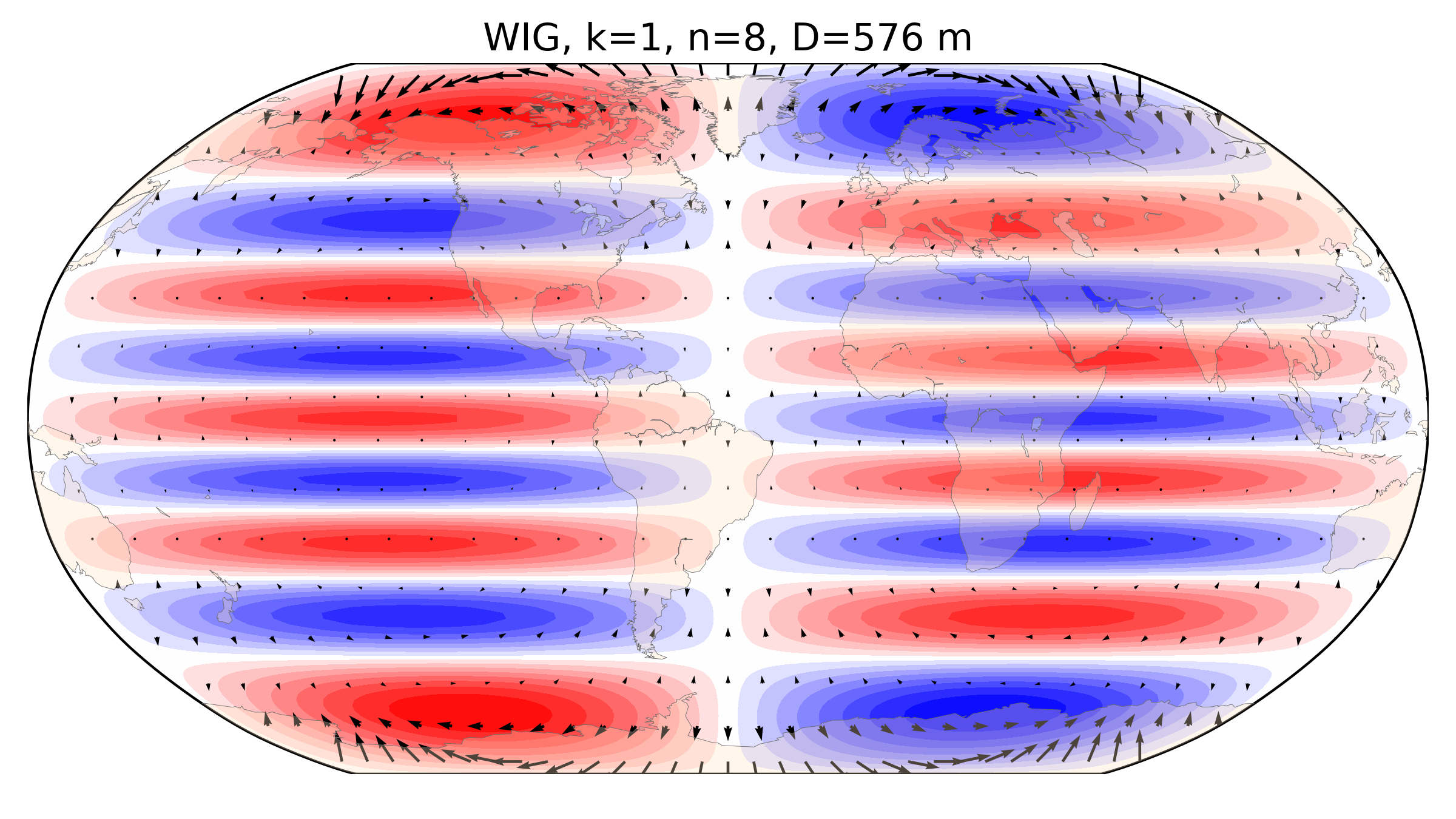
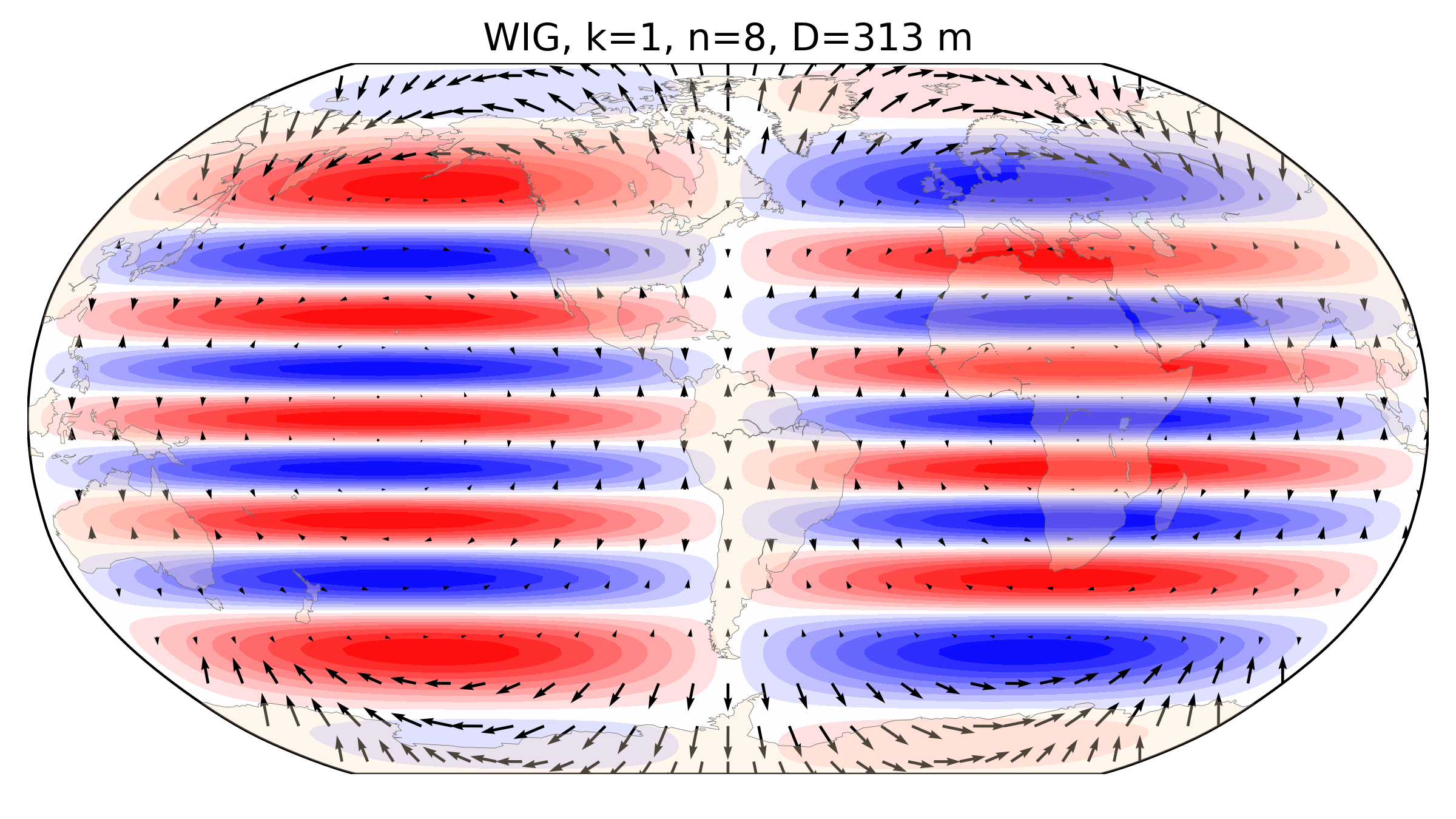
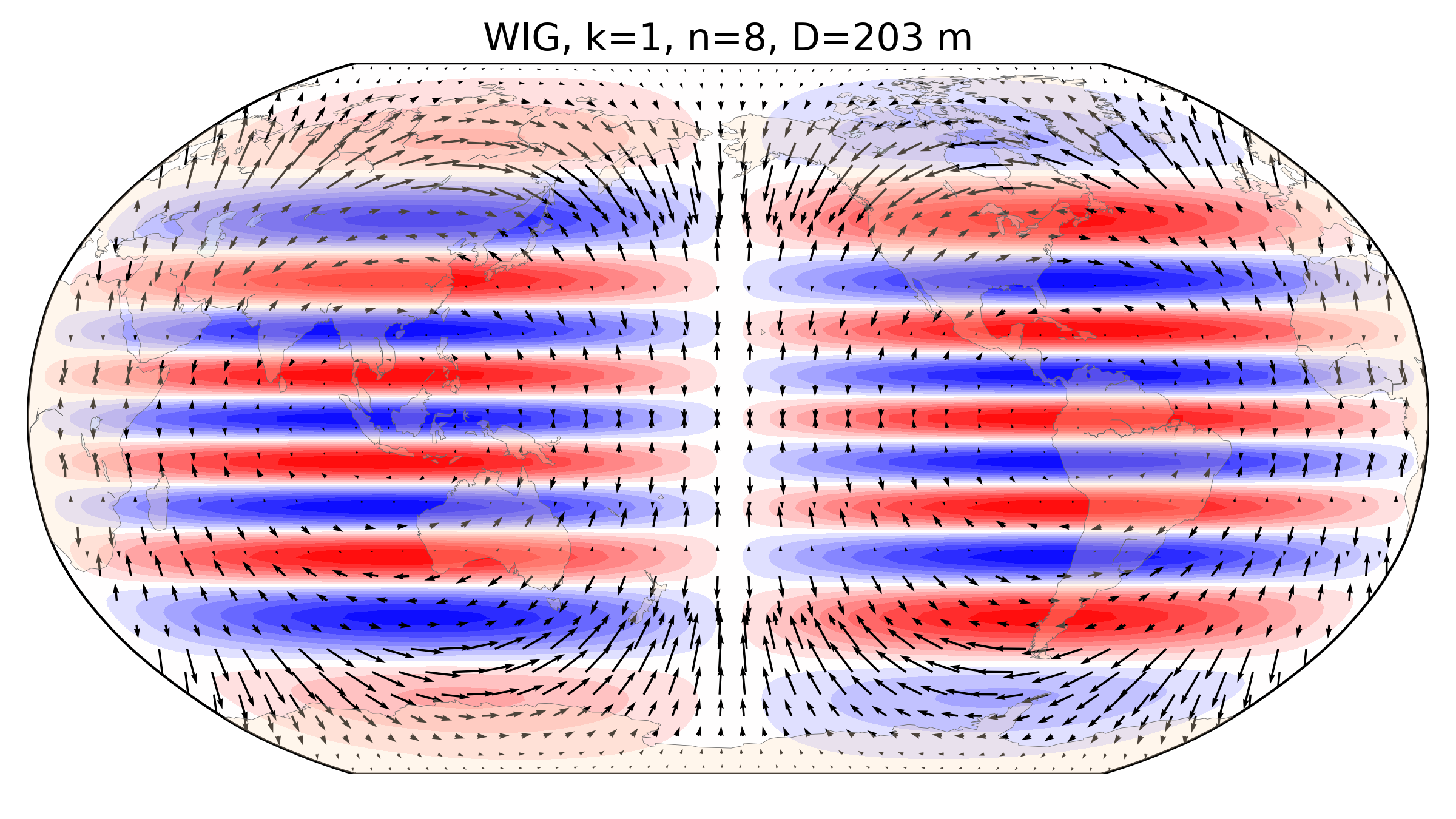
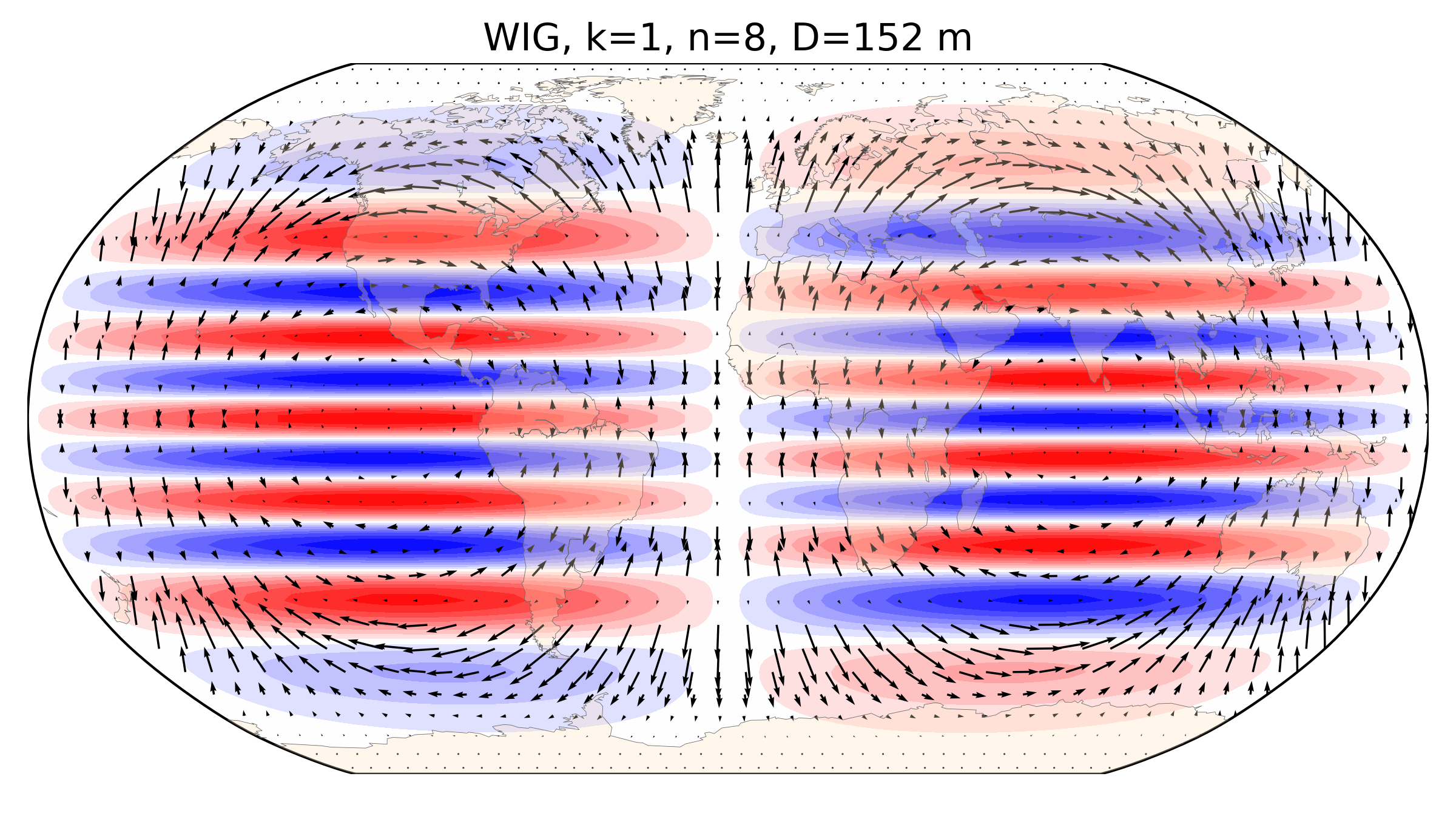
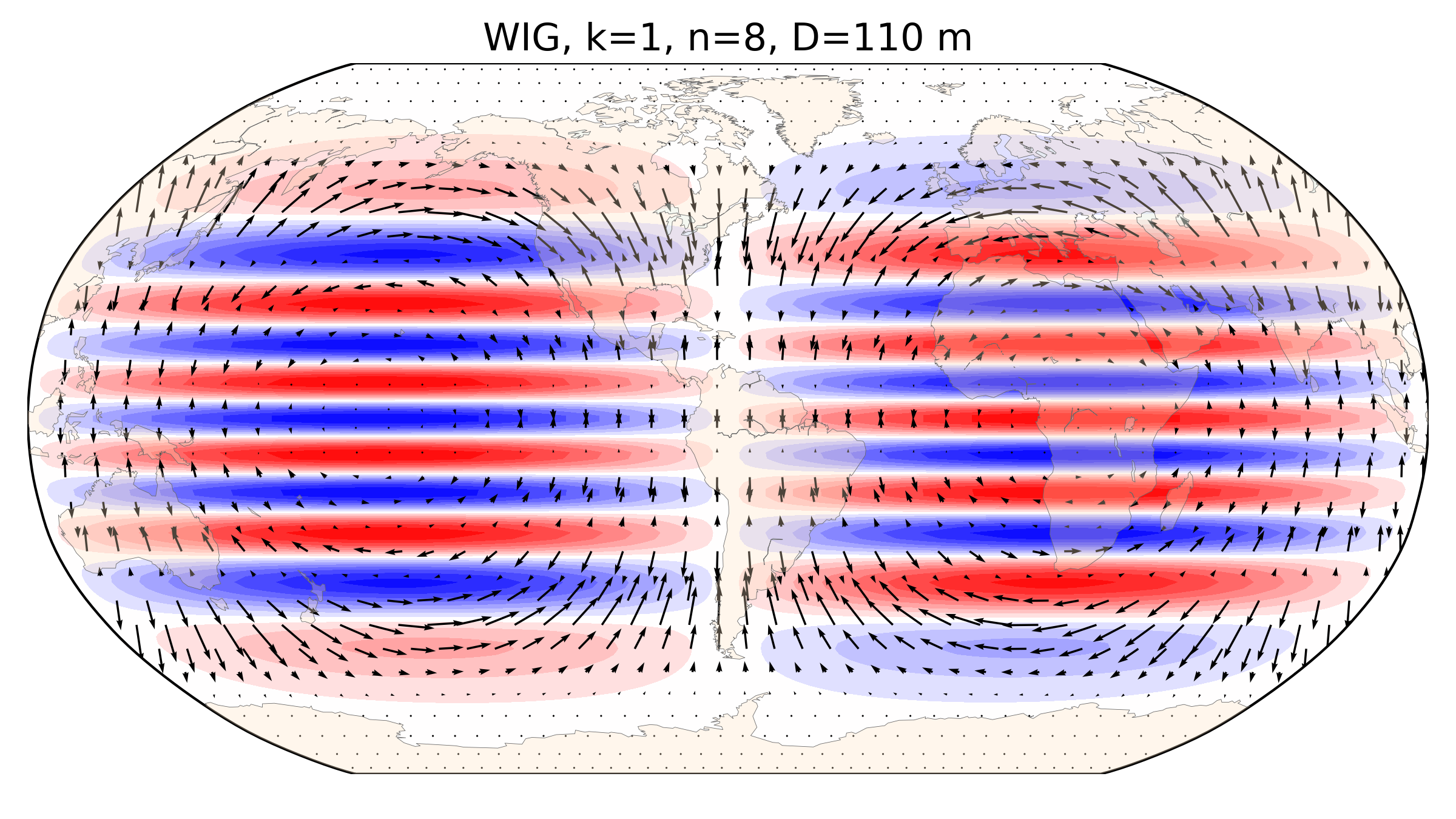
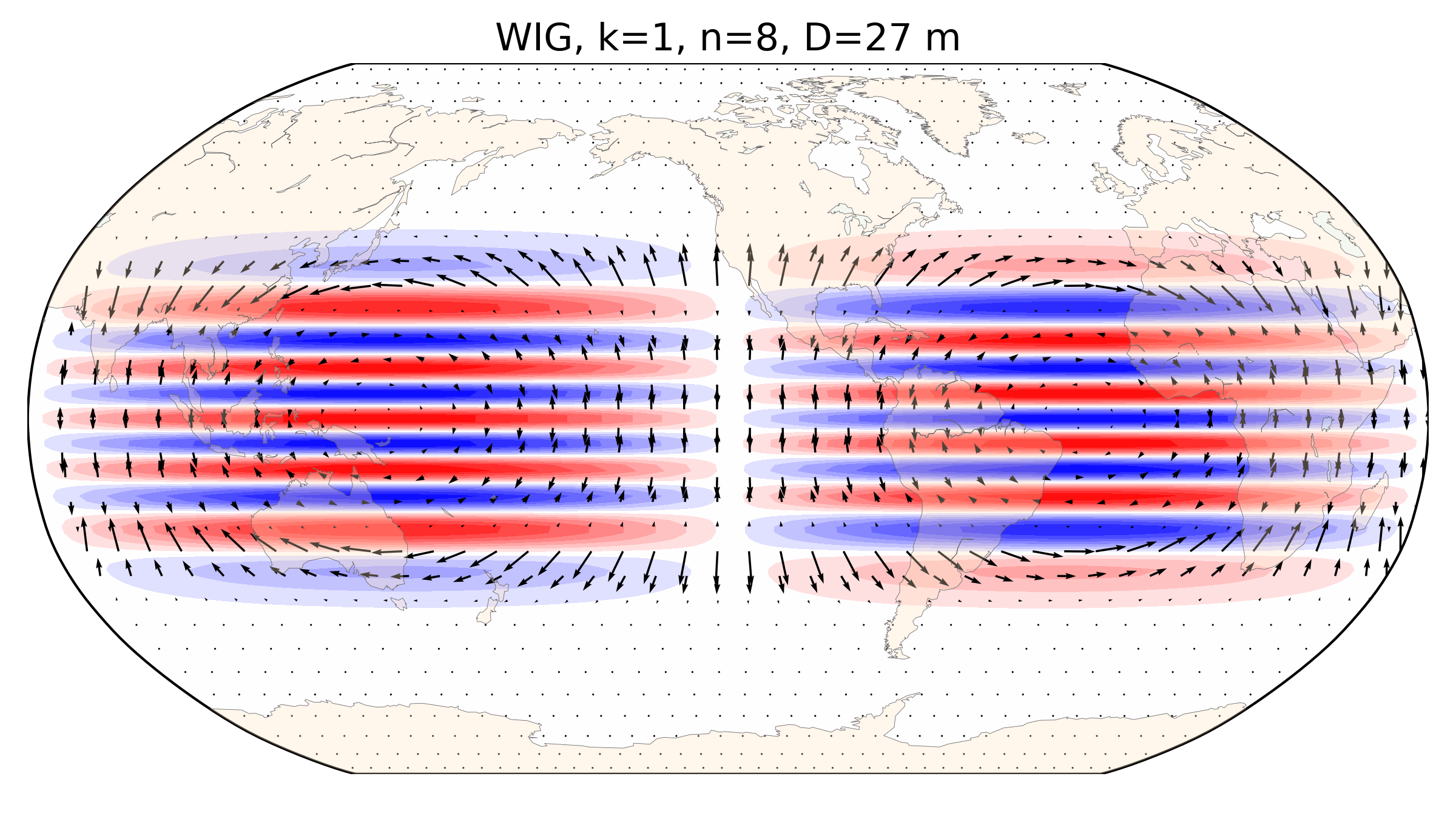
(e) Westward-propagating Inertia-gravity Waves
Horizontal structure of the slowest westward-propagating inertia-gravity (WIG) mode on the sphere, n=0 WIG wave. Geopotential height is scaled by the maximal height. Colours vary between -1 and +1 with positive/negative perturbations in red/blue. Wind components are scaled by the maximal wind speed.
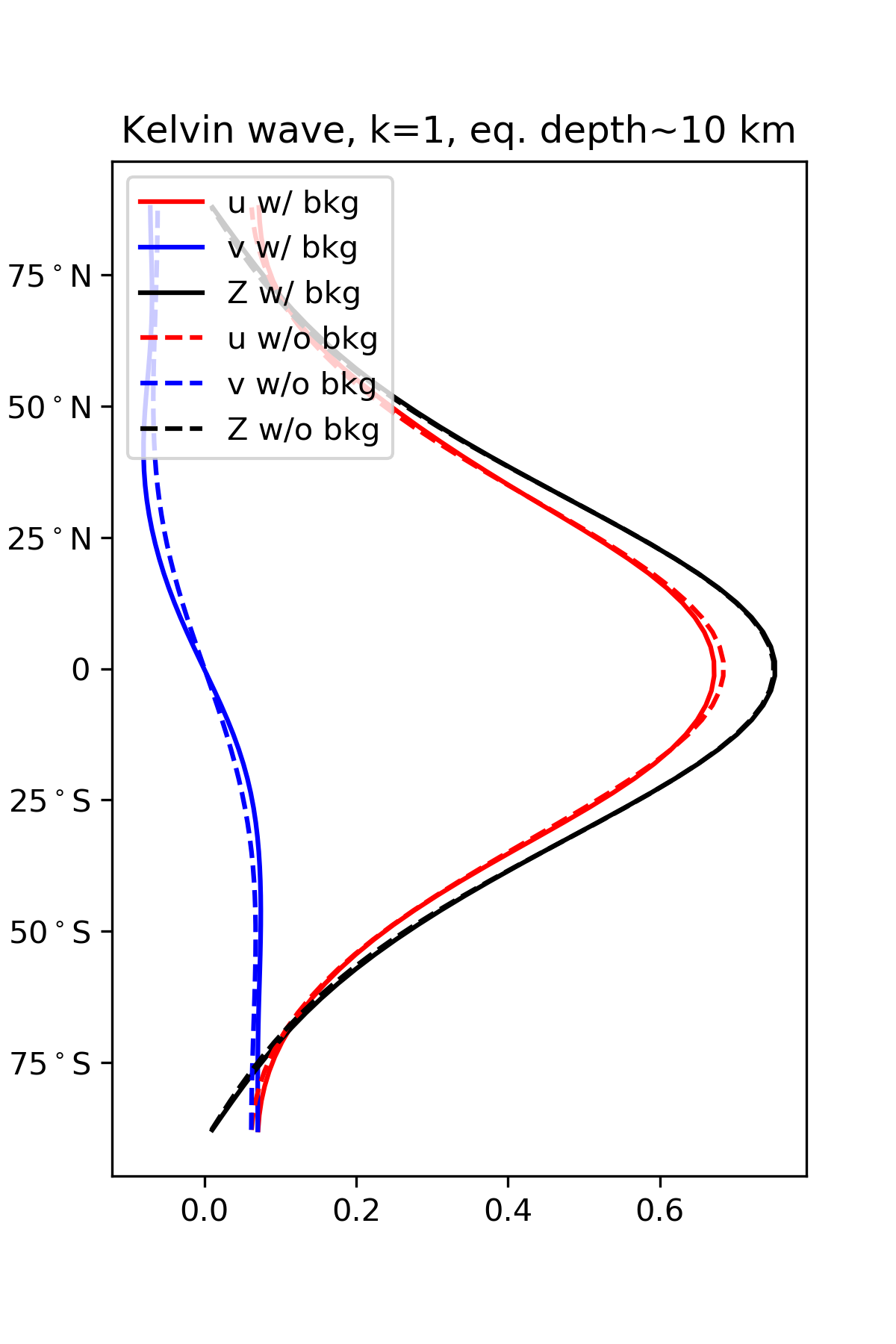
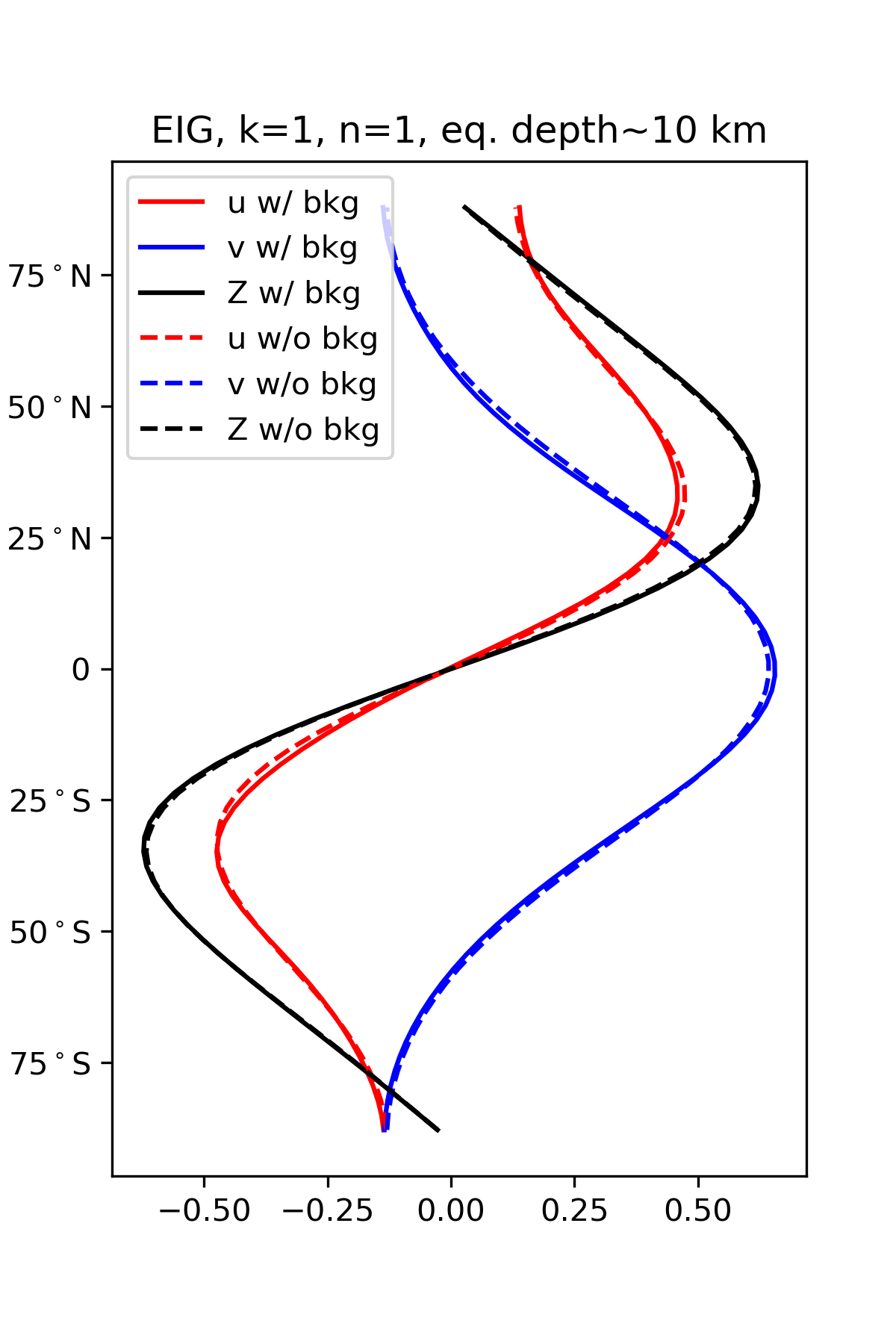
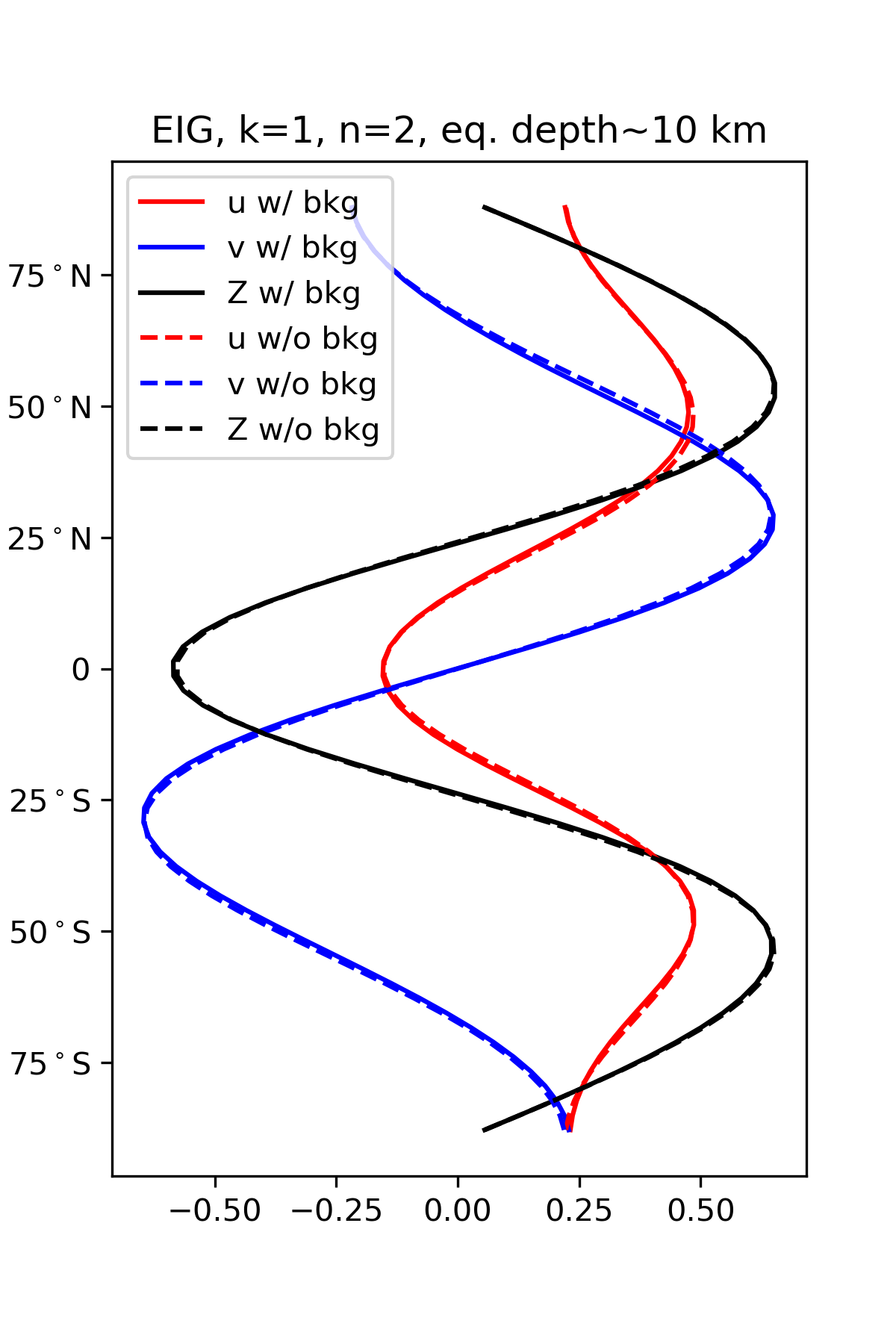
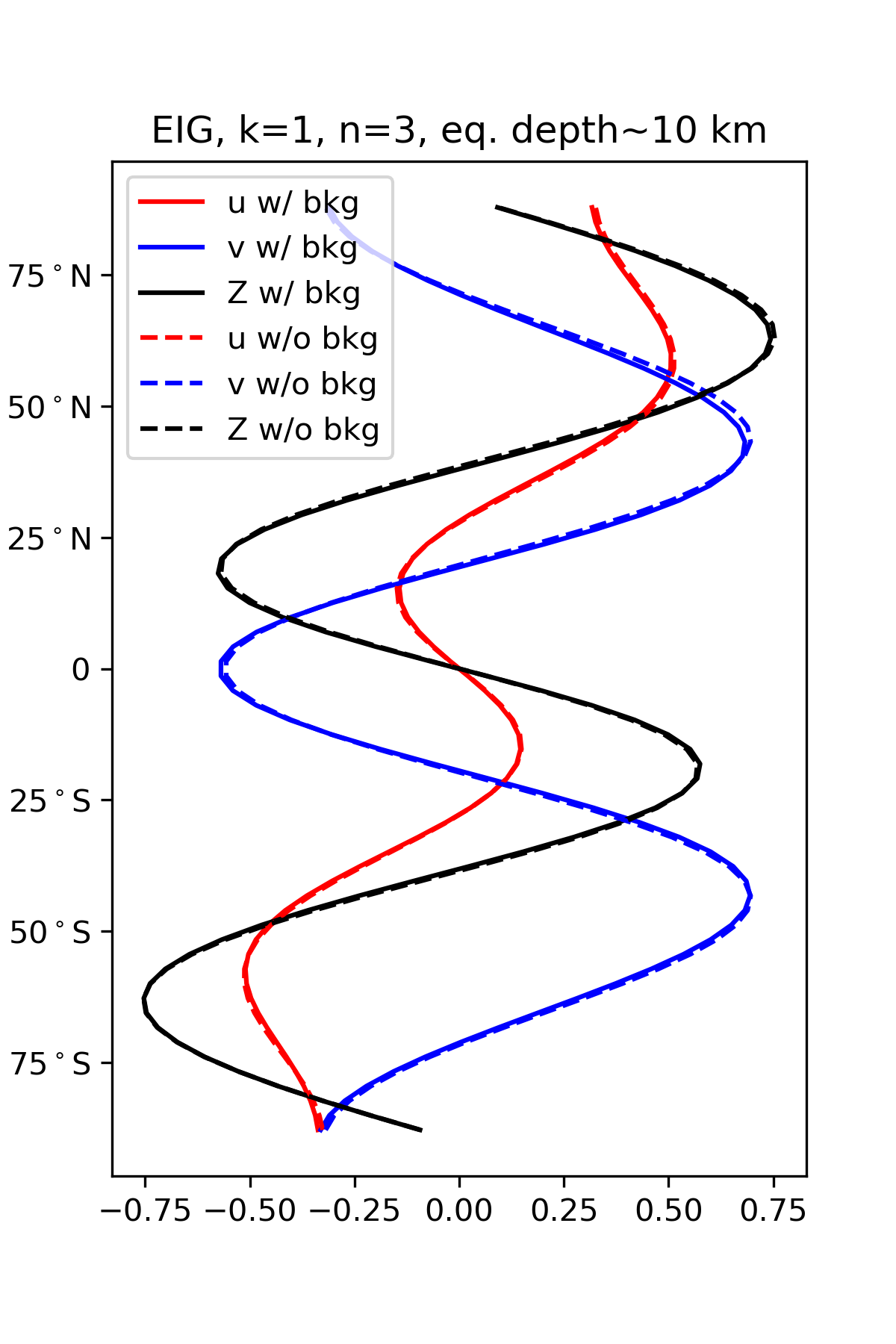
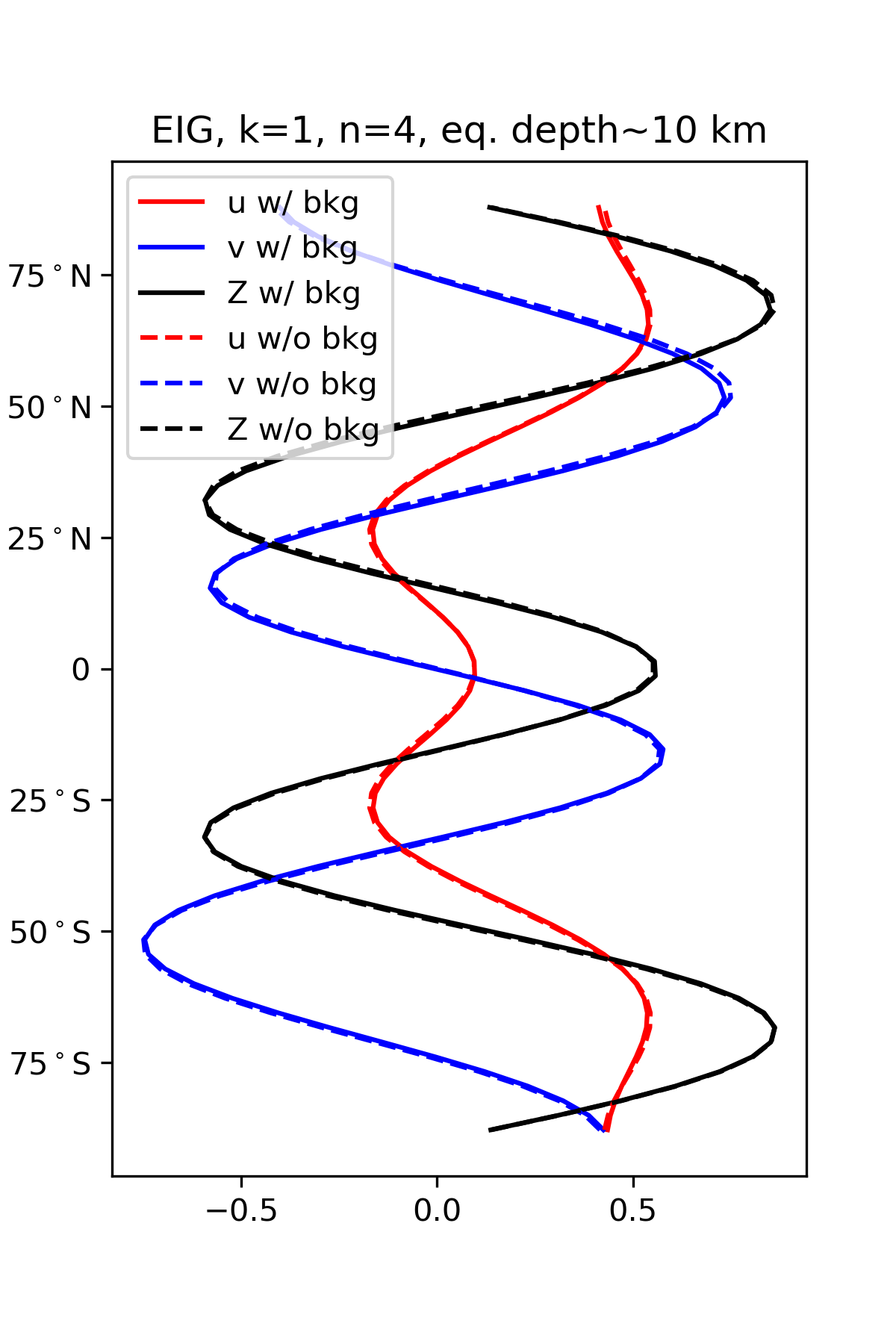
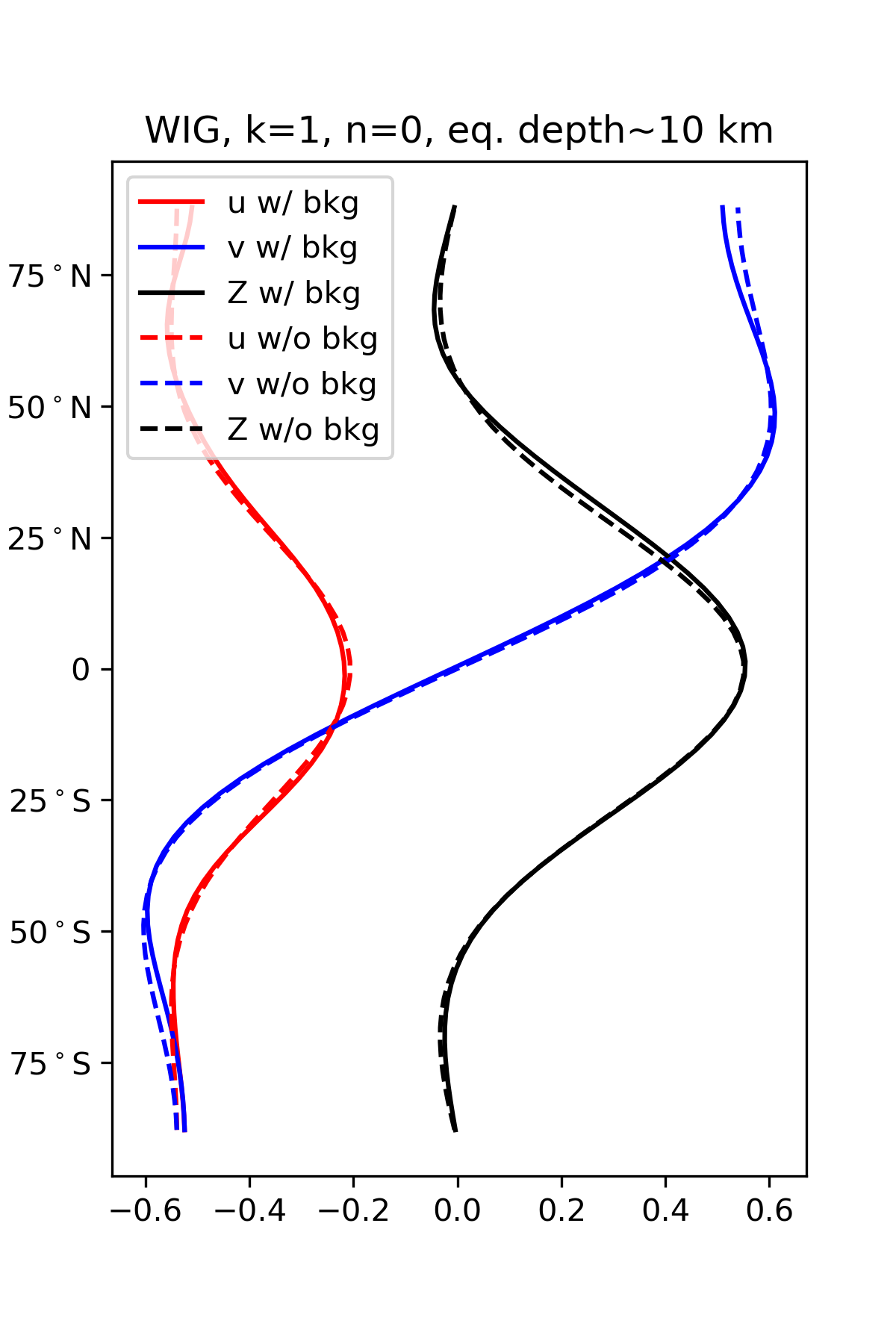
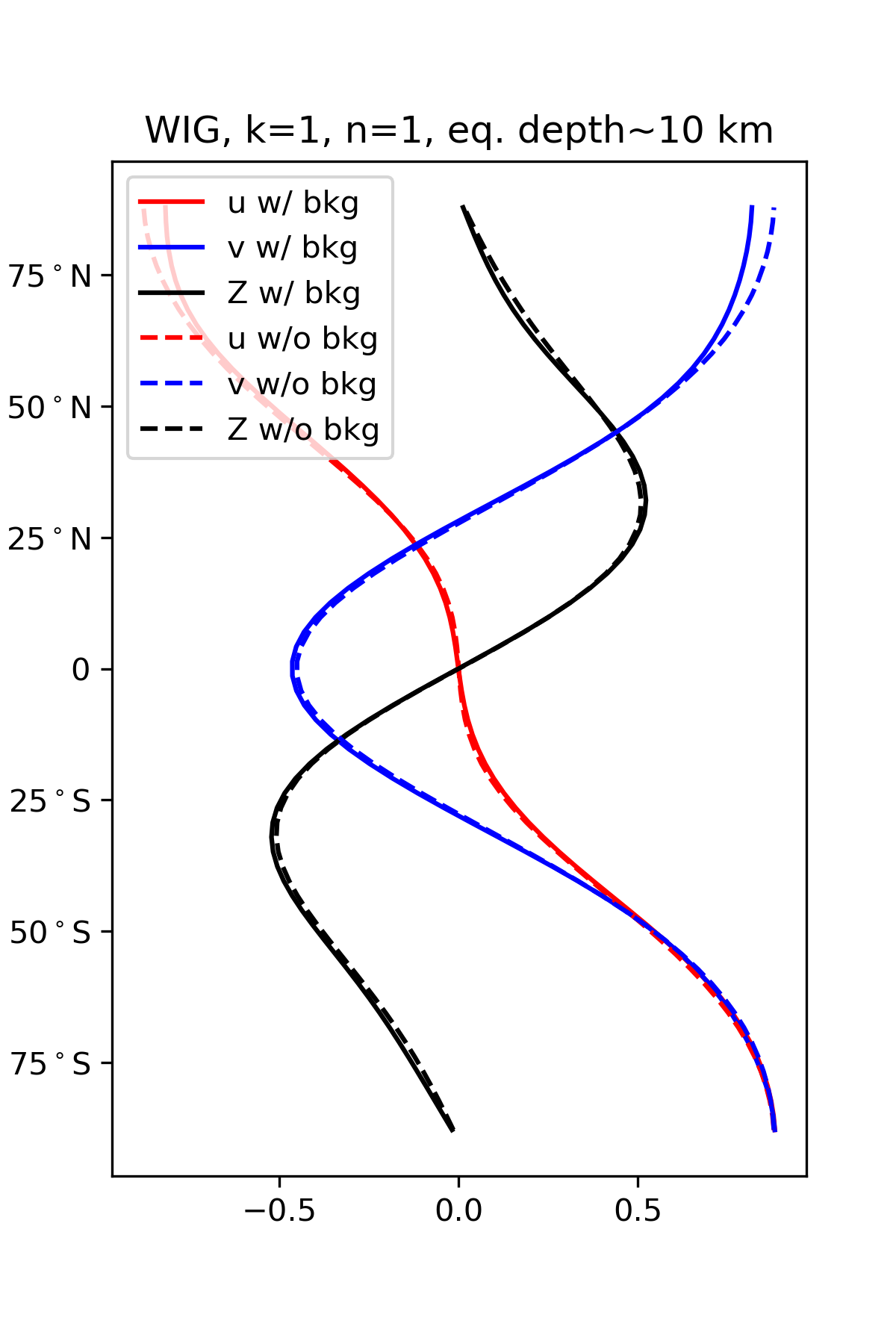
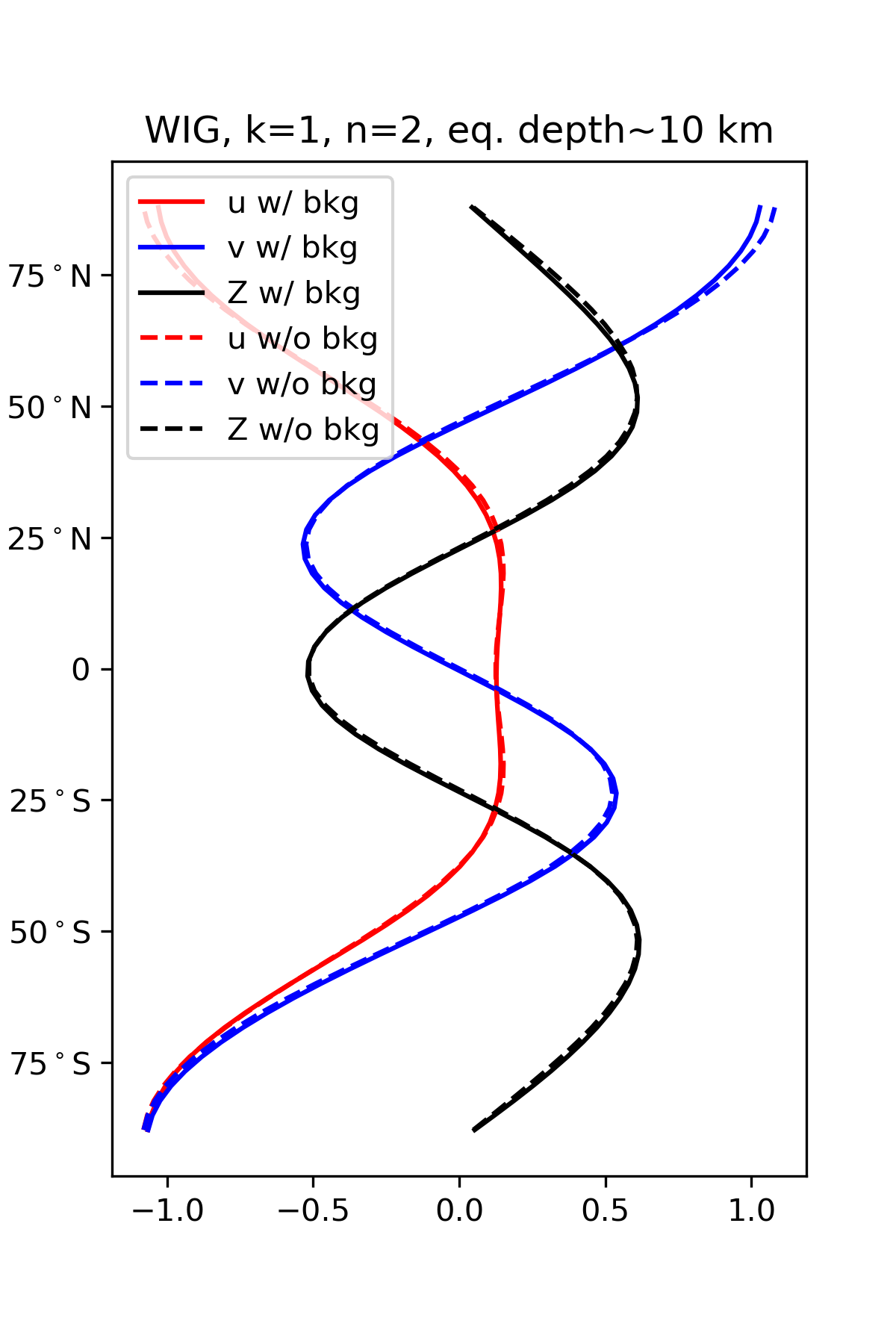
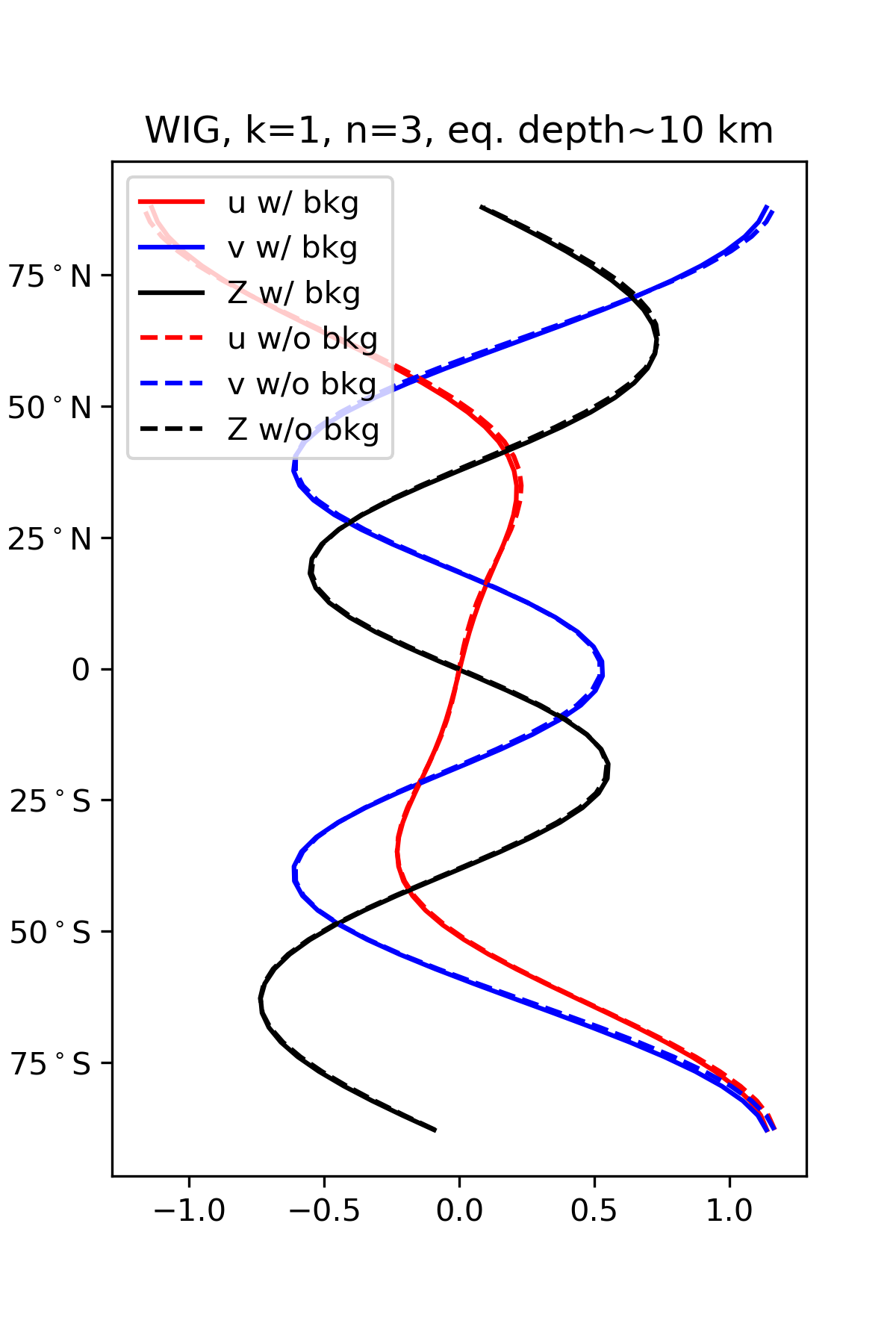
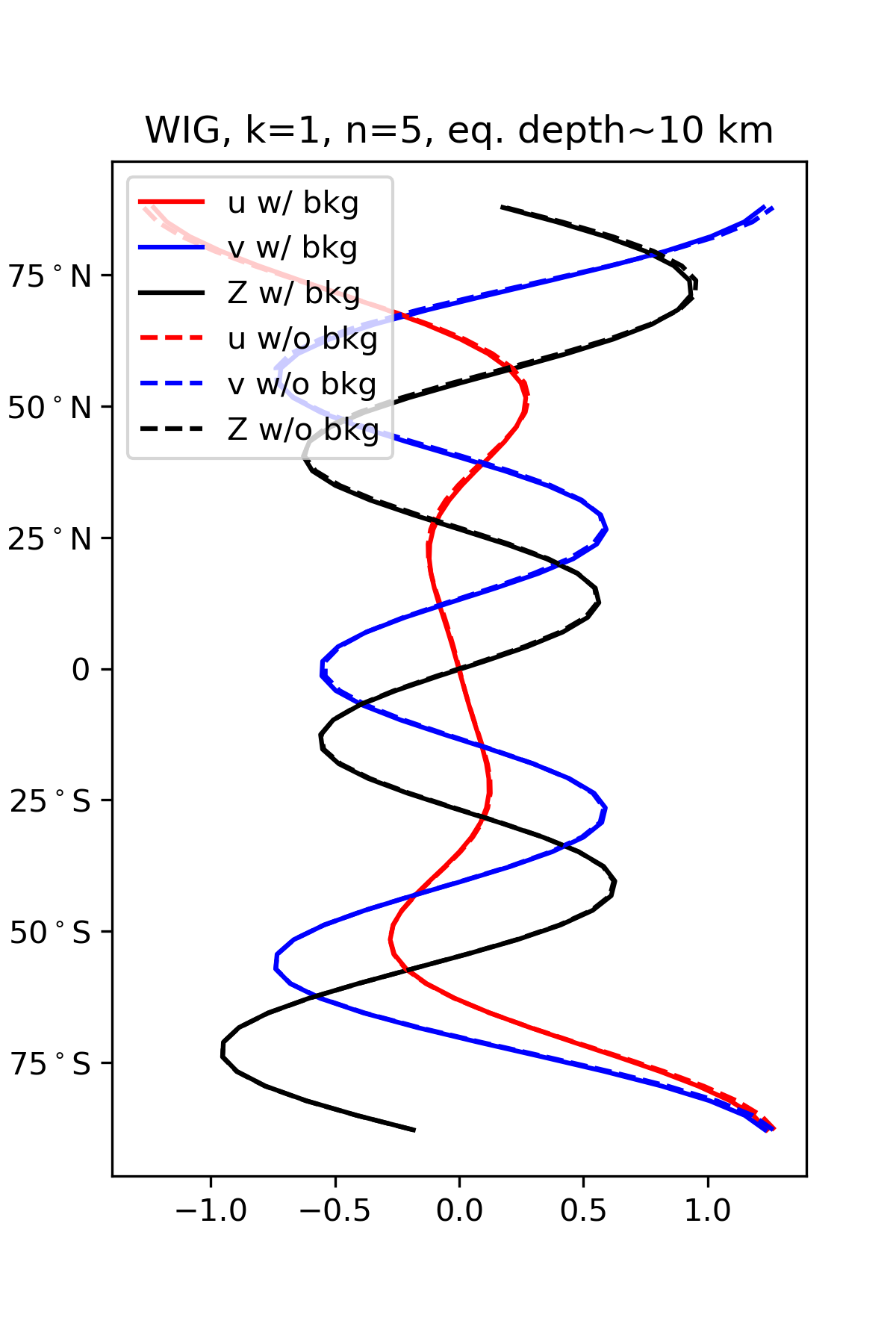
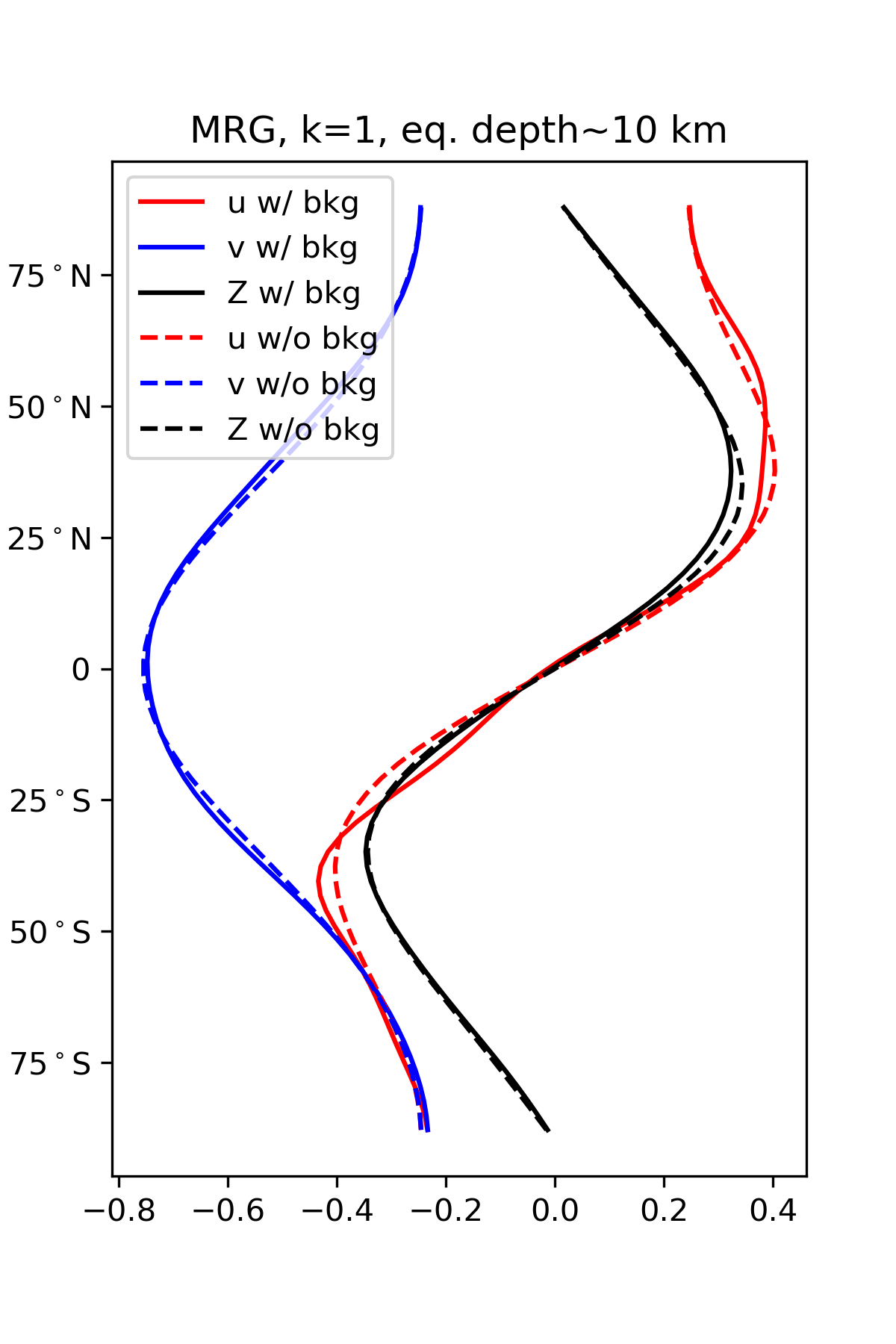
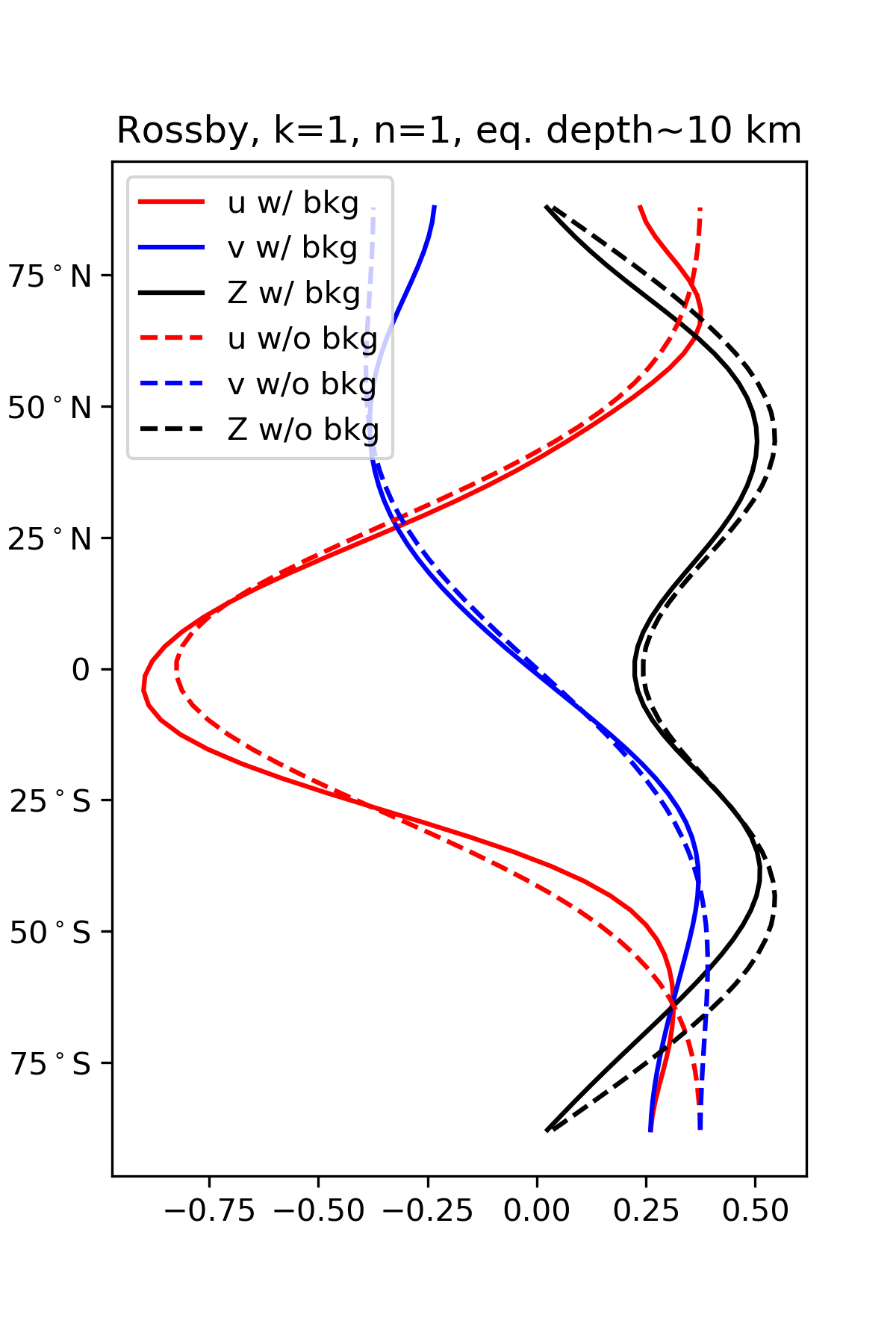
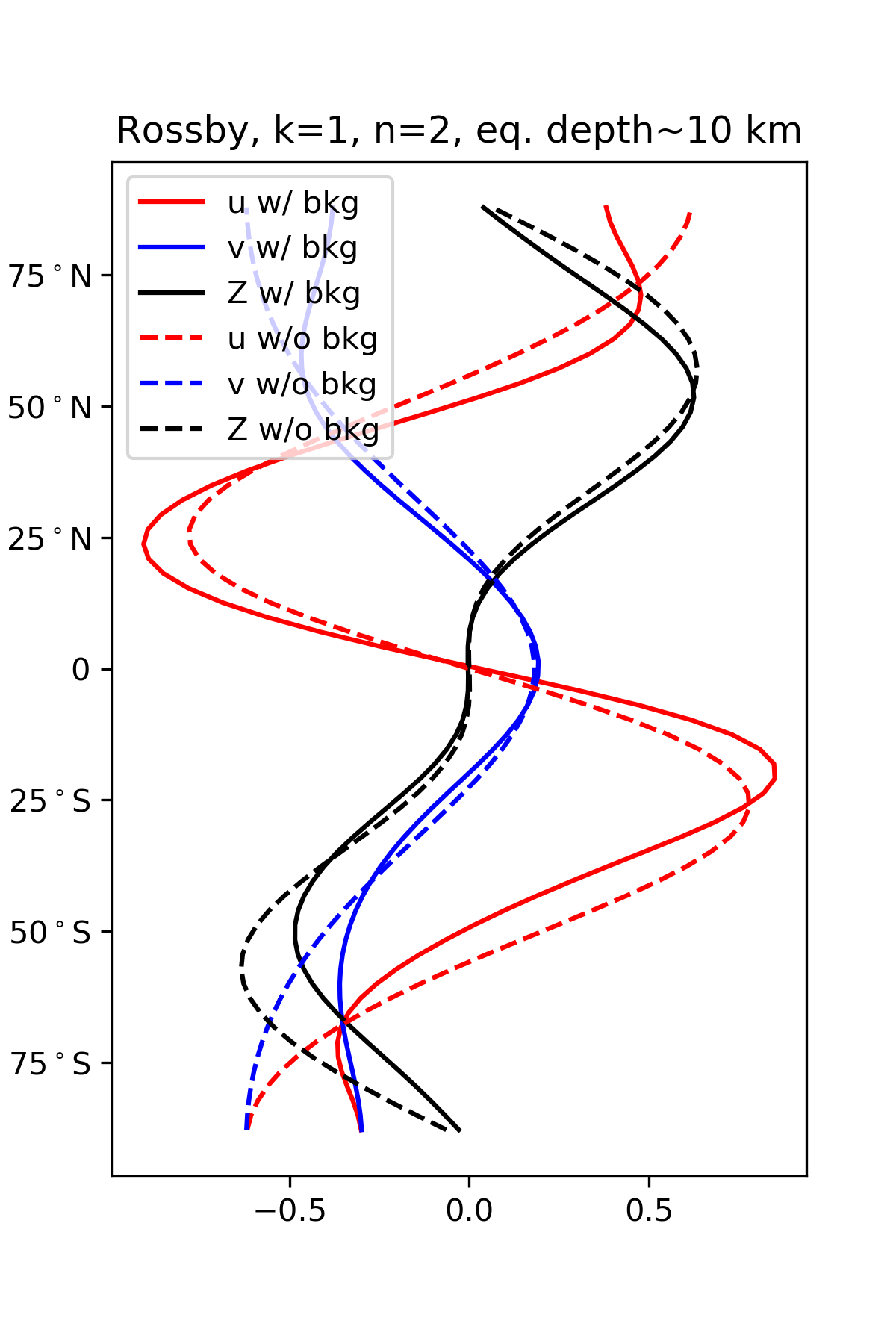
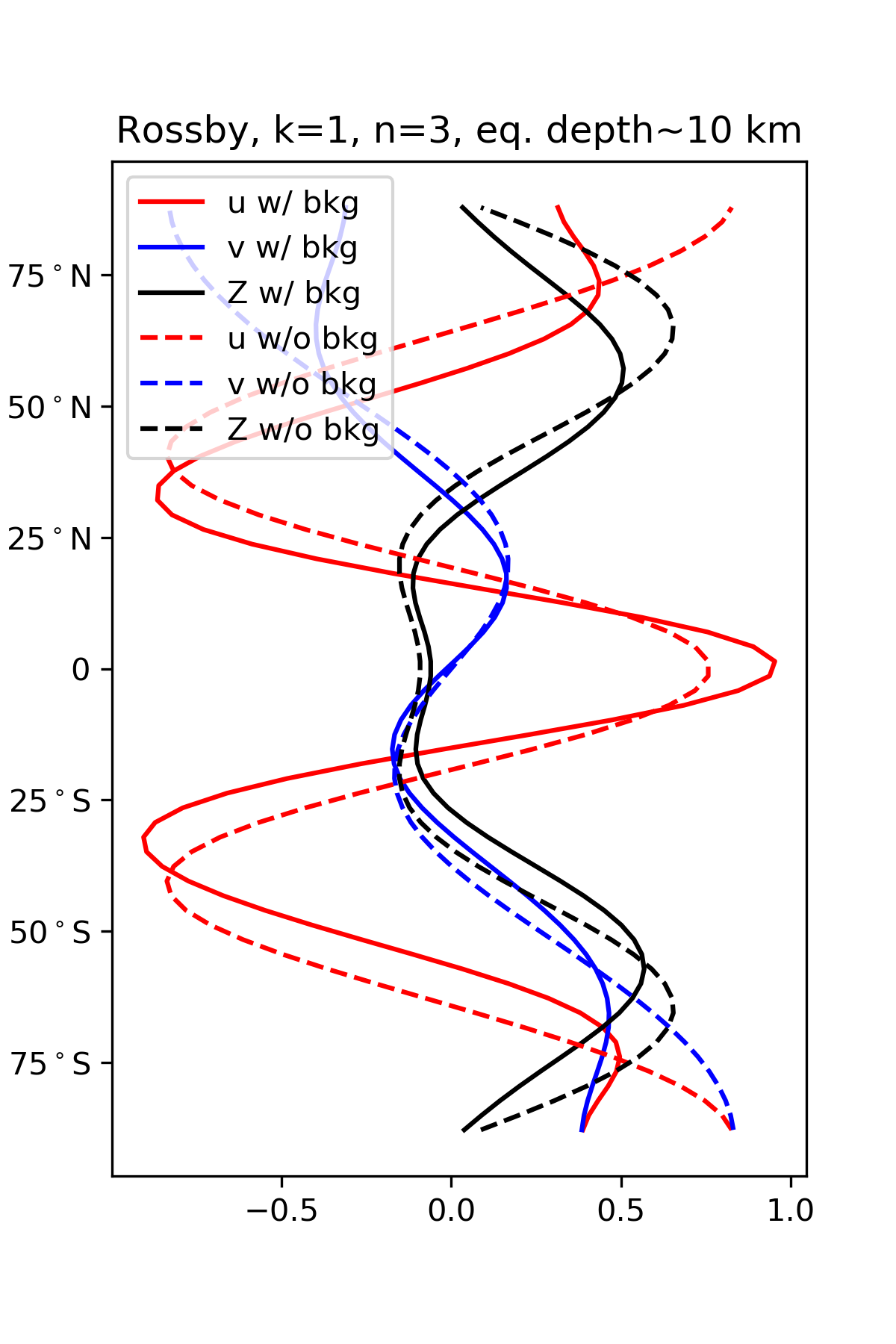
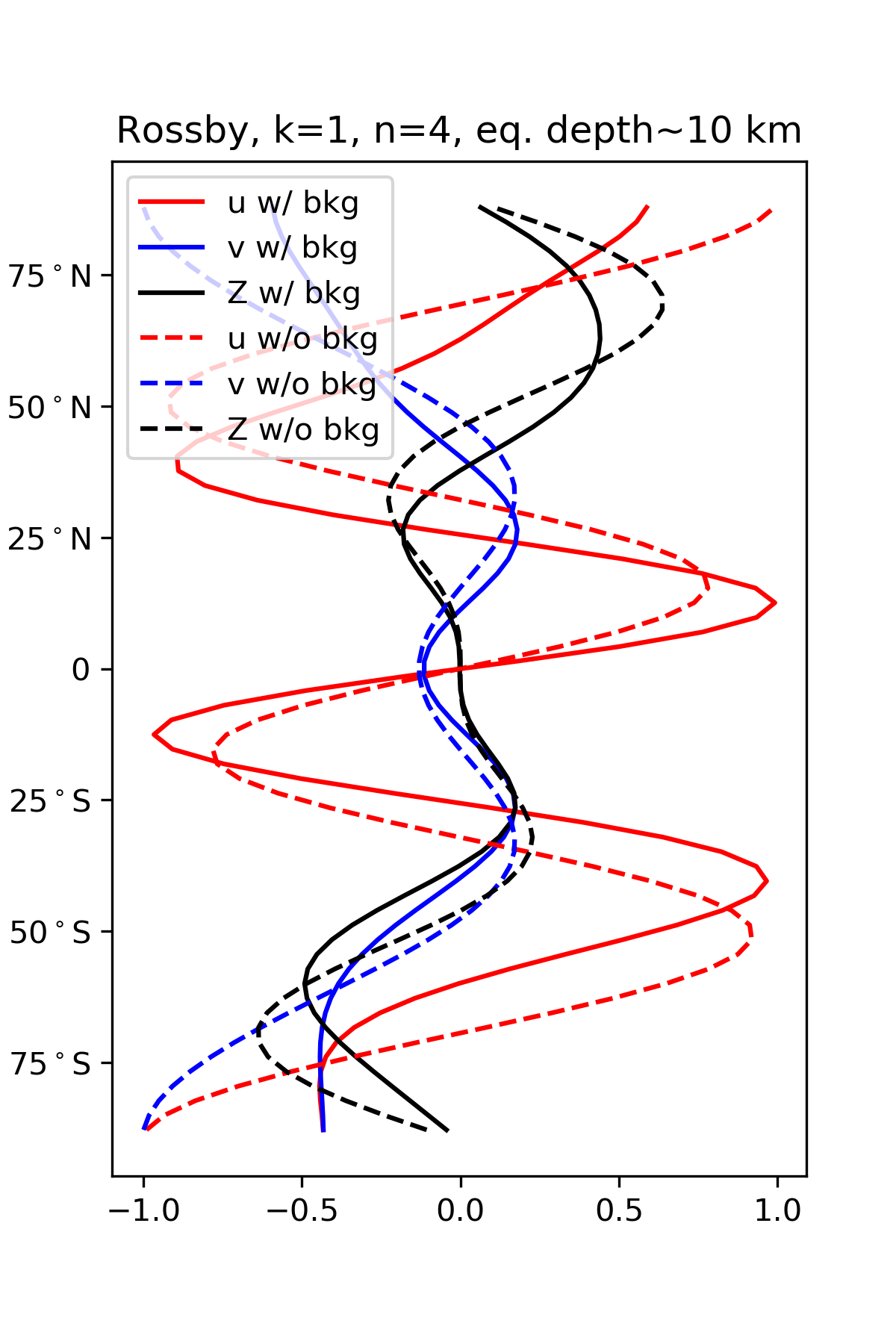
The Effect of the Background Flow on the Meridional Structure of the Hough Functions
Meridional structures of the Hough functions for the zonal wavenumber k=1 and equivalent depth 10 km. The comparison is between the case of the background state at rest and the background flow derived from ERA5 reanalysis data for level 500 hPa and MAM season.
These results reproduce ones from Kasahara, A., 1981: Corrigendum, J. Atmos. Sci. 38, 2284-2285. The original article is Kasahara, A., 1980: Effect of Zonal Flows on the Free Oscillations of a Barotropic Atmosphere, J. Atmos. Sci. 37, 917-929.
 |
||
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
Hough Harmonics
These and further Hough harmonics are available for two projections:
https://modes.cen.uni-hamburg.de/ebla/MODES/Robinson and
https://modes.cen.uni-hamburg.de/ebla/MODES/EquidistantCylindrical
Feel free to use them. Please acknowledge the MODES project webpage.
|
Kelvin Waves |
|
|---|---|
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
|
Mixed Rossby-gravity Waves |
|
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
|
Eastward-propagating Inertia-gravity Waves |
|
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
|
Rossby Waves |
|
 |
 |
 |
 |
 |
 |
 |
 |
 |
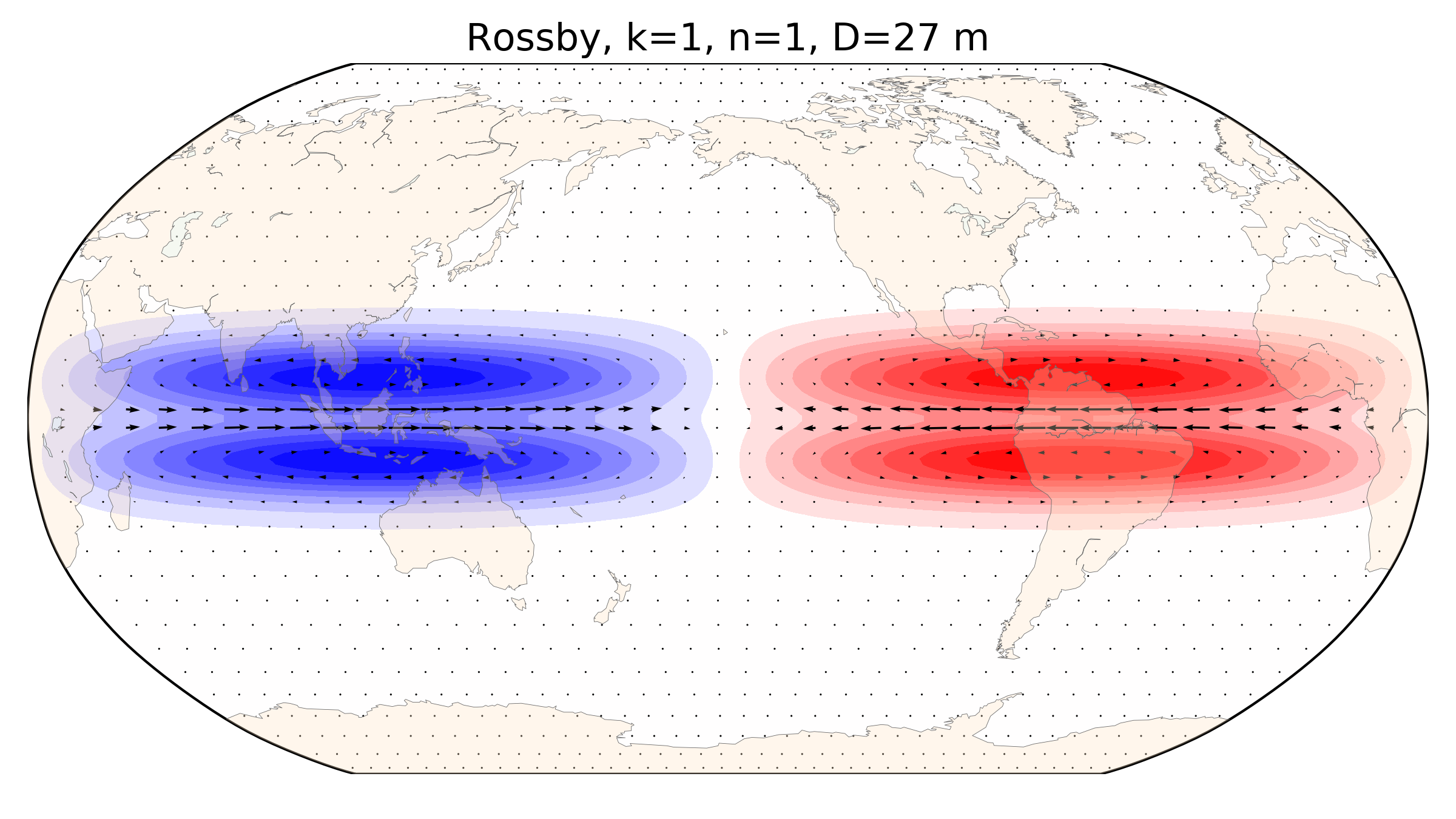 |
 |
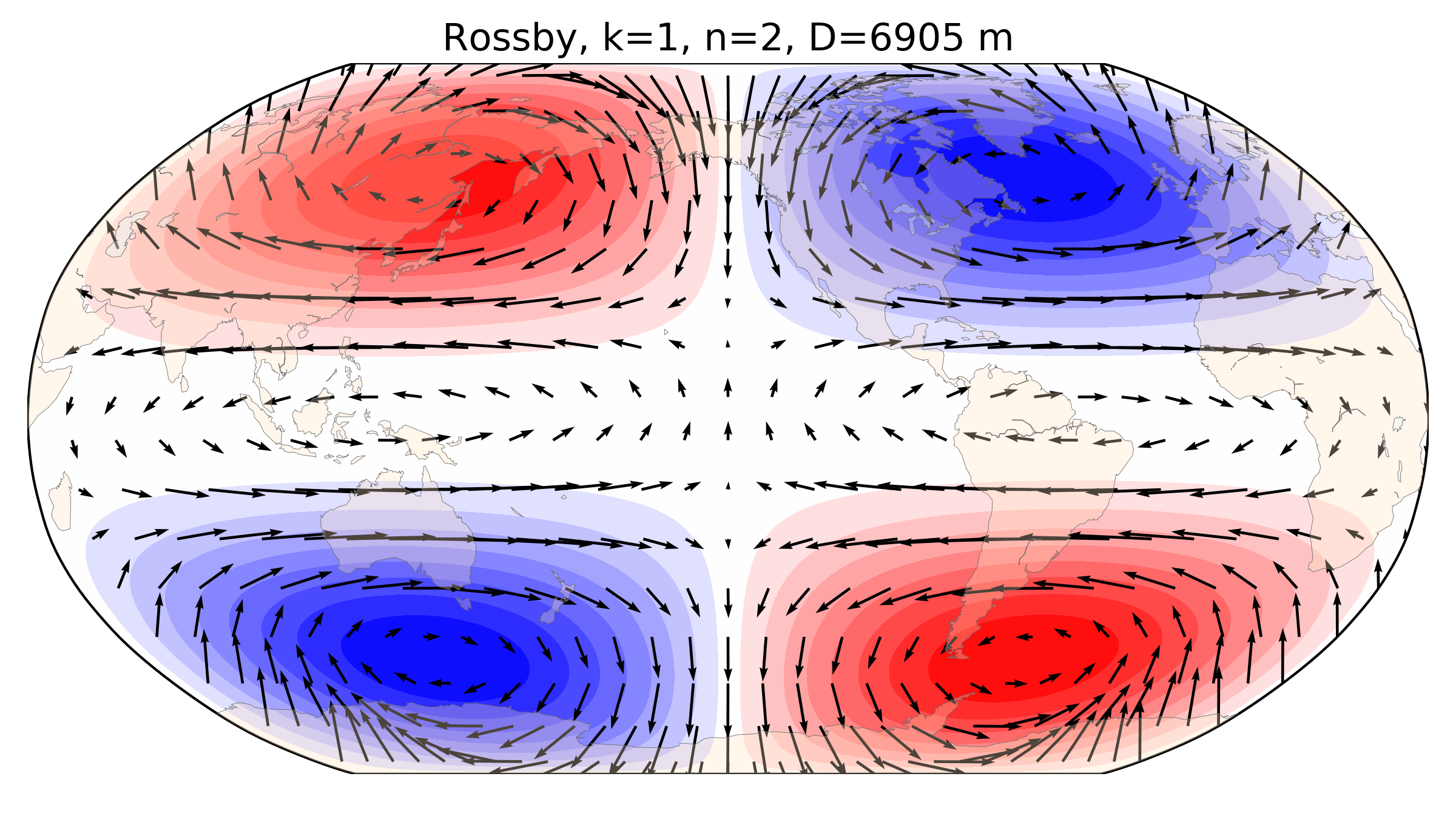 |
 |
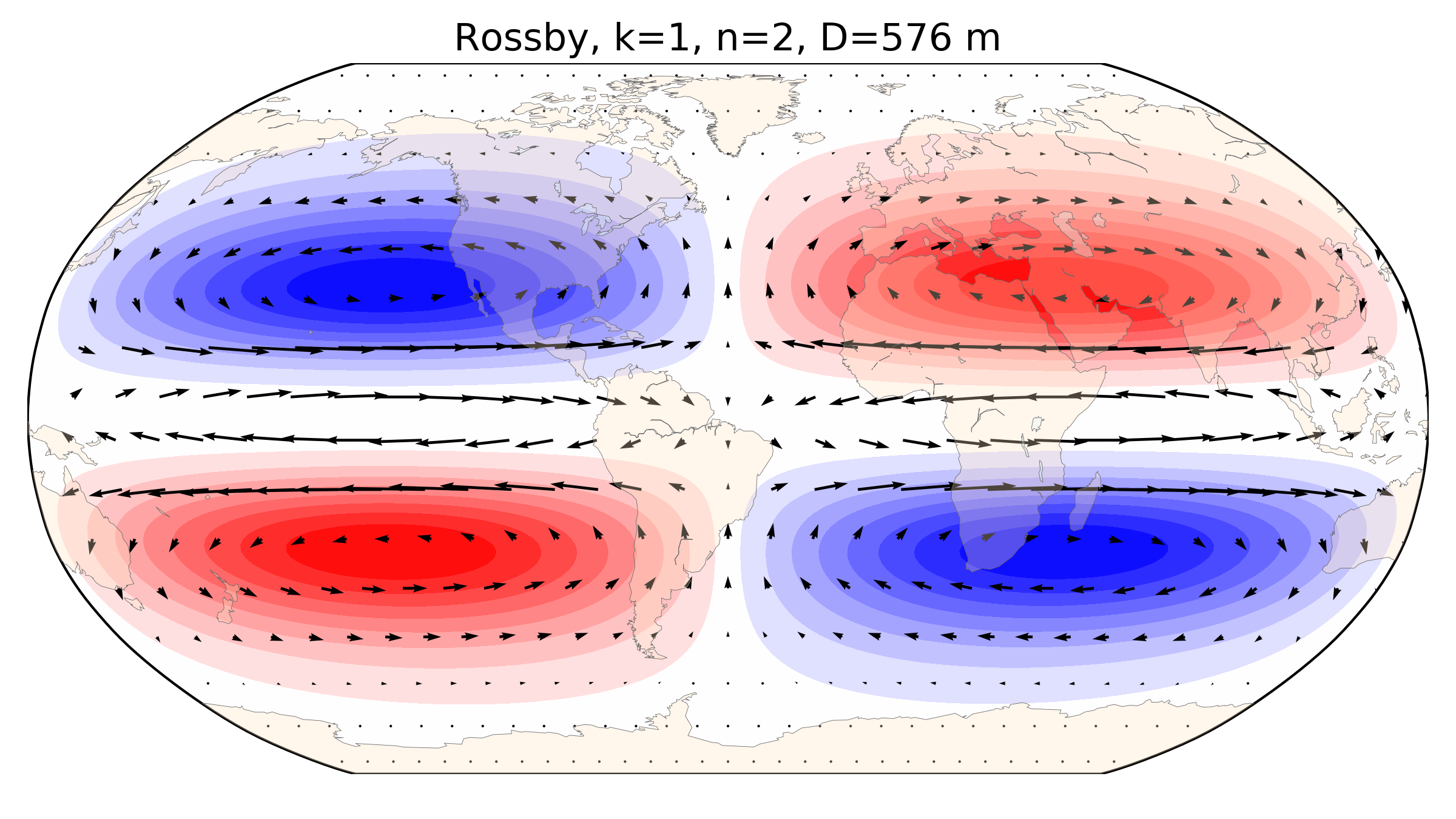 |
 |
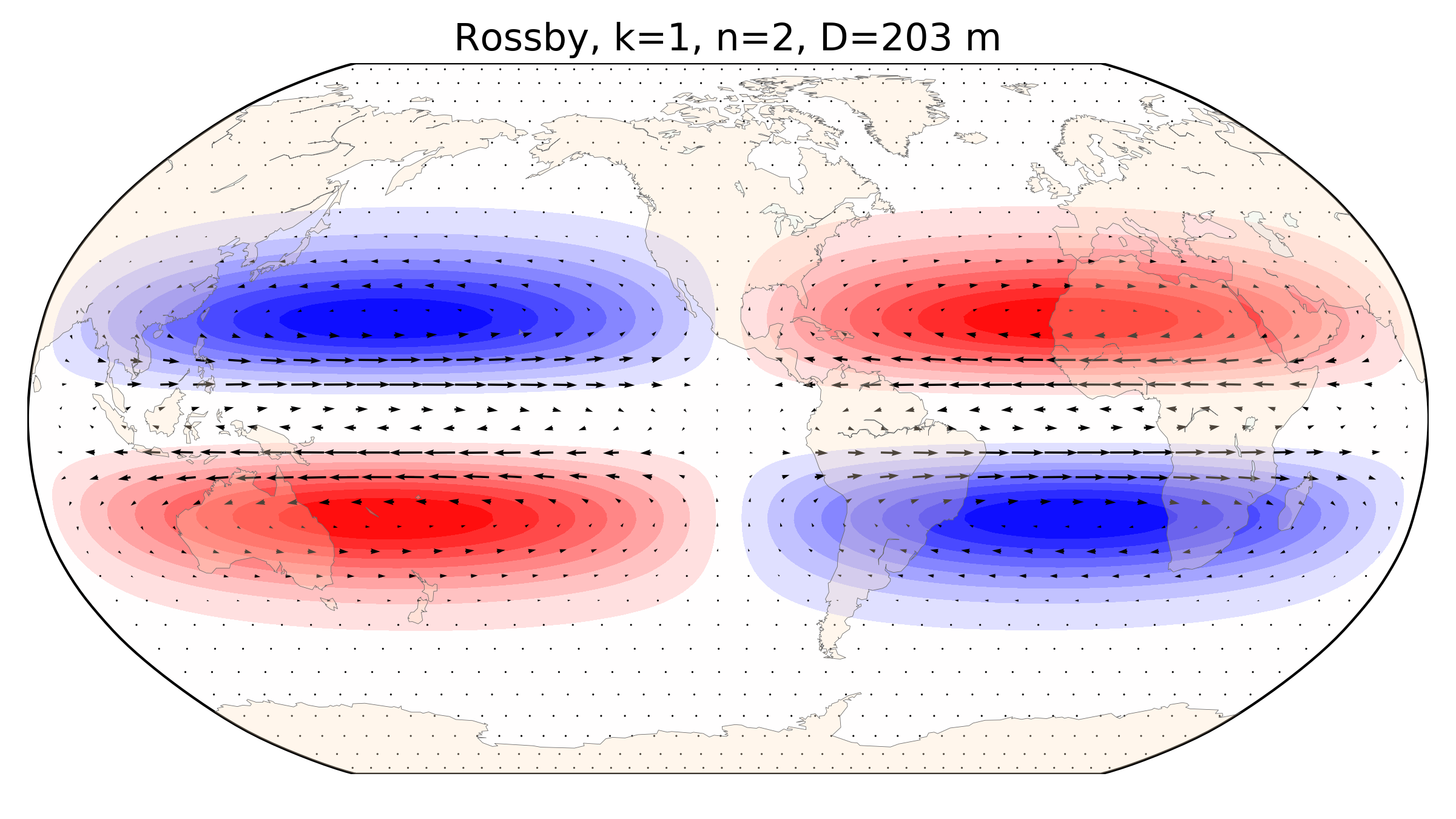 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
|
Westward-propagating Inertia-gravity Waves |
|
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
